No CrossRef data available.
Article contents
EMBEDDING OF METRIC GRAPHS ON HYPERBOLIC SURFACES
Published online by Cambridge University Press: 13 February 2019
Abstract
An embedding of a metric graph 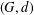 $(G,d)$ on a closed hyperbolic surface is essential if each complementary region has a negative Euler characteristic. We show, by construction, that given any metric graph, its metric can be rescaled so that it admits an essential and isometric embedding on a closed hyperbolic surface. The essential genus
$(G,d)$ on a closed hyperbolic surface is essential if each complementary region has a negative Euler characteristic. We show, by construction, that given any metric graph, its metric can be rescaled so that it admits an essential and isometric embedding on a closed hyperbolic surface. The essential genus 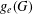 $g_{e}(G)$ of
$g_{e}(G)$ of 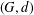 $(G,d)$ is the lowest genus of a surface on which such an embedding is possible. We establish a formula to compute
$(G,d)$ is the lowest genus of a surface on which such an embedding is possible. We establish a formula to compute 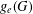 $g_{e}(G)$ and show that, for every integer
$g_{e}(G)$ and show that, for every integer 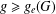 $g\geq g_{e}(G)$, there is an embedding of
$g\geq g_{e}(G)$, there is an embedding of  $(G,d)$ (possibly after a rescaling of
$(G,d)$ (possibly after a rescaling of  $d$) on a surface of genus
$d$) on a surface of genus 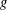 $g$. Next, we study minimal embeddings where each complementary region has Euler characteristic
$g$. Next, we study minimal embeddings where each complementary region has Euler characteristic  $-1$. The maximum essential genus
$-1$. The maximum essential genus  $g_{e}^{\max }(G)$ of
$g_{e}^{\max }(G)$ of 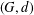 $(G,d)$ is the largest genus of a surface on which the graph is minimally embedded. We describe a method for an essential embedding of
$(G,d)$ is the largest genus of a surface on which the graph is minimally embedded. We describe a method for an essential embedding of  $(G,d)$, where
$(G,d)$, where 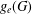 $g_{e}(G)$ and
$g_{e}(G)$ and  $g_{e}^{\max }(G)$ are realised.
$g_{e}^{\max }(G)$ are realised.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © 2019 Australian Mathematical Publishing Association Inc.
Footnotes
The author has been supported by a Post Doctoral Fellowship funded by a J. C. Bose fellowship of Professor Mahan Mj.

