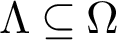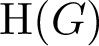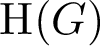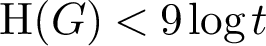2 results
ON THE HEIGHT AND RELATIONAL COMPLEXITY OF A FINITE PERMUTATION GROUP
- Part of
-
- Journal:
- Nagoya Mathematical Journal / Volume 246 / June 2022
- Published online by Cambridge University Press:
- 13 July 2021, pp. 372-411
- Print publication:
- June 2022
-
- Article
- Export citation
Institutional Linkages with the State and Organizational Practices in Corporate Social Responsibility: Evidence from China
-
- Journal:
- Management and Organization Review / Volume 13 / Issue 3 / September 2017
- Published online by Cambridge University Press:
- 07 August 2017, pp. 539-573
-
- Article
- Export citation