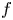3 results
8 - Dissipative Interactions and Decoherence
-
- Book:
- Introductory Quantum Optics
- Published online:
- 14 December 2023
- Print publication:
- 30 November 2023, pp 257-279
-
- Chapter
- Export citation
G - Interaction between Light and Atoms
-
- Book:
- Quantum Processes and Measurement
- Published online:
- 27 July 2023
- Print publication:
- 10 August 2023, pp 240-251
-
- Chapter
- Export citation
Borderline gradient continuity for fractional heat type operators
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 153 / Issue 5 / October 2023
- Published online by Cambridge University Press:
- 14 October 2022, pp. 1651-1682
- Print publication:
- October 2023
-
- Article
- Export citation