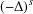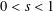9 results
8 - Introduction to Optimisation
-
- Book:
- Mathematics for Economics and Finance
- Published online:
- 24 May 2024
- Print publication:
- 30 May 2024, pp 73-84
-
- Chapter
- Export citation
Gradient estimates for the constant mean curvature equation in hyperbolic space
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 150 / Issue 6 / December 2020
- Published online by Cambridge University Press:
- 09 December 2019, pp. 3216-3230
- Print publication:
- December 2020
-
- Article
- Export citation
INFINITELY MANY SOLUTIONS FOR NONLOCAL SYSTEMS INVOLVING FRACTIONAL LAPLACIAN UNDER NONCOMPACT SETTINGS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 107 / Issue 2 / October 2019
- Published online by Cambridge University Press:
- 21 December 2018, pp. 215-233
- Print publication:
- October 2019
-
- Article
-
- You have access
- Export citation
GLOBAL ANALYSIS OF ONE-DIMENSIONAL VARIATIONAL PROBLEMS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 95 / Issue 1 / February 2017
- Published online by Cambridge University Press:
- 28 November 2016, pp. 167-168
- Print publication:
- February 2017
-
- Article
-
- You have access
- Export citation
Sequences of Weak Solutions for Non-Local Elliptic Problems with Dirichlet Boundary Condition
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 57 / Issue 3 / October 2014
- Published online by Cambridge University Press:
- 16 April 2014, pp. 779-809
-
- Article
-
- You have access
- Export citation
Étanchéités statiques des écoulements de filtration auvoisinage du point critique
-
- Journal:
- Mechanics & Industry / Volume 12 / Issue 2 / 2011
- Published online by Cambridge University Press:
- 12 April 2011, pp. 123-130
- Print publication:
- 2011
-
- Article
- Export citation
Multiplicity theorems for semilinear elliptic problems depending on a parameter
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 52 / Issue 1 / February 2009
- Published online by Cambridge University Press:
- 02 February 2009, pp. 171-180
-
- Article
-
- You have access
- Export citation
Crise d'ébullition : inhibition du détachement de la bulle de vapeur par la force de recul
-
- Journal:
- Mechanics & Industry / Volume 5 / Issue 5 / September 2004
- Published online by Cambridge University Press:
- 19 November 2004, pp. 553-558
- Print publication:
- September 2004
-
- Article
- Export citation
Phase transitions of some non-linear stochastic models
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 32 / Issue 1 / March 1995
- Published online by Cambridge University Press:
- 14 July 2016, pp. 193-201
- Print publication:
- March 1995
-
- Article
- Export citation