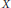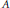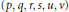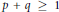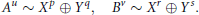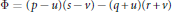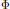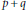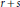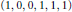We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let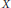 
$X$ and 
$Y$ be Banach spaces isomorphic to complemented subspaces of each other with supplements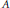 
$A$ and 
$B$. In 1996, W. T. Gowers solved the Schroeder–Bernstein (or Cantor–Bernstein) problem for Banach spaces by showing that 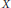$X$ is not necessarily isomorphic to $Y$. In this paper, we obtain a necessary and sufficient condition on the sextuples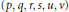 
$\left( p,\,q,\,r,\,s,\,u,\,v \right)$ in 
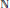$\mathbb{N}$ with 
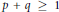$p\,+\,q\,\ge \,1$, 
$r+s\ge 1$ and 
$u,\,v\,\in \,{{\mathbb{N}}^{*}}$, to provide that 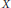$X$ is isomorphic to $Y$, whenever these spaces satisfy the following decomposition scheme
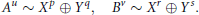$${{A}^{u}}\,\sim \,{{X}^{p}}\,\oplus \,{{Y}^{q}},\,{{B}^{v}}\,\sim \,{{X}^{r}}\,\oplus \,{{Y}^{s}}.$$
Namely,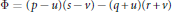 
$\Phi \,=\,\left( p\,-\,u \right)\left( s\,-\,v \right)-\left( q\,+\,u \right)\left( r\,+\,v \right)$ is different from zero and 
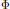$\Phi $ divides 
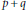$p\,+\,q$ and 
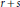$r\,+\,s$. These sextuples are called Cantor–Bernstein sextuples for Banach spaces. The simplest case 
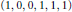$\left( 1,0,0,1,1,1 \right)$ indicates the well-known Pełczyński's decomposition method in Banach space. On the other hand, by interchanging some Banach spaces in the above decomposition scheme, refinements of the Schroeder– Bernstein problem become evident.
