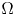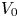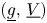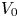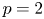On the optimization of the first weighted eigenvalue
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 153 / Issue 6 / December 2023
- Published online by Cambridge University Press:
- 12 September 2022, pp. 1777-1804
- Print publication:
- December 2023
-
- Article
- Export citation
-
For N\geq 2, a bounded smooth domain \Omega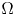 in \mathbb {R}^{N}, and g_0,\, V_0 \in L^{1}_{loc}(\Omega ), we study the optimization of the first eigenvalue for the following weighted eigenvalue problem:
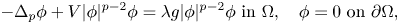
-\Delta_p \phi + V |\phi|^{p-2}\phi = \lambda g |\phi|^{p-2}\phi \text{ in } \Omega, \quad \phi=0 \text{ on } \partial \Omega,where g and V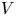 vary over the rearrangement classes of g_0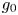 and V_0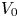, respectively. We prove the existence of a minimizing pair (\underline {g},\,\underline {V})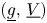 and a maximizing pair (\overline {g},\,\overline {V}) for g_0 and V_0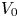 lying in certain Lebesgue spaces. We obtain various qualitative properties such as polarization invariance, Steiner symmetry of the minimizers as well as the associated eigenfunctions for the case p=2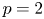. For annular domains, we prove that the minimizers and the corresponding eigenfunctions possess the foliated Schwarz symmetry.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
