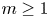Refine search
Actions for selected content:
48 results
Part II - Separation Theorem, Extreme Points, Recessive Directions, and Geometry of Polyhedral Sets
-
- Book:
- Essential Mathematics for Convex Optimization
- Published online:
- 22 October 2025
- Print publication:
- 26 June 2025, pp 81-150
-
- Chapter
- Export citation
4 - Majorization
- from Part II - Tools and Methods
-
- Book:
- Quantum Resource Theories
- Published online:
- 03 May 2025
- Print publication:
- 10 April 2025, pp 135-207
-
- Chapter
- Export citation
12 - Pure-State Entanglement
- from Part IV - Entanglement Theory
-
- Book:
- Quantum Resource Theories
- Published online:
- 03 May 2025
- Print publication:
- 10 April 2025, pp 507-537
-
- Chapter
- Export citation
Robust Canonical Discriminant Analysis
-
- Journal:
- Psychometrika / Volume 59 / Issue 4 / December 1994
- Published online by Cambridge University Press:
- 01 January 2025, pp. 485-507
-
- Article
- Export citation
Weighted Least Squares Fitting Using Ordinary Least Squares Algorithms
-
- Journal:
- Psychometrika / Volume 62 / Issue 2 / June 1997
- Published online by Cambridge University Press:
- 01 January 2025, pp. 251-266
-
- Article
- Export citation
A Generalization of Takane's Algorithm for Dedicom
-
- Journal:
- Psychometrika / Volume 55 / Issue 1 / March 1990
- Published online by Cambridge University Press:
- 01 January 2025, pp. 151-158
-
- Article
- Export citation
The Integration of Multidimensional Scaling and Multivariate Analysis with Optimal Transformations
-
- Journal:
- Psychometrika / Volume 57 / Issue 4 / December 1992
- Published online by Cambridge University Press:
- 01 January 2025, pp. 539-565
-
- Article
- Export citation
Minimization of a Class of Matrix Trace Functions by means of Refined Majorization
-
- Journal:
- Psychometrika / Volume 57 / Issue 3 / September 1992
- Published online by Cambridge University Press:
- 01 January 2025, pp. 371-382
-
- Article
- Export citation
Majorization as a Tool for Optimizing a Class of Matrix Functions
-
- Journal:
- Psychometrika / Volume 55 / Issue 3 / September 1990
- Published online by Cambridge University Press:
- 01 January 2025, pp. 417-428
-
- Article
- Export citation
A Monotonically Convergent Algorithm for Orthogonal Congruence Rotation
-
- Journal:
- Psychometrika / Volume 61 / Issue 2 / June 1996
- Published online by Cambridge University Press:
- 01 January 2025, pp. 375-389
-
- Article
- Export citation
A Monotonically Convergent Algorithm for Factals
-
- Journal:
- Psychometrika / Volume 58 / Issue 4 / December 1993
- Published online by Cambridge University Press:
- 01 January 2025, pp. 567-574
-
- Article
- Export citation
Stochastic comparison on active redundancy allocation to K-out-of-N systems with statistically dependent component and redundancy lifetimes
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 55 / Issue 4 / December 2023
- Published online by Cambridge University Press:
- 05 May 2023, pp. 1085-1115
- Print publication:
- December 2023
-
- Article
- Export citation
Optimal allocation of policy limits in layer reinsurance treaties
- Part of
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 2 / April 2023
- Published online by Cambridge University Press:
- 24 November 2022, pp. 546-566
-
- Article
- Export citation
8 - More on the Lieb–Thirring Constants
- from Part Three - Sharp Constants in Lieb–Thirring Inequalities
-
- Book:
- Schrödinger Operators: Eigenvalues and Lieb–Thirring Inequalities
- Published online:
- 03 November 2022
- Print publication:
- 17 November 2022, pp 451-470
-
- Chapter
- Export citation
On stochastic ordering among extreme shock models
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 4 / October 2023
- Published online by Cambridge University Press:
- 28 October 2022, pp. 961-972
-
- Article
- Export citation
Dynamical fitness models: evidence of universality classes for preferential attachment graphs
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 59 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 21 June 2022, pp. 609-630
- Print publication:
- September 2022
-
- Article
- Export citation
On transform orders for largest claim amounts
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 58 / Issue 4 / December 2021
- Published online by Cambridge University Press:
- 22 November 2021, pp. 1064-1085
- Print publication:
- December 2021
-
- Article
- Export citation
Stochastic properties of generalized finite α-mixtures
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 36 / Issue 4 / October 2022
- Published online by Cambridge University Press:
- 15 July 2021, pp. 1055-1079
-
- Article
-
- You have access
- HTML
- Export citation
Derivatives of symplectic eigenvalues and a Lidskii type theorem
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 74 / Issue 2 / April 2022
- Published online by Cambridge University Press:
- 02 December 2020, pp. 457-485
- Print publication:
- April 2022
-
- Article
-
- You have access
- Export citation
STOCHASTIC ORDERING OF LIFETIMES OF PARALLEL AND SERIES SYSTEMS COMPRISING HETEROGENEOUS DEPENDENT COMPONENTS WITH GENERALIZED BIRNBAUM-SAUNDERS DISTRIBUTIONS
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 36 / Issue 1 / January 2022
- Published online by Cambridge University Press:
- 25 August 2020, pp. 49-65
-
- Article
- Export citation