5 results
A mass transference principle for systems of linear forms and its applications
- Part of
-
- Journal:
- Compositio Mathematica / Volume 154 / Issue 5 / May 2018
- Published online by Cambridge University Press:
- 03 April 2018, pp. 1014-1047
- Print publication:
- May 2018
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
SUBGROUPS OF FRACTIONAL DIMENSION IN NILPOTENT OR SOLVABLE LIE GROUPS
- Part of
-
- Journal:
- Mathematika / Volume 59 / Issue 2 / July 2013
- Published online by Cambridge University Press:
- 23 May 2013, pp. 497-511
- Print publication:
- July 2013
-
- Article
- Export citation
ON THE DIOPHANTINE PROPERTIES OF λ-EXPANSIONS
- Part of
-
- Journal:
- Mathematika / Volume 59 / Issue 1 / January 2013
- Published online by Cambridge University Press:
- 05 December 2012, pp. 65-86
- Print publication:
- January 2013
-
- Article
- Export citation
Hausdorff dimensions of self-similar and self-affine fractals in the Heisenberg group
-
- Journal:
- Proceedings of the London Mathematical Society / Volume 91 / Issue 1 / July 2005
- Published online by Cambridge University Press:
- 22 June 2005, pp. 153-183
- Print publication:
- July 2005
-
- Article
- Export citation
HAUSDORFF AND PACKING MEASURE OF SETS OF GENERIC POINTS: A ZERO-INFINITY LAW
-
- Journal:
- Journal of the London Mathematical Society / Volume 69 / Issue 2 / April 2004
- Published online by Cambridge University Press:
- 29 March 2004, pp. 383-406
- Print publication:
- April 2004
-
- Article
- Export citation

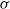
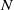
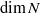
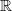
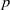
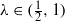 and
and 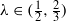 , the upper bound of
, the upper bound of 