Refine search
Actions for selected content:
11 results
Equivalences of stable categories of Gorenstein local rings
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 68 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 08 January 2025, pp. 338-348
- Print publication:
- March 2025
-
- Article
- Export citation
A power structure over the Grothendieck ring of geometric dg categories
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 68 / Issue 1 / February 2025
- Published online by Cambridge University Press:
- 05 December 2024, pp. 300-318
-
- Article
- Export citation
Persistence and the Sheaf-Function Correspondence
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 11 / 2023
- Published online by Cambridge University Press:
- 18 December 2023, e113
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
ISOMORPHISMS UP TO BOUNDED TORSION BETWEEN RELATIVE
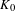 $K_{0}$-GROUPS AND CHOW GROUPS WITH MODULUS
$K_{0}$-GROUPS AND CHOW GROUPS WITH MODULUS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 20 / Issue 6 / November 2021
- Published online by Cambridge University Press:
- 18 March 2020, pp. 1947-1968
- Print publication:
- November 2021
-
- Article
-
- You have access
- Open access
- Export citation
ON THE WEAK GROTHENDIECK GROUP OF A BEZOUT RING
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 58 / Issue 3 / September 2016
- Published online by Cambridge University Press:
- 21 July 2015, pp. 617-635
- Print publication:
- September 2016
-
- Article
-
- You have access
- Export citation
Hochster's theta pairing and numerical equivalence
-
- Journal:
- Journal of K-Theory / Volume 14 / Issue 3 / December 2014
- Published online by Cambridge University Press:
- 28 July 2014, pp. 495-525
- Print publication:
- December 2014
-
- Article
- Export citation
A Candidate for the Abelian Category of Mixed Elliptic Motives
-
- Journal:
- Journal of K-Theory / Volume 12 / Issue 3 / December 2013
- Published online by Cambridge University Press:
- 15 November 2013, pp. 569-600
- Print publication:
- December 2013
-
- Article
- Export citation
Hopfological algebra
- Part of
-
- Journal:
- Compositio Mathematica / Volume 150 / Issue 1 / January 2014
- Published online by Cambridge University Press:
- 07 October 2013, pp. 1-45
- Print publication:
- January 2014
-
- Article
- Export citation
K′-Theory of a Local Ring of Finite Cohen-Macaulay Type
-
- Journal:
- Journal of K-Theory / Volume 12 / Issue 3 / December 2013
- Published online by Cambridge University Press:
- 07 August 2013, pp. 405-432
- Print publication:
- December 2013
-
- Article
- Export citation
Quillen's solution of Serre's Problem
-
- Journal:
- Journal of K-Theory / Volume 11 / Issue 3 / June 2013
- Published online by Cambridge University Press:
- 11 March 2013, pp. 549-552
- Print publication:
- June 2013
-
- Article
- Export citation
ℤ[1/p]-motivic resolution of singularities
- Part of
-
- Journal:
- Compositio Mathematica / Volume 147 / Issue 5 / September 2011
- Published online by Cambridge University Press:
- 01 June 2011, pp. 1434-1446
- Print publication:
- September 2011
-
- Article
-
- You have access
- Export citation
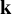
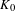
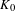
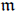 ) be a local hypersurface with an isolated singularity. We show that Hochster's theta pairing
) be a local hypersurface with an isolated singularity. We show that Hochster's theta pairing 