The Pólya-Vinogradov inequality states that for any non-principal character x modulo q and any N ≧ 1,
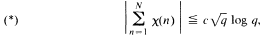
where c is an absolute constant. We show that (*) holds with c = 2/(3π2) + o(1) in the case x is primitive and x (— 1) =1 with c = 1/(3π) + o(l) in the case x is primitive and x(— 1) = — 1- This improves by a factor 2/3 the previously best-known values for these constants.