1 Introduction
Since the seminal work of Horton & Rogers (Reference Horton and Rogers1945) and Lapwood (Reference Lapwood1948), buoyancy-driven convection in a cell occupied by a porous medium has been adopted as a model for many applications. Some examples of these applications include oil recovery, ground water flow and geothermal energy extraction. Convection in porous media may be caused by heat or mass transfer. The key governing parameter is the Rayleigh–Darcy number (
![]() $Ra$
–
$Ra$
–
![]() $Da$
), which is more often referred to as the Rayleigh number (
$Da$
), which is more often referred to as the Rayleigh number (
![]() $Ra$
). It is a combination of a traditional Rayleigh number
$Ra$
). It is a combination of a traditional Rayleigh number
![]() $Ra_{f}$
and a Darcy number
$Ra_{f}$
and a Darcy number
![]() $Da$
. A traditional Rayleigh number describes the ratio of buoyancy to viscosity within a fluid, multiplied by the Prandtl number (for heat transfer) or Schmidt number (for mass transfer). A Darcy number describes the ratio between the permeability and the characteristic area. A porous medium produces two effects on convection. First, the largest length scale of fluid motions becomes limited by pore size, and thus heat/mass transfer is reduced (Jin et al.
Reference Jin, Uth, Kuznetsov and Herwig2015; Uth et al.
Reference Uth, Jin, Kuznetsov and Herwig2016; Jin & Kuznetsov Reference Jin and Kuznetsov2017). Second, the interaction between flow and porous elements may lead to dispersion processes within the pores, which can enhance heat/mass transfer (Nield & Bejan Reference Nield and Bejan2017).
$Da$
. A traditional Rayleigh number describes the ratio of buoyancy to viscosity within a fluid, multiplied by the Prandtl number (for heat transfer) or Schmidt number (for mass transfer). A Darcy number describes the ratio between the permeability and the characteristic area. A porous medium produces two effects on convection. First, the largest length scale of fluid motions becomes limited by pore size, and thus heat/mass transfer is reduced (Jin et al.
Reference Jin, Uth, Kuznetsov and Herwig2015; Uth et al.
Reference Uth, Jin, Kuznetsov and Herwig2016; Jin & Kuznetsov Reference Jin and Kuznetsov2017). Second, the interaction between flow and porous elements may lead to dispersion processes within the pores, which can enhance heat/mass transfer (Nield & Bejan Reference Nield and Bejan2017).
In recent years, natural convection in porous media has received increasing attention due to the interest in the long-term storage of CO2 in deep saline aquifers (Huppert & Neufeld Reference Huppert and Neufeld2014), where CO2 sequestration is driven by gradients of CO2 concentration. The efficiency of CO2 sequestration is determined by the Sherwood number (
![]() $Sh$
), which is the ratio of the total mass transfer rate (by convection and mass diffusion) to the diffusive mass transfer rate across a wall surface. Most reservoirs have small Rayleigh numbers (
$Sh$
), which is the ratio of the total mass transfer rate (by convection and mass diffusion) to the diffusive mass transfer rate across a wall surface. Most reservoirs have small Rayleigh numbers (
![]() $Ra<1500$
) (Hassanzadeh, Pooladi-Darvish & Keith Reference Hassanzadeh, Pooladi-Darvish and Keith2007), but some CO2 reservoirs have a thickness of several hundred metres, and are thus characterized by very high Rayleigh numbers (
$Ra<1500$
) (Hassanzadeh, Pooladi-Darvish & Keith Reference Hassanzadeh, Pooladi-Darvish and Keith2007), but some CO2 reservoirs have a thickness of several hundred metres, and are thus characterized by very high Rayleigh numbers (
![]() $Ra\approx 12\,000$
) (Neufeld et al.
Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010).
$Ra\approx 12\,000$
) (Neufeld et al.
Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010).
Many experimental, theoretical and numerical studies have been performed to determine the scaling
![]() $Sh=f(Ra)$
. Theoretical and numerical studies rely on the Darcy–Oberbeck–Boussinesq (DOB) equations (Nield & Bejan Reference Nield and Bejan2017), where the microscopic details of the porous media are solely accounted for by the permeability and effective diffusivity through
$Sh=f(Ra)$
. Theoretical and numerical studies rely on the Darcy–Oberbeck–Boussinesq (DOB) equations (Nield & Bejan Reference Nield and Bejan2017), where the microscopic details of the porous media are solely accounted for by the permeability and effective diffusivity through
![]() $Ra$
. This is explained by the loss of information during volume averaging.
$Ra$
. This is explained by the loss of information during volume averaging.
Convection in porous media can be classified into five regimes based on the Rayleigh number (Nield & Bejan Reference Nield and Bejan2017):
-
(I) the conducting regime (
 $0\leqslant Ra\leqslant 4\unicode[STIX]{x03C0}^{2}$
);
$0\leqslant Ra\leqslant 4\unicode[STIX]{x03C0}^{2}$
); -
(II) the steady state regime (
 $4\unicode[STIX]{x03C0}^{2}\leqslant Ra\leqslant 350$
);
$4\unicode[STIX]{x03C0}^{2}\leqslant Ra\leqslant 350$
); -
(III) the quasi-periodic regime, which is dominated by two coherent convecting cells (
 $350\leqslant Ra\leqslant 1300$
);
$350\leqslant Ra\leqslant 1300$
); -
(IV) the high Rayleigh regime (
 $1300\leqslant Ra\leqslant 10\,000$
), which is considered chaotic and ‘turbulent’ without large coherent structures; and
$1300\leqslant Ra\leqslant 10\,000$
), which is considered chaotic and ‘turbulent’ without large coherent structures; and -
(V) the ultimate Rayleigh regime (
 $Ra\geqslant 10\,000$
), which differs from the high Rayleigh regime only by the increasing self-organization of the inner flow field.
$Ra\geqslant 10\,000$
), which differs from the high Rayleigh regime only by the increasing self-organization of the inner flow field.
Howard (Reference Howard and Görtler1964) suggested a linear scaling of
![]() $Sh$
versus
$Sh$
versus
![]() $Ra$
at asymptotically large Rayleigh numbers. This was later proven in the analytical study of Doering & Constantin (Reference Doering and Constantin1998). With the advent of high-performance computing, several numerical studies of the DOB equations (herein DOB simulations) for large Rayleigh numbers have been performed. The maximum Rayleigh numbers in these studies reach up to
$Ra$
at asymptotically large Rayleigh numbers. This was later proven in the analytical study of Doering & Constantin (Reference Doering and Constantin1998). With the advent of high-performance computing, several numerical studies of the DOB equations (herein DOB simulations) for large Rayleigh numbers have been performed. The maximum Rayleigh numbers in these studies reach up to
![]() $Ra=O(10^{4})$
. For example, the DOB simulations of Otero et al. (Reference Otero, Dontcheva, Johnston, Worthing, Kurganov, Petrova and Doering2004) suggest scaling of the form
$Ra=O(10^{4})$
. For example, the DOB simulations of Otero et al. (Reference Otero, Dontcheva, Johnston, Worthing, Kurganov, Petrova and Doering2004) suggest scaling of the form
![]() $Sh\sim Ra^{\unicode[STIX]{x1D6FC}}$
with
$Sh\sim Ra^{\unicode[STIX]{x1D6FC}}$
with
![]() $\unicode[STIX]{x1D6FC}\approx 0.9$
for
$\unicode[STIX]{x1D6FC}\approx 0.9$
for
![]() $Ra\leqslant 10\,000$
, which is slightly different from the theoretical linear scaling. However, subsequent DOB simulations, with
$Ra\leqslant 10\,000$
, which is slightly different from the theoretical linear scaling. However, subsequent DOB simulations, with
![]() $Ra$
up to 40 000, confirmed the linear scaling of
$Ra$
up to 40 000, confirmed the linear scaling of
![]() $Sh$
with
$Sh$
with
![]() $Ra$
for both isotropic (Hewitt, Neufeld & Lister Reference Hewitt, Neufeld and Lister2012, Reference Hewitt, Neufeld and Lister2013, Reference Hewitt, Neufeld and Lister2014; Wen, Corson & Chini Reference Wen, Corson and Chini2015) and anisotropic porous media (Paoli, Zonta & Soldati Reference Paoli, Zonta and Soldati2016).
$Ra$
for both isotropic (Hewitt, Neufeld & Lister Reference Hewitt, Neufeld and Lister2012, Reference Hewitt, Neufeld and Lister2013, Reference Hewitt, Neufeld and Lister2014; Wen, Corson & Chini Reference Wen, Corson and Chini2015) and anisotropic porous media (Paoli, Zonta & Soldati Reference Paoli, Zonta and Soldati2016).
Despite this encouraging agreement between theory and numerical simulations, experimental measurements of mass transfer yielded lower exponents. Backhaus, Turitsyn & Ecke (Reference Backhaus, Turitsyn and Ecke2011) and Neufeld et al. (Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010) reported
![]() $Sh\sim Ra^{0.8}$
. The experiments were also performed at very large Rayleigh numbers (
$Sh\sim Ra^{0.8}$
. The experiments were also performed at very large Rayleigh numbers (
![]() $Ra\gg 1300$
). Hence, discrepancies occur between the theoretical value of the exponent (
$Ra\gg 1300$
). Hence, discrepancies occur between the theoretical value of the exponent (
![]() $\unicode[STIX]{x1D6FC}=1$
) and the values of the exponent obtained from laboratory experiments.
$\unicode[STIX]{x1D6FC}=1$
) and the values of the exponent obtained from laboratory experiments.
It should be noted that many early numerical studies demonstrated nonlinear scaling of the Sherwood number, see Trevisan & Bejan (Reference Trevisan and Bejan1987), Robinson & O’Sullivan (Reference Robinson and O’Sullivan1976) and Caltagirone (Reference Caltagirone1975). However, the maximum Rayleigh number simulated by Trevisan & Bejan (Reference Trevisan and Bejan1987) and Caltagirone (Reference Caltagirone1975) was approximately 2000, which is only marginally larger than the transition Rayleigh number between regimes III and IV. Robinson & O’Sullivan (Reference Robinson and O’Sullivan1976) assumed that the flow is steady. Therefore, the nonlinear relationship between
![]() $Sh$
and
$Sh$
and
![]() $Ra$
found in the earlier numerical studies cannot explain the discrepancies between numerical and experimental results observed at very large Rayleigh numbers.
$Ra$
found in the earlier numerical studies cannot explain the discrepancies between numerical and experimental results observed at very large Rayleigh numbers.
These discrepancies cast doubt on the key hypothesis underlying the DOB equations, namely that the sole control parameter of convection in porous media is the Rayleigh number. This may result in serious model errors because of the many physical simplifications it carries. For example, Mijic, Laforce & Muggeridge (Reference Mijic, Laforce and Muggeridge2014) argued that the Forchheimer term (accounting for the quadratic drag typical of turbulence flows) may have an important effect on convection, whereas Wen, Chang & Hesse (Reference Wen, Chang and Hesse2018) argued that convection in a porous medium is also influenced by the dispersion term. Thus, the fundamental question arises as to whether natural convection in porous media is governed by parameters other than
![]() $Ra$
through additional physical mechanisms.
$Ra$
through additional physical mechanisms.
In this paper, we probe this question by performing pore-resolved direct numerical simulations (DNS) of convection in porous media, thereby accounting for all scales of motion. We compare our DNS to traditional DOB simulations to determine whether parameters other than
![]() $Ra$
influence convection in porous media.
$Ra$
influence convection in porous media.
2 Governing equations and numerical methods
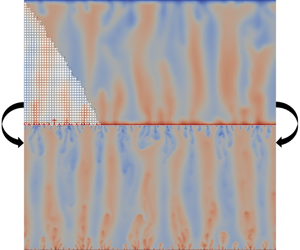
We considered natural convection in a chamber filled with porous elements, see figure 1(a). The upper and lower walls were kept at species concentrations
![]() $c_{1}$
and
$c_{1}$
and
![]() $c_{0}$
, respectively. The density difference caused by the different species concentrations at the lower and upper walls leads to natural convection in the chamber.
$c_{0}$
, respectively. The density difference caused by the different species concentrations at the lower and upper walls leads to natural convection in the chamber.

Figure 1. Porous matrices used in our DNS. In all cases, periodic boundary conditions are used in the horizontal direction. (a) Porous matrix for simulating convection in a porous medium. (b) Porous matrix for calculating the permeability
![]() $K$
. (c) Porous matrix for calculating the effective mass diffusivity
$K$
. (c) Porous matrix for calculating the effective mass diffusivity
![]() $D_{m}$
. In (c), the mean velocity of the fluid is zero, thus mass transfer is via diffusion only.
$D_{m}$
. In (c), the mean velocity of the fluid is zero, thus mass transfer is via diffusion only.
2.1 Governing equations for DNS
The governing equations for the DNS are the Navier–Stokes equations, with the buoyancy force accounted for by the Boussinesq approximation, and the species concentration equation. Using the Einstein’s summation convention, these equations are
where
![]() $ui$
is the
$ui$
is the
![]() $i$
th component of the velocity vector,
$i$
th component of the velocity vector,
![]() $p$
is the pressure,
$p$
is the pressure,
![]() $\unicode[STIX]{x1D70C}$
is the density,
$\unicode[STIX]{x1D70C}$
is the density,
![]() $\unicode[STIX]{x1D6FD}$
is the concentration expansion coefficient defined as
$\unicode[STIX]{x1D6FD}$
is the concentration expansion coefficient defined as
![]() $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FD}(c_{0})=-(1/\unicode[STIX]{x1D70C}_{0})(\unicode[STIX]{x2202}\unicode[STIX]{x1D70C}/\unicode[STIX]{x2202}c)_{c_{0}}$
,
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FD}(c_{0})=-(1/\unicode[STIX]{x1D70C}_{0})(\unicode[STIX]{x2202}\unicode[STIX]{x1D70C}/\unicode[STIX]{x2202}c)_{c_{0}}$
,
![]() $\boldsymbol{g}_{i}$
is the
$\boldsymbol{g}_{i}$
is the
![]() $i\text{th}$
component of the gravitational vector,
$i\text{th}$
component of the gravitational vector,
![]() $\unicode[STIX]{x1D708}$
is the kinematic viscosity and
$\unicode[STIX]{x1D708}$
is the kinematic viscosity and
![]() $D_{f}$
is the mass diffusivity of the species. The no-slip boundary condition is used at all solid surfaces, whereas the mass transfer rates at the surfaces of the solid obstacles are set to zero because they cannot be penetrated by CO2.
$D_{f}$
is the mass diffusivity of the species. The no-slip boundary condition is used at all solid surfaces, whereas the mass transfer rates at the surfaces of the solid obstacles are set to zero because they cannot be penetrated by CO2.
Using the characteristic concentration difference
![]() $\unicode[STIX]{x0394}c=c_{1}-c_{0}$
, velocity
$\unicode[STIX]{x0394}c=c_{1}-c_{0}$
, velocity
![]() $u_{m}=\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cgK/\unicode[STIX]{x1D708}$
, length
$u_{m}=\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cgK/\unicode[STIX]{x1D708}$
, length
![]() $H$
and time
$H$
and time
![]() $t_{m}=H/u_{m}$
, we obtained the following dimensionless governing equations:
$t_{m}=H/u_{m}$
, we obtained the following dimensionless governing equations:
where
![]() $\tilde{c}=(c-c_{0})/(c_{1}-c_{0})$
is the non-dimensional species concentration,
$\tilde{c}=(c-c_{0})/(c_{1}-c_{0})$
is the non-dimensional species concentration,
![]() $Ra_{f}=H^{3}\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cg/\unicode[STIX]{x1D708}D_{f}$
is the Rayleigh number for the free fluid flow between the bounded walls,
$Ra_{f}=H^{3}\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cg/\unicode[STIX]{x1D708}D_{f}$
is the Rayleigh number for the free fluid flow between the bounded walls,
![]() $Sc=\unicode[STIX]{x1D708}/D_{f}$
is the Schmidt number,
$Sc=\unicode[STIX]{x1D708}/D_{f}$
is the Schmidt number,
![]() $Da=K/H^{2}$
is the Darcy number,
$Da=K/H^{2}$
is the Darcy number,
![]() $H$
is the height of the chamber,
$H$
is the height of the chamber,
![]() $g$
is gravity,
$g$
is gravity,
![]() $z_{i}$
is the
$z_{i}$
is the
![]() $i\text{th}$
component of the unit vector pointing in the direction of gravity and
$i\text{th}$
component of the unit vector pointing in the direction of gravity and
![]() $K$
is the permeability.
$K$
is the permeability.
We calculated the Sherwood number from our DNS as the ratio of the total mass transfer rate
![]() ${\dot{m}}$
(by convection and diffusion) to diffusive mass transfer rate
${\dot{m}}$
(by convection and diffusion) to diffusive mass transfer rate
![]() ${\dot{m}}_{diff}$
across the wall
${\dot{m}}_{diff}$
across the wall
 $$\begin{eqnarray}Sh={\dot{m}}/{\dot{m}}_{diff}=\frac{\displaystyle \int _{w}\overline{{\displaystyle \frac{\unicode[STIX]{x2202}\tilde{c}}{\unicode[STIX]{x2202}\tilde{x}_{2}}}}\,\text{d}A}{\displaystyle \int _{w}\left.\overline{{\displaystyle \frac{\unicode[STIX]{x2202}\tilde{c}}{\unicode[STIX]{x2202}\tilde{x}_{2}}}}\right|_{Ra=0}\,\text{d}A}.\end{eqnarray}$$
$$\begin{eqnarray}Sh={\dot{m}}/{\dot{m}}_{diff}=\frac{\displaystyle \int _{w}\overline{{\displaystyle \frac{\unicode[STIX]{x2202}\tilde{c}}{\unicode[STIX]{x2202}\tilde{x}_{2}}}}\,\text{d}A}{\displaystyle \int _{w}\left.\overline{{\displaystyle \frac{\unicode[STIX]{x2202}\tilde{c}}{\unicode[STIX]{x2202}\tilde{x}_{2}}}}\right|_{Ra=0}\,\text{d}A}.\end{eqnarray}$$
The subscript
![]() $w$
denotes either the lower or upper wall surface, and the overbar
$w$
denotes either the lower or upper wall surface, and the overbar
![]() $\overline{\,\vphantom{a}\,}$
denotes the time average.
$\overline{\,\vphantom{a}\,}$
denotes the time average.
2.2 Governing equations for macroscopic simulations
We derived the governing equations for the macroscopic simulations using an approach similar to that used in de Lemos (Reference de Lemos2012), who averaged the governing equations (2.4)–(2.6) over volume and time. By contrast, only volume averaging was used in our derivation. By averaging (2.4)–(2.6) in each representative elementary volume (REV) and accounting for the zero mass flux at solid surfaces, we obtained the following macroscopic equations:
where
![]() $\hat{\;}$
denotes a volume-averaged dimensionless quantity,
$\hat{\;}$
denotes a volume-averaged dimensionless quantity,
![]() $\unicode[STIX]{x1D719}$
is the porosity and
$\unicode[STIX]{x1D719}$
is the porosity and
![]() $\hat{u} _{i}=\unicode[STIX]{x1D719}\langle \tilde{u} _{i}\rangle ^{i}$
and
$\hat{u} _{i}=\unicode[STIX]{x1D719}\langle \tilde{u} _{i}\rangle ^{i}$
and
![]() ${\hat{c}}=(\langle c\rangle ^{i}-c_{0})/(c_{1}-c_{0})$
are the volume-averaged dimensionless velocity and species concentration, respectively. Here, the Rayleigh number for convection in porous media is defined as
${\hat{c}}=(\langle c\rangle ^{i}-c_{0})/(c_{1}-c_{0})$
are the volume-averaged dimensionless velocity and species concentration, respectively. Here, the Rayleigh number for convection in porous media is defined as
where
![]() $\unicode[STIX]{x1D6FE}_{m}=D_{m}/D_{f}$
is the ratio of the effective mass diffusivity,
$\unicode[STIX]{x1D6FE}_{m}=D_{m}/D_{f}$
is the ratio of the effective mass diffusivity,
![]() $D_{m}$
, and the fluid mass diffusivity,
$D_{m}$
, and the fluid mass diffusivity,
![]() $D_{f}$
. The effective mass diffusivity,
$D_{f}$
. The effective mass diffusivity,
![]() $D_{m}$
, is a macroscopic parameter which describes the mass diffusion through the porous medium. Further, note that the parameter
$D_{m}$
, is a macroscopic parameter which describes the mass diffusion through the porous medium. Further, note that the parameter
![]() $D_{m}$
is different from
$D_{m}$
is different from
![]() $D_{f}$
due to the effect of the porous matrix. Quantities
$D_{f}$
due to the effect of the porous matrix. Quantities
![]() $\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i}$
and
$\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i}$
and
![]() $\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{c}\rangle ^{i}$
represent the momentum dispersion and species concentration dispersion, respectively.
$\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{c}\rangle ^{i}$
represent the momentum dispersion and species concentration dispersion, respectively.
The dimensionless total drag,
![]() $\hat{R}_{i}$
, is usually modelled by the sum of the Darcy term and the Forchheimer term, i.e.
$\hat{R}_{i}$
, is usually modelled by the sum of the Darcy term and the Forchheimer term, i.e.
where
![]() $C_{F}$
is the (empirical) Forchheimer coefficient. Neglecting the higher-order terms with respect to
$C_{F}$
is the (empirical) Forchheimer coefficient. Neglecting the higher-order terms with respect to
![]() $Da=K/H^{2}$
and the dispersion terms
$Da=K/H^{2}$
and the dispersion terms
![]() $\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
and
$\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
and
![]() $\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{c}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
, one obtains the traditional DOB equations:
$\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{c}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
, one obtains the traditional DOB equations:
where
![]() $\hat{p}=RaDa\langle \tilde{p}\rangle ^{i}/\unicode[STIX]{x1D6FE}_{m}Sc$
is the normalized pressure. The dimensionless time is modified to be
$\hat{p}=RaDa\langle \tilde{p}\rangle ^{i}/\unicode[STIX]{x1D6FE}_{m}Sc$
is the normalized pressure. The dimensionless time is modified to be
![]() $\hat{t}^{\ast }=\hat{t}/\unicode[STIX]{x1D719}$
. The Sherwood number for DOB studies is calculated as
$\hat{t}^{\ast }=\hat{t}/\unicode[STIX]{x1D719}$
. The Sherwood number for DOB studies is calculated as
 $$\begin{eqnarray}Sh=\frac{\displaystyle \int _{w}\overline{{\displaystyle \frac{\unicode[STIX]{x2202}{\hat{c}}}{\unicode[STIX]{x2202}\hat{x}_{2}}}}\,\text{d}A}{A_{w}},\end{eqnarray}$$
$$\begin{eqnarray}Sh=\frac{\displaystyle \int _{w}\overline{{\displaystyle \frac{\unicode[STIX]{x2202}{\hat{c}}}{\unicode[STIX]{x2202}\hat{x}_{2}}}}\,\text{d}A}{A_{w}},\end{eqnarray}$$
where
![]() $A_{w}$
is the surface area of the wall. Note that the definitions given by (2.7) and (2.16) are equivalent and are in accordance with the definitions in previous DOB studies (Hewitt et al.
Reference Hewitt, Neufeld and Lister2012, Reference Hewitt, Neufeld and Lister2013, Reference Hewitt, Neufeld and Lister2014).
$A_{w}$
is the surface area of the wall. Note that the definitions given by (2.7) and (2.16) are equivalent and are in accordance with the definitions in previous DOB studies (Hewitt et al.
Reference Hewitt, Neufeld and Lister2012, Reference Hewitt, Neufeld and Lister2013, Reference Hewitt, Neufeld and Lister2014).
In the framework of the DOB equations (2.1)–(2.3), convection in porous media is uniquely determined by
![]() $Ra$
, as defined in (2.11). Obviously, a prerequisite for the validity of the DOB equations is that the Darcy number is small in order for the terms of
$Ra$
, as defined in (2.11). Obviously, a prerequisite for the validity of the DOB equations is that the Darcy number is small in order for the terms of
![]() $O(1/Da)$
to dominate in (2.9). However, the Darcy number may affect the governing equations if
$O(1/Da)$
to dominate in (2.9). However, the Darcy number may affect the governing equations if
![]() $Da$
is not small enough. In addition, convection in porous media may also be affected by the Schmidt number
$Da$
is not small enough. In addition, convection in porous media may also be affected by the Schmidt number
![]() $Sc$
, the porosity
$Sc$
, the porosity
![]() $\unicode[STIX]{x1D719}$
or pore-scale factors such as the pore scale
$\unicode[STIX]{x1D719}$
or pore-scale factors such as the pore scale
![]() $s$
. The momentum and species dispersion terms may also influence the convection in porous media. Understanding whether these factors should be accounted for in the macroscopic equations requires further analysis.
$s$
. The momentum and species dispersion terms may also influence the convection in porous media. Understanding whether these factors should be accounted for in the macroscopic equations requires further analysis.
2.3 Numerical method
A finite-volume method was employed in the DNS. The solver was developed based on the open source code package OpenFoam 2.4. The solutions of the DNS were advanced in time with the second-order implicit backward method. A second-order central-difference scheme was used for the spatial discretization. The pressure and velocity fields were corrected by the pressure-implicit scheme with splitting of operators pressure–velocity coupling (Versteeg & Malalasekera Reference Versteeg and Malalasekera2007). A stabilized preconditioned (bi-)conjugate gradient solver was utilized to solve the pressure field and the momentum and species concentration equations.
A streamfunction method was used to solve the DOB equations (2.13)–(2.15). A streamfunction,
![]() $\unicode[STIX]{x1D713}$
, was introduced for the velocity field, i.e.
$\unicode[STIX]{x1D713}$
, was introduced for the velocity field, i.e.
The curl of the momentum equation, equation (2.14), gives
In the streamfunction method, (2.15) is advanced in time to update the mass concentration field. Then, the Poisson equation, equation (2.18), is solved to calculate the streamfunction,
![]() $\unicode[STIX]{x1D713}$
. Finally, the velocity is updated with (2.17). The second-order implicit backward method was used in the streamfunction method for the time discretization. For the convection terms, we used linear interpolation to determine the variables at the cell interfaces. A second-order central-difference scheme was used for spatial discretization. A stabilized preconditioned (bi-)conjugate gradient solver was used to solve the Poisson equation (2.18). A preconditioned (bi-)conjugate gradient solver was used to solve the species concentration equations.
$\unicode[STIX]{x1D713}$
. Finally, the velocity is updated with (2.17). The second-order implicit backward method was used in the streamfunction method for the time discretization. For the convection terms, we used linear interpolation to determine the variables at the cell interfaces. A second-order central-difference scheme was used for spatial discretization. A stabilized preconditioned (bi-)conjugate gradient solver was used to solve the Poisson equation (2.18). A preconditioned (bi-)conjugate gradient solver was used to solve the species concentration equations.
The code validation for our DNS solver was performed in our previous studies (Jin et al. Reference Jin, Uth, Kuznetsov and Herwig2015; Uth et al. Reference Uth, Jin, Kuznetsov and Herwig2016; Jin & Kuznetsov Reference Jin and Kuznetsov2017). In these studies, as well as the present study, the finite volume method was employed to simulate turbulent flows in channels with smooth and rough walls, and in porous media. The DNS results were compared with the DNS and experimental results reported in other references, as well as our DNS results obtained with the lattice Boltzmann method. The code validation for our DOB solver was performed in Kränzien & Jin (Reference Kränzien and Jin2019), where the obtained results were compared with Hewitt et al. (Reference Hewitt, Neufeld and Lister2012).
3 Studied test cases
The chosen two-dimensional porous matrix was composed of periodically arranged square obstacles of size
![]() $d$
, which are a distance
$d$
, which are a distance
![]() $s$
apart in the lateral and vertical directions. The solid matrix was assumed to be adiabatic, i.e. the flux of species through the obstacles is zero. The porous matrix and the REV are shown in figure 1(a). The porosity for the current porous matrix can be directly calculated from figure 1(a) as
$s$
apart in the lateral and vertical directions. The solid matrix was assumed to be adiabatic, i.e. the flux of species through the obstacles is zero. The porous matrix and the REV are shown in figure 1(a). The porosity for the current porous matrix can be directly calculated from figure 1(a) as
The porous matrix was composed of 800–20 000 REVs, and each REV was resolved by 1600 to 6400 mesh cells, yielding up to 72 million cells in the simulations. The same initial fields (
![]() $u_{i}=0$
and
$u_{i}=0$
and
![]() $c=(c_{0}+c_{1})/2$
) were used in both DOB and DNS solutions. The largest DNS case was calculated using 384 processors in parallel for 960 h.
$c=(c_{0}+c_{1})/2$
) were used in both DOB and DNS solutions. The largest DNS case was calculated using 384 processors in parallel for 960 h.
The temporal evolution of the instantaneous Sherwood number
![]() $Sh$
for a typical DNS is shown in figure 2. Time averaging was performed after
$Sh$
for a typical DNS is shown in figure 2. Time averaging was performed after
![]() $Sh$
reached a statistically steady state, as indicated by the vertical dashed line. The computational time needed for this process depended on the flow parameters, such as
$Sh$
reached a statistically steady state, as indicated by the vertical dashed line. The computational time needed for this process depended on the flow parameters, such as
![]() $Ra$
,
$Ra$
,
![]() $H/s$
and
$H/s$
and
![]() $Sc$
.
$Sc$
.

Figure 2. Sherwood number (
![]() $Sh$
) versus dimensionless time
$Sh$
) versus dimensionless time
![]() $\tilde{t}$
.
$\tilde{t}$
.
![]() $Ra=20\,000$
,
$Ra=20\,000$
,
![]() $Sc=250$
and
$Sc=250$
and
![]() $H/s=100$
. The initial fields have
$H/s=100$
. The initial fields have
![]() $u_{i}=0$
and
$u_{i}=0$
and
![]() $c=(c_{0}+c_{1})/2$
.
$c=(c_{0}+c_{1})/2$
.
To compare the DNS results with the DOB results, the permeability,
![]() $K$
, was estimated by simulating forced convection in the porous matrix shown in figure 1(b). Using this method,
$K$
, was estimated by simulating forced convection in the porous matrix shown in figure 1(b). Using this method,
![]() $K$
was calculated as the ratio of the applied pressure gradient to the mean velocity
$K$
was calculated as the ratio of the applied pressure gradient to the mean velocity
![]() $u_{m}$
. To determine the effective mass diffusivity,
$u_{m}$
. To determine the effective mass diffusivity,
![]() $D_{m}$
, due to tortuosity, we simulated mass transfer in the same porous matrix, but bounded between two impermeable walls with
$D_{m}$
, due to tortuosity, we simulated mass transfer in the same porous matrix, but bounded between two impermeable walls with
![]() $u_{m}=0$
(see figure 1
c).
$u_{m}=0$
(see figure 1
c).
![]() $D_{m}$
was calculated as
$D_{m}$
was calculated as
![]() $(HD_{f}/\unicode[STIX]{x0394}cA_{w})\int _{w}(\overline{\unicode[STIX]{x2202}c}/\unicode[STIX]{x2202}x_{2})\,\text{d}A$
, where
$(HD_{f}/\unicode[STIX]{x0394}cA_{w})\int _{w}(\overline{\unicode[STIX]{x2202}c}/\unicode[STIX]{x2202}x_{2})\,\text{d}A$
, where
![]() $A_{w}$
is the area of the upper (or lower) wall. Therefore, the Rayleigh number in our pore-resolved DNS study is the same as the one for the macroscopic (continuum scale) simulation. The values of the parameters used in our simulations are given in table 1.
$A_{w}$
is the area of the upper (or lower) wall. Therefore, the Rayleigh number in our pore-resolved DNS study is the same as the one for the macroscopic (continuum scale) simulation. The values of the parameters used in our simulations are given in table 1.
Table 1. Main parameters for DNS and DOB cases.

We investigated cases characterized by
![]() $Ra\leqslant 20\,000$
, scale ratios
$Ra\leqslant 20\,000$
, scale ratios
![]() $H/s$
between 20 and 100, Schmidt numbers
$H/s$
between 20 and 100, Schmidt numbers
![]() $Sc=1$
and
$Sc=1$
and
![]() $Sc=250$
, and Darcy numbers between
$Sc=250$
, and Darcy numbers between
![]() $2.8\times 10^{-7}$
and
$2.8\times 10^{-7}$
and
![]() $8.7\times 10^{-6}$
. According to the Kozeny’s equation (Nield & Bejan Reference Nield and Bejan2017), the permeability
$8.7\times 10^{-6}$
. According to the Kozeny’s equation (Nield & Bejan Reference Nield and Bejan2017), the permeability
![]() $K$
can be approximated as
$K$
can be approximated as
![]() $K^{\ast }$
:
$K^{\ast }$
:
The pore size can be approximated from the permeability
![]() $K^{\ast }$
and the porosity
$K^{\ast }$
and the porosity
![]() $\unicode[STIX]{x1D719}$
as
$\unicode[STIX]{x1D719}$
as
where the empirical model coefficient
![]() $\unicode[STIX]{x1D6FD}=126$
was used in this study. This value was obtained by fitting the values of
$\unicode[STIX]{x1D6FD}=126$
was used in this study. This value was obtained by fitting the values of
![]() $s^{\ast }$
to the real pore size
$s^{\ast }$
to the real pore size
![]() $s$
. The maximum difference between
$s$
. The maximum difference between
![]() $s^{\ast }$
and
$s^{\ast }$
and
![]() $s$
was 4.8 %, which we deemed an acceptable approximation.
$s$
was 4.8 %, which we deemed an acceptable approximation.
We studied the sensitivity of the numerical results to the mesh resolution and time step for a test case with
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
) and
$s/d=1.5$
) and
![]() $Ra=20\,000$
(see table 2). The numerical results showed that the Sherwood number is over-predicted when the mesh resolution is insufficient (case ‘f’), while it is under-predicted when the time step, indicated by the maximum Courant number (
$Ra=20\,000$
(see table 2). The numerical results showed that the Sherwood number is over-predicted when the mesh resolution is insufficient (case ‘f’), while it is under-predicted when the time step, indicated by the maximum Courant number (
![]() $Co_{max}$
), is too large (case ‘b’). The Sherwood numbers calculated for cases ‘a’ and ‘c’ through ‘e’ vary by a maximum of 2.5 %. We adopted this range of mesh resolutions and
$Co_{max}$
), is too large (case ‘b’). The Sherwood numbers calculated for cases ‘a’ and ‘c’ through ‘e’ vary by a maximum of 2.5 %. We adopted this range of mesh resolutions and
![]() $Co_{max}$
for all other test cases, including the cases with small
$Co_{max}$
for all other test cases, including the cases with small
![]() $Ra$
numbers. Thus, we estimate numerical errors in our DNS studies to be below 2.5 %.
$Ra$
numbers. Thus, we estimate numerical errors in our DNS studies to be below 2.5 %.
Table 2. Effects of the mesh resolution and maximum Courant number on the Sherwood number. The test case has the parameters
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
) and
$s/d=1.5$
) and
![]() $Ra=20\,000$
. The cases that are shaded grey were considered as converged (mesh and time step independent).
$Ra=20\,000$
. The cases that are shaded grey were considered as converged (mesh and time step independent).
![]() $N_{x}$
and
$N_{x}$
and
![]() $N_{y}$
are the REV numbers in horizontal and vertical directions, while
$N_{y}$
are the REV numbers in horizontal and vertical directions, while
![]() $N_{REV}$
is the number of mesh cells in each REV.
$N_{REV}$
is the number of mesh cells in each REV.

4 Results and discussion
4.1 Mega- and proto-plumes
A local Reynolds number
![]() $Re_{K}$
may be defined based on the permeability
$Re_{K}$
may be defined based on the permeability
![]() $K$
and the local velocity magnitude
$K$
and the local velocity magnitude
![]() $|\boldsymbol{u}|$
, i.e.
$|\boldsymbol{u}|$
, i.e.
Nield & Bejan (Reference Nield and Bejan2017) indicated that the Darcy’s term dominates the drag when
![]() $Re_{K}\ll 1$
, while the Forchheimer’s term has a greater effect on the flow for
$Re_{K}\ll 1$
, while the Forchheimer’s term has a greater effect on the flow for
![]() $Re_{K}>1$
. It should be noted that
$Re_{K}>1$
. It should be noted that
![]() $|\boldsymbol{u}|$
in (4.1) cannot be approximated by the characteristic (macroscopic) velocity of the flow
$|\boldsymbol{u}|$
in (4.1) cannot be approximated by the characteristic (macroscopic) velocity of the flow
![]() $u_{m}$
, which is much larger than
$u_{m}$
, which is much larger than
![]() $|\boldsymbol{u}|$
.
$|\boldsymbol{u}|$
.

Figure 3. Snapshots of the instantaneous Reynolds number
![]() $Re_{K}$
based on the permeability at
$Re_{K}$
based on the permeability at
![]() $Ra=20\,000$
from DNS results with
$Ra=20\,000$
from DNS results with
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
). (a)
$s/d=1.5$
). (a)
![]() $Sc=250$
,
$Sc=250$
,
![]() $H/s=20$
; (b)
$H/s=20$
; (b)
![]() $Sc=250$
,
$Sc=250$
,
![]() $H/s=50$
; (c)
$H/s=50$
; (c)
![]() $Sc=1$
,
$Sc=1$
,
![]() $H/s=20$
.
$H/s=20$
.
Figure 3 shows typical instantaneous fields of
![]() $Re_{K}$
for
$Re_{K}$
for
![]() $Ra=20\,000$
. The
$Ra=20\,000$
. The
![]() $Re_{K}$
values of all the cases for
$Re_{K}$
values of all the cases for
![]() $Sc=250$
are much smaller than unity and thus the flow is in the Darcy’s regime. According to our DNS, the flow in the pores is laminar, despite the large Rayleigh number, because of the very small local Reynolds numbers (
$Sc=250$
are much smaller than unity and thus the flow is in the Darcy’s regime. According to our DNS, the flow in the pores is laminar, despite the large Rayleigh number, because of the very small local Reynolds numbers (
![]() $Re_{K}$
). The macroscopic flows at large Rayleigh numbers are nevertheless strongly nonlinear and chaotic, exhibiting ‘macroscopic turbulence’. The convection for
$Re_{K}$
). The macroscopic flows at large Rayleigh numbers are nevertheless strongly nonlinear and chaotic, exhibiting ‘macroscopic turbulence’. The convection for
![]() $Sc=1$
is also generally in the Darcy’s regime. The largest value of
$Sc=1$
is also generally in the Darcy’s regime. The largest value of
![]() $Re_{K}$
for
$Re_{K}$
for
![]() $Sc=1$
is about 2.8, which occurs for the case of
$Sc=1$
is about 2.8, which occurs for the case of
![]() $Ra=20\,000$
,
$Ra=20\,000$
,
![]() $H/s=20$
and
$H/s=20$
and
![]() $\unicode[STIX]{x1D719}=0.56$
. These results suggest that the Forchheimer’s term may have more effect in heat transfer problems than in mass transfer problems because a Prandtl number of one is common in heat transfer, whereas typical Schmidt number values are larger than unity.
$\unicode[STIX]{x1D719}=0.56$
. These results suggest that the Forchheimer’s term may have more effect in heat transfer problems than in mass transfer problems because a Prandtl number of one is common in heat transfer, whereas typical Schmidt number values are larger than unity.

Figure 4. Snapshots of instantaneous mass concentration fields at
![]() $Ra=20\,000$
and
$Ra=20\,000$
and
![]() $Sc=1$
. (a) DNS with
$Sc=1$
. (a) DNS with
![]() $H/s=50$
and
$H/s=50$
and
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
). Here, the macroscopic mass concentration was obtained by volume averaging the microscopic mass concentration over each REV; (b) DNS with
$s/d=1.5$
). Here, the macroscopic mass concentration was obtained by volume averaging the microscopic mass concentration over each REV; (b) DNS with
![]() $H/s=20$
and
$H/s=20$
and
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
); (c) DOB simulation.
$s/d=1.5$
); (c) DOB simulation.
The instantaneous species concentration fields obtained from our DNS and DOB simulations at
![]() $Ra=20\,000$
are shown in figure 4. The macroscopic species concentration field
$Ra=20\,000$
are shown in figure 4. The macroscopic species concentration field
![]() $\langle c\rangle$
was either obtained from the DNS results by volume averaging over each REV (figure 4
a,b), or calculated directly in DOB simulations (figure 4
c). Both DNS and DOB mass concentration fields exhibit two boundary layer regions and an interior region. The interior region is dominated by transient mega-plumes, whereas the boundary layers are filled with small proto-plumes. These small proto-plumes are products of the growing instabilities in the boundary layers that cause low concentration fluid to rise and high concentration fluid to sink.
$\langle c\rangle$
was either obtained from the DNS results by volume averaging over each REV (figure 4
a,b), or calculated directly in DOB simulations (figure 4
c). Both DNS and DOB mass concentration fields exhibit two boundary layer regions and an interior region. The interior region is dominated by transient mega-plumes, whereas the boundary layers are filled with small proto-plumes. These small proto-plumes are products of the growing instabilities in the boundary layers that cause low concentration fluid to rise and high concentration fluid to sink.
Interestingly, the instantaneous species concentration fields obtained by DNS and displayed in figures 4(a) and 4(b) suggest that the size of mega- and proto-plumes increases with the pore size. This phenomenon is absent in classical Rayleigh–Bénard convection (without a porous medium) and cannot be captured by the DOB equations where pore effects enter the equations only via
![]() $Ra$
.
$Ra$
.

Figure 5. Instantaneous profiles (a) and average spectra (b) of the dimensionless mass concentration,
![]() ${\hat{c}}$
, at mid-height
${\hat{c}}$
, at mid-height
![]() $x_{2}/H=0.5$
.
$x_{2}/H=0.5$
.
![]() $Ra=20\,000$
,
$Ra=20\,000$
,
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
) and
$s/d=1.5$
) and
![]() $Sc=1$
.
$Sc=1$
.
Figure 5 shows several instantaneous profiles of
![]() ${\hat{c}}$
along with their corresponding time-averaged discrete Fourier transforms at mid-height for
${\hat{c}}$
along with their corresponding time-averaged discrete Fourier transforms at mid-height for
![]() $Ra=20\,000$
and
$Ra=20\,000$
and
![]() $Sc=1$
. The mean concentration
$Sc=1$
. The mean concentration
![]() $\langle {\hat{c}}\rangle ^{x1}$
, where the operator
$\langle {\hat{c}}\rangle ^{x1}$
, where the operator
![]() $\langle \cdot \rangle ^{x1}$
denotes horizontal averaging, was extracted from
$\langle \cdot \rangle ^{x1}$
denotes horizontal averaging, was extracted from
![]() ${\hat{c}}$
. The number of
${\hat{c}}$
. The number of
![]() ${\hat{c}}$
maxima, which corresponds to the number of mega-plumes, decreases with increases in pore size
${\hat{c}}$
maxima, which corresponds to the number of mega-plumes, decreases with increases in pore size
![]() $s$
(see figure 5
a). In the DOB simulations, the peak amplitude occurs when the wavenumber
$s$
(see figure 5
a). In the DOB simulations, the peak amplitude occurs when the wavenumber
![]() $k$
equals
$k$
equals
![]() $9\unicode[STIX]{x03C0}$
. Wen et al. (Reference Wen, Corson and Chini2015) indicted that the wavenumber is not unique for
$9\unicode[STIX]{x03C0}$
. Wen et al. (Reference Wen, Corson and Chini2015) indicted that the wavenumber is not unique for
![]() $Ra>39\,716$
. However, the wavenumber is well approximated by
$Ra>39\,716$
. However, the wavenumber is well approximated by
![]() $k=0.48Ra^{0.4}$
when
$k=0.48Ra^{0.4}$
when
![]() $Ra$
is smaller than this critical value. Figure 6 shows that our DOB results are close to this correlation, as well as to the DOB results of Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) and Wen et al. (Reference Wen, Corson and Chini2015). A variability of the peak wavenumber due to long-time-scale fluctuations was observed in Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) when different initial field or aspect ratio (
$Ra$
is smaller than this critical value. Figure 6 shows that our DOB results are close to this correlation, as well as to the DOB results of Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) and Wen et al. (Reference Wen, Corson and Chini2015). A variability of the peak wavenumber due to long-time-scale fluctuations was observed in Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) when different initial field or aspect ratio (
![]() $L/H$
) were used, see hollow circles in figure 6. This variability was not found in our study since the same initial field and aspect ratio were used in both our DNS and DOB solutions.
$L/H$
) were used, see hollow circles in figure 6. This variability was not found in our study since the same initial field and aspect ratio were used in both our DNS and DOB solutions.
In the DNS, the peaks are broader and the dominant wavenumber increases from
![]() $4\unicode[STIX]{x03C0}$
to
$4\unicode[STIX]{x03C0}$
to
![]() $7\unicode[STIX]{x03C0}$
as
$7\unicode[STIX]{x03C0}$
as
![]() $H/s$
increases from 20 to 50. This emphasizes the importance of the pore scale
$H/s$
increases from 20 to 50. This emphasizes the importance of the pore scale
![]() $s$
in shaping the mega-plumes. Motions at even larger length scales (
$s$
in shaping the mega-plumes. Motions at even larger length scales (
![]() $k\approx \unicode[STIX]{x03C0}$
), which corresponds to the length of the box (
$k\approx \unicode[STIX]{x03C0}$
), which corresponds to the length of the box (
![]() $2H$
), were observed in the DNS.
$2H$
), were observed in the DNS.

Figure 6. Peak wavenumber
![]() $k$
for the mega-plumes of the DOB results for different Rayleigh numbers. The maximum error bar for the peak wavenumber is estimated according to the wavenumber step size
$k$
for the mega-plumes of the DOB results for different Rayleigh numbers. The maximum error bar for the peak wavenumber is estimated according to the wavenumber step size
![]() $\unicode[STIX]{x0394}k=\unicode[STIX]{x03C0}$
in our discrete fast Fourier transform (FFT).
$\unicode[STIX]{x0394}k=\unicode[STIX]{x03C0}$
in our discrete fast Fourier transform (FFT).
Figure 7 shows several instantaneous profiles of
![]() ${\hat{c}}$
and their corresponding time-averaged Fourier transforms for
${\hat{c}}$
and their corresponding time-averaged Fourier transforms for
![]() $Ra=20\,000$
and
$Ra=20\,000$
and
![]() $Sc=250$
. Similar to the results for
$Sc=250$
. Similar to the results for
![]() $Sc=1$
, the dominant wavenumber increases from
$Sc=1$
, the dominant wavenumber increases from
![]() $4\unicode[STIX]{x03C0}$
to
$4\unicode[STIX]{x03C0}$
to
![]() $7\unicode[STIX]{x03C0}$
as
$7\unicode[STIX]{x03C0}$
as
![]() $H/s$
increases from 20 to 100. Here, the smallest Darcy number
$H/s$
increases from 20 to 100. Here, the smallest Darcy number
![]() $Da$
is
$Da$
is
![]() $3.5\times 10^{-7}$
, which corresponds to
$3.5\times 10^{-7}$
, which corresponds to
![]() $H/s=100$
. Large-length-scale (
$H/s=100$
. Large-length-scale (
![]() $k=\unicode[STIX]{x03C0}$
) motions were also found in this test case, which makes the DNS results distinctly different from the DOB results. However, more detailed investigations are required to clarify the origin of these motions. We expect that the plumes will keep getting smaller as
$k=\unicode[STIX]{x03C0}$
) motions were also found in this test case, which makes the DNS results distinctly different from the DOB results. However, more detailed investigations are required to clarify the origin of these motions. We expect that the plumes will keep getting smaller as
![]() $H/s\rightarrow \infty$
and may even eventually converge toward the DOB results, see figure 8. However, although
$H/s\rightarrow \infty$
and may even eventually converge toward the DOB results, see figure 8. However, although
![]() $Re_{k}$
for the DNS case is much smaller than 1 (see figure 3
b for
$Re_{k}$
for the DNS case is much smaller than 1 (see figure 3
b for
![]() $H/s=50$
) and the pore size
$H/s=50$
) and the pore size
![]() $s$
(
$s$
(
![]() $0.01H$
for
$0.01H$
for
![]() $H/s=100$
) is much smaller than the plume size (approximately
$H/s=100$
) is much smaller than the plume size (approximately
![]() $0.23H$
for
$0.23H$
for
![]() $Ra=20\,000$
, the DOB results), the peak wavenumbers from the DNS results are still clearly different from the DOB results. A possible reason is that no matter how small the pore size, the pore-scale structure may always affect part of the boundary layer in the vicinity of the wall.
$Ra=20\,000$
, the DOB results), the peak wavenumbers from the DNS results are still clearly different from the DOB results. A possible reason is that no matter how small the pore size, the pore-scale structure may always affect part of the boundary layer in the vicinity of the wall.

Figure 7. Instantaneous profiles (a) and average spectra (b) of the dimensionless mass concentration,
![]() ${\hat{c}}$
, at mid-height
${\hat{c}}$
, at mid-height
![]() $x_{2}/H=0.5$
,
$x_{2}/H=0.5$
,
![]() $Ra=20\,000$
,
$Ra=20\,000$
,
![]() $\unicode[STIX]{x1D719}=0.56$
(
$\unicode[STIX]{x1D719}=0.56$
(
![]() $s/d=1.5$
) and
$s/d=1.5$
) and
![]() $Sc=250$
.
$Sc=250$
.

Figure 8. Peak wavenumber
![]() $k$
for the mega-plumes of the DNS results for different Darcy numbers,
$k$
for the mega-plumes of the DNS results for different Darcy numbers,
![]() $Ra=20\,000$
. The maximum error bar for the peak wavenumber is estimated according to the wavenumber step size
$Ra=20\,000$
. The maximum error bar for the peak wavenumber is estimated according to the wavenumber step size
![]() $\unicode[STIX]{x0394}k=\unicode[STIX]{x03C0}$
in our discrete FFT.
$\unicode[STIX]{x0394}k=\unicode[STIX]{x03C0}$
in our discrete FFT.
4.2 Sherwood number
The scaling of the Sherwood number for
![]() $Sc=1$
obtained from our DNS results, with various
$Sc=1$
obtained from our DNS results, with various
![]() $H/s$
and porosity values, is shown in figure 9. The DOB simulations of Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) and our own DOB results are compared with our DNS results (shown by solid and dashed lines in figure 9
a). It appears that the DOB simulations overestimate the mass transfer rate for
$H/s$
and porosity values, is shown in figure 9. The DOB simulations of Hewitt et al. (Reference Hewitt, Neufeld and Lister2012) and our own DOB results are compared with our DNS results (shown by solid and dashed lines in figure 9
a). It appears that the DOB simulations overestimate the mass transfer rate for
![]() $Ra$
in the range between 600 and 4000, whereas at large
$Ra$
in the range between 600 and 4000, whereas at large
![]() $Ra$
, they fall amidst the values obtained by DNS for the porosities studied here. Although the scale ratio
$Ra$
, they fall amidst the values obtained by DNS for the porosities studied here. Although the scale ratio
![]() $H/s$
appears to determine the scale of mega-plumes, it only mildly influences
$H/s$
appears to determine the scale of mega-plumes, it only mildly influences
![]() $Sh$
and without a clear trend.
$Sh$
and without a clear trend.

Figure 9. Time- and surface-averaged Sherwood number. Lines, DOB results; symbols, DNS results. The porosity
![]() $\unicode[STIX]{x1D719}$
and scale ratio
$\unicode[STIX]{x1D719}$
and scale ratio
![]() $H/s$
are varied. The results for
$H/s$
are varied. The results for
![]() $Sc=1$
are shown in a log–log scale (a), and a linear scale for
$Sc=1$
are shown in a log–log scale (a), and a linear scale for
![]() $Ra>5000$
(b).
$Ra>5000$
(b).
The DNS results show that the porosity has a significant effect on
![]() $Sh$
in the high Rayleigh number regime (
$Sh$
in the high Rayleigh number regime (
![]() $Ra>10\,000$
). For example, at
$Ra>10\,000$
). For example, at
![]() $Ra=20\,000$
,
$Ra=20\,000$
,
![]() $Sc=1$
and
$Sc=1$
and
![]() $H/s=20$
,
$H/s=20$
,
![]() $Sh$
decreases from 158 to 96 while the porosity increases from
$Sh$
decreases from 158 to 96 while the porosity increases from
![]() $\unicode[STIX]{x1D719}=0.36$
(
$\unicode[STIX]{x1D719}=0.36$
(
![]() $s/d=1.25$
) to 0.56 (
$s/d=1.25$
) to 0.56 (
![]() $s/d=1.5$
). At large Rayleigh numbers (
$s/d=1.5$
). At large Rayleigh numbers (
![]() $Ra>5000$
), the relationship between
$Ra>5000$
), the relationship between
![]() $Sh$
and
$Sh$
and
![]() $Ra$
can be well approximated by
$Ra$
can be well approximated by
![]() $Sh=8+0.0076Ra$
for
$Sh=8+0.0076Ra$
for
![]() $\unicode[STIX]{x1D719}=0.36$
. This linear relationship is in accordance with our own DOB results (Kränzien & Jin Reference Kränzien and Jin2019), as well as the DOB results by Hewitt et al. (Reference Hewitt, Neufeld and Lister2012). However, for
$\unicode[STIX]{x1D719}=0.36$
. This linear relationship is in accordance with our own DOB results (Kränzien & Jin Reference Kränzien and Jin2019), as well as the DOB results by Hewitt et al. (Reference Hewitt, Neufeld and Lister2012). However, for
![]() $\unicode[STIX]{x1D719}=0.56$
, the correlation
$\unicode[STIX]{x1D719}=0.56$
, the correlation
![]() $Sh=0.033Ra^{0.8}$
better fits the data.
$Sh=0.033Ra^{0.8}$
better fits the data.
The Sherwood number slightly increases as the Schmidt number is increased from 1 to 250. However, the relationship between
![]() $Sh$
and
$Sh$
and
![]() $Ra$
does not change qualitatively (see figure 10). At large Rayleigh numbers (
$Ra$
does not change qualitatively (see figure 10). At large Rayleigh numbers (
![]() $Ra>5000$
), the Sherwood number is still higher for a lower porosity. The
$Ra>5000$
), the Sherwood number is still higher for a lower porosity. The
![]() $Sh=f(Ra)$
scaling changes from a linear scaling (
$Sh=f(Ra)$
scaling changes from a linear scaling (
![]() $Sh=16+0.0076Ra$
) for
$Sh=16+0.0076Ra$
) for
![]() $\unicode[STIX]{x1D719}=0.36$
to a nonlinear one (
$\unicode[STIX]{x1D719}=0.36$
to a nonlinear one (
![]() $Sh=0.045Ra^{0.8}$
) for
$Sh=0.045Ra^{0.8}$
) for
![]() $\unicode[STIX]{x1D719}=0.56$
. The exponential coefficients for
$\unicode[STIX]{x1D719}=0.56$
. The exponential coefficients for
![]() $Sc=250$
are the same as those for
$Sc=250$
are the same as those for
![]() $Sc=1$
(
$Sc=1$
(
![]() $\unicode[STIX]{x1D6FC}=1$
for
$\unicode[STIX]{x1D6FC}=1$
for
![]() $\unicode[STIX]{x1D719}=0.36$
and
$\unicode[STIX]{x1D719}=0.36$
and
![]() $\unicode[STIX]{x1D6FC}=0.8$
for
$\unicode[STIX]{x1D6FC}=0.8$
for
![]() $\unicode[STIX]{x1D719}=0.56$
), which indicates that the relationship between
$\unicode[STIX]{x1D719}=0.56$
), which indicates that the relationship between
![]() $Sh$
and
$Sh$
and
![]() $Ra$
does not change qualitatively if
$Ra$
does not change qualitatively if
![]() $Sc$
is varied. Our DNS results suggest that the nonlinear scaling laws found in the experiments by Backhaus et al. (Reference Backhaus, Turitsyn and Ecke2011) and Neufeld et al. (Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010) may be related to the porosity or pore-scale factors, such as the pore size
$Sc$
is varied. Our DNS results suggest that the nonlinear scaling laws found in the experiments by Backhaus et al. (Reference Backhaus, Turitsyn and Ecke2011) and Neufeld et al. (Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010) may be related to the porosity or pore-scale factors, such as the pore size
![]() $s$
.
$s$
.

Figure 10. The time and surface (over the wall surface) averaged Sherwood number,
![]() $Sc=250$
. Lines, DOB results; symbols, DNS results. The porosity
$Sc=250$
. Lines, DOB results; symbols, DNS results. The porosity
![]() $\unicode[STIX]{x1D719}$
and scale ratio
$\unicode[STIX]{x1D719}$
and scale ratio
![]() $H/s$
are varied. The results are shown in the log scale (a) and linear scale for
$H/s$
are varied. The results are shown in the log scale (a) and linear scale for
![]() $Ra>5000$
(b).
$Ra>5000$
(b).
It is worth noting that the small Schmidt number (
![]() $Sc=1$
) cases could also be representative of convective heat transfer in a porous medium with a Prandtl number of
$Sc=1$
) cases could also be representative of convective heat transfer in a porous medium with a Prandtl number of
![]() $Pr=1$
, which is common. However, in an experiment for heat transfer, Keene & Goldstein (Reference Keene and Goldstein2015) found a significantly smaller scaling exponent for the Nusselt number,
$Pr=1$
, which is common. However, in an experiment for heat transfer, Keene & Goldstein (Reference Keene and Goldstein2015) found a significantly smaller scaling exponent for the Nusselt number,
![]() $Nu\sim Ra^{0.319}$
. The possible cause of this discrepancy is that conjugate heat transfer may play an important role, as we here assumed that the wall surfaces of the porous elements are adiabatic because we considered mass transfer. Clearly, the effect of conjugate heat transfer on convection in porous media deserves further investigation.
$Nu\sim Ra^{0.319}$
. The possible cause of this discrepancy is that conjugate heat transfer may play an important role, as we here assumed that the wall surfaces of the porous elements are adiabatic because we considered mass transfer. Clearly, the effect of conjugate heat transfer on convection in porous media deserves further investigation.
4.3 Mass concentration and velocity statistics
Figure 11 shows the vertical profiles of the temporally and horizontally averaged macroscopic mass concentration
![]() $\langle \overline{{\hat{c}}}\rangle ^{x1}$
obtained from DOB simulations. Here, the species concentration profiles at different
$\langle \overline{{\hat{c}}}\rangle ^{x1}$
obtained from DOB simulations. Here, the species concentration profiles at different
![]() $Ra$
numbers become almost identical when the distance from the lower wall
$Ra$
numbers become almost identical when the distance from the lower wall
![]() $\hat{x}_{2}$
is normalized by
$\hat{x}_{2}$
is normalized by
![]() $1/Ra$
. This is in accordance with the statement by Huppert & Neufeld (Reference Huppert and Neufeld2014) that the boundary layer thickness is determined by
$1/Ra$
. This is in accordance with the statement by Huppert & Neufeld (Reference Huppert and Neufeld2014) that the boundary layer thickness is determined by
![]() $1/Ra$
. More details of our DOB results can be found in Kränzien & Jin (Reference Kränzien and Jin2019).
$1/Ra$
. More details of our DOB results can be found in Kränzien & Jin (Reference Kränzien and Jin2019).

Figure 11. DOB results. (a) vertical profiles of time- and line-averaged species concentration; (b) root mean square (r.m.s.) of species concentration fluctuation
![]() $\langle {\hat{c}}^{rms}\rangle ^{x1}$
; (c)
$\langle {\hat{c}}^{rms}\rangle ^{x1}$
; (c)
![]() $u_{1}$
fluctuation; (d)
$u_{1}$
fluctuation; (d)
![]() $u_{2}$
fluctuation.
$u_{2}$
fluctuation.
By contrast, the profiles computed by resolving the flow at the pore scale with DNS exhibit a distinct length scale. Figure 12 shows strong deviations from the DNS results if
![]() $\hat{x}_{2}$
is scaled with
$\hat{x}_{2}$
is scaled with
![]() $1/Ra$
. However, similar trends are observed if
$1/Ra$
. However, similar trends are observed if
![]() $\hat{x}_{2}$
is scaled with the pore scale
$\hat{x}_{2}$
is scaled with the pore scale
![]() ${\hat{s}}=s/H$
(figure 13). To explain this, one need to recall that the pore size
${\hat{s}}=s/H$
(figure 13). To explain this, one need to recall that the pore size
![]() $s$
can be approximated by using
$s$
can be approximated by using
![]() $s^{\ast }$
, defined in (3.3) for a general two-dimensional porous matrix. The influence of the bounding walls is generally limited to within the first three REVs next to the walls, which leads to a steep gradient of
$s^{\ast }$
, defined in (3.3) for a general two-dimensional porous matrix. The influence of the bounding walls is generally limited to within the first three REVs next to the walls, which leads to a steep gradient of
![]() $\langle \overline{{\hat{c}}}\rangle ^{x1}$
therein. When the distance from the lower wall is normalized by the pore size
$\langle \overline{{\hat{c}}}\rangle ^{x1}$
therein. When the distance from the lower wall is normalized by the pore size
![]() $s$
, the
$s$
, the
![]() $\langle \overline{{\hat{c}}}\rangle ^{x1}$
obtained at different Rayleigh numbers collapse together. Hence, the boundary layer thickness for convection in porous media is not determined by
$\langle \overline{{\hat{c}}}\rangle ^{x1}$
obtained at different Rayleigh numbers collapse together. Hence, the boundary layer thickness for convection in porous media is not determined by
![]() $Ra$
, but by the pore size
$Ra$
, but by the pore size
![]() $s$
.
$s$
.

Figure 12. Vertical profiles of time- and line-averaged mass concentration for
![]() $Sc=1$
, DNS results. ▫:
$Sc=1$
, DNS results. ▫:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.36$
,
$\unicode[STIX]{x1D719}=0.36$
,
![]() $Ra=5000$
. ○:
$Ra=5000$
. ○:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=5000$
. +:
$Ra=5000$
. +:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=20\,000$
. ×:
$Ra=20\,000$
. ×:
![]() $H/s=50$
,
$H/s=50$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=5000$
.
$Ra=5000$
.

Figure 13. DNS results for
![]() $Sc=1$
. (a) Vertical profiles of time- and line-averaged mass concentration; (b) r.m.s. of mass concentration fluctuation; (c)
$Sc=1$
. (a) Vertical profiles of time- and line-averaged mass concentration; (b) r.m.s. of mass concentration fluctuation; (c)
![]() $u_{1}$
fluctuation; (d)
$u_{1}$
fluctuation; (d)
![]() $u_{2}$
fluctuation. ▫:
$u_{2}$
fluctuation. ▫:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.36,Ra=5000$
. ○:
$\unicode[STIX]{x1D719}=0.36,Ra=5000$
. ○:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=5000$
. +:
$Ra=5000$
. +:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=20\,000$
. ×:
$Ra=20\,000$
. ×:
![]() $H/s=50$
,
$H/s=50$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=5000$
.
$Ra=5000$
.
Figure 13 also shows the r.m.s. macroscopic mass concentration and velocity fluctuations,
![]() $\langle {\hat{c}}^{rms}\rangle ^{x1}$
,
$\langle {\hat{c}}^{rms}\rangle ^{x1}$
,
![]() $\langle \hat{u} _{1}^{rms}\rangle ^{x1}$
, and
$\langle \hat{u} _{1}^{rms}\rangle ^{x1}$
, and
![]() $\langle \hat{u} _{2}^{rms}\rangle ^{x1}$
, respectively, for
$\langle \hat{u} _{2}^{rms}\rangle ^{x1}$
, respectively, for
![]() $Sc=1$
. The velocity fluctuations were normalized with the characteristic velocity,
$Sc=1$
. The velocity fluctuations were normalized with the characteristic velocity,
![]() $u_{m}=\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cgK/\unicode[STIX]{x1D708}$
. The DNS results show that, besides
$u_{m}=\unicode[STIX]{x1D6FD}\unicode[STIX]{x0394}cgK/\unicode[STIX]{x1D708}$
. The DNS results show that, besides
![]() $Ra$
, the scale ratios
$Ra$
, the scale ratios
![]() $H/s$
and porosity
$H/s$
and porosity
![]() $\unicode[STIX]{x1D719}$
also have significant effects on r.m.s. quantities. These effects are absent in DOB simulations. In addition,
$\unicode[STIX]{x1D719}$
also have significant effects on r.m.s. quantities. These effects are absent in DOB simulations. In addition,
![]() $\langle \hat{u} _{1}^{rms}\rangle ^{x1}$
in figure 13(c) is distinctly different from the DOB results, which have unphysical (non-zero) velocity fluctuations at the wall surface (Hewitt et al.
Reference Hewitt, Neufeld and Lister2012; Kränzien & Jin Reference Kränzien and Jin2019). A possible reason for these discrepancies is that momentum dispersion is not accounted for in the DOB equations.
$\langle \hat{u} _{1}^{rms}\rangle ^{x1}$
in figure 13(c) is distinctly different from the DOB results, which have unphysical (non-zero) velocity fluctuations at the wall surface (Hewitt et al.
Reference Hewitt, Neufeld and Lister2012; Kränzien & Jin Reference Kränzien and Jin2019). A possible reason for these discrepancies is that momentum dispersion is not accounted for in the DOB equations.

Figure 14. DNS results for
![]() $Sc=250$
. (a) Vertical profiles of time- and line-averaged mass concentration; (b) r.m.s. of mass concentration fluctuation; (c)
$Sc=250$
. (a) Vertical profiles of time- and line-averaged mass concentration; (b) r.m.s. of mass concentration fluctuation; (c)
![]() $u_{1}$
fluctuation; (d)
$u_{1}$
fluctuation; (d)
![]() $u_{2}$
fluctuation. ▫:
$u_{2}$
fluctuation. ▫:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.36$
,
$\unicode[STIX]{x1D719}=0.36$
,
![]() $Ra=5000$
. ○:
$Ra=5000$
. ○:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=5000$
. +:
$Ra=5000$
. +:
![]() $H/s=20$
,
$H/s=20$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=20\,000$
. ×:
$Ra=20\,000$
. ×:
![]() $H/s=50$
,
$H/s=50$
,
![]() $\unicode[STIX]{x1D719}=0.56$
,
$\unicode[STIX]{x1D719}=0.56$
,
![]() $Ra=20\,000$
.
$Ra=20\,000$
.
Figure 14 shows the temporally and horizontally averaged macroscopic results for
![]() $Sc=250$
. Similar to the results for
$Sc=250$
. Similar to the results for
![]() $Sc=1$
(see figure 13), the influence of the bounding walls is still limited to within the first three REVs next to the walls. Again, our DNS results confirm that the boundary layer thickness for convection in porous media is determined by the pore size
$Sc=1$
(see figure 13), the influence of the bounding walls is still limited to within the first three REVs next to the walls. Again, our DNS results confirm that the boundary layer thickness for convection in porous media is determined by the pore size
![]() $s$
(or
$s$
(or
![]() $s^{\ast }$
for a porous matrix with permeability
$s^{\ast }$
for a porous matrix with permeability
![]() $K$
and porosity
$K$
and porosity
![]() $\unicode[STIX]{x1D719}$
).
$\unicode[STIX]{x1D719}$
).
As the boundary layer thickness is determined by the pore size (which can be characterized by the square root of the permeability,
![]() $\sqrt{K}$
), the momentum dispersion term
$\sqrt{K}$
), the momentum dispersion term
![]() $\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
and the viscous diffusion term
$\unicode[STIX]{x2202}(\unicode[STIX]{x1D719}\langle \text{}^{i}{\tilde{u} _{i}\,}^{i}\tilde{u} _{j}\rangle ^{i})/\unicode[STIX]{x2202}\hat{x}_{j}$
and the viscous diffusion term
![]() $(Sc/\unicode[STIX]{x1D6FE}_{m}Ra)(\unicode[STIX]{x2202}^{2}\hat{u} _{i}/\unicode[STIX]{x2202}\hat{x}_{j}^{2})$
in the macroscopic equation (2.9) are expected to scale as
$(Sc/\unicode[STIX]{x1D6FE}_{m}Ra)(\unicode[STIX]{x2202}^{2}\hat{u} _{i}/\unicode[STIX]{x2202}\hat{x}_{j}^{2})$
in the macroscopic equation (2.9) are expected to scale as
![]() $1/K$
. Thus, the momentum dispersion and viscous diffusion terms should be of order
$1/K$
. Thus, the momentum dispersion and viscous diffusion terms should be of order
![]() $1/Da=H^{2}/K$
, exactly as the Darcy term itself. Thus, our DNS results suggest that the pore scale significantly influences convection in porous media through the momentum dispersion and viscous diffusion terms, which are neglected in the DOB equations. We conclude that these terms cannot be neglected in the macroscopic equations even if the pore size is small. In addition, the pore size should be used as the characteristic length when the dispersion term is modelled.
$1/Da=H^{2}/K$
, exactly as the Darcy term itself. Thus, our DNS results suggest that the pore scale significantly influences convection in porous media through the momentum dispersion and viscous diffusion terms, which are neglected in the DOB equations. We conclude that these terms cannot be neglected in the macroscopic equations even if the pore size is small. In addition, the pore size should be used as the characteristic length when the dispersion term is modelled.
5 Conclusions
Natural convection in a porous medium, made of two-dimensional square obstacles, was studied with DNS by fully resolving the flow field within the pores. Upon comparing our DNS and DOB results, we found significant effects of the pore scale on the macroscopic flow and mass concentration fields. These effects are summarized in what follows.
The boundary layer thickness is not determined by
![]() $1/Ra$
, as DOB simulations suggest (Huppert & Neufeld Reference Huppert and Neufeld2014), but by the pore size. In addition, the sizes of the mega-plumes in the interior region increase with increasing pore size. The DNS exhibit motions with even larger length scales, reaching up to the domain size. This is different from the DOB simulations, where the sizes of mega-plumes in the interior region depend only on the Rayleigh number. Furthermore, note that the spectra of the DNS exhibit much broader peaks and many secondary peaks at larger and smaller wavenumbers, which are entirely missing in the DOB case. Hence, even if the dominant wavenumber appears to converge to the DOB case, it is unclear whether the entire spectrum will converge to it. Overall, pore-scale effects at a low Schmidt number (
$1/Ra$
, as DOB simulations suggest (Huppert & Neufeld Reference Huppert and Neufeld2014), but by the pore size. In addition, the sizes of the mega-plumes in the interior region increase with increasing pore size. The DNS exhibit motions with even larger length scales, reaching up to the domain size. This is different from the DOB simulations, where the sizes of mega-plumes in the interior region depend only on the Rayleigh number. Furthermore, note that the spectra of the DNS exhibit much broader peaks and many secondary peaks at larger and smaller wavenumbers, which are entirely missing in the DOB case. Hence, even if the dominant wavenumber appears to converge to the DOB case, it is unclear whether the entire spectrum will converge to it. Overall, pore-scale effects at a low Schmidt number (
![]() $Sc=1$
) are qualitatively similar to those at a high Schmidt number (
$Sc=1$
) are qualitatively similar to those at a high Schmidt number (
![]() $Sc=250$
).
$Sc=250$
).
The porosity was found to have a strong impact on the mass transfer. At high Rayleigh numbers, increasing the porosity resulted in a lower Sherwood number. More importantly, the
![]() $Sh=f(Ra)$
relationship changed from a linear scaling law (
$Sh=f(Ra)$
relationship changed from a linear scaling law (
![]() $Sh\sim Ra$
) for
$Sh\sim Ra$
) for
![]() $\unicode[STIX]{x1D719}=0.36$
to a nonlinear scaling law (
$\unicode[STIX]{x1D719}=0.36$
to a nonlinear scaling law (
![]() $Sh\sim Ra^{0.8}$
) for
$Sh\sim Ra^{0.8}$
) for
![]() $\unicode[STIX]{x1D719}=0.56$
. This is in accordance with the study of Bérnard–Rayleigh flows without a porous medium. For example, Shishkina & Wagner (Reference Shishkina and Wagner2016) suggested the scaling
$\unicode[STIX]{x1D719}=0.56$
. This is in accordance with the study of Bérnard–Rayleigh flows without a porous medium. For example, Shishkina & Wagner (Reference Shishkina and Wagner2016) suggested the scaling
![]() $Nu\sim Ra^{1/4}$
for large
$Nu\sim Ra^{1/4}$
for large
![]() $Pr$
and
$Pr$
and
![]() $Ra$
numbers. This is also observed in the experiments of Backhaus et al. (Reference Backhaus, Turitsyn and Ecke2011) and Neufeld et al. (Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010). However, this comparison must be taken with caution because the geometrical details of porous matrices used in the experiments are not specified in their studies.
$Ra$
numbers. This is also observed in the experiments of Backhaus et al. (Reference Backhaus, Turitsyn and Ecke2011) and Neufeld et al. (Reference Neufeld, Hesse, Riaz, Hallworth, Techelepi and Huppert2010). However, this comparison must be taken with caution because the geometrical details of porous matrices used in the experiments are not specified in their studies.
One limitation of our results is the relatively small
![]() $H/s\leqslant 100$
values used in the DNS (to keep computational costs manageable), whereas problems such as CO2 sequestration are characterized by very large
$H/s\leqslant 100$
values used in the DNS (to keep computational costs manageable), whereas problems such as CO2 sequestration are characterized by very large
![]() $H/s$
ratios. However, the
$H/s$
ratios. However, the
![]() $H/s$
values in our study ensured that most cases (all cases for
$H/s$
values in our study ensured that most cases (all cases for
![]() $Sc=250$
) were within the Darcy’s regime. Therefore,
$Sc=250$
) were within the Darcy’s regime. Therefore,
![]() $H/s$
only has a mild influence on
$H/s$
only has a mild influence on
![]() $Sh$
without a clear trend within the range of our computational parameters. This suggests that DNS with reduced
$Sh$
without a clear trend within the range of our computational parameters. This suggests that DNS with reduced
![]() $H/s$
values may be used for tackling practical problems such as CO2 sequestration despite a much larger
$H/s$
values may be used for tackling practical problems such as CO2 sequestration despite a much larger
![]() $H/s$
ratio in that situation.
$H/s$
ratio in that situation.
We stress that none of the effects revealed here can be captured by state-of-the-art DOB simulations, where all the microscopic details of the porous media are lumped into the Rayleigh number. Our simulations open avenues for the extension and parametrization of improved DOB equations including non-Darcy terms, accounting for the effect of porosity and the pore scale. More specifically, the momentum dispersion and viscous diffusion terms should be accounted for in the macroscopic equations, even when the pore size is much smaller than the plume size and
![]() $Re_{k}\ll 1$
. The pore size should be used as the characteristic length when the dispersion term is modelled. To achieve this goal, more extensive numerical and experimental studies involving more realistic porous matrices to predict CO2 sequestration are needed.
$Re_{k}\ll 1$
. The pore size should be used as the characteristic length when the dispersion term is modelled. To achieve this goal, more extensive numerical and experimental studies involving more realistic porous matrices to predict CO2 sequestration are needed.
Acknowledgements
The authors gratefully acknowledge the support of this study by the DFG (Deutsche Forschungsgemeinschaft) and the HLRN (North-German Supercomputing Alliance). A.V.K. acknowledges with gratitude the support of the National Science Foundation (award CBET-1642262) and the Alexander von Humboldt Foundation through the Humboldt Research Award.
Declaration of interests
The authors report no conflict of interest.