The Fitting class (of finite, soluble, groups), , is said to be Hall π-closed (where π is a set of primes) if whenever G is a group in and H is a Hall π-subgroup of G, then H belongs to . In this paper, we study the Hall π-closure of products of Fitting classes. Our main result is a characterisation of the Hall π-closedFitting classes of the form 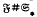(where 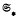denotes the so-called smallest normal Fitting class), subject to a restriction connecting π with the characteristic of 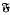. We also characterise those Fitting classes 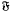(respectively, 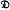) such that 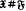(respectively, 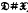) is Hall π-closed for all Fitting classes 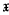. In each case, part of the proof uses a concrete group construction. As a bonus, one of these construction also yields a “cancellation result” for certain products of Fitting classes.

The quaternion algebra of degree 2 over a commutative ring as defined by S. Parimala and R. Sridharan is generalized to a separable cyclic extension B[j] of degree n over a noncommutative ring B. A characterization of such an extension is given, and a relation between Azumaya algebras and Galois extensions for B[j] is also obtained.

The spectral duality theory of H.-E. Porst and M. B. Wischnewsky is examined in more generality, and examples based on topological fields are described.

We show that the classical interpretation of H3(G, A) is equivalent to Taylor's solutions of compound extensions of groups. It is also equivalent to the exactness to an eight term sequence. Only halves of the equivalences are fully shown in the paper but the other halves are clear.

The aim of this note is to investigate the structure of general surjectivity problem for a continuous linear map between locally convex spaces. We shall do so by using the method introduced in Yamamuro (1980). Its basic notion is that of calibrations which has been introduced in Yamamuro (1975), studied in detail in Yamamuro (1979) and appliced to several problems in Yamamuro (1978) and Yamamuro (1979a).

It is well known that in any near-ring, any intersection of prime ideals is a semiprime ideal. The aim of this paper is to prove that any semiprime ideal I in a near-ring N is the intersection of all minimal prime ideals of I in N. As a consequence of this we have any seimprime ideal I is the intersectionof all prime ideals containing I.

We show that the Harris-Sevast'yanov transformation for supercritical Galton-Watson processes with positive extinction probability q can be modified in such a way that the extinction probability of the new process takes any value between 0 and q. We give a probabilistic interpretation for the new process. This note is closely related to Athreya and Ney (1972), Chapter 1.12.

If G is the special orthogonal group O+(V) of a quadratic space V over a finite field of characteristic p, and r is a positive integer, we determine the abelian p-subgroups of largest order in G whose fixed subspaces in V have dimension at least r. In particular, we determine the abelian subgroups of largest order in a Sylow p-subgroup of G, extending some results obtained with different methods by Barry (1979).

The aim of this article is to review the progress made in the last few years in the representation theory of solutions of parabolic systems in the sense of Petrowskii.