1. Introduction
The frequency regime of Terahertz (THz) radiation lies between that of microwaves and optical waves. Because of shortage of effective sources, the THz regime was often considered as a gap in the usable electromagnetic-wave spectrum. Generation and manipulation of THz radiation (or T-rays) have been extensively investigated because of its relevance in many areas of basic science and application. In particular, it has potential use in medical and security-detection imaging and communication, as well as in investigating molecular dynamics in matter in the condensed phase.
Many approaches have been proposed for generating T-rays, including synchrotron radiation [Reference Nakazato, Oyamada and Niimura1], optical transition radiation [Reference Happek, Sievers and Blum2, Reference Leemans, Geddes and Faure3], and nonlinear frequency upconversion [Reference Savage, Joshi and Mori4]. Optical transition radiation is produced by relativistic electrons as they cross the interface of different dielectric media. Frequency up- or downconversion of electromagnetic radiation can be realized through nonlinear wave-matter and wave-wave interaction. THz radiation can also be produced in intense laser interaction with matter [Reference Leemans, Geddes and Faure3, Reference Hamster, Sullivan, Gordon, White and Falcone5, Reference Hamster, Sullivan, Gordon and Falcone6–Reference Hu and Yi14]. T-rays can also be produced by the oscillating electrons in laser-induced wakefields [Reference Cao, Yu and Xu15–Reference Wang, Gibbon, Sheng and Li17], Smith–Purcell effect of the electron beams in laser-matter interactions [Reference Gopal, Woldegeorgis and Herzer18], two-color laser gas-plasma interaction [Reference Magesh Kumar, Kumar, Yuan, Sheng and Chen19, Reference Ahmad, Mahmoud, Purohit and Khan20], transition-Cherenkov effect from laser filaments [Reference Hu, Shen, Lei, Li and Xu21], laser-driven wire-guided helical undulators [Reference Tian, Liu and Bai22], and linear and nonlinear mode conversion [Reference Winner, Schomburg and Brand23], as well as laser interaction with strongly magnetized plasmas [Reference Bakhtiari, Esmaeilzadeh and Ghafary24–Reference Purohit, Rawat and Rawat27]. However, most such sources of T-rays are rather complex and inconvenient to realize in practice.
We consider in this paper an unusual but simple source of T-rays, namely, a plasma cylinder with embedded axial magnetic electric and radial fields. Two-dimensional (2D) particle-in-cell (PIC) simulations show that electrons in the plasma cylinder oscillate in the azimuthal as well as radial directions. The evolution of the oscillating current distribution is investigated. It is found that resonance-like behavior between the electron oscillations near the plasma and cyclotron frequencies can take place, and the resulting current pattern can produce T-rays in the axial direction.
This paper is organized as follows. In Section 2, the proposed scheme and simulation parameters are described. Section 3 presents the simulation results at the initial stage, and Section 4 considers the long-time behavior. The far-field power of the T-ray emitted from the plasma cylinder is given in Section 5. Section 6 gives the summary.
2. Proposed Scheme and Simulation Parameters
We consider here an unusual source of THz radiation, namely, from a plasma cylinder with external radial electric field and axial magnetic field, as shown in Figure 1. The electric field producing the plasma could be from a radial capacitor discharge and the magnetic field from a solenoid coil outside the cylinder. The electron motion is governed by
where u is the electron velocity, γ is the relativistic factor, and e, m, and c are the electron charge and mass, and the speed of light, respectively. The dynamics of both electrons and (hydrogen) ions are fully included in the PIC simulations [Reference Xu, Chang, Zhuo, Cao and Yue28]. However, as expected, within the picosecond time of interest here, the electron motion is dominant. The constant embedded electric and magnetic fields are
![]() and
and
![]() , where E 0 = 3.4 × 1010 V/m and B 0 = 11.4 T, which we note are readily realizable. The cylindrical plasma cylinder is of radius R = 3286 μm and initially of uniform density n 0 = 1.26 × 1020 m−3. The temperature of the electrons and ions is 300 eV. The parameters have been chosen such that the electron cyclotron frequency
, where E 0 = 3.4 × 1010 V/m and B 0 = 11.4 T, which we note are readily realizable. The cylindrical plasma cylinder is of radius R = 3286 μm and initially of uniform density n 0 = 1.26 × 1020 m−3. The temperature of the electrons and ions is 300 eV. The parameters have been chosen such that the electron cyclotron frequency
![]() and plasma frequency
and plasma frequency
![]() are both in the THz regime, so that resonance of the electron oscillations can be expected.
are both in the THz regime, so that resonance of the electron oscillations can be expected.

Figure 1: The plasma cylinder with external radial electric field E 0 and axial magnetic field B 0, where E 0 = 3.4 × 1010 V/m and B 0 = 11.4 T. The (hydrogen) plasma density is n 0 = 1.26 × 1020 m−3.
To investigate the evolution of such a plasma cylinder, we use the 2D3V (two-dimensional in space and three-dimensional in velocity) PIC simulation code LAPINE [Reference Xu, Chang, Zhuo, Cao and Yue28]. The size of the simulation box is 9418 μm × 9418 μm. The spatial mesh has 1024 × 1024 cells, containing 4 × 107 each of electrons and ions, and the simulation time step is 13 fs. As mentioned, the ion dynamics is fully included, as they might play a role in the long-time behavior of the system.
3. Initial Stage of the Evolution
Figure 2 shows the self-consistently induced electric field ΔEr at t = 13.18 ps and t = 16.11 ps. In this beginning stage of the evolution, besides the cyclotron motion, the plasma electrons are driven radially by external electric field E 0 and acquire a radial velocity υ r. The induced electric field ΔEr, of order hundreds V/m, is much weaker than E 0. The Lorentz force from B 0 and υ r also cause the electrons to move in the azimuthal direction, and the resulting azimuthal velocity in turn causes the electrons to move in the radial direction, leading to the oscillations. The much heavier ions have hardly moved (not shown).

Figure 2: Snapshots of the induced electric field ΔEr at (a) t = 13.18 ps and (b) t = 16.11 ps. Note that the induced electric field is much weaker than the embedded electric field.
The evolution of the current density distribution in the early stage, involving about two oscillation periods, is shown in Figure 3. Figure 3(a) shows that, at t = 11.72 ps, the current density near the cylinder center is larger than that near the boundary. Furthermore, the local current vectors (black arrows) indicate that the stronger current is in the anticlockwise direction (note that the electrons near the center rotate in the clockwise direction). For t = 13.18 ps, Figure 3(b) shows that the outward-moving electrons approach the boundary of the circular column. They are then reflected by the sheath there and move inwards toward the center, as can be seen in Figure 3(c). Roughly, Figures 3(a) and 3(c) correspond to near-minimum amplitudes and Figures 3(b) and 3(d) to near-maximum amplitudes, of the current oscillations. The evolution of the current density in Figure 3 shows that besides rotating in the anticlockwise direction, the electrons oscillate in a rather complicated manner.
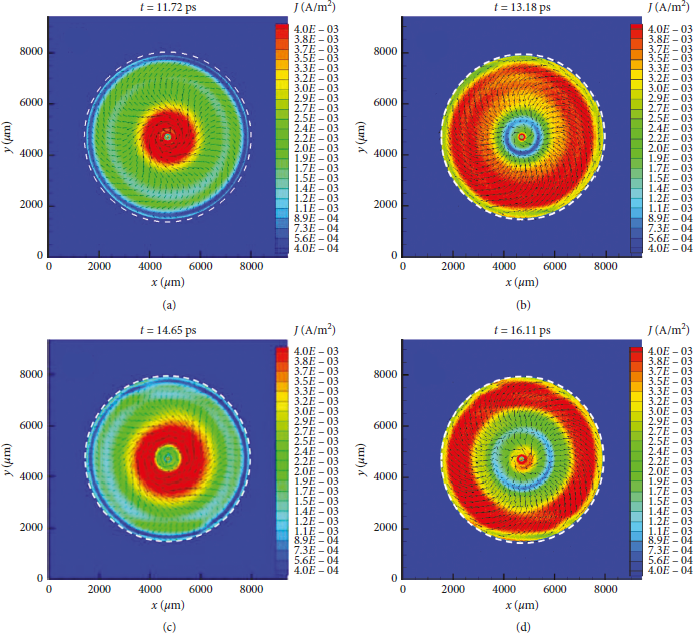
Figure 3: Snapshots of the current distribution at (a) t =11.72, (b)t =13.18, (c) t =14.65, and (d) t =16.11 ps. The black arrows represent local current vectors. Close inspection shows that the currents oscillate both radially and azimuthally. The white dashed circles mark the boundary of the initial plasma cylinder.
4. Long-Time Behavior
Figure 3 shows the electron dynamics in the initial two oscillation cycles. To see the behavior at longer times, we now focus on a fixed location inside the plasma cylinder. Figures 4(a) and 4(c) show the evolution of the radial and azimuthal currents at (x, y) = (7346.04 μm, 7346.04 μm). One can see that, during the 80 ps long interval, the electron currents J r and J φ have undergone many oscillation cycles. Figures 4(b) and 4(d) show the corresponding frequency spectra. We can see in both the radial and azimuthal spectra a dominant peak at f∼0.35 THz (or ω ∼ 2.2 rad/sec). In fact, their overall spectral profiles are almost identical, suggesting that a 2D normal-mode-like structure has formed.
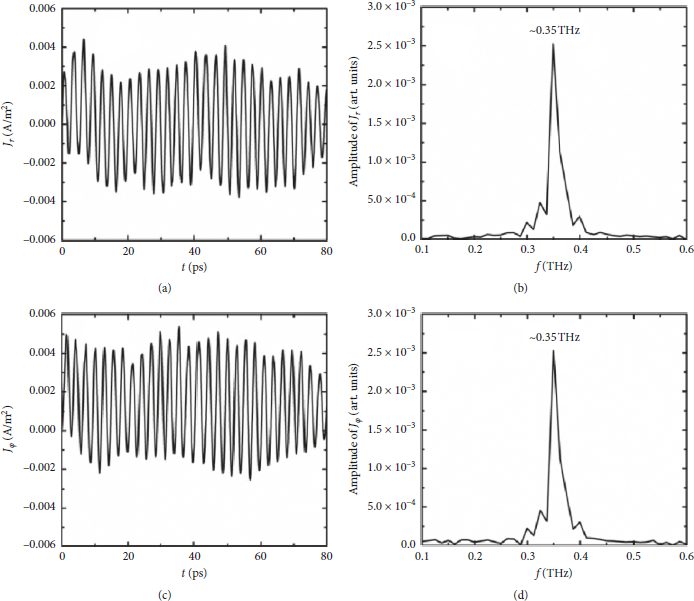
Figure 4: Evolution of the (a) radial current density J r and (c) azimuthal current density J φ at (x, y) = (7346.04 μm, 7346.04 μm). (b, d) The corresponding frequency spectra.
Figure 5 shows the evolution of the total current density
![]() in the simulation box. Figure 5(a) shows that the initial oscillations of the total current density are of large amplitude (to be expected since the initial plasma and fields do not form a self-consistent system), and the corresponding rate of decrease of the envelope is − 4.9 A/m2/ps. At later times, the rate of decrease becomes − 0.0012 A/m2/ps. Figure 5(b) shows the corresponding frequency spectrum, showing that the characteristic frequency of the current oscillations at this location is also ∼0.35 THz.
in the simulation box. Figure 5(a) shows that the initial oscillations of the total current density are of large amplitude (to be expected since the initial plasma and fields do not form a self-consistent system), and the corresponding rate of decrease of the envelope is − 4.9 A/m2/ps. At later times, the rate of decrease becomes − 0.0012 A/m2/ps. Figure 5(b) shows the corresponding frequency spectrum, showing that the characteristic frequency of the current oscillations at this location is also ∼0.35 THz.
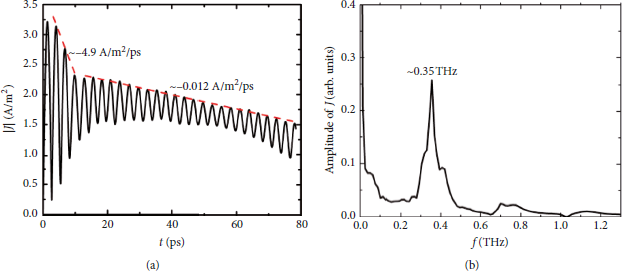
Figure 5: Evolution of the (a) total current density
![]() and (b) its frequency spectrum.
and (b) its frequency spectrum.
5. Terahertz Radiation
Using the temporal and spatial current density from the simulation, one can obtain the far-field radiation. The total electromagnetic energy per unit solid angle Ω per unit frequency is given by the following equation [Reference Cao, Yu and Xu15, Reference Jackson29]:
where
![]() is the direction of the observer with respect to the radiating body. The intensity distribution of the radiation in the z-direction is then [Reference Cao, Yu and Xu15]
is the direction of the observer with respect to the radiating body. The intensity distribution of the radiation in the z-direction is then [Reference Cao, Yu and Xu15]
where
![]() . The corresponding result is shown in Figure 6. We can see that the radiation spectrum has a broad peak centered at f
0∼0.35 THz, as to be expected in view of the current density spectra discussed above. The full-width-at-half-maximum bandwidth Δf/f 0 is about 0.057.
. The corresponding result is shown in Figure 6. We can see that the radiation spectrum has a broad peak centered at f
0∼0.35 THz, as to be expected in view of the current density spectra discussed above. The full-width-at-half-maximum bandwidth Δf/f 0 is about 0.057.
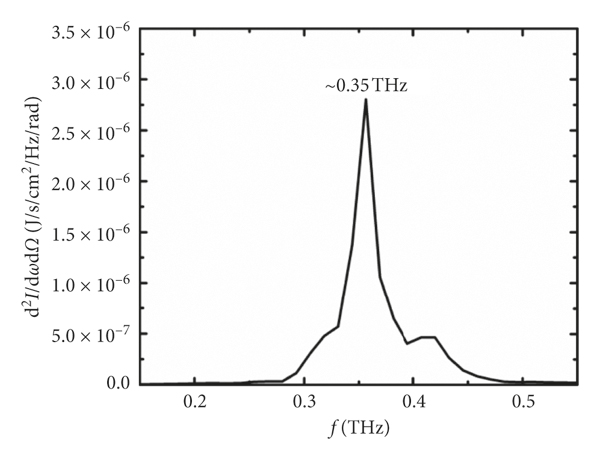
Figure 6: The frequency spectrum of the THz radiation. The peak is at ∼0.35 THz, and the bandwidth is ∼5.7%.
6. Summary
In this paper, we have shown that a bounded plasma cylinder with embedded radial electric and axial magnetic fields can emit T-rays if the parameters of the plasma and the electric and magnetic fields are such that the electron plasma and cyclotron frequencies nearly match and are in the THz regime. As a result, resonance-like behavior of the electrons can occur. The electrons not only rotate in the azimuthal direction but also oscillate in both the radial and azimuthal directions. The resulting currents can emit THz radiation. Finally, it may be of interest to note that, in a nonperturbative analysis of the cold fluid and Maxwell equations, where all the electric and magnetic fields are self-consistent, similar coupled oscillation behavior of the charged particles can exist in an unbounded and self-consistently expanding plasma [Reference Karimov, Stenflo and Yu30].
Data Availability
The data used to support the findings of this study are available from the corresponding author upon reasonable request.
Conflicts of Interest
The authors declare that they have no conflicts of interest.
Acknowledgments
This work was supported by the National Natural Science Foundation of China (Grant no. 11875091), the National K&D Program of China (Grant no. 2016YFA0401100), and the Science and Technology on Plasma Physics Laboratory at CAEP.








