Article contents
Escape components of McMullen maps
Published online by Cambridge University Press: 28 November 2022
Abstract
We consider the McMullen maps 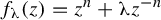 $f_{\unicode{x3bb} }(z)=z^{n}+\unicode{x3bb} z^{-n}$ with
$f_{\unicode{x3bb} }(z)=z^{n}+\unicode{x3bb} z^{-n}$ with 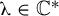 $\unicode{x3bb} \in \mathbb {C}^{*}$ and
$\unicode{x3bb} \in \mathbb {C}^{*}$ and 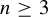 $n \geq 3$. We prove that the closures of escape hyperbolic components are pairwise disjoint and the boundaries of all bounded escape components (the McMullen domain and Sierpiński holes) are quasi-circles with Hausdorff dimension strictly between
$n \geq 3$. We prove that the closures of escape hyperbolic components are pairwise disjoint and the boundaries of all bounded escape components (the McMullen domain and Sierpiński holes) are quasi-circles with Hausdorff dimension strictly between 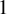 $1$ and
$1$ and 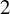 $2$.
$2$.
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
Footnotes
The original version of this article contained an error in the name Pascale Roesch. This error has been corrected. A notice detailing this error has been published.
References
A correction has been issued for this article:
- 1
- Cited by
Linked content
Please note a has been issued for this article.



