1. Introduction
In Rayleigh–Bénard convection (RBC) of smoke laden air (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011; Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014), as shown in figure 1(a), dark plumes are seen when a horizontal laser sheet is passed through a plane parallel and close to the bottom hot plate. A side view of one such dark line in the top view, observed with a vertical laser sheet (see figure 1b), shows boundary layer like, particle-free regions that appear dark, which later turn upwards to give rise to the dark plumes in the top view. The presence of such ‘dust-free’ regions, even though helpful in visualisations, prevents tracer-based measurements of the near-wall phenomena in turbulent convection; a critical requirement for clarifying the phenomenology of turbulent convection. In addition to the fundamental importance of understanding these phenomena, formation and scaling of such dust-free regions near horizontal hot surfaces is important in several industrial applications, like electronic manufacturing (Stratmann et al. Reference Stratmann, Fissan, Papperger and Friedlander1988; Besling, Van der Put & Schoonman Reference Besling, Van der Put and Schoonman1995), heat transfer by nanofluids (Putra, Roetzel & Das Reference Putra, Roetzel and Das2003), soot removal in combustion (Eisner & Rosner Reference Eisner and Rosner1985), aerosol deposition in nuclear reactors (Fernandes & Loyalka Reference Fernandes and Loyalka1996), dust samplers (Wen & Wexler Reference Wen and Wexler2007) and preventing dust deposition on solar photovoltaic cells (Gupta et al. Reference Gupta, Sharma, Pachauri and Babu2019; Oh, Figgis & Rashkeev Reference Oh, Figgis and Rashkeev2020). Many natural phenomena of importance like settling and re-suspension of atmospheric dust particles (Nicholson Reference Nicholson1988), as well as crystal settling in magma chambers (Martin & Nokes Reference Martin and Nokes1988), also depend on an understanding of this phenomenon which occurs near hot surfaces. In this paper, using Lagrangian simulations of a particle-laden natural convection boundary layer on a horizontal hot surface, we show that the particle migration from the hot surface due to the temperature gradient could be one of the possible reasons for the occurrence of such ‘dust-free’ regions near horizontal hot surfaces. We then obtain the scaling for the extent of such dust-free regions above horizontal hot surfaces as a function of the relevant dimensionless numbers.

Figure 1. Images of dust-free regions over horizontal hot surfaces in natural convection: (a) top view of dust-free plumes seen as dark lines in visualisations with a horizontal laser sheet close to the hot plate (Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014); (b) an image that was captured for the present work, showing the side view of two dust-free regions inside the boundary layer turning into a plume.
Migration of small particles away from hot surfaces, known as thermophoresis, is a phenomenon known for over a century (Tyndall Reference Tyndall1870; Aitken Reference Aitken1884); see Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980) for a review. Such a migration, due to the collision between higher kinetic energy gas molecules from the hotter side of the fluid and the particle surfaces, which could give rise to dust-free regions near hot surfaces, has also been known for a long time (Cawood Reference Cawood1936). Extensive studies on particle interaction with shear dominant, forced convection boundary layers have been conducted, often with the intention of obtaining the particle deposition rate for cold surfaces (Homsy, Geyling & Walker Reference Homsy, Geyling and Walker1981; Batchelor & Shen Reference Batchelor and Shen1985; Gokoglu & Rosner Reference Gokoglu and Rosner1986). The extent of the dust-free region over the hot surfaces in such flows were obtained by Goren (Reference Goren1977) by numerically solving the particle concentration equations along with the similarity equations of forced convection boundary layers. They found that for a given thermophoretic coefficient, wall temperature ![]() $T_w$ and Prandtl number
$T_w$ and Prandtl number ![]() $Pr$, the bounding curve of the dust-free space scaled in the same way as the boundary layer thickness; the dependence of the dust-free region on these three parameters was however left undetermined. Measurements of the dust-free region in forced convection by Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980) also showed that the dust-free region thickness
$Pr$, the bounding curve of the dust-free space scaled in the same way as the boundary layer thickness; the dependence of the dust-free region on these three parameters was however left undetermined. Measurements of the dust-free region in forced convection by Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980) also showed that the dust-free region thickness ![]() $\delta _{df}$ is parallel to the boundary layer thickness
$\delta _{df}$ is parallel to the boundary layer thickness ![]() $\delta$, but smaller. Hereinafter, the subscript
$\delta$, but smaller. Hereinafter, the subscript ![]() $df$ indicates the dust-free region. However, in contrast to the observations of Goren (Reference Goren1977), they found
$df$ indicates the dust-free region. However, in contrast to the observations of Goren (Reference Goren1977), they found ![]() $\delta _{df}$ to be independent of
$\delta _{df}$ to be independent of ![]() $T_w$ and the free stream velocity
$T_w$ and the free stream velocity ![]() $U_\infty$. Recently, Oh et al. (Reference Oh, Figgis and Rashkeev2020), in a similar analysis as that of Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980) but including gravitational effects, showed that the particle trajectory deviates from the fluid trajectory and can form dust-free regions over hot surfaces in forced convection, when the temperature differences are sufficient. Clearly, no conclusive scaling of the dust-free region height is yet available even in shear dominant, forced convection boundary layers.
$U_\infty$. Recently, Oh et al. (Reference Oh, Figgis and Rashkeev2020), in a similar analysis as that of Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980) but including gravitational effects, showed that the particle trajectory deviates from the fluid trajectory and can form dust-free regions over hot surfaces in forced convection, when the temperature differences are sufficient. Clearly, no conclusive scaling of the dust-free region height is yet available even in shear dominant, forced convection boundary layers.
When buoyancy becomes dominant in these boundary layers, the dynamics become more involved since the temperature difference drives the flow as well as the thermophoresis; such studies have been limited. Most studies on such natural convection boundary layers limit their analysis to vertical surfaces alone (Zernik Reference Zernik1957; Epstein, Hauser & Henry Reference Epstein, Hauser and Henry1985; Nazaroff & Cass Reference Nazaroff and Cass1987), where the thermophoretic and the gravitational forces are perpendicular to each other, and the gravitational settling does not directly affect the dust-free region. For such natural convection boundary layers over vertical surfaces, using the fluid flow field given by an earlier similarity solution, by balancing the thermophoretic force with the Stokes drag, Zernik (Reference Zernik1957) proposed that the dimensionless dust-free region scaled as ![]() $\eta _{df}=f(T')$, where
$\eta _{df}=f(T')$, where ![]() $\eta _{df}$ is the value of
$\eta _{df}$ is the value of ![]() $\eta$, the similarity variable for the flow, at the dust-free region edge and
$\eta$, the similarity variable for the flow, at the dust-free region edge and ![]() $f(T')$ is a function of the dimensionless temperature difference
$f(T')$ is a function of the dimensionless temperature difference ![]() $T'=2T_w/\Delta {T_w}$, with
$T'=2T_w/\Delta {T_w}$, with ![]() $\Delta {T_w}=T_w-T_\infty$, where
$\Delta {T_w}=T_w-T_\infty$, where ![]() $T_\infty$ is the ambient temperature. Zernik's (Reference Zernik1957) result, however, does not quantify the effects of the ratio of the fluid and the particle thermal conductivities
$T_\infty$ is the ambient temperature. Zernik's (Reference Zernik1957) result, however, does not quantify the effects of the ratio of the fluid and the particle thermal conductivities ![]() $k_f/k_p$ and the Knudsen number
$k_f/k_p$ and the Knudsen number ![]() $Kn=\lambda /a$, where
$Kn=\lambda /a$, where ![]() $\lambda$ is the mean free path of the gas and
$\lambda$ is the mean free path of the gas and ![]() $a$ is the particle radius;
$a$ is the particle radius; ![]() $k_f/k_p$ and
$k_f/k_p$ and ![]() $Kn$ do affect
$Kn$ do affect ![]() $\eta _{df}$ through their influence on the thermophoretic force. Additionally, Zernik's (Reference Zernik1957) result also does not include the effects of Prandtl number
$\eta _{df}$ through their influence on the thermophoretic force. Additionally, Zernik's (Reference Zernik1957) result also does not include the effects of Prandtl number ![]() $Pr=\nu /\alpha$, the ratio of kinematic viscosity of the fluid to its thermal diffusivity.
$Pr=\nu /\alpha$, the ratio of kinematic viscosity of the fluid to its thermal diffusivity.
When such buoyancy dominant boundary layers occur on horizontal surfaces, as in RBC and the other applications mentioned above, the presence or absence of such particle-free regions and the scaling of such regions depend on complex interactions among the gravitational settling, the thermophoretic force exerted by the temperature gradient in the boundary layer, the inertia and the viscous drag exerted by the boundary layer, and for larger and denser particles, on the inertia of the particles. Studies on the interaction of such natural convection boundary layers on horizontal surfaces with particles are rare, in spite of their common occurrence and importance in many industrial applications. In the only available study, assuming similarity, Guha & Samanta (Reference Guha and Samanta2014) solved the boundary layer particle concentration equation along with the natural convection boundary layer equations for a horizontal plate in an Eulerian framework, and showed that when Brownian effects are negligible, in the absence of gravitational settling, at a specific temperature difference, a particle-free region is formed close to the hot surface at a constant value of the similarity variable ![]() $\eta$. However, the study neglected particle inertia, which has been shown to create deviations in particle trajectories from the flow streamlines (Maxey & Corrsin Reference Maxey and Corrsin1986). Further, the assumption of thermophoretic diffusion coefficients and the unrealistic particle concentration boundary condition at the wall, makes their simulations approximate; Guha & Samanta (Reference Guha and Samanta2014) also did not provide a scaling for the particle-free region.
$\eta$. However, the study neglected particle inertia, which has been shown to create deviations in particle trajectories from the flow streamlines (Maxey & Corrsin Reference Maxey and Corrsin1986). Further, the assumption of thermophoretic diffusion coefficients and the unrealistic particle concentration boundary condition at the wall, makes their simulations approximate; Guha & Samanta (Reference Guha and Samanta2014) also did not provide a scaling for the particle-free region.
It is hence clear that scaling of the extent of particle-free regions near horizontal surfaces, where buoyancy is predominant so that natural convection boundary layers form, is poorly understood; such a situation occurs in many applications of importance. In the present work, we study the interaction of spherical point particles with a two-dimensional natural convection boundary layer that forms on a horizontal hot surface. We use a Lagrangian approach of solving the Maxey & Riley (Reference Maxey and Riley1983) equation for small volume fraction of particles, when Brownian motion is negligible, for the flow field given by the similarity solutions of Rotem & Claassen (Reference Rotem and Claassen1969). Since the similarity solutions of Rotem & Claassen (Reference Rotem and Claassen1969) approximate the flow in the local boundary layers on either side of the line plumes on the hot plate in RBC at intermediate Rayleigh numbers (![]() $Ra$) (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005), the present study is expected to model the formation of dust-free regions in the natural convection boundary layer over a hot horizontal plate and in the local boundary layers on the hot plate in RBC, both at intermediate
$Ra$) (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005), the present study is expected to model the formation of dust-free regions in the natural convection boundary layer over a hot horizontal plate and in the local boundary layers on the hot plate in RBC, both at intermediate ![]() $Ra$. By including inertia of the particles and the gravitational forces on them, we obtain realistic particle trajectories from these simulations. The particle trajectories show the formation of particle-free regions close to the hot surface for finite values of the thermophoretic number,
$Ra$. By including inertia of the particles and the gravitational forces on them, we obtain realistic particle trajectories from these simulations. The particle trajectories show the formation of particle-free regions close to the hot surface for finite values of the thermophoretic number,
where ![]() $C_B$ is a particle slip correction factor and
$C_B$ is a particle slip correction factor and ![]() $C_T$ is the thermophoretic coefficient in (2.2), which is a function of
$C_T$ is the thermophoretic coefficient in (2.2), which is a function of ![]() $k_f/k_p$ and
$k_f/k_p$ and ![]() $Kn$, as given by Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980). We show that the longitudinal extent of the dust-free region reduces with increasing ratio of gravitational settling velocity (
$Kn$, as given by Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980). We show that the longitudinal extent of the dust-free region reduces with increasing ratio of gravitational settling velocity (![]() $u_g$) to the characteristic boundary layer velocity (
$u_g$) to the characteristic boundary layer velocity (![]() $U_w$); we define this ratio as the gravitational number
$U_w$); we define this ratio as the gravitational number ![]() $Gn$ in (2.13) and (2.15). With increase in
$Gn$ in (2.13) and (2.15). With increase in ![]() $Gn$, at any given
$Gn$, at any given ![]() $Th$, particles settle beyond decreasing distances. For small values of
$Th$, particles settle beyond decreasing distances. For small values of ![]() $Gn$, when gravitational settling is negligible, by obtaining an expression for a separatrix, which we observe to occur always at the dust-free region boundary, we obtain a scaling for the dust-free region height. The obtained scaling of the dust-free region height shows that the dust-free region boundary is parallel to the boundary layer edge, but smaller in height. The ratio of the dust-free region height to the boundary layer height is shown to be a function of
$Gn$, when gravitational settling is negligible, by obtaining an expression for a separatrix, which we observe to occur always at the dust-free region boundary, we obtain a scaling for the dust-free region height. The obtained scaling of the dust-free region height shows that the dust-free region boundary is parallel to the boundary layer edge, but smaller in height. The ratio of the dust-free region height to the boundary layer height is shown to be a function of ![]() $Th$ and
$Th$ and ![]() $Pr$; we obtain these functions for small, intermediate and large
$Pr$; we obtain these functions for small, intermediate and large ![]() $Pr$.
$Pr$.
The paper is organised as follows. We formulate the dimensionless particle motion equation in § 2.1, where the relevant dimensionless numbers that determine the particle motion are obtained; the limiting case of the equation for small Stokes number (![]() $\widehat {St}$) is discussed in § 2.2. The natural convection boundary layer flow and the temperature fields given by Rotem & Claassen (Reference Rotem and Claassen1969) are briefly discussed in § 2.3, with the numerical method of solution of the particle motion equation discussed in § 2.4. Section 3.1 discusses the particle trajectories and the variation of the extents of the dust-free regions for various finite
$\widehat {St}$) is discussed in § 2.2. The natural convection boundary layer flow and the temperature fields given by Rotem & Claassen (Reference Rotem and Claassen1969) are briefly discussed in § 2.3, with the numerical method of solution of the particle motion equation discussed in § 2.4. Section 3.1 discusses the particle trajectories and the variation of the extents of the dust-free regions for various finite ![]() $Th$ and
$Th$ and ![]() $Gn$, along with the formation of the separatrix at the boundary of the dust-free region. An expression for the separatrix, as well as the scaling for the dust-free region height in the small and the large
$Gn$, along with the formation of the separatrix at the boundary of the dust-free region. An expression for the separatrix, as well as the scaling for the dust-free region height in the small and the large ![]() $\eta$ limits, are obtained for
$\eta$ limits, are obtained for ![]() $Gn \ll Th$ and
$Gn \ll Th$ and ![]() $\widehat {St} \ll 1$ in § 3.3. In § 4, explicit scaling laws for small
$\widehat {St} \ll 1$ in § 3.3. In § 4, explicit scaling laws for small ![]() $Pr$, large
$Pr$, large ![]() $Pr$ and intermediate values of
$Pr$ and intermediate values of ![]() $Pr$ are proposed for the dust-free region height when
$Pr$ are proposed for the dust-free region height when ![]() $Gn=0$, before concluding in § 5.
$Gn=0$, before concluding in § 5.
2. Formulation
2.1. Particle motion equation
We formulate the governing equation for the particle motion in natural convection over hot surfaces by adding thermophoretic force to the Maxey & Riley (Reference Maxey and Riley1983) equation; the corresponding formulation for forced convection was done by Talbot et al. (Reference Talbot, Cheng, Schefer and Willis1980). For high density, micron sized aerosol particles, as the Basset–Boussinesq history force is much smaller compared with the viscous drag term in the dimensionless Maxey & Riley (Reference Maxey and Riley1983) equation, we neglect this history force (Bergougnoux et al. Reference Bergougnoux, Bouchet, Lopez and Guazzelli2014). Since the particle sizes that we consider are small, Faxen's correction, ![]() $(-a^2/6) \nabla ^2u$, to the viscous drag can also be neglected (Maxey & Riley Reference Maxey and Riley1983) in comparison to the terms that are linear in fluid and particle velocities. The governing equation then reduces to
$(-a^2/6) \nabla ^2u$, to the viscous drag can also be neglected (Maxey & Riley Reference Maxey and Riley1983) in comparison to the terms that are linear in fluid and particle velocities. The governing equation then reduces to
 \begin{align} \rho_p \frac{{\rm d}\boldsymbol{v}}{{\rm d}t}&=\rho_f\frac{{\rm D}\boldsymbol{u}}{{\rm D}t}-\frac{9}{2}\frac{\mu}{a^2}\frac{1}{C_B}(\boldsymbol{v}-\boldsymbol{u})- \frac{\rho_f}{2}\left(\frac{{\rm d}\boldsymbol{v}}{{\rm d}t}-\frac{{\rm D}\boldsymbol{u}}{{\rm D}t}\right)\nonumber\\ &\quad -\frac{9\rho_f\nu^2}{a^2}C_T\frac{\boldsymbol{\nabla} T}{\langle T_p\rangle}+(\rho_p-\rho_f)\boldsymbol{g}, \end{align}
\begin{align} \rho_p \frac{{\rm d}\boldsymbol{v}}{{\rm d}t}&=\rho_f\frac{{\rm D}\boldsymbol{u}}{{\rm D}t}-\frac{9}{2}\frac{\mu}{a^2}\frac{1}{C_B}(\boldsymbol{v}-\boldsymbol{u})- \frac{\rho_f}{2}\left(\frac{{\rm d}\boldsymbol{v}}{{\rm d}t}-\frac{{\rm D}\boldsymbol{u}}{{\rm D}t}\right)\nonumber\\ &\quad -\frac{9\rho_f\nu^2}{a^2}C_T\frac{\boldsymbol{\nabla} T}{\langle T_p\rangle}+(\rho_p-\rho_f)\boldsymbol{g}, \end{align}
where the term on the left-hand side is the particle inertia with ![]() ${{\rm d}}/{{\rm d}t}= {\partial }/{\partial t} + \boldsymbol{v}\boldsymbol{\cdot } \boldsymbol{\nabla }$ being the material derivative along the particle trajectory and
${{\rm d}}/{{\rm d}t}= {\partial }/{\partial t} + \boldsymbol{v}\boldsymbol{\cdot } \boldsymbol{\nabla }$ being the material derivative along the particle trajectory and ![]() $\boldsymbol{v}$ the particle velocity vector. In the first term on the right in (2.1),
$\boldsymbol{v}$ the particle velocity vector. In the first term on the right in (2.1), ![]() ${{\rm D}}/{{\rm D}t} = {\partial }/{\partial t} + \boldsymbol{u}\boldsymbol{\cdot }\boldsymbol{\nabla }$ is the material derivative along the fluid trajectory, with
${{\rm D}}/{{\rm D}t} = {\partial }/{\partial t} + \boldsymbol{u}\boldsymbol{\cdot }\boldsymbol{\nabla }$ is the material derivative along the fluid trajectory, with ![]() $\boldsymbol{u}$ being the fluid velocity vector. The second term on the right-hand side of (2.1) is the viscous drag where
$\boldsymbol{u}$ being the fluid velocity vector. The second term on the right-hand side of (2.1) is the viscous drag where ![]() $C_B$ is any appropriate slip correction factor, such as Basset or Cunningham slip correction factors, to accommodate non-continuum effects, and
$C_B$ is any appropriate slip correction factor, such as Basset or Cunningham slip correction factors, to accommodate non-continuum effects, and ![]() $\mu$ is the dynamic viscosity of the fluid. The third term on the right-hand side of (2.1) is the added mass, where
$\mu$ is the dynamic viscosity of the fluid. The third term on the right-hand side of (2.1) is the added mass, where ![]() $\rho _f$ is the fluid density. The next term on the right is the thermophoretic force term, where
$\rho _f$ is the fluid density. The next term on the right is the thermophoretic force term, where ![]() $T$ is the fluid temperature field and
$T$ is the fluid temperature field and ![]() $\langle T_p \rangle$ the undisturbed fluid temperature at the particle centre if the particle was not present at that point. The thermophoretic coefficient in this term is
$\langle T_p \rangle$ the undisturbed fluid temperature at the particle centre if the particle was not present at that point. The thermophoretic coefficient in this term is
with the coefficients ![]() $C_m=1.14$,
$C_m=1.14$, ![]() $C_s=1.17$ and
$C_s=1.17$ and ![]() $C_t=2.18$ (Talbot et al. Reference Talbot, Cheng, Schefer and Willis1980);
$C_t=2.18$ (Talbot et al. Reference Talbot, Cheng, Schefer and Willis1980); ![]() $k_f$ and
$k_f$ and ![]() $k_p$ being the fluid and particle thermal conductivities. An alternative expression for
$k_p$ being the fluid and particle thermal conductivities. An alternative expression for ![]() $C_T$ in terms of
$C_T$ in terms of ![]() $Kn$ and
$Kn$ and ![]() $k_f/k_p$, given by Beresnev & Chernyak (Reference Beresnev and Chernyak1995), which was shown to be more accurate in the transition regime of Knudsen numbers by Guha & Samanta (Reference Guha and Samanta2014), could also be used instead of (2.2). The last term in (2.1) is the buoyancy acting on the particle, where
$k_f/k_p$, given by Beresnev & Chernyak (Reference Beresnev and Chernyak1995), which was shown to be more accurate in the transition regime of Knudsen numbers by Guha & Samanta (Reference Guha and Samanta2014), could also be used instead of (2.2). The last term in (2.1) is the buoyancy acting on the particle, where ![]() $\boldsymbol{g}$ is the acceleration vector due to gravity and
$\boldsymbol{g}$ is the acceleration vector due to gravity and ![]() $\rho _p$ the particle density.
$\rho _p$ the particle density.
We normalise (2.1) with the characteristic scales near the hot plate in RBC (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005), namely, the near-wall length scale
 \begin{equation} Z_w = \left(\frac{\nu \alpha}{g\beta \Delta T_w} \right)^{1/3}, \end{equation}
\begin{equation} Z_w = \left(\frac{\nu \alpha}{g\beta \Delta T_w} \right)^{1/3}, \end{equation}
where ![]() $\beta$ is the thermal expansivity of the fluid, the near-wall velocity scale
$\beta$ is the thermal expansivity of the fluid, the near-wall velocity scale
and the near-wall time scale
The dimensionless governing equation for particle motion then becomes
where all the starred variables denote the dimensionless variables corresponding to the dimensional variables in (2.1), ![]() $\epsilon = \rho _f/\rho _p$ and the dimensionless temperature,
$\epsilon = \rho _f/\rho _p$ and the dimensionless temperature,
Equation (2.6) shows that the following dimensionless numbers determine the particle dynamics. The modified Stokes number, ![]() $\widehat {St}$ in (2.6) is
$\widehat {St}$ in (2.6) is
where the Stokes number
by using (2.5), (2.3) and (2.4). Here, the particle Rayleigh number,
 \begin{equation} Ra_p = \left(\frac{g\beta\Delta T_w a^3}{\nu \alpha}\right)^{1/3}, \end{equation}
\begin{equation} Ra_p = \left(\frac{g\beta\Delta T_w a^3}{\nu \alpha}\right)^{1/3}, \end{equation}
is the ratio of the time scales of thermal transport by diffusion (![]() $a^2/\sqrt {\nu \alpha })$ to the thermal transport by convection
$a^2/\sqrt {\nu \alpha })$ to the thermal transport by convection ![]() $(\sqrt {\nu \alpha }/g \beta \Delta T_w a)$, both evaluated at the particle length scale. The thermophoretic number,
$(\sqrt {\nu \alpha }/g \beta \Delta T_w a)$, both evaluated at the particle length scale. The thermophoretic number,
in (2.6) represents the dimensionless strength of the thermophoretic effects on the particle, where the Reynolds number
by using (2.3) and (2.4). In (2.6),
 \begin{equation} Gn = \frac{C_B(1-\epsilon)St}{Fr^2} = \frac{2(1-\epsilon)\widehat{St}}{(2+\epsilon)Fr^2} \end{equation}
\begin{equation} Gn = \frac{C_B(1-\epsilon)St}{Fr^2} = \frac{2(1-\epsilon)\widehat{St}}{(2+\epsilon)Fr^2} \end{equation}is the gravitational number, where the Froude number
By using (2.8), (2.9) and (2.14) in (2.13),
 \begin{equation} Gn = \frac{C_B\dfrac{4}{3}{\rm \pi} a^3 (\rho_p-\rho_f)g}{6{\rm \pi} \mu a} \frac{1}{U_w} = \frac{u_{g}}{U_w} = {u^*_{g}}, \end{equation}
\begin{equation} Gn = \frac{C_B\dfrac{4}{3}{\rm \pi} a^3 (\rho_p-\rho_f)g}{6{\rm \pi} \mu a} \frac{1}{U_w} = \frac{u_{g}}{U_w} = {u^*_{g}}, \end{equation}
where ![]() $u^*_{g}$ is the dimensionless gravitational settling velocity and
$u^*_{g}$ is the dimensionless gravitational settling velocity and ![]() $u_g$ the gravitational settling velocity. Typical values of these dimensionless numbers for olive oil droplets in air are tabulated in table 1 for different diameters of droplets. We note that
$u_g$ the gravitational settling velocity. Typical values of these dimensionless numbers for olive oil droplets in air are tabulated in table 1 for different diameters of droplets. We note that ![]() $\widehat {St} \ll 1$ for the common droplet sizes in aerosols. We have ignored the role of particle interactions and inertial lift forces in the present formulation, effects that could lead to the modification of particle trajectories. Both hydrodynamic and non-hydrodynamic interactions between aerosol particles can play a significant role in the trajectories of aerosol particles, which subsequently influences phenomena like coagulation and deposition (see Patra, Koch & Roy Reference Patra, Koch and Roy2022 and references therein). In the present study, we are interested in the highly dilute limit; thus, the role of interactions is neglected. A particle has a non-zero slip with respect to the local fluid velocity; for small
$\widehat {St} \ll 1$ for the common droplet sizes in aerosols. We have ignored the role of particle interactions and inertial lift forces in the present formulation, effects that could lead to the modification of particle trajectories. Both hydrodynamic and non-hydrodynamic interactions between aerosol particles can play a significant role in the trajectories of aerosol particles, which subsequently influences phenomena like coagulation and deposition (see Patra, Koch & Roy Reference Patra, Koch and Roy2022 and references therein). In the present study, we are interested in the highly dilute limit; thus, the role of interactions is neglected. A particle has a non-zero slip with respect to the local fluid velocity; for small ![]() $St$, the deviation is due to the thermophoretic velocity (to be discussed in detail in the next section). Since a particle has a non-zero slip velocity in a local shear flow, it could experience an inertial lift force as was derived in the case of a simple shear flow by Saffman (Reference Saffman1965) (see Candelier, Mehlig & Magnaudet Reference Candelier, Mehlig and Magnaudet2019 for more extensions to more general linear flows). The relative magnitude of the Saffman lift force would depend on the particle Reynolds number defined based on the local shear rate,
$St$, the deviation is due to the thermophoretic velocity (to be discussed in detail in the next section). Since a particle has a non-zero slip velocity in a local shear flow, it could experience an inertial lift force as was derived in the case of a simple shear flow by Saffman (Reference Saffman1965) (see Candelier, Mehlig & Magnaudet Reference Candelier, Mehlig and Magnaudet2019 for more extensions to more general linear flows). The relative magnitude of the Saffman lift force would depend on the particle Reynolds number defined based on the local shear rate, ![]() $Re_{\gamma }$. In the present problem,
$Re_{\gamma }$. In the present problem, ![]() $Re_{\gamma }=Ra_p^2/\sqrt {Pr}$. From the listed values of
$Re_{\gamma }=Ra_p^2/\sqrt {Pr}$. From the listed values of ![]() $Ra_p$ in table 1, we see
$Ra_p$ in table 1, we see ![]() $Re_{\gamma }\ll 1$ and thus, the effect of inertial lift is negligible in the present study. This assumption is further corroborated by Yang et al. (Reference Yang, Zhang, Wang, Dong and Zhou2022) where they found the effect of Saffman lift negligible in the study of particle dynamics in Rayleigh–Bénard convection.
$Re_{\gamma }\ll 1$ and thus, the effect of inertial lift is negligible in the present study. This assumption is further corroborated by Yang et al. (Reference Yang, Zhang, Wang, Dong and Zhou2022) where they found the effect of Saffman lift negligible in the study of particle dynamics in Rayleigh–Bénard convection.
Table 1. Values of the various dimensionless numbers for olive oil droplets in air at ![]() $Pr=0.7$ for
$Pr=0.7$ for ![]() $\Delta T_w \approx 10$ K and
$\Delta T_w \approx 10$ K and ![]() $\langle T\rangle \approx 300$ K.
$\langle T\rangle \approx 300$ K.

2.2. Limiting cases
When ![]() $\widehat {St} \ll 1$, the first term on the right-hand side of (2.6) can be neglected since
$\widehat {St} \ll 1$, the first term on the right-hand side of (2.6) can be neglected since ![]() $1/\widehat {St}$ appears on all the other terms on the right-hand side of (2.6). Then, since
$1/\widehat {St}$ appears on all the other terms on the right-hand side of (2.6). Then, since ![]() $\boldsymbol{v}^* = {\rm d}\kern0.07em\boldsymbol{x}^*/{\rm d}t^*$, for
$\boldsymbol{v}^* = {\rm d}\kern0.07em\boldsymbol{x}^*/{\rm d}t^*$, for ![]() $\widehat {St} \ll 1$, (2.6) reduces to
$\widehat {St} \ll 1$, (2.6) reduces to
In (2.16), ![]() $\widehat {St}$ can approach zero only if
$\widehat {St}$ can approach zero only if ![]() $St$ is approaching zero, as shown by (2.8). Hence, when
$St$ is approaching zero, as shown by (2.8). Hence, when ![]() $\widehat {St} \rightarrow 0$, since both
$\widehat {St} \rightarrow 0$, since both ![]() $\widehat {St}$ and
$\widehat {St}$ and ![]() $Gn$ have a similar dependence on
$Gn$ have a similar dependence on ![]() $St$, as evident from (2.8) and (2.13),
$St$, as evident from (2.8) and (2.13), ![]() $Gn/\widehat {St}$ can still remain finite depending on the values that
$Gn/\widehat {St}$ can still remain finite depending on the values that ![]() $\epsilon$ and
$\epsilon$ and ![]() $Fr^2$ take. So when
$Fr^2$ take. So when ![]() $\widehat {St} \rightarrow 0$ (or
$\widehat {St} \rightarrow 0$ (or ![]() $St=0$), the first and the last terms in (2.16) are negligible whereas the second and the third terms remain finite. Further, the term
$St=0$), the first and the last terms in (2.16) are negligible whereas the second and the third terms remain finite. Further, the term ![]() $-Th\nabla ^*{\theta }$ in (2.16) can be re-written by using (2.12), (2.11) and (2.7) as
$-Th\nabla ^*{\theta }$ in (2.16) can be re-written by using (2.12), (2.11) and (2.7) as
where ![]() $\boldsymbol{u}_{t}$ is the thermophoretic velocity and
$\boldsymbol{u}_{t}$ is the thermophoretic velocity and ![]() $\boldsymbol{u}^*_{t}$ its dimensionless form. Hence, when
$\boldsymbol{u}^*_{t}$ its dimensionless form. Hence, when ![]() $\widehat {St} \rightarrow 0$, (2.16) reduces to
$\widehat {St} \rightarrow 0$, (2.16) reduces to
the zeroth-order equation for the particle velocity. Equation (2.18) implies that when ![]() $\widehat {St} \rightarrow 0$, the particle velocity in fluid flows with finite temperature gradients, like thermal boundary layers and plumes, is a vector sum of the fluid velocity and the thermophoretic velocity. Note that this result holds even when
$\widehat {St} \rightarrow 0$, the particle velocity in fluid flows with finite temperature gradients, like thermal boundary layers and plumes, is a vector sum of the fluid velocity and the thermophoretic velocity. Note that this result holds even when ![]() $\epsilon \rightarrow 1$ for neutrally buoyant particles, usually employed in particle image velocimetry (PIV) studies. Therefore, even when
$\epsilon \rightarrow 1$ for neutrally buoyant particles, usually employed in particle image velocimetry (PIV) studies. Therefore, even when ![]() $St = 0$, neutrally buoyant particles with
$St = 0$, neutrally buoyant particles with ![]() $\epsilon = 1$ do not follow the fluid trajectory in regions where
$\epsilon = 1$ do not follow the fluid trajectory in regions where ![]() $\boldsymbol{u}^*_{t}$ is significant. Hence, PIV measurements inside regions like thermal boundary layers and plumes may not be reliable if
$\boldsymbol{u}^*_{t}$ is significant. Hence, PIV measurements inside regions like thermal boundary layers and plumes may not be reliable if ![]() $\boldsymbol{u}^*_{t}$ values there are not negligible. It is obvious from (2.16) that particles follow the fluid trajectory when
$\boldsymbol{u}^*_{t}$ values there are not negligible. It is obvious from (2.16) that particles follow the fluid trajectory when ![]() $Gn \ll 1$,
$Gn \ll 1$, ![]() $Th \ll 1$ and
$Th \ll 1$ and ![]() $\widehat {St} = 0$, since
$\widehat {St} = 0$, since ![]() $\boldsymbol{v}^* = \boldsymbol{u}^*$ in such a case. In all other cases, the particles would deviate from fluid trajectories under the influence of thermophoretic and gravitational forces, even when
$\boldsymbol{v}^* = \boldsymbol{u}^*$ in such a case. In all other cases, the particles would deviate from fluid trajectories under the influence of thermophoretic and gravitational forces, even when ![]() $St = 0$.
$St = 0$.
Since the fluid velocity field and the temperature field that we consider are steady, it can be noted from (2.18) that the particle velocity field also approaches a steady state as ![]() $\widehat {St}\rightarrow 0$. This is because in such a case, when
$\widehat {St}\rightarrow 0$. This is because in such a case, when ![]() $\widehat {St}\rightarrow 0$, the response time required for the particles to reach the velocity of the flow field becomes infinitely small due to the low inertia and hence, the time evolution of the particles in the unsteady state can be neglected in such a case. Then, the initial injection velocities of particles into the flow field become irrelevant in such cases.
$\widehat {St}\rightarrow 0$, the response time required for the particles to reach the velocity of the flow field becomes infinitely small due to the low inertia and hence, the time evolution of the particles in the unsteady state can be neglected in such a case. Then, the initial injection velocities of particles into the flow field become irrelevant in such cases.
2.3. Fluid flow field
We assume that the particles do not modify the flow around them, which is valid for the case of a dilute mixture of small particles, when mass loading of the particle phase is low (Guha Reference Guha2008). It is shown in Appendix A that those conditions are valid in the present study. This assumption allows us to substitute an existing solution of natural convection boundary layers for ![]() $\boldsymbol{u}^*(x,y)$ and
$\boldsymbol{u}^*(x,y)$ and ![]() $\theta (x,y)$ in (2.16) to study the evolution of particles in that flow field. The natural convection boundary layer flow above a horizontal surface has been obtained by Rotem & Claassen (Reference Rotem and Claassen1969), Pera & Gebhart (Reference Pera and Gebhart1973) and Samanta & Guha (Reference Samanta and Guha2012); we here use the similarity solutions proposed by Rotem & Claassen (Reference Rotem and Claassen1969).
$\theta (x,y)$ in (2.16) to study the evolution of particles in that flow field. The natural convection boundary layer flow above a horizontal surface has been obtained by Rotem & Claassen (Reference Rotem and Claassen1969), Pera & Gebhart (Reference Pera and Gebhart1973) and Samanta & Guha (Reference Samanta and Guha2012); we here use the similarity solutions proposed by Rotem & Claassen (Reference Rotem and Claassen1969).
Rotem & Claassen (Reference Rotem and Claassen1969) gave the dimensionless stream function,
and the dimensionless temperature difference,
as functions of the similarity variable
Here, the dimensionless ![]() $x$ coordinate,
$x$ coordinate,
where ![]() $L$ is the length of the plate. The stretched
$L$ is the length of the plate. The stretched ![]() $y$ coordinate,
$y$ coordinate,
where the Grashoff number
where ![]() $Ra_L = (-g\beta \Delta TL^3)/\nu \alpha$ is the Rayleigh number based on
$Ra_L = (-g\beta \Delta TL^3)/\nu \alpha$ is the Rayleigh number based on ![]() $L$, so that, using (2.21), (2.22), (2.23) and (2.24),
$L$, so that, using (2.21), (2.22), (2.23) and (2.24),
 \begin{equation} \eta = \frac{y}{L}\left(\frac{x}{L}\right)^{{-}2/5}\left(\frac{Ra_L}{Pr}\right)^{1/5}= \frac{y}{(x^2Z_w^3)^{1/5}}\frac{1}{Pr}. \end{equation}
\begin{equation} \eta = \frac{y}{L}\left(\frac{x}{L}\right)^{{-}2/5}\left(\frac{Ra_L}{Pr}\right)^{1/5}= \frac{y}{(x^2Z_w^3)^{1/5}}\frac{1}{Pr}. \end{equation}From Rotem & Claassen's (Reference Rotem and Claassen1969) stretched horizontal and vertical velocity components, given in (D3) and (D4), we obtain the dimensionless velocity components for the present study as
and
by using (D1), (B1), (B2), (2.3) and (2.4).
Values of ![]() $F(\eta )$ from (2.19) and
$F(\eta )$ from (2.19) and ![]() $H(\eta )$ from (2.20) for various
$H(\eta )$ from (2.20) for various ![]() $Pr$ are given by Rotem & Claassen (Reference Rotem and Claassen1969) by numerical solution of the natural convection boundary layer equations (2.28). The values of
$Pr$ are given by Rotem & Claassen (Reference Rotem and Claassen1969) by numerical solution of the natural convection boundary layer equations (2.28). The values of ![]() $\eta$, and hence the values of the velocity components (2.26), (2.27) and the temperatures (2.20) at any location inside the boundary layer are then determined by the values of
$\eta$, and hence the values of the velocity components (2.26), (2.27) and the temperatures (2.20) at any location inside the boundary layer are then determined by the values of ![]() $Ra_L$ and
$Ra_L$ and ![]() $Pr$ through (2.25). Here,
$Pr$ through (2.25). Here, ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$ are obtained by numerically solving the following boundary layer equations of Rotem & Claassen (Reference Rotem and Claassen1969),
$H(\eta )$ are obtained by numerically solving the following boundary layer equations of Rotem & Claassen (Reference Rotem and Claassen1969),
and
where ![]() $'$ indicates derivative with respect to
$'$ indicates derivative with respect to ![]() $\eta$. Additionally,
$\eta$. Additionally,
is the dimensionless pressure function, where ![]() $p$ is the local fluid pressure,
$p$ is the local fluid pressure, ![]() $p_{\infty }$ the pressure of the ambient fluid far away from the plate and
$p_{\infty }$ the pressure of the ambient fluid far away from the plate and ![]() $\rho _{\infty }$ the corresponding density. The boundary conditions for solving (2.28) include
$\rho _{\infty }$ the corresponding density. The boundary conditions for solving (2.28) include
and
2.4. Numerical method
Numerical solutions of (2.16) were obtained for 5000 particles, initially placed randomly in the domain with zero initial velocity at ![]() $\widehat {St} = 10^{-5}$ for different combinations of
$\widehat {St} = 10^{-5}$ for different combinations of ![]() $Gn$ and
$Gn$ and ![]() $Th$. The horizontal domain started at
$Th$. The horizontal domain started at ![]() $x = 0.01Z_w$ (or
$x = 0.01Z_w$ (or ![]() $x^* = 0.01$) to exclude the origin of the boundary layer, which is a singular point. The domain extended horizontally up to
$x^* = 0.01$) to exclude the origin of the boundary layer, which is a singular point. The domain extended horizontally up to ![]() $50Z_w$, approximately the average length of a growing boundary layer before it turns into a plume (Theerthan & Arakeri Reference Theerthan and Arakeri1998). In the vertical direction, since the boundary layer thickness
$50Z_w$, approximately the average length of a growing boundary layer before it turns into a plume (Theerthan & Arakeri Reference Theerthan and Arakeri1998). In the vertical direction, since the boundary layer thickness ![]() $\delta \sim Z_w$, the domain extended from
$\delta \sim Z_w$, the domain extended from ![]() $y=0$ to
$y=0$ to ![]() $y=40Z_w$ to ensure that both the thermal and the velocity boundary layers were well within the domain.
$y=40Z_w$ to ensure that both the thermal and the velocity boundary layers were well within the domain.
To maintain a constant number of particles in the domain, whenever a particle exited, another particle was introduced through the top of the domain at a random ![]() $x$ position with zero velocity. As discussed in § 2.2, the initial velocities of the particles become insignificant when
$x$ position with zero velocity. As discussed in § 2.2, the initial velocities of the particles become insignificant when ![]() $\widehat {St} \ll 1$, as they reach the surrounding fluid velocity in a negligible amount of time. Therefore, the initial velocities at the start of the computation and the injection velocities of particles through the top boundary were set to zero for convenience.
$\widehat {St} \ll 1$, as they reach the surrounding fluid velocity in a negligible amount of time. Therefore, the initial velocities at the start of the computation and the injection velocities of particles through the top boundary were set to zero for convenience.
Equation (2.16) was numerically solved using the fifth-order Runge–Kutta method with adaptive step size selection, implemented via the ODE45 tool in MATLAB![]() $\circledR$. The particles evolved in the steady flow field described by (2.26), (2.27) and (2.20). To solve (2.16) for particle positions,
$\circledR$. The particles evolved in the steady flow field described by (2.26), (2.27) and (2.20). To solve (2.16) for particle positions, ![]() $u_x^*$ in (2.26),
$u_x^*$ in (2.26), ![]() $u_y^*$ in (2.27) and
$u_y^*$ in (2.27) and ![]() $\theta$ in (2.20) were obtained by interpolating the solutions of the boundary layer equations (2.28). For efficient implementation of interpolation, (2.16) was integrated using a curve-fitted form of
$\theta$ in (2.20) were obtained by interpolating the solutions of the boundary layer equations (2.28). For efficient implementation of interpolation, (2.16) was integrated using a curve-fitted form of ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$, which were used to obtain
$H(\eta )$, which were used to obtain ![]() $u^*_x$ in (2.26),
$u^*_x$ in (2.26), ![]() $u^*_y$ in (2.27) and
$u^*_y$ in (2.27) and ![]() $\theta$ in (2.20). The fits for
$\theta$ in (2.20). The fits for ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$ are shown in figure 2.
$H(\eta )$ are shown in figure 2.

Figure 2. Comparison of the curve fits obtained for ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$ to the data points obtained from Rotem & Claassen (Reference Rotem and Claassen1969) for
$H(\eta )$ to the data points obtained from Rotem & Claassen (Reference Rotem and Claassen1969) for ![]() $Pr=0.7$. Data points from Rotem & Claassen (Reference Rotem and Claassen1969) for:
$Pr=0.7$. Data points from Rotem & Claassen (Reference Rotem and Claassen1969) for: ![]() $\triangledown$,
$\triangledown$, ![]() $F(\eta )$; and red
$F(\eta )$; and red ![]() $\triangledown$,
$\triangledown$, ![]() $H(\eta )$. Curve fits for: black – – –,
$H(\eta )$. Curve fits for: black – – –, ![]() $F(\eta )$; and blue – – –,
$F(\eta )$; and blue – – –, ![]() $H(\eta )$.
$H(\eta )$.
The ODE45 algorithm automatically selects smaller time steps where the solutions change abruptly, ensuring the accuracy of the solution based on the specified tolerance. The current simulations were conducted with a relative tolerance of ![]() $10^{-6}$. Solving the trajectory of a particle from one end of the domain to the other took approximately 5 minutes. Since each particle trajectory could be independently solved using multiple threads in parallel, the computational time was drastically reduced.
$10^{-6}$. Solving the trajectory of a particle from one end of the domain to the other took approximately 5 minutes. Since each particle trajectory could be independently solved using multiple threads in parallel, the computational time was drastically reduced.
The curve fits in figure 2 for ![]() $Pr = 0.7$ were used only to obtain the particle dynamics discussed in § 3. The scaling laws in the subsequent sections were derived using
$Pr = 0.7$ were used only to obtain the particle dynamics discussed in § 3. The scaling laws in the subsequent sections were derived using ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$ obtained from numerical solutions of the boundary layer equations in (2.28).
$H(\eta )$ obtained from numerical solutions of the boundary layer equations in (2.28).
3. Particle dynamics
3.1. Flow streamlines and particle trajectories
We analysed the dynamics of particles inside the boundary layer at ![]() $\widehat {St}=10^{-5}$ for different combinations of
$\widehat {St}=10^{-5}$ for different combinations of ![]() $Gn$ and
$Gn$ and ![]() $Th$. Figure 3(a) shows the particle positions and fluid's streamlines, while figure 3(b) shows the particle trajectories and fluid streamlines corresponding to
$Th$. Figure 3(a) shows the particle positions and fluid's streamlines, while figure 3(b) shows the particle trajectories and fluid streamlines corresponding to ![]() $Gn=0$ and
$Gn=0$ and ![]() $Th=1$ at
$Th=1$ at ![]() $t^*=45$. Figure 3(c,d) shows the same at
$t^*=45$. Figure 3(c,d) shows the same at ![]() ${Gn=0.14}$ and
${Gn=0.14}$ and ![]() $Th=1$ at
$Th=1$ at ![]() $t^*=60$. The corresponding motion of particles can be clearly viewed in the supplementary movies for the cases with and without gravity. The particle trajectories in figure 3(b,d) and the motion of the particles in the two videos corresponding to them show that the particles at the top of the domain are initially dragged down by the entrainment fluid flow into the boundary layer. For the low-gravitational-force situations, as in figure 3(a), once the particles enter the boundary layer, they mostly take a right turn before finally exiting the domain through the right end. For cases when
$t^*=60$. The corresponding motion of particles can be clearly viewed in the supplementary movies for the cases with and without gravity. The particle trajectories in figure 3(b,d) and the motion of the particles in the two videos corresponding to them show that the particles at the top of the domain are initially dragged down by the entrainment fluid flow into the boundary layer. For the low-gravitational-force situations, as in figure 3(a), once the particles enter the boundary layer, they mostly take a right turn before finally exiting the domain through the right end. For cases when ![]() $Gn$ is non-negligible compared with
$Gn$ is non-negligible compared with ![]() $Th$, as in figure 3(c), many of the particles settle on the plate, as they move to the right; the location of settling depends on the relative strength of the gravitational force that the particles experience.
$Th$, as in figure 3(c), many of the particles settle on the plate, as they move to the right; the location of settling depends on the relative strength of the gravitational force that the particles experience.

Figure 3. For ![]() $Gn=0$ and
$Gn=0$ and ![]() $Th=1$: (a) instantaneous particle positions and fluid streamlines; (b) fluid streamlines and particle trajectories. (c,d) The same for
$Th=1$: (a) instantaneous particle positions and fluid streamlines; (b) fluid streamlines and particle trajectories. (c,d) The same for ![]() $Gn=0.14$ and
$Gn=0.14$ and ![]() $Th=1$; blue ——, fluid stream lines; blue – – – –, velocity boundary layer edge; – – – –, particle trajectories; Please refer to the particle motion videos corresponding to panel (a) at
$Th=1$; blue ——, fluid stream lines; blue – – – –, velocity boundary layer edge; – – – –, particle trajectories; Please refer to the particle motion videos corresponding to panel (a) at ![]() $Gn=0$ and to panel (c) at
$Gn=0$ and to panel (c) at ![]() $Gn=0.14$, provided in the supplementary movies, to see the evolution of particle positions.
$Gn=0.14$, provided in the supplementary movies, to see the evolution of particle positions.
In figure 3(a,c), we see a dust-free region within the boundary layer, which forms under the influence of thermophoretic force. Both the figures show the final steady-state situation, after the initial transient development of the dust-free region is completed, which takes a dimensionless time of approximately ![]() $t^*\sim 50$. In figure 3(a), for the
$t^*\sim 50$. In figure 3(a), for the ![]() $Gn=0$ case, we see a dust-free region that keeps growing in height as we move along the positive
$Gn=0$ case, we see a dust-free region that keeps growing in height as we move along the positive ![]() $x$ direction; there is no settling of particles on the hot surface. For this case, the height of the dust-free region at any given
$x$ direction; there is no settling of particles on the hot surface. For this case, the height of the dust-free region at any given ![]() $x$ depends on the particular value of
$x$ depends on the particular value of ![]() $Th$. In contrast, the height of the dust-free region in figure 3(c), after an initial increase, keeps decreasing as
$Th$. In contrast, the height of the dust-free region in figure 3(c), after an initial increase, keeps decreasing as ![]() $x$ increases. In the finite
$x$ increases. In the finite ![]() $Gn$ case, the dust-free region ends at a finite distance from the origin of the boundary layer, beyond which the particles start to settle under the influence of gravity; the length of this dust-free region in figure 3(c) is approximately
$Gn$ case, the dust-free region ends at a finite distance from the origin of the boundary layer, beyond which the particles start to settle under the influence of gravity; the length of this dust-free region in figure 3(c) is approximately ![]() $x^* = 45$. We observed that if
$x^* = 45$. We observed that if ![]() $Gn$ is increased, the particles start to settle at a shorter
$Gn$ is increased, the particles start to settle at a shorter ![]() $x^*$ making the dust-free region smaller in size and height; increasing
$x^*$ making the dust-free region smaller in size and height; increasing ![]() $Th$ was seen to have an opposite effect. In both the figures, particles that reach a region close to the leading edge of the boundary layer turn towards the left and exit the domain through the left end of the domain.
$Th$ was seen to have an opposite effect. In both the figures, particles that reach a region close to the leading edge of the boundary layer turn towards the left and exit the domain through the left end of the domain.
Since the particle flow divides into left and right near the leading edge, there exists a saddle point close to the leading edge in the flow field, characterised by zero particle velocity and intersecting particle trajectories.
We obtained such a saddle point in the flow field by solving (2.16) numerically and obtaining the co-ordinates where the particle velocity goes to zero; the intersecting particle trajectories were then obtained by solving (2.16) either backwards in time or forwards in time starting from any point infinitesimally close to the saddle point. Figure 4 shows the obtained particle trajectories close to the saddle point for the case in figure 3(a), where the dashed lines show the trajectories of the particles and the solid lines showing the separatrices that pass through the saddle point. At the saddle point, four particle trajectories, two of which end there starting from the top and the bottom of the domain, and two leaving the saddle point towards the left and right so as to exit the domain can be observed in figure 4; we call these trajectories separatrices. For the ![]() $Gn=0$ case shown in figure 4, the separatrix leaving to the right grows in height and forms the boundary of the dust-free region. For the corresponding
$Gn=0$ case shown in figure 4, the separatrix leaving to the right grows in height and forms the boundary of the dust-free region. For the corresponding ![]() $Gn=0.14$ case, as we show later in figure 5, the height of the separatrix leaving to the right would diminish as
$Gn=0.14$ case, as we show later in figure 5, the height of the separatrix leaving to the right would diminish as ![]() $x$ increases and the separatrix would eventually touch the plate surface at a finite distance from the origin, making the dust-free region finite in size. For such cases, all trajectories of the particles meet the plate at finite distances from the origin.
$x$ increases and the separatrix would eventually touch the plate surface at a finite distance from the origin, making the dust-free region finite in size. For such cases, all trajectories of the particles meet the plate at finite distances from the origin.

Figure 4. Particle trajectories close to the saddle point for the case shown in figure 3(a) with ![]() $Gn=0$ and
$Gn=0$ and ![]() $Th=1$. The solid lines show the four separatrices with arrows showing the direction of the particle motion on the separatrix. The dashed lines show the trajectories of the other particles in the flow field. Particle positions in the domain at a later instant after the dust-free region has formed are shown with black dots.
$Th=1$. The solid lines show the four separatrices with arrows showing the direction of the particle motion on the separatrix. The dashed lines show the trajectories of the other particles in the flow field. Particle positions in the domain at a later instant after the dust-free region has formed are shown with black dots.

Figure 5. Separatrices for varying ratios of ![]() $Gn$ to
$Gn$ to ![]() $Th$ for
$Th$ for ![]() $Th=1$ and
$Th=1$ and ![]() $Th=10$ for
$Th=10$ for ![]() $\widehat {St}=10^{-5}$. The blue set of curves corresponds to
$\widehat {St}=10^{-5}$. The blue set of curves corresponds to ![]() $Th=1$ and the black set of curves corresponds to
$Th=1$ and the black set of curves corresponds to ![]() $Th=10$. ——,
$Th=10$. ——, ![]() $Gn/Th=0$; – – – –,
$Gn/Th=0$; – – – –, ![]() $Gn/Th=0.001$;
$Gn/Th=0.001$; ![]() $\cdots \cdots$,
$\cdots \cdots$, ![]() $Gn/Th=0.1$; and -
$Gn/Th=0.1$; and -![]() $\cdot$-
$\cdot$-![]() $\cdot$-
$\cdot$-![]() $\cdot$-,
$\cdot$-, ![]() $Gn/Th=0.14$. The red dashed lines correspond to
$Gn/Th=0.14$. The red dashed lines correspond to ![]() $y^* \sim {x^*}^{2/5}$, the scaling of natural convection boundary layer thickness.
$y^* \sim {x^*}^{2/5}$, the scaling of natural convection boundary layer thickness.
The separatrices then divide the flow into four sub regions, labelled as I to IV in figure 4, which are characterised by a different behaviour of particles. In region I, the particles coming down from the top take a right turn and move in the positive ![]() $x$ direction. In region II, particles coming down take a left turn and move in the negative
$x$ direction. In region II, particles coming down take a left turn and move in the negative ![]() $x$ direction. In regions III and IV, the particles close to the plate are lifted off due to the upward component of the thermophoretic force and then take a left or a right turn, respectively. As is clear from the trajectories shown in figure 4, all the particles in regions III and IV either exit from the domain through the right/left or settle on the plate; there is no supply of particles to these regions from the regions I and II on the top. The regions III and IV will hence evolve with time into a dust-free region, with the separatrix AOB as its top boundary; the separatrix AOB is hence the steady-state boundary of the dust-free region close to the hot surface.
$x$ direction. In regions III and IV, the particles close to the plate are lifted off due to the upward component of the thermophoretic force and then take a left or a right turn, respectively. As is clear from the trajectories shown in figure 4, all the particles in regions III and IV either exit from the domain through the right/left or settle on the plate; there is no supply of particles to these regions from the regions I and II on the top. The regions III and IV will hence evolve with time into a dust-free region, with the separatrix AOB as its top boundary; the separatrix AOB is hence the steady-state boundary of the dust-free region close to the hot surface.
3.2. Particle velocity for small and finite  $\widehat {St}$
$\widehat {St}$
For finite but small ![]() $\widehat {St}$, for which case the present simulations are conducted, the above flow pattern with saddle point and separatrices can be better understood based on the simplification of (2.16). For
$\widehat {St}$, for which case the present simulations are conducted, the above flow pattern with saddle point and separatrices can be better understood based on the simplification of (2.16). For ![]() $\widehat {St} \ll 1$, but not equal to zero, (2.18) can be modified as
$\widehat {St} \ll 1$, but not equal to zero, (2.18) can be modified as
where ![]() $\boldsymbol{a}^*$ is an inertial correction that needs to be evaluated. Substituting (3.1) in (2.6), neglecting all terms of order higher than
$\boldsymbol{a}^*$ is an inertial correction that needs to be evaluated. Substituting (3.1) in (2.6), neglecting all terms of order higher than ![]() $\widehat {St}$ and then using (2.15), we obtain the inertia corrected particle velocity as
$\widehat {St}$ and then using (2.15), we obtain the inertia corrected particle velocity as
Equation (3.2) is a modification of the slow manifold (Sapsis & Haller Reference Sapsis and Haller2008) of Maxey & Riley (Reference Maxey and Riley1983) equations with thermophoretic effects. When ![]() $\widehat {St} \ll 1$, the last term in (3.2) is negligible while the third term on the right-hand side of (3.2) may still be significant. Hence, for
$\widehat {St} \ll 1$, the last term in (3.2) is negligible while the third term on the right-hand side of (3.2) may still be significant. Hence, for ![]() $\widehat {St} \rightarrow 0$ but finite, (3.2) simplifies to
$\widehat {St} \rightarrow 0$ but finite, (3.2) simplifies to
implying that the particle velocity will be a superposition of the fluid velocity, the thermophoretic velocity (2.17) and the Stokes settling velocity (2.15).
The presence of a saddle point and separatrices in the flow field can now be better understood in terms of (3.3). Since ![]() $\boldsymbol{u}_{t}^*$ acts opposite to the direction of the temperature gradient, the horizontal and the vertical components of
$\boldsymbol{u}_{t}^*$ acts opposite to the direction of the temperature gradient, the horizontal and the vertical components of ![]() $\boldsymbol{u}_{t}^*$, namely
$\boldsymbol{u}_{t}^*$, namely ![]() ${u_{t_x}^*}$ and
${u_{t_x}^*}$ and ![]() $u_{t_y}^*$, expressions for which are derived in Appendix B, will be in the negative
$u_{t_y}^*$, expressions for which are derived in Appendix B, will be in the negative ![]() $x$ direction and in the positive
$x$ direction and in the positive ![]() $y$ direction, respectively. Since the entraining fluid coming down towards the plate takes a turn in the positive
$y$ direction, respectively. Since the entraining fluid coming down towards the plate takes a turn in the positive ![]() $x$ direction inside the boundary layer, the horizontal component
$x$ direction inside the boundary layer, the horizontal component ![]() $u^*_x$ of the fluid velocity in the boundary layer will be in the positive
$u^*_x$ of the fluid velocity in the boundary layer will be in the positive ![]() $x$ direction;
$x$ direction; ![]() $u_y^*$ will be in the negative
$u_y^*$ will be in the negative ![]() $y$ direction. Here,
$y$ direction. Here, ![]() $\boldsymbol{u}_g^*$ has only a vertical component in the negative
$\boldsymbol{u}_g^*$ has only a vertical component in the negative ![]() $y$ direction in the case of a horizontal plate. The saddle point is the point where
$y$ direction in the case of a horizontal plate. The saddle point is the point where ![]() $u_{t_y}^*$ balances
$u_{t_y}^*$ balances ![]() $u_y^*$ and
$u_y^*$ and ![]() $u_g^*$, as well as where
$u_g^*$, as well as where ![]() $u_{t_x}^*$ balances
$u_{t_x}^*$ balances ![]() $u^*_x$. Then, in regions I and IV,
$u^*_x$. Then, in regions I and IV, ![]() $u^*_x$ is more than
$u^*_x$ is more than ![]() $u_{t_x}^*$, and similarly, in regions II and III,
$u_{t_x}^*$, and similarly, in regions II and III, ![]() $u_{t_x}^*$ is more than
$u_{t_x}^*$ is more than ![]() $u^*_x$. The thermophoretic velocity
$u^*_x$. The thermophoretic velocity ![]() $\boldsymbol{u}^*_t$ hence balances the components of
$\boldsymbol{u}^*_t$ hence balances the components of ![]() $\boldsymbol{u}^*$ and
$\boldsymbol{u}^*$ and ![]() $\boldsymbol{u}^*_g$ to create a saddle point, with the four separatrices in the flow field separating regions where the different forces dominate.
$\boldsymbol{u}^*_g$ to create a saddle point, with the four separatrices in the flow field separating regions where the different forces dominate.
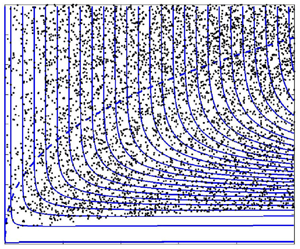
Figure 5 shows the plots of separatrices for four different values of ![]() $Gn/Th$ at
$Gn/Th$ at ![]() $Th=1$ and
$Th=1$ and ![]() $Th=10$. As expected, for both values of
$Th=10$. As expected, for both values of ![]() $Th$, the areas under the separatrix, which are the dust-free regions, reduce as
$Th$, the areas under the separatrix, which are the dust-free regions, reduce as ![]() $Gn$ increases and the gravitational force becomes more dominant. The dust-free region shrinks to a small patch close to the origin of the boundary layer when
$Gn$ increases and the gravitational force becomes more dominant. The dust-free region shrinks to a small patch close to the origin of the boundary layer when ![]() $Gn$ is of the same order as
$Gn$ is of the same order as ![]() $Th$. Figure 5 shows that when
$Th$. Figure 5 shows that when ![]() $Gn=0$ or when
$Gn=0$ or when ![]() $Gn \ll Th$, the height of the dust-free region, at distances away from the saddle point, scales as
$Gn \ll Th$, the height of the dust-free region, at distances away from the saddle point, scales as ![]() $y^* \sim {x^*}^{2/5}$, shown as the red dashed line, in the same manner as the natural convection boundary layer thickness. However, as is obvious from figure 3(a), the thickness of the dust-free region is much lower than the boundary layer thickness. Further, the thickness of the dust-free region is also a function of
$y^* \sim {x^*}^{2/5}$, shown as the red dashed line, in the same manner as the natural convection boundary layer thickness. However, as is obvious from figure 3(a), the thickness of the dust-free region is much lower than the boundary layer thickness. Further, the thickness of the dust-free region is also a function of ![]() $Th$, as shown in figure 5, unlike the boundary layer thickness. As we saw earlier, since the separatrix AOB is the boundary of the dust-free region, an expression obtained for the separatrix would also be an equation for the dust-free region that would expectedly capture the dependence of the dust-free region on the fluid and the flow properties. We now obtain such an expression for the separatrix when the Stokes settling velocity
$Th$, as shown in figure 5, unlike the boundary layer thickness. As we saw earlier, since the separatrix AOB is the boundary of the dust-free region, an expression obtained for the separatrix would also be an equation for the dust-free region that would expectedly capture the dependence of the dust-free region on the fluid and the flow properties. We now obtain such an expression for the separatrix when the Stokes settling velocity ![]() $\boldsymbol{u}^*_g \ll \boldsymbol{u}^*_{t}$, the thermophoretic velocity, which occurs when
$\boldsymbol{u}^*_g \ll \boldsymbol{u}^*_{t}$, the thermophoretic velocity, which occurs when ![]() $Gn \ll Th$.
$Gn \ll Th$.
The present novel method of finding the dust-free region boundary, by finding the equation for the separatrix, was chosen as earlier proposed conditions to obtain dust-free regions failed in the present situation. Stratmann et al. (Reference Stratmann, Fissan, Papperger and Friedlander1988) used the locus of all points having zero vertical velocity as the dust-free region boundary; however, such a curve was observed to be a constant ![]() $\eta$ curve, different from the dust-free region curve, in the present case. The locus of the maximum negative values of the divergence of the particle velocity field (3.3) was also considered as a criterion to identify the boundary of the dust-free region. This criterion was considered since the particles move out of the dust-free region to the boundary of the dust-free region, and due to its similarity with the recently proposed condition by Shevkar et al. (Reference Shevkar, Vishnu, Mohanan, Koothur, Mathur and Puthenveettil2022) to identify plumes, which are converging flow regions. However, the locus of all such points was found to be another constant
$\eta$ curve, different from the dust-free region curve, in the present case. The locus of the maximum negative values of the divergence of the particle velocity field (3.3) was also considered as a criterion to identify the boundary of the dust-free region. This criterion was considered since the particles move out of the dust-free region to the boundary of the dust-free region, and due to its similarity with the recently proposed condition by Shevkar et al. (Reference Shevkar, Vishnu, Mohanan, Koothur, Mathur and Puthenveettil2022) to identify plumes, which are converging flow regions. However, the locus of all such points was found to be another constant ![]() $\eta$ line, which did not coincide with the dust-free region boundary.
$\eta$ line, which did not coincide with the dust-free region boundary.
3.3. Scaling of the dust-free region height when  $Gn \ll Th$ and
$Gn \ll Th$ and  $\widehat {St}\ll 1$
$\widehat {St}\ll 1$
As we saw in § 3.2, when ![]() $Gn \ll Th$, the dust-free region height has a similar dependence on
$Gn \ll Th$, the dust-free region height has a similar dependence on ![]() $x^*$ as the boundary layer thickness, but which, in contrast to the boundary layer thickness, depends on
$x^*$ as the boundary layer thickness, but which, in contrast to the boundary layer thickness, depends on ![]() $Th$. For such cases, an instance of which is shown in figure 4, we now find an expression for the separatrix AOB; this gives the scaling of the dust-free region height for
$Th$. For such cases, an instance of which is shown in figure 4, we now find an expression for the separatrix AOB; this gives the scaling of the dust-free region height for ![]() $Gn \ll Th$. For
$Gn \ll Th$. For ![]() $\widehat {St} \ll 1$, by taking the ratio of
$\widehat {St} \ll 1$, by taking the ratio of ![]() $y$ and
$y$ and ![]() $x$ components of (3.3), we obtain a differential equation for the general trajectory of a particle as
$x$ components of (3.3), we obtain a differential equation for the general trajectory of a particle as
From (2.15) and (2.17), ![]() $u_{g}^*$ in (3.4) can be neglected in comparison to
$u_{g}^*$ in (3.4) can be neglected in comparison to ![]() $u_{t_y}^*$ since
$u_{t_y}^*$ since ![]() $Gn \ll Th$, to obtain
$Gn \ll Th$, to obtain
As we saw in § 3, ![]() $u^*_{t_x}$ is expected to be smaller than
$u^*_{t_x}$ is expected to be smaller than ![]() $u^*_x$ in regions I and IV, and hence, also along the separatrix OB. The expressions for
$u^*_x$ in regions I and IV, and hence, also along the separatrix OB. The expressions for ![]() $u^*_x$ and
$u^*_x$ and ![]() $u^*_{t_x}$ given by (2.26) and (B7) further show that for a given
$u^*_{t_x}$ given by (2.26) and (B7) further show that for a given ![]() $Th$ on constant
$Th$ on constant ![]() $\eta$ line, like the separatrix,
$\eta$ line, like the separatrix, ![]() $u^*_{t_x}/u^*_x \sim {x^*}^{- 6/5}$. Hence, for a given
$u^*_{t_x}/u^*_x \sim {x^*}^{- 6/5}$. Hence, for a given ![]() $Th$ far away from O, since the separatrix follows an
$Th$ far away from O, since the separatrix follows an ![]() $\eta =$ constant variation along OB in figure 4,
$\eta =$ constant variation along OB in figure 4, ![]() $u^*_{t_x}$ can be neglected in comparison to
$u^*_{t_x}$ can be neglected in comparison to ![]() $u^*_x$; (3.5) then reduces to
$u^*_x$; (3.5) then reduces to
Substituting (2.26), (2.27) and (B8) for ![]() $u_x^*$,
$u_x^*$, ![]() $u_y^*$ and
$u_y^*$ and ![]() $u_{t_y}^*$ in (3.6) results in
$u_{t_y}^*$ in (3.6) results in
We now integrate (3.7) with respect to ![]() $x^*$ for
$x^*$ for ![]() $\eta =$ constant, a property of the separatrix for
$\eta =$ constant, a property of the separatrix for ![]() $Gn \ll Th$ (see figure 5), to obtain the equation for the separatrix at any
$Gn \ll Th$ (see figure 5), to obtain the equation for the separatrix at any ![]() $Th$, when
$Th$, when ![]() $Gn \ll Th$ and
$Gn \ll Th$ and ![]() $\widehat {St} \ll 1$, as
$\widehat {St} \ll 1$, as
Substituting ![]() $\eta = {x^*}^{-{2}/{5}} y^* Pr^{-{1}/{5}}$ from (B2), (3.8) simplifies to
$\eta = {x^*}^{-{2}/{5}} y^* Pr^{-{1}/{5}}$ from (B2), (3.8) simplifies to
an equation for the separatrix at any ![]() $Th$ and
$Th$ and ![]() $Pr$ when
$Pr$ when ![]() $Gn \ll Th$ and
$Gn \ll Th$ and ![]() $\widehat {St}\ll 1$. Using the expression for
$\widehat {St}\ll 1$. Using the expression for ![]() $Re$ from (2.12) in (2.11), and defining a modified thermophoretic number,
$Re$ from (2.12) in (2.11), and defining a modified thermophoretic number,
as a ![]() $Pr$ independent dimensionless number for the thermophoretic force, (3.9) can be rewritten as
$Pr$ independent dimensionless number for the thermophoretic force, (3.9) can be rewritten as
Since ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ are functions of
$H'(\eta )$ are functions of ![]() $Pr$, the solution of (3.11) will be a function of
$Pr$, the solution of (3.11) will be a function of ![]() $Th_m$ and
$Th_m$ and ![]() $Pr$. Using the values of
$Pr$. Using the values of ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ obtained from the numerical solution of (2.28a) to (2.28c) for any
$H'(\eta )$ obtained from the numerical solution of (2.28a) to (2.28c) for any ![]() $Pr$, in (3.11), for any given value of
$Pr$, in (3.11), for any given value of ![]() $Th_m$, we get the value of
$Th_m$, we get the value of ![]() $\eta$ which specifies the separatrix OB in figure 4, at distances far from the saddle point O. We will hereinafter refer to these values as
$\eta$ which specifies the separatrix OB in figure 4, at distances far from the saddle point O. We will hereinafter refer to these values as ![]() $\eta _{df}$, the subscript
$\eta _{df}$, the subscript ![]() $df$ indicating that these curves specify the boundary of the dust-free region. Figure 6 shows the separatrices obtained from the numerical solution of (2.16) for
$df$ indicating that these curves specify the boundary of the dust-free region. Figure 6 shows the separatrices obtained from the numerical solution of (2.16) for ![]() $Th=1$,
$Th=1$, ![]() $Th=10$ and
$Th=10$ and ![]() $Th=100$ at
$Th=100$ at ![]() $Pr=0.7$,
$Pr=0.7$, ![]() $Gn=0$ and
$Gn=0$ and ![]() $\widehat {St}=10^{-5}$, along with the
$\widehat {St}=10^{-5}$, along with the ![]() $\eta _{df}$ values predicted by (3.11) for the same values of
$\eta _{df}$ values predicted by (3.11) for the same values of ![]() $Th$ and
$Th$ and ![]() $Pr$. It is clear from the figure that the curves of separatrices predicted by (3.11) approximate the dust-free region fairly well in the entire domain, and more accurately, in the regions away from the saddle point. The plots of
$Pr$. It is clear from the figure that the curves of separatrices predicted by (3.11) approximate the dust-free region fairly well in the entire domain, and more accurately, in the regions away from the saddle point. The plots of ![]() $\eta _{df}$ versus
$\eta _{df}$ versus ![]() $Th_m$ for
$Th_m$ for ![]() $Pr$ ranging from
$Pr$ ranging from ![]() $0.1$ to
$0.1$ to ![]() $10$, obtained from the solution of (3.11), are shown in figure 7. It is observed from the figure that for smaller
$10$, obtained from the solution of (3.11), are shown in figure 7. It is observed from the figure that for smaller ![]() $\eta _{df}$ (
$\eta _{df}$ (![]() $\eta _{df}\le 1$),
$\eta _{df}\le 1$), ![]() $\eta _{df}$ increases as
$\eta _{df}$ increases as ![]() $Pr$ increases while for larger
$Pr$ increases while for larger ![]() $\eta _{df}$ (
$\eta _{df}$ (![]() $\eta _{df}\ge 3$),
$\eta _{df}\ge 3$), ![]() $\eta _{df}$ decreases as
$\eta _{df}$ decreases as ![]() $Pr$ increases. Figure 7 also shows that
$Pr$ increases. Figure 7 also shows that ![]() $\eta _{df}$ has different scaling dependences on
$\eta _{df}$ has different scaling dependences on ![]() $Th_m$ for small and large
$Th_m$ for small and large ![]() $\eta _{df}$; we now obtain these scaling laws for small and large
$\eta _{df}$; we now obtain these scaling laws for small and large ![]() $\eta$, with the
$\eta$, with the ![]() $Pr$ dependence obtained later in § 4.
$Pr$ dependence obtained later in § 4.

Figure 6. Comparison of separatrices from the numerical solution of (2.16) with the solution of (3.11) for ![]() $\widehat {St} = 10^{-5}$,
$\widehat {St} = 10^{-5}$, ![]() $Gn=0$ and
$Gn=0$ and ![]() $Pr=0.7$, for different
$Pr=0.7$, for different ![]() $Th$. Numerical solution of (2.16) for: -
$Th$. Numerical solution of (2.16) for: -![]() $\cdot$-
$\cdot$-![]() $\cdot$-,
$\cdot$-, ![]() $Th=1$; ——,
$Th=1$; ——, ![]() $Th=10$; – – –,
$Th=10$; – – –, ![]() $Th=100$. The solution of (3.11) for: –
$Th=100$. The solution of (3.11) for: –![]() $\diamond$–,
$\diamond$–, ![]() $Th=1$; –
$Th=1$; –![]() $\triangle$–,
$\triangle$–, ![]() $Th=10$; –
$Th=10$; –![]() $\square$–,
$\square$–, ![]() $Th=100$.
$Th=100$.

Figure 7. Variation of dimensionless dust-free region height ![]() $\eta _{df}$ with the modified thermophoretic number (3.10) for various
$\eta _{df}$ with the modified thermophoretic number (3.10) for various ![]() $Pr$: red
$Pr$: red ![]() $\circ$,
$\circ$, ![]() $Pr=0.1$;
$Pr=0.1$; ![]() $*$,
$*$, ![]() $Pr=0.3$; orange
$Pr=0.3$; orange ![]() $\diamond$,
$\diamond$, ![]() $Pr=0.5$;
$Pr=0.5$; ![]() $\triangledown$,
$\triangledown$, ![]() $Pr=0.7$; green star,
$Pr=0.7$; green star, ![]() $Pr=1$; red
$Pr=1$; red ![]() $\triangleright$,
$\triangleright$, ![]() $Pr=3$;
$Pr=3$; ![]() $+$,
$+$, ![]() $Pr=7$; blue
$Pr=7$; blue ![]() $\square$,
$\square$, ![]() $Pr=5$; brown
$Pr=5$; brown ![]() $\triangle$,
$\triangle$, ![]() $Pr=10$; – – –,
$Pr=10$; – – –, ![]() $\eta _{df} \sim \sqrt {Th_m}$;
$\eta _{df} \sim \sqrt {Th_m}$; ![]() $\cdots \cdots \cdots$,
$\cdots \cdots \cdots$, ![]() $\eta _{df} \sim \ln (Th_m)$. The inset shows the curves
$\eta _{df} \sim \ln (Th_m)$. The inset shows the curves ![]() $-(5/3)Th_mH'(\eta )$ for; blue – – –,
$-(5/3)Th_mH'(\eta )$ for; blue – – –, ![]() $Th_m =1$; green – – –,
$Th_m =1$; green – – –, ![]() $Th_m = 5$; magenta – – –,
$Th_m = 5$; magenta – – –, ![]() $Th_m = 10$; and ——,
$Th_m = 10$; and ——, ![]() $F(\eta )$ for
$F(\eta )$ for ![]() $Pr=0.7$.
$Pr=0.7$.
3.3.1. Limit  $\eta \rightarrow 0$
$\eta \rightarrow 0$
We obtain the dependence of ![]() $\eta _{df}$ on
$\eta _{df}$ on ![]() $Th_m$ for smaller values of
$Th_m$ for smaller values of ![]() $\eta _{df}$ by using approximations of
$\eta _{df}$ by using approximations of ![]() $F'(\eta )$ and
$F'(\eta )$ and ![]() $H(\eta )$ in (3.11) as
$H(\eta )$ in (3.11) as ![]() $\eta \rightarrow 0$. A local analysis of (2.28a) to (2.28c) around
$\eta \rightarrow 0$. A local analysis of (2.28a) to (2.28c) around ![]() $\eta =0$, given in Appendix C.1, shows that
$\eta =0$, given in Appendix C.1, shows that ![]() $F'(\eta )$ and
$F'(\eta )$ and ![]() $H(\eta )$ are linear functions in
$H(\eta )$ are linear functions in ![]() $\eta$ as
$\eta$ as ![]() $\eta \rightarrow 0$ (see (C8)). We hence approximate
$\eta \rightarrow 0$ (see (C8)). We hence approximate ![]() $F'(\eta ) = 2 M(Pr) \eta$ and
$F'(\eta ) = 2 M(Pr) \eta$ and ![]() $H(\eta ) = 1- N(Pr) \eta$ so that
$H(\eta ) = 1- N(Pr) \eta$ so that
and
where ![]() $M(Pr)$ and
$M(Pr)$ and ![]() $N(Pr)$ are some positive real functions of
$N(Pr)$ are some positive real functions of ![]() $Pr$. Using (3.12a) and (3.12b) in (3.11), we obtain
$Pr$. Using (3.12a) and (3.12b) in (3.11), we obtain
where using (2.22), (2.23) and (2.3) in (2.21),
is the dimensionless dust-free region height, with ![]() $y_{df}$ being the dimensional dust-free region height and
$y_{df}$ being the dimensional dust-free region height and
 \begin{equation} Q(Pr) = \sqrt{\frac{5}{3} \frac{N(Pr)}{M(Pr)}} \end{equation}
\begin{equation} Q(Pr) = \sqrt{\frac{5}{3} \frac{N(Pr)}{M(Pr)}} \end{equation}
is a function of ![]() $N(Pr)$ and
$N(Pr)$ and ![]() $M(Pr)$. Since both
$M(Pr)$. Since both ![]() $N(Pr)$ and
$N(Pr)$ and ![]() $M(Pr)$ are positive real functions,
$M(Pr)$ are positive real functions, ![]() $Q(Pr)$ is always positive for a hot plate, because of which, for all
$Q(Pr)$ is always positive for a hot plate, because of which, for all ![]() $Pr$,
$Pr$, ![]() $\eta _{df}$ always increase as
$\eta _{df}$ always increase as ![]() $\sqrt {Th_m}$ for small
$\sqrt {Th_m}$ for small ![]() $\eta$, as shown in figure 7.
$\eta$, as shown in figure 7.
3.3.2. Limit  $\eta \rightarrow \infty$
$\eta \rightarrow \infty$
The dependence of ![]() $\eta _{df}$ on
$\eta _{df}$ on ![]() $Th_m$ for large values of
$Th_m$ for large values of ![]() $\eta _{df}$ is obtained as follows. As
$\eta _{df}$ is obtained as follows. As ![]() $\eta \rightarrow \infty$, the boundary condition (2.30b) implies that
$\eta \rightarrow \infty$, the boundary condition (2.30b) implies that
where ![]() $C_1(Pr)$ is a positive real function of
$C_1(Pr)$ is a positive real function of ![]() $Pr$. Using (3.16a) in (2.28c) and then integrating gives
$Pr$. Using (3.16a) in (2.28c) and then integrating gives
where ![]() $C_2(Pr)$ is a negative real function of
$C_2(Pr)$ is a negative real function of ![]() $Pr$. Using (3.16a) and (3.16b) in (3.11) gives
$Pr$. Using (3.16a) and (3.16b) in (3.11) gives
where
are functions of ![]() $Pr$. Since
$Pr$. Since ![]() $S(Pr)$ is a positive real function in
$S(Pr)$ is a positive real function in ![]() $Pr$, we see that
$Pr$, we see that ![]() $\eta _{df}$ increases as
$\eta _{df}$ increases as ![]() $\ln (Th_m)$ for all
$\ln (Th_m)$ for all ![]() $Pr$ at large
$Pr$ at large ![]() $\eta$ as shown in figure 7; a higher
$\eta$ as shown in figure 7; a higher ![]() $\eta _{df}$ always corresponds to a higher value of
$\eta _{df}$ always corresponds to a higher value of ![]() $Th_m$ for a given
$Th_m$ for a given ![]() $Pr$. This dependence could also be explained from a graphical depiction of (3.11). The inset of figure 7 shows a plot of
$Pr$. This dependence could also be explained from a graphical depiction of (3.11). The inset of figure 7 shows a plot of ![]() $-(5/3)Th_mH'(\eta )$, the right-hand side of (3.11), versus
$-(5/3)Th_mH'(\eta )$, the right-hand side of (3.11), versus ![]() $\eta$ for
$\eta$ for ![]() $Pr=0.7$ along with the plot for
$Pr=0.7$ along with the plot for ![]() $F(\eta )$, the left-hand side of (3.11), versus
$F(\eta )$, the left-hand side of (3.11), versus ![]() $\eta$ for the same
$\eta$ for the same ![]() $Pr$. The curves for
$Pr$. The curves for ![]() $-(5/3)Th_mH'(\eta )$ corresponding to
$-(5/3)Th_mH'(\eta )$ corresponding to ![]() $Th_m=1$,
$Th_m=1$, ![]() $Th_m=5$ and
$Th_m=5$ and ![]() $Th_m=10$ cross the curve for
$Th_m=10$ cross the curve for ![]() $F(\eta )$ at higher values of
$F(\eta )$ at higher values of ![]() $\eta$, as
$\eta$, as ![]() $Th_m$ is increased from 1 to 10. In (3.13) and (3.17), the
$Th_m$ is increased from 1 to 10. In (3.13) and (3.17), the ![]() $Pr$ dependence of
$Pr$ dependence of ![]() $\eta _{df}$ is not fully specified; we now obtain the complete expressions for
$\eta _{df}$ is not fully specified; we now obtain the complete expressions for ![]() $\eta _{df}$ in terms of
$\eta _{df}$ in terms of ![]() $Pr$ and
$Pr$ and ![]() $Th_m$ for different ranges of
$Th_m$ for different ranges of ![]() $Pr$.
$Pr$.
4. Scaling laws for dust-free region heights
The velocity function ![]() $F'$ and the temperature function
$F'$ and the temperature function ![]() $H$ in the boundary layer equations (2.28) uncouple for
$H$ in the boundary layer equations (2.28) uncouple for ![]() $Pr \rightarrow 0$ and
$Pr \rightarrow 0$ and ![]() $Pr \rightarrow \infty$. This, however, occurs since (2.28a–c) are not valid for these asymptotic
$Pr \rightarrow \infty$. This, however, occurs since (2.28a–c) are not valid for these asymptotic ![]() $Pr$ cases. When
$Pr$ cases. When ![]() $Pr \gg 1$, the thermal boundary layer thickness is much smaller than the velocity boundary layer thickness; the opposite occurs when
$Pr \gg 1$, the thermal boundary layer thickness is much smaller than the velocity boundary layer thickness; the opposite occurs when ![]() $Pr \ll 1$. Rotem & Claassen (Reference Rotem and Claassen1969) treats these two asymptotic
$Pr \ll 1$. Rotem & Claassen (Reference Rotem and Claassen1969) treats these two asymptotic ![]() $Pr$ cases as two separate boundary layer problems. They solve a set of inner boundary layer equations for the thin boundary layer inside, and a set of outer boundary layer equations for the larger boundary layer region outside; we follow the same approach to obtain scaling laws for
$Pr$ cases as two separate boundary layer problems. They solve a set of inner boundary layer equations for the thin boundary layer inside, and a set of outer boundary layer equations for the larger boundary layer region outside; we follow the same approach to obtain scaling laws for ![]() $\eta _{df}$ for
$\eta _{df}$ for ![]() $Pr \ll 1$ and
$Pr \ll 1$ and ![]() $Pr \gg 1$. Hereinafter, we use the subscripts
$Pr \gg 1$. Hereinafter, we use the subscripts ![]() $1$ and
$1$ and ![]() $2$ to denote the inner and outer boundary layer parameters, and a single tilde and a double tilde to denote the
$2$ to denote the inner and outer boundary layer parameters, and a single tilde and a double tilde to denote the ![]() $Pr\gg 1$ and the
$Pr\gg 1$ and the ![]() $Pr\ll 1$ cases, respectively. A brief explanation of the stretched variables used by Rotem & Claassen (Reference Rotem and Claassen1969) in these regimes of
$Pr\ll 1$ cases, respectively. A brief explanation of the stretched variables used by Rotem & Claassen (Reference Rotem and Claassen1969) in these regimes of ![]() $Pr$ is provided in Appendix D.
$Pr$ is provided in Appendix D.
4.1. Scaling for  $Pr\gg 1$
$Pr\gg 1$
4.1.1. Scaling for small  $\eta$
$\eta$
Rotem & Claassen (Reference Rotem and Claassen1969) provides the inner boundary layer equations for the case of ![]() $Pr \gg 1$ as follows:
$Pr \gg 1$ as follows:
where ![]() ${\tilde {F}}_{1}$,
${\tilde {F}}_{1}$, ![]() ${\tilde {G}}_{1}$ and
${\tilde {G}}_{1}$ and ![]() ${\tilde {H}}_{1}$ are the dimensionless velocity, pressure and temperature functions obtained by using appropriate transformations as in (D6) and (D7). The boundary conditions include
${\tilde {H}}_{1}$ are the dimensionless velocity, pressure and temperature functions obtained by using appropriate transformations as in (D6) and (D7). The boundary conditions include
and
where ![]() $\tilde {\eta }_{1}$ is the similarity variable defined for
$\tilde {\eta }_{1}$ is the similarity variable defined for ![]() $Pr\gg 1$ in (D6). Since there is no
$Pr\gg 1$ in (D6). Since there is no ![]() $Pr$ appearing in (4.1),
$Pr$ appearing in (4.1), ![]() $\tilde {F}_{1}$ and
$\tilde {F}_{1}$ and ![]() $\tilde {H}_{1}$ will only depend of
$\tilde {H}_{1}$ will only depend of ![]() $\tilde {\eta }_{1}$ and not on
$\tilde {\eta }_{1}$ and not on ![]() $Pr$.
$Pr$.
A local analysis of (4.1) near ![]() $\tilde {\eta }_{1}=0$, given in Appendix C.2, shows that
$\tilde {\eta }_{1}=0$, given in Appendix C.2, shows that ![]() $\tilde {F}'_{1}(\tilde {\eta }_{1})$ and
$\tilde {F}'_{1}(\tilde {\eta }_{1})$ and ![]() $\tilde {H}_{1}(\tilde {\eta }_{1})$ are linear when
$\tilde {H}_{1}(\tilde {\eta }_{1})$ are linear when ![]() $\tilde {\eta }_{1} \rightarrow 0$. In such a case,
$\tilde {\eta }_{1} \rightarrow 0$. In such a case,
where ![]() $C_1=\tilde {F}''_{1}(\tilde {\eta }_{1}=0)/2=0.49$ and
$C_1=\tilde {F}''_{1}(\tilde {\eta }_{1}=0)/2=0.49$ and
where ![]() $C_2=\tilde {H}'_{1}(0)=-0.46$. It is to be noted that
$C_2=\tilde {H}'_{1}(0)=-0.46$. It is to be noted that ![]() $\tilde {F}_{1}(\tilde {\eta }_{1})$ in (4.3) and
$\tilde {F}_{1}(\tilde {\eta }_{1})$ in (4.3) and ![]() $\tilde {H}'_{1}(\tilde {\eta }_{1})$ in (4.4) are independent of
$\tilde {H}'_{1}(\tilde {\eta }_{1})$ in (4.4) are independent of ![]() $Pr$, since the boundary layer equations (4.1) do not have
$Pr$, since the boundary layer equations (4.1) do not have ![]() $Pr$ in them. So
$Pr$ in them. So ![]() $C_1$ and
$C_1$ and ![]() $C_2$ are just constants and not functions of
$C_2$ are just constants and not functions of ![]() $Pr$. From (D9), (D3) and (D5b), we have
$Pr$. From (D9), (D3) and (D5b), we have
Similarly, from (D5a), (D6c) and (2.21), we obtain
Using (4.6) in (4.5) and integrating, since ![]() $\tilde {F}_{1}(0)$ and
$\tilde {F}_{1}(0)$ and ![]() $F(0)$ are equal to zero gives us
$F(0)$ are equal to zero gives us
Differentiating (D6b) and using (4.6), we get
Even though both ![]() $\tilde {F}_{1}(\tilde {\eta }_{1})$ and
$\tilde {F}_{1}(\tilde {\eta }_{1})$ and ![]() $\tilde {H}'_1(\tilde {\eta }_{1})$ are independent of
$\tilde {H}'_1(\tilde {\eta }_{1})$ are independent of ![]() $Pr$ (see (4.3) and (4.4)), the transformation back to the original boundary layer variables
$Pr$ (see (4.3) and (4.4)), the transformation back to the original boundary layer variables ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ in (4.7) and (4.8) brings back the
$H'(\eta )$ in (4.7) and (4.8) brings back the ![]() $Pr$ dependence of the velocity and the temperature fields. Using (4.7) and (4.8) to convert (4.3) and (4.4) to be in terms of
$Pr$ dependence of the velocity and the temperature fields. Using (4.7) and (4.8) to convert (4.3) and (4.4) to be in terms of ![]() $\eta$, then substituting in (3.11) and using (4.6), we get
$\eta$, then substituting in (3.11) and using (4.6), we get
Equation (4.9) is the theoretically obtained scaling relation for ![]() $\eta _{df}$ in the small
$\eta _{df}$ in the small ![]() $\eta$ limit for
$\eta$ limit for ![]() $Pr \gg 1$. A comparison of (3.13) with (4.9) makes it clear that the unspecified
$Pr \gg 1$. A comparison of (3.13) with (4.9) makes it clear that the unspecified ![]() $Pr$ dependence in (3.13) is
$Pr$ dependence in (3.13) is
In figure 8, we compare (4.9) with ![]() $\eta _{df}$ obtained from (3.11) for
$\eta _{df}$ obtained from (3.11) for ![]() $Pr=2$,
$Pr=2$, ![]() $Pr=10$ and
$Pr=10$ and ![]() $Pr=100$; where
$Pr=100$; where ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ corresponding to
$H'(\eta )$ corresponding to ![]() $Pr=2$,
$Pr=2$, ![]() $Pr=10$ and
$Pr=10$ and ![]() $Pr=100$ used in (3.11) were obtained by numerically solving (2.28). Since (4.9) is valid for
$Pr=100$ used in (3.11) were obtained by numerically solving (2.28). Since (4.9) is valid for ![]() $Pr \gg 1$, we expect the data of
$Pr \gg 1$, we expect the data of ![]() $Pr=100$ to approach (4.9) in the small
$Pr=100$ to approach (4.9) in the small ![]() $\eta$ limit, while the data of
$\eta$ limit, while the data of ![]() $Pr=2$ to deviate. Surprisingly, in figure 8, the data of
$Pr=2$ to deviate. Surprisingly, in figure 8, the data of ![]() $Pr=2$,
$Pr=2$, ![]() $Pr=10$ and
$Pr=10$ and ![]() $Pr=100$ collapse onto (4.9) suggesting that (4.9) is valid not just for
$Pr=100$ collapse onto (4.9) suggesting that (4.9) is valid not just for ![]() $Pr \gg 1$, but also for any
$Pr \gg 1$, but also for any ![]() $Pr>1$, for small
$Pr>1$, for small ![]() $\eta$.
$\eta$.

Figure 8. Scaling of the dimensionless dust-free region height ![]() $\eta _{df}$ for
$\eta _{df}$ for ![]() $Pr \gg 1$ in the small and the large
$Pr \gg 1$ in the small and the large ![]() $\eta$ limits. Numerical solution of (3.11) for: blue
$\eta$ limits. Numerical solution of (3.11) for: blue ![]() $\circ$,
$\circ$, ![]() $Pr=2$; green
$Pr=2$; green ![]() $\triangle$,
$\triangle$, ![]() $Pr=10$; and red
$Pr=10$; and red ![]() $\diamond$,
$\diamond$, ![]() $Pr=100$. The scaling relations ———, (4.9) and – – – –, (4.13). The inset shows the variation of
$Pr=100$. The scaling relations ———, (4.9) and – – – –, (4.13). The inset shows the variation of ![]() $\tilde {F}(\tilde {\eta })$ with
$\tilde {F}(\tilde {\eta })$ with ![]() $\tilde {\eta }$ for
$\tilde {\eta }$ for ![]() $Pr \gg 1$, obtained by solving the inner boundary layer equations (4.1).
$Pr \gg 1$, obtained by solving the inner boundary layer equations (4.1).
4.1.2. Scaling for large  $\eta$
$\eta$
For ![]() $Pr \gg 1$, the thermal boundary layer is an inner boundary layer; there is negligible temperature gradient above it in the velocity boundary layer. Since thermophoresis and the resultant formation of the dust-free region are due to temperature gradients, for
$Pr \gg 1$, the thermal boundary layer is an inner boundary layer; there is negligible temperature gradient above it in the velocity boundary layer. Since thermophoresis and the resultant formation of the dust-free region are due to temperature gradients, for ![]() $Pr \gg 1$, we expect the dust-free region to not extend beyond this inner thermal boundary layer. The scaling of the dust-free region height in the large
$Pr \gg 1$, we expect the dust-free region to not extend beyond this inner thermal boundary layer. The scaling of the dust-free region height in the large ![]() $\eta$ limit has to be then obtained from an analysis of the top regions of the inner thermal boundary layer. We hence look at the inner boundary layer equations (4.1), with the corresponding boundary conditions (4.2) to find the large
$\eta$ limit has to be then obtained from an analysis of the top regions of the inner thermal boundary layer. We hence look at the inner boundary layer equations (4.1), with the corresponding boundary conditions (4.2) to find the large ![]() $\eta$ scaling of
$\eta$ scaling of ![]() $\eta _{df}$ at
$\eta _{df}$ at ![]() $Pr\gg 1$.
$Pr\gg 1$.
From the ![]() $\tilde {\eta }_{1} \rightarrow \infty$ boundary condition for the velocity function in (4.2b), we find that
$\tilde {\eta }_{1} \rightarrow \infty$ boundary condition for the velocity function in (4.2b), we find that ![]() $\tilde {F}_{1}$ should be varying linearly as
$\tilde {F}_{1}$ should be varying linearly as
This linear variation of ![]() $\tilde {F}_{1}$ for large
$\tilde {F}_{1}$ for large ![]() $\tilde {\eta }_{1}$ can be seen in the inset of figure 8, which shows the variation of
$\tilde {\eta }_{1}$ can be seen in the inset of figure 8, which shows the variation of ![]() $\tilde {F}_{1}(\tilde {\eta }_{1})$ with
$\tilde {F}_{1}(\tilde {\eta }_{1})$ with ![]() $\tilde {\eta }_{1}$ obtained by solving (4.1). We obtain
$\tilde {\eta }_{1}$ obtained by solving (4.1). We obtain ![]() $C_3=1.04$ and
$C_3=1.04$ and ![]() $C_4=1.15$ from the
$C_4=1.15$ from the ![]() $y$ intercept and the slope of the linear portion of this plot. Substituting (4.11) in (4.1c) and integrating gives us
$y$ intercept and the slope of the linear portion of this plot. Substituting (4.11) in (4.1c) and integrating gives us ![]() $\tilde {H}'_{1}(\tilde {\eta }_{1})$ at large
$\tilde {H}'_{1}(\tilde {\eta }_{1})$ at large ![]() $\tilde {\eta }_{1}$ as
$\tilde {\eta }_{1}$ as
A curve fit to the values of ![]() $\tilde {H}'(\tilde {\eta })$ for
$\tilde {H}'(\tilde {\eta })$ for ![]() $Pr \gg 1$, obtained by numerically solving (4.1), showed that
$Pr \gg 1$, obtained by numerically solving (4.1), showed that ![]() $C_5=-0.38$. Converting (4.11) and (4.12) into
$C_5=-0.38$. Converting (4.11) and (4.12) into ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ by using (4.7) and (4.8), and then substituting in (3.11) and using (4.6), we get
$H'(\eta )$ by using (4.7) and (4.8), and then substituting in (3.11) and using (4.6), we get
where ![]() $W_0(x)$ is the Lambert W function, which is the inverse function of
$W_0(x)$ is the Lambert W function, which is the inverse function of ![]() $y\,{\rm e}^y=x$.
$y\,{\rm e}^y=x$.
Equation (4.13) is the expression for ![]() $\eta _{df}$ in the large
$\eta _{df}$ in the large ![]() $\eta$ limit for
$\eta$ limit for ![]() $Pr \gg 1$. In figure 8, we compare (4.13) with
$Pr \gg 1$. In figure 8, we compare (4.13) with ![]() $\eta _{df}$ obtained from (3.11) for
$\eta _{df}$ obtained from (3.11) for ![]() $Pr=2$,
$Pr=2$, ![]() $Pr=10$ and
$Pr=10$ and ![]() $Pr=100$, where the
$Pr=100$, where the ![]() $F(\eta )$ and the
$F(\eta )$ and the ![]() $H'(\eta )$ corresponding to
$H'(\eta )$ corresponding to ![]() $Pr=2$,
$Pr=2$, ![]() $Pr=10$ and
$Pr=10$ and ![]() $Pr=100$ used in (3.11) were obtained by numerically solving (2.28). As expected, the data of
$Pr=100$ used in (3.11) were obtained by numerically solving (2.28). As expected, the data of ![]() $Pr=100$ are very close to the curve of (4.13), which was derived for the limit of
$Pr=100$ are very close to the curve of (4.13), which was derived for the limit of ![]() $Pr \gg 1$, while the data of
$Pr \gg 1$, while the data of ![]() $Pr=2$ deviate from (4.13). Thus, (4.13) seems to hold as an analytic expression for the dust-free region height for
$Pr=2$ deviate from (4.13). Thus, (4.13) seems to hold as an analytic expression for the dust-free region height for ![]() $Pr \ge 100$ for large
$Pr \ge 100$ for large ![]() $\eta _{df}$ values corresponding to higher
$\eta _{df}$ values corresponding to higher ![]() $Th_m$. We now examine the scaling laws for the large and the small
$Th_m$. We now examine the scaling laws for the large and the small ![]() $\eta$ cases for low
$\eta$ cases for low ![]() $Pr$.
$Pr$.
4.2. Scaling for  $Pr\ll 1$
$Pr\ll 1$
4.2.1. Scaling for large  $\eta$
$\eta$
For ![]() $Pr \ll 1$, Rotem & Claassen (Reference Rotem and Claassen1969) gives the outer boundary layer equations in terms of the dimensionless velocity
$Pr \ll 1$, Rotem & Claassen (Reference Rotem and Claassen1969) gives the outer boundary layer equations in terms of the dimensionless velocity ![]() $\tilde {\tilde {F_2}}$ in (D12a), pressure
$\tilde {\tilde {F_2}}$ in (D12a), pressure ![]() $\tilde {\tilde {G_2}}$ in (D13) and temperature function
$\tilde {\tilde {G_2}}$ in (D13) and temperature function ![]() $\tilde {\tilde {H_2}}$ in (D12b) in the outer boundary layer as
$\tilde {\tilde {H_2}}$ in (D12b) in the outer boundary layer as
with the boundary conditions
and
The subscript 2 in the above equations is for the outer boundary layer.
From (D15), (D3) and (D11c), we have
Similarly, we have
from (D11a), (D11b), (D12c) and (2.21). Using (4.17) in (4.16), integrating, and since ![]() $F(0)$ and
$F(0)$ and ![]() $\tilde {\tilde {F_2}}(0)$ are equal to zero from (2.30a) and (4.15a), respectively, we get
$\tilde {\tilde {F_2}}(0)$ are equal to zero from (2.30a) and (4.15a), respectively, we get
Differentiating (D12b) and using (2.20) and (4.17), we get
From the boundary condition for velocity function in (4.15b) for ![]() $\tilde {\tilde {\eta _2}} \rightarrow \infty$, we see that
$\tilde {\tilde {\eta _2}} \rightarrow \infty$, we see that
where the constant ![]() $C_6=1.78$ is obtained from the numerical solution of (4.14). Using (4.20) in (4.1c) and integrating gives
$C_6=1.78$ is obtained from the numerical solution of (4.14). Using (4.20) in (4.1c) and integrating gives
where ![]() $C_7=-1.97$, as obtained by numerically solving (4.14) for
$C_7=-1.97$, as obtained by numerically solving (4.14) for ![]() $\tilde {\tilde {H'_2}}(\tilde {\tilde {\eta _2}})$.
$\tilde {\tilde {H'_2}}(\tilde {\tilde {\eta _2}})$.
Using (4.17), (4.18) and (4.19) to convert (4.20) and (4.21) to be in terms of ![]() $\eta$, and then substituting in (3.11), we get the dimensionless dust-free region height at large
$\eta$, and then substituting in (3.11), we get the dimensionless dust-free region height at large ![]() $\eta$ for
$\eta$ for ![]() $Pr \ll 1$ as
$Pr \ll 1$ as
In figure 9(a), we compare (4.22) with the ![]() $\eta _{df}$ obtained from (3.11) by using the numerical solution of (2.28) for
$\eta _{df}$ obtained from (3.11) by using the numerical solution of (2.28) for ![]() $Pr=0.1$ and
$Pr=0.1$ and ![]() $Pr=1$. Since (4.22) was obtained from the equations of Rotem & Claassen (Reference Rotem and Claassen1969) for
$Pr=1$. Since (4.22) was obtained from the equations of Rotem & Claassen (Reference Rotem and Claassen1969) for ![]() $Pr \ll 1$ in (4.14), as expected, the numerical solution for
$Pr \ll 1$ in (4.14), as expected, the numerical solution for ![]() $Pr=0.1$ agrees with the scaling relation (4.22) for large
$Pr=0.1$ agrees with the scaling relation (4.22) for large ![]() $\eta$. However, surprisingly, the data for
$\eta$. However, surprisingly, the data for ![]() $Pr=1$ also match with (4.22) for large
$Pr=1$ also match with (4.22) for large ![]() $\eta$, suggesting that (4.22) is valid even for
$\eta$, suggesting that (4.22) is valid even for ![]() $Pr\le 1$. Thus, (4.22) gives the scaling for the dimensionless dust-free region height for
$Pr\le 1$. Thus, (4.22) gives the scaling for the dimensionless dust-free region height for ![]() $Pr\le 1$ for large
$Pr\le 1$ for large ![]() $Th_m$.
$Th_m$.

Figure 9. Scaling of the dimensionless dust-free region height (![]() $\eta _{df}$) at
$\eta _{df}$) at ![]() $Pr \ll 1$ in the large and the small
$Pr \ll 1$ in the large and the small ![]() $\eta$ limits. (a) Large
$\eta$ limits. (a) Large ![]() $\eta$ limit. Numerical solutions at green
$\eta$ limit. Numerical solutions at green ![]() $\circ$,
$\circ$, ![]() $Pr=0.1$ and magenta
$Pr=0.1$ and magenta ![]() $\diamond$,
$\diamond$, ![]() $Pr=1$; – – – –, scaling relation (4.22). (b) Small
$Pr=1$; – – – –, scaling relation (4.22). (b) Small ![]() $\eta$ limit. Numerical solutions at green
$\eta$ limit. Numerical solutions at green ![]() $\circ$,
$\circ$, ![]() $Pr=0.1$ and magenta
$Pr=0.1$ and magenta ![]() $\diamond$,
$\diamond$, ![]() $Pr=1$; – – – –, scaling relation (4.29). The inset shows the variation of
$Pr=1$; – – – –, scaling relation (4.29). The inset shows the variation of ![]() $\tilde {\tilde{H}}^{\prime}_2(\tilde {\tilde {\eta }})$ with
$\tilde {\tilde{H}}^{\prime}_2(\tilde {\tilde {\eta }})$ with ![]() $\tilde {\tilde {\eta }}$ for
$\tilde {\tilde {\eta }}$ for ![]() $Pr\ll 1$, obtained by solving the outer boundary layer equations (4.14).
$Pr\ll 1$, obtained by solving the outer boundary layer equations (4.14).
4.2.2. Scaling for small  $\eta$
$\eta$
Rotem & Claassen (Reference Rotem and Claassen1969) provides the inner boundary layer equations for ![]() $Pr \ll 1$ as
$Pr \ll 1$ as
subjected to the boundary conditions,
and
Subscript ![]() $1$ in the above equations denote the inner boundary layer. However, (4.23b) implies that the temperature gradient inside the inner velocity boundary layer,
$1$ in the above equations denote the inner boundary layer. However, (4.23b) implies that the temperature gradient inside the inner velocity boundary layer, ![]() $\tilde {\tilde {H'_1}}=0$; this is quite unphysical since the maximum temperature gradient in natural convection flows occurs at the wall. So we assume that the temperature gradient in the outer velocity boundary layer, which approaches a constant value at lower
$\tilde {\tilde {H'_1}}=0$; this is quite unphysical since the maximum temperature gradient in natural convection flows occurs at the wall. So we assume that the temperature gradient in the outer velocity boundary layer, which approaches a constant value at lower ![]() $\tilde {\tilde {\eta _2}}$, is maintained throughout the inner thermal boundary layer. By numerically solving the set of outer boundary layer equations (4.14), we get
$\tilde {\tilde {\eta _2}}$, is maintained throughout the inner thermal boundary layer. By numerically solving the set of outer boundary layer equations (4.14), we get
as ![]() $\tilde {\tilde {\eta _2}} \rightarrow 0$, shown as the
$\tilde {\tilde {\eta _2}} \rightarrow 0$, shown as the ![]() $y$-intercept in the inset of figure 9(b). From a local analysis of (4.23) near
$y$-intercept in the inset of figure 9(b). From a local analysis of (4.23) near ![]() $\tilde {\tilde {\eta _1}}=0$, given in Appendix C.3, we see that
$\tilde {\tilde {\eta _1}}=0$, given in Appendix C.3, we see that ![]() $\tilde {\tilde {F_1}}$ can be approximated to
$\tilde {\tilde {F_1}}$ can be approximated to
As given by Rotem & Claassen (Reference Rotem and Claassen1969),
and
 \begin{equation} \eta=\frac{Pr^{1/10}\tilde{\tilde{\eta_1}}}{\sqrt{\tilde{\tilde{F'_2}}(0)}}. \end{equation}
\begin{equation} \eta=\frac{Pr^{1/10}\tilde{\tilde{\eta_1}}}{\sqrt{\tilde{\tilde{F'_2}}(0)}}. \end{equation}
Using (4.27) to convert (4.26) into ![]() $F(\eta )$, (4.19) to convert (4.25) into
$F(\eta )$, (4.19) to convert (4.25) into ![]() $H'(\eta )$, substituting these in (3.11), and using (4.28), we get
$H'(\eta )$, substituting these in (3.11), and using (4.28), we get
as the scaling law to predict ![]() $\eta _{df}$ for
$\eta _{df}$ for ![]() $Pr \ll 1$ in the small
$Pr \ll 1$ in the small ![]() $\eta$ limit. Here, the pre-factor
$\eta$ limit. Here, the pre-factor ![]() $1.75$ is chosen to match (4.29) with the data from the solution of (3.11) for
$1.75$ is chosen to match (4.29) with the data from the solution of (3.11) for ![]() $Pr<1$, plotted in figure 9(b). Figure 9(b) shows the comparison of (4.29) with the dust-free region height obtained from the numerical solutions of (3.11) for
$Pr<1$, plotted in figure 9(b). Figure 9(b) shows the comparison of (4.29) with the dust-free region height obtained from the numerical solutions of (3.11) for ![]() $Pr=0.1$ and
$Pr=0.1$ and ![]() $Pr=1$. We again note from the figure that (4.29) is valid for any
$Pr=1$. We again note from the figure that (4.29) is valid for any ![]() $Pr\le 1$, even though it was derived for
$Pr\le 1$, even though it was derived for ![]() $Pr \ll 1$.
$Pr \ll 1$.
4.2.3. Large  $\eta$ scaling for intermediate
$\eta$ scaling for intermediate  $Pr$ (
$Pr$ ( $1< Pr<10$)
$1< Pr<10$)
We saw in §§ 4.1.1 and 4.2.2 that the scaling laws for the dust-free region heights obtained in the small ![]() $\eta$ limit for
$\eta$ limit for ![]() $Pr \gg 1$ in (4.9) and for
$Pr \gg 1$ in (4.9) and for ![]() $Pr \ll 1$ in (4.29) are valid for any
$Pr \ll 1$ in (4.29) are valid for any ![]() $Pr>1$ and
$Pr>1$ and ![]() $Pr<1$. Similarly, the scaling law for the large
$Pr<1$. Similarly, the scaling law for the large ![]() $\eta$ limit for
$\eta$ limit for ![]() $Pr \ll 1$ in (4.22) was shown to be valid for any
$Pr \ll 1$ in (4.22) was shown to be valid for any ![]() $Pr<1$. However, the scaling law in the large
$Pr<1$. However, the scaling law in the large ![]() $\eta$ limit for
$\eta$ limit for ![]() $Pr \gg 1$ in (4.13) deviates from the simulation data for
$Pr \gg 1$ in (4.13) deviates from the simulation data for ![]() $Pr=2$ (see figure 8). Then in the intermediate range of
$Pr=2$ (see figure 8). Then in the intermediate range of ![]() $Pr$ values which are not much larger than one, for large
$Pr$ values which are not much larger than one, for large ![]() $Th_m$ that push the
$Th_m$ that push the ![]() $\eta _{df}$ to large
$\eta _{df}$ to large ![]() $\eta$ limits, the prediction of
$\eta$ limits, the prediction of ![]() $\eta _{df}$ becomes difficult with the results presented so far. To overcome this limitation, we now obtain an expression, by trial and error using numerical solutions of
$\eta _{df}$ becomes difficult with the results presented so far. To overcome this limitation, we now obtain an expression, by trial and error using numerical solutions of ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H'(\eta )$ obtained from (2.28), which collapse the
$H'(\eta )$ obtained from (2.28), which collapse the ![]() $\eta _{df}$ data from the solutions of (3.11) for
$\eta _{df}$ data from the solutions of (3.11) for ![]() $1< Pr<10$. A logarithmic function,
$1< Pr<10$. A logarithmic function,
and the corresponding data from the solution of (3.11) are compared in figure 10 for the large ![]() $\eta$ limit for
$\eta$ limit for ![]() $2< Pr<10$; the data of simulations collapse on to (4.30) fairly well for this range of intermediate
$2< Pr<10$; the data of simulations collapse on to (4.30) fairly well for this range of intermediate ![]() $Pr$.
$Pr$.

Figure 10. Comparison of the scaling of the dimensionless dust-free region height for ![]() $1< Pr<10$ with
$1< Pr<10$ with ![]() $\eta _{df}$ obtained from the numerical solution; blue
$\eta _{df}$ obtained from the numerical solution; blue ![]() $\triangle$,
$\triangle$, ![]() $Pr=2$; red
$Pr=2$; red ![]() $\circ$,
$\circ$, ![]() $Pr=5$; green
$Pr=5$; green ![]() $*$,
$*$, ![]() $Pr=7$; magenta
$Pr=7$; magenta ![]() $\diamond$,
$\diamond$, ![]() $Pr=10$; – – – –, (4.30).
$Pr=10$; – – – –, (4.30).
5. Conclusions
The primary contribution of the present work includes the identification of a saddle point and a separatrix for particle flows inside natural convection boundary layers over horizontal surfaces and the scaling of the dust-free region boundary in such flows for the whole range of Prandtl numbers, ![]() $Pr\ll 1$ to
$Pr\ll 1$ to ![]() $Pr\gg 1$. The saddle point and the separatrix were identified by studying the particle trajectories obtained by simulating the motion of low-Stokes-number (
$Pr\gg 1$. The saddle point and the separatrix were identified by studying the particle trajectories obtained by simulating the motion of low-Stokes-number (![]() $\widehat {St}$ (2.8)) particles in the flow field of laminar natural convection boundary layer given by Rotem & Claassen (Reference Rotem and Claassen1969), by including thermophoretic force, particle inertia, viscous drag and gravitational force. The saddle point and the four separatrices that originate from it were shown to divide the flow field of particles into four regions. The regions below the two separatrices leaving the saddle point always turned into a dust-free zone. For a given fluid and flow conditions, the size of this zone was shown to depend on the gravitational number,
$\widehat {St}$ (2.8)) particles in the flow field of laminar natural convection boundary layer given by Rotem & Claassen (Reference Rotem and Claassen1969), by including thermophoretic force, particle inertia, viscous drag and gravitational force. The saddle point and the four separatrices that originate from it were shown to divide the flow field of particles into four regions. The regions below the two separatrices leaving the saddle point always turned into a dust-free zone. For a given fluid and flow conditions, the size of this zone was shown to depend on the gravitational number, ![]() $Gn$ in (2.13), and the thermophoretic number,
$Gn$ in (2.13), and the thermophoretic number, ![]() $Th$ in (2.11), when
$Th$ in (2.11), when ![]() $\widehat {St}\ll 1$ and Brownian effects were not significant. The size of the dust-free zone was shown to decrease with an increase in
$\widehat {St}\ll 1$ and Brownian effects were not significant. The size of the dust-free zone was shown to decrease with an increase in ![]() $Gn$ and diminish to zero when
$Gn$ and diminish to zero when ![]() $Gn\gg Th_m$.
$Gn\gg Th_m$.
For small particle sizes and appreciable temperature differences across the boundary layer, so that ![]() $\widehat {St}\ll 1$ and
$\widehat {St}\ll 1$ and ![]() $Gn\ll Th$, the boundary of the dust-free region was shown to be parallel to the boundary layer edge, but smaller in height and dependent on the values of
$Gn\ll Th$, the boundary of the dust-free region was shown to be parallel to the boundary layer edge, but smaller in height and dependent on the values of ![]() $Th$ and
$Th$ and ![]() $Pr$. For such commonly occurring conditions, since the separatrix formed the boundary of the dust-free region, by considering the dominant velocity components on the separatrix, we obtained a differential equation (3.7) for the particle trajectory on these separatrices, and from which, an equation (3.11) for the dimensionless boundary layer
$Pr$. For such commonly occurring conditions, since the separatrix formed the boundary of the dust-free region, by considering the dominant velocity components on the separatrix, we obtained a differential equation (3.7) for the particle trajectory on these separatrices, and from which, an equation (3.11) for the dimensionless boundary layer ![]() $y$-coordinate (
$y$-coordinate (![]() $\eta _{df}$) associated with the separatrix. This equation showed that on the separatrix, which is the boundary of the dust-free region, the dimensionless stream function
$\eta _{df}$) associated with the separatrix. This equation showed that on the separatrix, which is the boundary of the dust-free region, the dimensionless stream function ![]() $F(\eta )$ in (2.19) is proportional to the dimensionless derivative (
$F(\eta )$ in (2.19) is proportional to the dimensionless derivative (![]() $\partial /{\partial \eta }$) of the dimensionless temperature function
$\partial /{\partial \eta }$) of the dimensionless temperature function ![]() $H(\eta )$ in (2.20); the proportionality coefficient being
$H(\eta )$ in (2.20); the proportionality coefficient being ![]() $-(5/3)(Th/\sqrt {Pr})$. The numerical solution of this equation was shown to predict the dust-free regions very well at all locations of the boundary layer, except for a slight deviation close to the leading edge. However, (3.11) was not an equation that gave an explicit scaling relation for the dimensionless dust-free region boundary
$-(5/3)(Th/\sqrt {Pr})$. The numerical solution of this equation was shown to predict the dust-free regions very well at all locations of the boundary layer, except for a slight deviation close to the leading edge. However, (3.11) was not an equation that gave an explicit scaling relation for the dimensionless dust-free region boundary ![]() $\eta _{df}$; further, the solution of it required the similarity solutions of the boundary layer equations, corresponding to each
$\eta _{df}$; further, the solution of it required the similarity solutions of the boundary layer equations, corresponding to each ![]() $Pr$.
$Pr$.
Using the limiting forms of ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $H(\eta )$ for
$H(\eta )$ for ![]() $\eta \ll 1$, which implied small
$\eta \ll 1$, which implied small ![]() $Th$, in (3.11) for the dimensionless dust-free region height,
$Th$, in (3.11) for the dimensionless dust-free region height, ![]() $\eta _{df}=y_{df}/[Pr(x^2Z_w ^3)^{1/5}]$, where
$\eta _{df}=y_{df}/[Pr(x^2Z_w ^3)^{1/5}]$, where ![]() $Z_w$(2.3) is the near wall length scale in turbulent convection, we then obtained the scaling of dust-free region heights. In both the
$Z_w$(2.3) is the near wall length scale in turbulent convection, we then obtained the scaling of dust-free region heights. In both the ![]() $Pr$ cases,
$Pr$ cases, ![]() $\eta _{df}$ scaled as
$\eta _{df}$ scaled as ![]() $\sqrt {Th_m}$, with the
$\sqrt {Th_m}$, with the ![]() $Pr$ dependence being
$Pr$ dependence being ![]() $Pr^{3/10}$ for
$Pr^{3/10}$ for ![]() $Pr\gg 1$ and
$Pr\gg 1$ and ![]() $Pr^{7/20}$ for
$Pr^{7/20}$ for ![]() $Pr\ll 1$ (see (4.9) and (4.29)). For the large
$Pr\ll 1$ (see (4.9) and (4.29)). For the large ![]() $\eta$ cases,
$\eta$ cases, ![]() $\eta _{df}$ had a more complex dependence for large and small
$\eta _{df}$ had a more complex dependence for large and small ![]() $Pr$;
$Pr$; ![]() $\eta _{df}$ showed a dependence on the square root of the Lambert function of
$\eta _{df}$ showed a dependence on the square root of the Lambert function of ![]() $Th^2Pr$ (see (4.13)) for
$Th^2Pr$ (see (4.13)) for ![]() $Pr\gg 1$ and a dependence on the logarithm of
$Pr\gg 1$ and a dependence on the logarithm of ![]() $Th \sqrt {Pr}$ for
$Th \sqrt {Pr}$ for ![]() $Pr\ll 1$ (see (4.22)). All of these scalings matched well with the numerical solution of (3.11) even for the corresponding
$Pr\ll 1$ (see (4.22)). All of these scalings matched well with the numerical solution of (3.11) even for the corresponding ![]() $Pr\le 1$ and
$Pr\le 1$ and ![]() $Pr\ge 1$ cases, except for the
$Pr\ge 1$ cases, except for the ![]() $Pr\gg 1$,
$Pr\gg 1$, ![]() $\eta \gg 1$ scaling in (4.13), which matched with (3.11) only for
$\eta \gg 1$ scaling in (4.13), which matched with (3.11) only for ![]() $Pr\ge 100$. A scaling for
$Pr\ge 100$. A scaling for ![]() $\eta _{df}$ in the practically relevant regime of
$\eta _{df}$ in the practically relevant regime of ![]() $1< Pr < 10$ for
$1< Pr < 10$ for ![]() $\eta \gg 1$ is then empirically obtained as
$\eta \gg 1$ is then empirically obtained as ![]() $\eta _{df} \sim Pr^{-0.39}\ln (Th_mPr)$ (see (4.30)) so as to obtain the scaling of the dust-free region in the whole range of
$\eta _{df} \sim Pr^{-0.39}\ln (Th_mPr)$ (see (4.30)) so as to obtain the scaling of the dust-free region in the whole range of ![]() $Pr\ll 1$ to
$Pr\ll 1$ to ![]() $Pr \gg 1$.
$Pr \gg 1$.
The present study neglected Brownian effects, which become important with the decrease in the size of the particles. Brownian effects on the particles depend just on the absolute value of the temperature, whereas thermophoretic effects depend on the gradient of temperature across particles, which keeps increasing as we move towards the origin of the boundary layer. So, even in the presence of Brownian effects, we still expect the presence of a similar dust-free region above a hot horizontal plate. As the Brownian effects increase, one may expect the height of the dust-free region to decrease, finally making it bounded, as we had observed in the gravitational case. A further increase in Brownian effects may reduce the dust-free region size close to zero and confine it around the leading edge of the boundary layer. These effects, and the similarities that the dust-free region may show in such cases to the case when gravitational effects are increased, need to be investigated.
As we saw earlier, since at intermediate ![]() $Ra$ the local boundary layers on either side of the plumes in Rayleigh–Bénard convection could be modelled as a laminar natural convection boundary layer (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil Reference Puthenveettil2004; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005) the dust-free region heights predicted by the present study could be expected to occur near the hot plate in Rayleigh–Bénard convection at intermediate
$Ra$ the local boundary layers on either side of the plumes in Rayleigh–Bénard convection could be modelled as a laminar natural convection boundary layer (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil Reference Puthenveettil2004; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005) the dust-free region heights predicted by the present study could be expected to occur near the hot plate in Rayleigh–Bénard convection at intermediate ![]() $Ra$. The upper limit for
$Ra$. The upper limit for ![]() $Ra$ is discussed in Appendix E in detail for
$Ra$ is discussed in Appendix E in detail for ![]() $Pr=0.7$ and aspect ratio of
$Pr=0.7$ and aspect ratio of ![]() $1$. However, when
$1$. However, when ![]() $Ra$ is higher, these local boundary layers are sheared by the large-scale flow in the bulk, changing their nature to mixed convection boundary layers (Shevkar, Mohanan & Puthenveettil Reference Shevkar, Mohanan and Puthenveettil2023). Hence, the results of the present study may not be valid for high-
$Ra$ is higher, these local boundary layers are sheared by the large-scale flow in the bulk, changing their nature to mixed convection boundary layers (Shevkar, Mohanan & Puthenveettil Reference Shevkar, Mohanan and Puthenveettil2023). Hence, the results of the present study may not be valid for high-![]() $Ra$ Rayleigh–Bénard convection, even though the local boundary layers remain.
$Ra$ Rayleigh–Bénard convection, even though the local boundary layers remain.
Supplementary movies
Supplementary movies are available at https://doi.org/10.1017/jfm.2024.786.
Acknowledgements
The authors would like to acknowledge the partial financial support from DST, Government of India under the Core research grant CRG/2021/007497 and the FIST grants SR/FST/ETII-017/2003; SR/FST/ETII-064/2015 for this study.
Declaration of interests
The authors report no conflict of interest.
Appendix A. Dilute mixture assumption
The present study is done for ![]() $\widehat {St}=10^{-5}$ with the number of particles
$\widehat {St}=10^{-5}$ with the number of particles ![]() $N=5000$ in a domain of
$N=5000$ in a domain of ![]() $H= 40Z_w$ and
$H= 40Z_w$ and ![]() $L=50Z_w$. The area fraction that
$L=50Z_w$. The area fraction that ![]() $N$ particles of radius
$N$ particles of radius ![]() $a$ occupy is then
$a$ occupy is then
Since
 \begin{equation} Z_w = \frac{2^{1/3}H}{Ra_H^{1/3}}, \end{equation}
\begin{equation} Z_w = \frac{2^{1/3}H}{Ra_H^{1/3}}, \end{equation}where
is the Rayleigh number, (A1) can be written as
Using the expression for (2.8), (2.9) and (A2), for ![]() $\widehat {St}=10^{-5}$,
$\widehat {St}=10^{-5}$,
 \begin{equation} \frac{a}{H}= \left(\frac{2^{4/3}\times 40^2\times10^{{-}5}\times9}{C_B} \frac{\epsilon}{2+\epsilon}\right)^{1/2}\frac{Pr^{1/4}}{Ra_H^{2/3}}. \end{equation}
\begin{equation} \frac{a}{H}= \left(\frac{2^{4/3}\times 40^2\times10^{{-}5}\times9}{C_B} \frac{\epsilon}{2+\epsilon}\right)^{1/2}\frac{Pr^{1/4}}{Ra_H^{2/3}}. \end{equation}Substituting (A5) in (A4), we obtain
 \begin{equation} \phi= \frac{N\times3.6\times 10^{{-}4}}{C_B} \frac{\epsilon}{2+\epsilon}\frac{\sqrt{Pr}}{Ra_H^{2/3}}. \end{equation}
\begin{equation} \phi= \frac{N\times3.6\times 10^{{-}4}}{C_B} \frac{\epsilon}{2+\epsilon}\frac{\sqrt{Pr}}{Ra_H^{2/3}}. \end{equation}
The dilute mixture assumption is valid for ![]() $\phi \ll 1$. Using the values of
$\phi \ll 1$. Using the values of ![]() $N=5000$,
$N=5000$, ![]() $Pr=0.7$ and
$Pr=0.7$ and ![]() $C_B\simeq 1$ for particles in air, with
$C_B\simeq 1$ for particles in air, with ![]() $\epsilon /(2+\epsilon )\rightarrow 1/2$ when
$\epsilon /(2+\epsilon )\rightarrow 1/2$ when ![]() $\epsilon \rightarrow 0$ for particles in air, we obtain
$\epsilon \rightarrow 0$ for particles in air, we obtain
For the usual range of ![]() $Ra_H>10^6$, for which boundary layers form on the hot plate,
$Ra_H>10^6$, for which boundary layers form on the hot plate, ![]() $\phi <7.5\times 10^{-5}\ll 1$; the influence of particles on the flow field can then be ignored.
$\phi <7.5\times 10^{-5}\ll 1$; the influence of particles on the flow field can then be ignored.
Appendix B. Dimensionless stream function and thermophoretic velocity
In this section, we derive expressions for the dimensionless stream function used to obtain ![]() $u_{x}^*$ and
$u_{x}^*$ and ![]() $u_{y}^*$ in (2.26) and (2.27) and the dimensionless thermophoretic velocity components,
$u_{y}^*$ in (2.26) and (2.27) and the dimensionless thermophoretic velocity components, ![]() $u_{t_x}^*$ and
$u_{t_x}^*$ and ![]() $u_{t_y}^*$, used in (3.5).
$u_{t_y}^*$, used in (3.5).
B.1. Dimensionless stream function
Using (2.3) and (2.24), we get
Substituting (B1) and (2.3) in (2.21), we get
The dimensionless stream function, ![]() $\psi ^*$ in (2.19), can now be expressed in terms of
$\psi ^*$ in (2.19), can now be expressed in terms of ![]() $x^*$ by using (2.3), (2.22) and (B1) as
$x^*$ by using (2.3), (2.22) and (B1) as
The dimensionless fluid velocity components ![]() $u_x^* = {\partial \psi ^*}/{\partial \hat {y}}$ in (2.26) and
$u_x^* = {\partial \psi ^*}/{\partial \hat {y}}$ in (2.26) and ![]() $u_y^* = - {\partial \psi ^*}/{\partial {x_{rc}}^*}$ in (2.27) can now be obtained from (B3). Since
$u_y^* = - {\partial \psi ^*}/{\partial {x_{rc}}^*}$ in (2.27) can now be obtained from (B3). Since ![]() $F'(\eta )$ is always positive (see figure 2), it is clear from (2.26) that the horizontal component of fluid velocity,
$F'(\eta )$ is always positive (see figure 2), it is clear from (2.26) that the horizontal component of fluid velocity, ![]() $u_x^*$ is always positive. Equations (2.26) and (2.27) imply that along any constant
$u_x^*$ is always positive. Equations (2.26) and (2.27) imply that along any constant ![]() $\eta$ lines,
$\eta$ lines, ![]() $u_x^*$ monotonically increases with increase in
$u_x^*$ monotonically increases with increase in ![]() $x^*$, while the magnitude of vertical velocity,
$x^*$, while the magnitude of vertical velocity, ![]() $u_y^*$, decreases with increase in
$u_y^*$, decreases with increase in ![]() $x^*$.
$x^*$.
B.2. Dimensionless thermophoretic velocity components
Using (2.12) in (2.17), we can re-write the dimensionless thermophoretic velocity as
Comparing (2.17) and (B4), the thermophoretic number can be written as
Using
(B2), (B5) and (2.20) in (B4), the horizontal component of thermophoretic velocity becomes
Similarly, the vertical component of the thermophoretic velocity can be written as
Note that ![]() $u^*_{t_x}$ is always negative and
$u^*_{t_x}$ is always negative and ![]() $u^*_{t_y}$ is always positive as
$u^*_{t_y}$ is always positive as ![]() $H'$ is always negative (see figure 2). It is also evident from (B7) and (B8) that the magnitudes of
$H'$ is always negative (see figure 2). It is also evident from (B7) and (B8) that the magnitudes of ![]() $u^*_{t_x}$ and
$u^*_{t_x}$ and ![]() $u^*_{t_y}$ decrease as
$u^*_{t_y}$ decrease as ![]() $x^*$ increases along any constant
$x^*$ increases along any constant ![]() $\eta$ lines.
$\eta$ lines.
Appendix C. Limiting behaviours of dimensionless stream, temperature and pressure functions for  $\eta \rightarrow 0$
$\eta \rightarrow 0$
C.1. For  $0.1\le Pr \le 10$, behaviour of
$0.1\le Pr \le 10$, behaviour of  $F(\eta )$,
$F(\eta )$,  $G(\eta )$ and
$G(\eta )$ and  $H(\eta )$ near
$H(\eta )$ near  $\eta =0$
$\eta =0$
For small values of ![]() $\eta$, a first-order approximation to
$\eta$, a first-order approximation to ![]() $F(\eta )$ (see figure 2) can be made as
$F(\eta )$ (see figure 2) can be made as
where the coefficient ![]() $A$ and the exponent
$A$ and the exponent ![]() $\alpha$ are real numbers. The higher order terms in
$\alpha$ are real numbers. The higher order terms in ![]() $\eta$ can be neglected as
$\eta$ can be neglected as ![]() $\eta \rightarrow 0$. This approximation ensures that both
$\eta \rightarrow 0$. This approximation ensures that both ![]() $F(\eta )$ and
$F(\eta )$ and ![]() $F'(\eta )$ go to zero (see boundary conditions in (2.30a)) as
$F'(\eta )$ go to zero (see boundary conditions in (2.30a)) as ![]() $\eta \rightarrow 0$ when
$\eta \rightarrow 0$ when ![]() $\alpha$ is greater than one. Similarly, a first-order approximation of the decreasing function
$\alpha$ is greater than one. Similarly, a first-order approximation of the decreasing function ![]() $H(\eta )$ (see figure 2) can be made as
$H(\eta )$ (see figure 2) can be made as
where the coefficient ![]() $B$ and the exponent
$B$ and the exponent ![]() $\beta$ are real numbers. This approximation ensures that
$\beta$ are real numbers. This approximation ensures that ![]() $H(\eta ) \rightarrow 1$ as
$H(\eta ) \rightarrow 1$ as ![]() $\eta \rightarrow 0$, which is a boundary condition requirement in (2.30a), when
$\eta \rightarrow 0$, which is a boundary condition requirement in (2.30a), when ![]() $\beta$ is a positive real number. Using (C2) in (2.28b), we get the first-order approximation for
$\beta$ is a positive real number. Using (C2) in (2.28b), we get the first-order approximation for ![]() $G(\eta )$ at small values of
$G(\eta )$ at small values of ![]() $\eta$ as
$\eta$ as
where ![]() $C$ is a real constant which is independent of
$C$ is a real constant which is independent of ![]() $\eta$.
$\eta$.
We now use (C1), (C2) and (C3) in the boundary layer equations (2.28) to obtain the values of ![]() $\alpha$ and
$\alpha$ and ![]() $\beta$. Using (C1), (C2) in (2.28c) and dividing by
$\beta$. Using (C1), (C2) in (2.28c) and dividing by ![]() $-\beta B \eta ^{\beta -2}$, we get
$-\beta B \eta ^{\beta -2}$, we get
Since ![]() $\alpha$ is a real number greater than one, the second term on the left-hand side of (C4) goes to zero as
$\alpha$ is a real number greater than one, the second term on the left-hand side of (C4) goes to zero as ![]() $\eta \rightarrow 0$. In this limit,
$\eta \rightarrow 0$. In this limit, ![]() $(\beta -1)$ has to be then zero, i.e.
$(\beta -1)$ has to be then zero, i.e.
Using (C3), (C1) and (C5) in (2.28a) and dividing by ![]() $\eta ^{\alpha -3}$, we obtain
$\eta ^{\alpha -3}$, we obtain
The second term on the left-hand side goes to zero as ![]() $\eta \rightarrow 0$ since
$\eta \rightarrow 0$ since ![]() $\alpha >1$ and is real. In this limit,
$\alpha >1$ and is real. In this limit, ![]() $\alpha$ has to be then less than 3 for the third and fourth term in (C6) to tend to zero. To balance the equation when
$\alpha$ has to be then less than 3 for the third and fourth term in (C6) to tend to zero. To balance the equation when ![]() $\eta \rightarrow 0$, the first term has to be zero. This implies that since
$\eta \rightarrow 0$, the first term has to be zero. This implies that since ![]() $A\neq 0$ and
$A\neq 0$ and ![]() $\alpha >1$,
$\alpha >1$, ![]() $\alpha -2=0$, i.e.
$\alpha -2=0$, i.e.
Using (C5) and (C7), we can re-write (C1), (C2) and (C3) for ![]() $\eta \rightarrow 0$ as
$\eta \rightarrow 0$ as
So we see that for small values of ![]() $\eta$,
$\eta$, ![]() $F(\eta )$ is a quadratic function,
$F(\eta )$ is a quadratic function, ![]() $H(\eta )$ is a linear function and
$H(\eta )$ is a linear function and ![]() $G(\eta )$ is a quadratic polynomial in
$G(\eta )$ is a quadratic polynomial in ![]() $\eta$.
$\eta$.
C.2. For  $Pr\gg 1$, the behaviour of
$Pr\gg 1$, the behaviour of  $\tilde {F}_1(\tilde {\eta }_1)$,
$\tilde {F}_1(\tilde {\eta }_1)$,  $\tilde {G}_1(\tilde {\eta }_1)$ and
$\tilde {G}_1(\tilde {\eta }_1)$ and  $\tilde {H_1}(\tilde {\eta }_1)$ near
$\tilde {H_1}(\tilde {\eta }_1)$ near  $\tilde {\eta }_1=0$
$\tilde {\eta }_1=0$
A similar first-order approximation as in (C1) and (C2) can be made for ![]() $\tilde {F_1}(\tilde {\eta }_1)$ and
$\tilde {F_1}(\tilde {\eta }_1)$ and ![]() $\tilde {H_1}(\tilde {\eta }_1)$ in terms of
$\tilde {H_1}(\tilde {\eta }_1)$ in terms of ![]() $\tilde {\eta }_1$ as
$\tilde {\eta }_1$ as
when ![]() $\tilde {\eta }_1 \rightarrow 0$. For
$\tilde {\eta }_1 \rightarrow 0$. For ![]() $\tilde {F'_1}(\tilde {\eta }_1)$ to approach zero and
$\tilde {F'_1}(\tilde {\eta }_1)$ to approach zero and ![]() $\tilde {H_1}(\tilde {\eta }_1)$ to approach one when
$\tilde {H_1}(\tilde {\eta }_1)$ to approach one when ![]() $\tilde {\eta }_1 \rightarrow 0$ as per § 4.2a,
$\tilde {\eta }_1 \rightarrow 0$ as per § 4.2a, ![]() $\tilde {\alpha }$ has to be greater than one and
$\tilde {\alpha }$ has to be greater than one and ![]() $\tilde {\beta }$ has to be positive. Using (C9) in (4.1c) gives
$\tilde {\beta }$ has to be positive. Using (C9) in (4.1c) gives
Since ![]() $\tilde {\alpha }>1$, for
$\tilde {\alpha }>1$, for ![]() $\tilde {\eta }_1 \rightarrow 0$, the above equation can only be satisfied if
$\tilde {\eta }_1 \rightarrow 0$, the above equation can only be satisfied if
Using (C9) in (4.1b) to find ![]() $\tilde {G}$ provides a similar expression as (C3),
$\tilde {G}$ provides a similar expression as (C3),
 \begin{equation} \tilde{G}_1(\tilde{\eta}_1) \approx \tilde{\eta}_1 - \frac{\tilde{B} \tilde{\eta}^{\tilde{\beta}+1}}{{\tilde{\beta}+1}} + \tilde{C}, \end{equation}
\begin{equation} \tilde{G}_1(\tilde{\eta}_1) \approx \tilde{\eta}_1 - \frac{\tilde{B} \tilde{\eta}^{\tilde{\beta}+1}}{{\tilde{\beta}+1}} + \tilde{C}, \end{equation}
where ![]() $\tilde {C}$ is the constant of integration. Using the above results in (4.1a) gives
$\tilde {C}$ is the constant of integration. Using the above results in (4.1a) gives
where all the terms except the first term goes to zero when ![]() $\tilde {\alpha }<3$ for
$\tilde {\alpha }<3$ for ![]() $\tilde {\eta }_1 \rightarrow 0$. Since
$\tilde {\eta }_1 \rightarrow 0$. Since ![]() $\tilde {\alpha }>1$, (C13) can be satisfied only if
$\tilde {\alpha }>1$, (C13) can be satisfied only if
So it is found that ![]() $\tilde {F}_1$ is quadratic and
$\tilde {F}_1$ is quadratic and ![]() $\tilde {H}_1$ is linear near
$\tilde {H}_1$ is linear near ![]() $\tilde {\eta }_1$ equals zero.
$\tilde {\eta }_1$ equals zero.
C.3. For  $Pr\ll 1$, the behaviour of
$Pr\ll 1$, the behaviour of  $\tilde {\tilde {F_1}}(\tilde {\tilde {\eta }}_1)$,
$\tilde {\tilde {F_1}}(\tilde {\tilde {\eta }}_1)$,  $\tilde {\tilde {G_1}}(\tilde {\tilde {\eta }}_1)$ and
$\tilde {\tilde {G_1}}(\tilde {\tilde {\eta }}_1)$ and  $\tilde {\tilde {H_1}}(\tilde {\tilde {\eta }}_1)$ near
$\tilde {\tilde {H_1}}(\tilde {\tilde {\eta }}_1)$ near  $\tilde {\tilde {\eta }}_1=0$
$\tilde {\tilde {\eta }}_1=0$
An exactly similar approximation as in (C1) and (C9a) is used for ![]() $\tilde {\tilde {F_1}}(\tilde {\tilde {\eta }}_1)$ in (4.23a) to get
$\tilde {\tilde {F_1}}(\tilde {\tilde {\eta }}_1)$ in (4.23a) to get
For ![]() $\tilde {\tilde {F}}^{\prime}_1$ to tend to zero, as
$\tilde {\tilde {F}}^{\prime}_1$ to tend to zero, as ![]() $\tilde {\tilde {\eta }}_1 \rightarrow 0$,
$\tilde {\tilde {\eta }}_1 \rightarrow 0$, ![]() $\tilde {\tilde {\alpha }}$ has to be greater than one. So the second and third terms in (C15) tend to zero for
$\tilde {\tilde {\alpha }}$ has to be greater than one. So the second and third terms in (C15) tend to zero for ![]() $\tilde {\tilde {\alpha }}<3$ for small
$\tilde {\tilde {\alpha }}<3$ for small ![]() $\tilde {\tilde {\eta }}_1$. So (C15) can be satisfied only if
$\tilde {\tilde {\eta }}_1$. So (C15) can be satisfied only if
implying that ![]() $\tilde {\tilde {F}}_1$ is quadratic near
$\tilde {\tilde {F}}_1$ is quadratic near ![]() $\tilde {\tilde {\eta }}_1$ equals zero.
$\tilde {\tilde {\eta }}_1$ equals zero.
Appendix D. Rotem & Claassen's (Reference Rotem and Claassen1969) boundary layer parameters
D.1. Intermediate range of  $Pr$ (
$Pr$ ( $O(0.1)\le Pr \le O(10)$)
$O(0.1)\le Pr \le O(10)$)
For ![]() $O(0.1) \le Pr \le O(10)$, (2.28a–c) give the relevant boundary layer equations. The stretched variables used to obtain (2.28) are
$O(0.1) \le Pr \le O(10)$, (2.28a–c) give the relevant boundary layer equations. The stretched variables used to obtain (2.28) are
where ![]() ${u_{rc}^*}=u/(\nu /L)$ and
${u_{rc}^*}=u/(\nu /L)$ and ![]() ${v_{rc}^*}=v/(\nu /L)$. The similarity transformations used are given by (2.19), (2.20) and (2.21). Velocity components and stream function are related in the usual way as
${v_{rc}^*}=v/(\nu /L)$. The similarity transformations used are given by (2.19), (2.20) and (2.21). Velocity components and stream function are related in the usual way as
Using (D2a), (2.19) and (2.21), we get
while using (D2b), (2.19) and (2.21), we get
D.2. Large  $Pr$ (
$Pr$ ( $Pr\gg 1$)
$Pr\gg 1$)
The stretched variables used to obtain the inner boundary layer equations (4.1) for ![]() $Pr \gg 1$ are
$Pr \gg 1$ are
The similarity transformations used are
The pressure function is defined as
From the stream function, we can get the velocity components in the usual way as
Using (D8), and the definition of stream function and the similarity variable respectively in (D6a) and (D6c), we can write
and
D.3. Small  $Pr$ (
$Pr$ ( $Pr\ll 1$)
$Pr\ll 1$)
The outer boundary layer equations (4.14) for ![]() $Pr \ll 1$ are obtained by using the stretched variables,
$Pr \ll 1$ are obtained by using the stretched variables,
and the similarity transformations,
The pressure function used is
Velocity components are obtained from the stream function as
 \begin{equation} \tilde{\tilde{u}} = \frac{\partial\tilde{\tilde{\psi}}}{\partial\tilde{\tilde{y}}} \quad \mathrm{and} \quad \tilde{\tilde{v}} ={-}\frac{\partial\tilde{\tilde{\psi}}}{\partial \tilde{\tilde{x}}}. \end{equation}
\begin{equation} \tilde{\tilde{u}} = \frac{\partial\tilde{\tilde{\psi}}}{\partial\tilde{\tilde{y}}} \quad \mathrm{and} \quad \tilde{\tilde{v}} ={-}\frac{\partial\tilde{\tilde{\psi}}}{\partial \tilde{\tilde{x}}}. \end{equation}Using (D12a) and (D12c) in (D14), we can write
and
D.4. Conversion of variables from Rotem & Claassen (Reference Rotem and Claassen1969) to Pera & Gebhart (Reference Pera and Gebhart1973)
A conversion to Pera & Gebhart's (Reference Pera and Gebhart1973) variables is possible from Rotem & Claassen's (Reference Rotem and Claassen1969) and is provided in this section. Dimensional velocity components and temperature are calculated using both Rotem & Claassen's (Reference Rotem and Claassen1969) variables and Pera & Gebhart's (Reference Pera and Gebhart1973) variables, and then equated to obtain the following relationships among them:
where ![]() $\eta _{pg}=(y/x) (Gr_x/5)^{1/5}$ is the similarity variable used by Pera and Gebhart, and
$\eta _{pg}=(y/x) (Gr_x/5)^{1/5}$ is the similarity variable used by Pera and Gebhart, and ![]() $f(\eta _{pg})$ and
$f(\eta _{pg})$ and ![]() $\theta (\eta _{pg})$ are the corresponding velocity and temperature functions.
$\theta (\eta _{pg})$ are the corresponding velocity and temperature functions.
Appendix E. Upper limit of Ra for the present analysis
We now estimate the upper limit of the present analysis by estimating the ![]() $Ra$ at which the local boundary layers would be affected by the external shear due to the global boundary layer, so as to change their nature from a laminar natural convection boundary layer to a mixed convection boundary layer.
$Ra$ at which the local boundary layers would be affected by the external shear due to the global boundary layer, so as to change their nature from a laminar natural convection boundary layer to a mixed convection boundary layer.
Equation (4.10) of Shevkar et al. (Reference Shevkar, Mohanan and Puthenveettil2023) gives the characteristic velocity (![]() $U_c$) inside the local boundary layers that are subjected to shear by the global boundary layers. These are given as a perturbation series in Péclet number (
$U_c$) inside the local boundary layers that are subjected to shear by the global boundary layers. These are given as a perturbation series in Péclet number (![]() $Pe_\lambda$) based on
$Pe_\lambda$) based on ![]() $U_c$ and the no-shear plume spacing
$U_c$ and the no-shear plume spacing ![]() $\lambda _0$ as
$\lambda _0$ as
where
is a small parameter with ![]() $Re_\lambda =V_F\lambda _0/\nu$ and
$Re_\lambda =V_F\lambda _0/\nu$ and ![]() $Ra_\lambda =g\beta \Delta T_w\lambda _0^3/\nu \alpha$ being the Reynolds and Rayleigh numbers based on
$Ra_\lambda =g\beta \Delta T_w\lambda _0^3/\nu \alpha$ being the Reynolds and Rayleigh numbers based on ![]() $\lambda _0$, with
$\lambda _0$, with ![]() $V_F$ being the large-scale flow velocity and
$V_F$ being the large-scale flow velocity and ![]() $\Delta T_w$ the temperature drop across the local boundary layer. There is no effect of shear on the local boundary layers when
$\Delta T_w$ the temperature drop across the local boundary layer. There is no effect of shear on the local boundary layers when
in (E1).
As given by Shevkar et al. (Reference Shevkar, Mohanan and Puthenveettil2023),
 \begin{equation} Pe_{\lambda0}=\left(\frac{C_2}{8E}\right)^{2/5}Ra_\lambda^{2/5} \end{equation}
\begin{equation} Pe_{\lambda0}=\left(\frac{C_2}{8E}\right)^{2/5}Ra_\lambda^{2/5} \end{equation}and
where ![]() $E=f(Pr)=5$ for
$E=f(Pr)=5$ for ![]() $Pr=0.7$,
$Pr=0.7$, ![]() $n=0.5$,
$n=0.5$, ![]() $C_2=0.72$,
$C_2=0.72$, ![]() $A=u|_{z=\delta }/V_F$, the dimensionless velocity at the edge of the local boundary layer. The
$A=u|_{z=\delta }/V_F$, the dimensionless velocity at the edge of the local boundary layer. The ![]() $Re_\lambda$ in (E2) can be rewritten as
$Re_\lambda$ in (E2) can be rewritten as
with
and
where ![]() $\varGamma$ is the aspect ratio of the convection cell (Shevkar et al. Reference Shevkar, Mohanan and Puthenveettil2023),
$\varGamma$ is the aspect ratio of the convection cell (Shevkar et al. Reference Shevkar, Mohanan and Puthenveettil2023), ![]() $C_1=47.5$,
$C_1=47.5$, ![]() $n_1=0.1$ and
$n_1=0.1$ and ![]() $Z_w=(\nu \alpha /g\beta \Delta T_w)^{1/3}$ the near-wall length scale in convection (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011). Substituting (E7), (E8) and (E9) in (E6), and the resulting
$Z_w=(\nu \alpha /g\beta \Delta T_w)^{1/3}$ the near-wall length scale in convection (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011). Substituting (E7), (E8) and (E9) in (E6), and the resulting ![]() $Re_\lambda$ in (E2), with
$Re_\lambda$ in (E2), with ![]() $Ra_\lambda$ from (E7), we obtain
$Ra_\lambda$ from (E7), we obtain
Substituting (E4), (E5) and (E10) in (E3), we obtain the Rayleigh number below which shear does not affect the local boundary layers as
 \begin{equation} Ra^{1/9}<\left(\frac{C_1C_2^2E^3}{8}\right)^{1/5}\frac{5}{2^{1/3}1.169} \frac{\varGamma^{0.57}}{A}Pr^{n_1/5+n+2/3}. \end{equation}
\begin{equation} Ra^{1/9}<\left(\frac{C_1C_2^2E^3}{8}\right)^{1/5}\frac{5}{2^{1/3}1.169} \frac{\varGamma^{0.57}}{A}Pr^{n_1/5+n+2/3}. \end{equation}
Since the thickness of the local boundary layers are an order smaller than the thickness of the global boundary layer, ![]() $A\simeq 0.1$. Then for
$A\simeq 0.1$. Then for ![]() $Pr=0.7$, at
$Pr=0.7$, at ![]() $\varGamma =1$, the local boundary layers are not affected by the shear due to the global boundary layer at
$\varGamma =1$, the local boundary layers are not affected by the shear due to the global boundary layer at
Since the present study is limited to the case were the local boundary layers retain their natural convection boundary layer nature, (E12) gives the upper limit of the present study.
Appendix F. Validity of Oberbeck–Boussinesq (OB) approximation in the present study
The violation of OB approximation due to temperature variations occurs (Barletta Reference Barletta2022) when
The smaller the value of ![]() $\beta \Delta T$, the better the approximation. For the case of air that we consider in the present study, since
$\beta \Delta T$, the better the approximation. For the case of air that we consider in the present study, since ![]() $\beta \simeq 1/T_{ref}$, (F1) gives a value of
$\beta \simeq 1/T_{ref}$, (F1) gives a value of
for the violation of OB approximation. For the usually encountered bulk temperature of ![]() $T_{ref}\simeq 25\,^\circ {\rm C}$, (F2) then means that
$T_{ref}\simeq 25\,^\circ {\rm C}$, (F2) then means that ![]() $\Delta T > 25\,^\circ {\rm C}$, for the violation of the OB approximation. This is of similar order to the value of
$\Delta T > 25\,^\circ {\rm C}$, for the violation of the OB approximation. This is of similar order to the value of ![]() $\Delta T>28.6\,^\circ {\rm C}$ obtained by Gray & Giorgini (Reference Gray and Giorgini1976) for air at
$\Delta T>28.6\,^\circ {\rm C}$ obtained by Gray & Giorgini (Reference Gray and Giorgini1976) for air at ![]() $15\,^\circ$C using a more rigorous analysis. The present study is limited to the case where the temperature difference across the boundary layer thickness is
$15\,^\circ$C using a more rigorous analysis. The present study is limited to the case where the temperature difference across the boundary layer thickness is ![]() $\Delta T_w<10\,^\circ {\rm C}$. Hence, the OB approximation is far from violated in the present study.
$\Delta T_w<10\,^\circ {\rm C}$. Hence, the OB approximation is far from violated in the present study.
Alternatively, the OB approximation also gets violated when the length scales involved become large, so that the Gebhart number
for small fixed temperature differences (Barletta Reference Barletta2022). For a bulk temperature of 25 ![]() $^\circ$C for air, (F3) gives
$^\circ$C for air, (F3) gives ![]() $L>3.06\times 10^4$ m for the OB approximation to be violated. The length scales of boundary layers in the present case are of the order of mm. Hence, the OB approximation is far from violated in the present study under this consideration too.
$L>3.06\times 10^4$ m for the OB approximation to be violated. The length scales of boundary layers in the present case are of the order of mm. Hence, the OB approximation is far from violated in the present study under this consideration too.
Appendix G. Validity of the present study to turbulent RBC
The present study finds the scaling of dust-free region heights in laminar natural convection boundary layers over horizontal surfaces, and proposes that the same scaling of dust-free region heights could be expected in turbulent RBC. However, there are strong fluctuations near the plate in turbulent RBC, of the order of the mean quantities themselves, which makes the validity of the present analysis to turbulent RBC somewhat unclear. We now clarify this apparent contradiction below.
In turbulent RBC, it is well known that a shear boundary layer is created by the large-scale flow, which we call as the global boundary layer since it spans the length of the convection cell. This global boundary layer has been the concern of most of the scaling theories of RBC so far, with many assumptions about their nature, ranging from laminar zero pressure gradient shear boundary layer (Grossman & Lohse Reference Grossman and Lohse2000) to turbulent shear boundary layer (Siggia Reference Siggia1994). However, the dominant coherent structures on the hot and cold plate in RBC are line plumes. These plumes are the long-time outcomes of instability of boundary layers at their bases. It then follows that, in addition to the global boundary layer, there have to be boundary layers in between these plumes, which we refer to as local boundary layers. Figure 11 shows the global and the local boundary layers.

Figure 11. (a) Schematic of the large-scale flow, the global boundary layer and the plumes on the plates along with the local boundary layers in between them. (b) Side view of the vertical section in A–A in a plane perpendicular to the plane in panel (a), showing the local plumes driving the large scale flow (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011). (c) Zoomed view of the local boundary layers and plumes in the region shown with the dashed ellipse in panel (b). ![]() $B$ denotes local boundary layer and
$B$ denotes local boundary layer and ![]() $P$ the plume. The lines show the edges of the local velocity boundary layer and the velocity boundary layer of the plume, while the shaded regions show the local thermal boundary layer and the thermal boundary layer of the plume. (d) Zoomed view of the dashed ellipse in panel (c) showing the details of one plume and two local boundary layers at its base (Theerthan & Arakeri Reference Theerthan and Arakeri1998).
$P$ the plume. The lines show the edges of the local velocity boundary layer and the velocity boundary layer of the plume, while the shaded regions show the local thermal boundary layer and the thermal boundary layer of the plume. (d) Zoomed view of the dashed ellipse in panel (c) showing the details of one plume and two local boundary layers at its base (Theerthan & Arakeri Reference Theerthan and Arakeri1998).
As shown by Shevkar et al. (Reference Shevkar, Mohanan and Puthenveettil2023), the ratio of the thickness of the local to the global boundary layer ![]() $\delta _l/\delta _g\sim Ra^{-1/9}$; then for the range of the present study,
$\delta _l/\delta _g\sim Ra^{-1/9}$; then for the range of the present study, ![]() $1\times 10^6\le Ra\le 1\times 10^{15}$,
$1\times 10^6\le Ra\le 1\times 10^{15}$, ![]() $0.2\le \delta _l/\delta _g\le 0.02$. The length of the local boundary layers are decided by their instability, which decide when they turn upwards to form plumes. As given by Puthenveettil et al. (Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011), the length of the local boundary layers,
$0.2\le \delta _l/\delta _g\le 0.02$. The length of the local boundary layers are decided by their instability, which decide when they turn upwards to form plumes. As given by Puthenveettil et al. (Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011), the length of the local boundary layers,
where ![]() $Z_w$ (2.3) is the near-plate length scale in turbulent convection,
$Z_w$ (2.3) is the near-plate length scale in turbulent convection, ![]() $C_1=47.5$ and
$C_1=47.5$ and ![]() $n_1=0.1$. As shown in figure 11, the length of the global boundary layer
$n_1=0.1$. As shown in figure 11, the length of the global boundary layer ![]() $L_g\sim L$. Then, for aspect ratio
$L_g\sim L$. Then, for aspect ratio ![]() $\varGamma =1$,
$\varGamma =1$,
for ![]() $1\times 10^6\le Ra\le 1\times 10^{15}$,
$1\times 10^6\le Ra\le 1\times 10^{15}$, ![]() $0.58\le \lambda /L_g\le 5.8\times 10^{-4}$. The local boundary layers are then an order smaller in thickness (
$0.58\le \lambda /L_g\le 5.8\times 10^{-4}$. The local boundary layers are then an order smaller in thickness (![]() $\delta _l\ll \delta _g$) and length (
$\delta _l\ll \delta _g$) and length (![]() $\lambda \ll L_g$) than the global boundary layers and are hence embedded within the global boundary layers.
$\lambda \ll L_g$) than the global boundary layers and are hence embedded within the global boundary layers.
The global boundary layers are most likely turbulent, even at moderate ![]() $Ra$, since the bulk flows that cause them are turbulent in turbulent RBC. In contrast, the thin local boundary layers, which are embedded within these global boundary layers, are most likely laminar. This is so since plumes are outcomes of the spatial instability of these local boundary layers at their bases, then, the local boundary layers themselves, since they exist before their spatial instability has developed, have to be laminar. This hypothesis – of two local laminar natural convection boundary layers, giving rise to plumes, being the dominant coherent structure on the plates in turbulent RBC – has since been proved in the following multiple studies. The predictions based on the above hypothesis of (i) the mean plume spacings (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil Reference Puthenveettil2004; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005), (ii) the total length of plumes (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011), (iii) the mean merging velocity of plumes (Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014), (iv) the mean time for the appearance of plumes at a vacant spot (Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014), (v) the mean plume spacing in the presence of shear (Shevkar et al. Reference Shevkar, Gunasegarane, Mohanan and Puthenveettil2019) and (vi) the scaling of the peaks of the horizontal velocity distributions (Shevkar et al. Reference Shevkar, Mohanan and Puthenveettil2023), all done at large
$Ra$, since the bulk flows that cause them are turbulent in turbulent RBC. In contrast, the thin local boundary layers, which are embedded within these global boundary layers, are most likely laminar. This is so since plumes are outcomes of the spatial instability of these local boundary layers at their bases, then, the local boundary layers themselves, since they exist before their spatial instability has developed, have to be laminar. This hypothesis – of two local laminar natural convection boundary layers, giving rise to plumes, being the dominant coherent structure on the plates in turbulent RBC – has since been proved in the following multiple studies. The predictions based on the above hypothesis of (i) the mean plume spacings (Theerthan & Arakeri Reference Theerthan and Arakeri1998; Puthenveettil Reference Puthenveettil2004; Puthenveettil & Arakeri Reference Puthenveettil and Arakeri2005), (ii) the total length of plumes (Puthenveettil et al. Reference Puthenveettil, Gunasegarane, Agrawal, Schmeling, Bosbach and Arakeri2011), (iii) the mean merging velocity of plumes (Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014), (iv) the mean time for the appearance of plumes at a vacant spot (Gunasegarane & Puthenveettil Reference Gunasegarane and Puthenveettil2014), (v) the mean plume spacing in the presence of shear (Shevkar et al. Reference Shevkar, Gunasegarane, Mohanan and Puthenveettil2019) and (vi) the scaling of the peaks of the horizontal velocity distributions (Shevkar et al. Reference Shevkar, Mohanan and Puthenveettil2023), all done at large ![]() $Ra$ too, have been shown to match with measurements from experimental and computational studies of turbulent RBC.
$Ra$ too, have been shown to match with measurements from experimental and computational studies of turbulent RBC.
It is also known that there is no contradiction in the presence of fluctuations near the plate and within the global boundary layers, with the above assumption of laminar nature of local boundary layers. This is so since these fluctuations close to the plate are partly caused by the motion of these laminar local boundary layers and the plumes themselves, so that a local measurement at a fixed point would measure properties from local boundary layers, plumes and the turbulent bulk at different times, each of these having different values, the time series then appearing as a fluctuating signal. This phenomenology has substantial proof since the vertical profiles of vertical velocity fluctuations, temperature fluctuations and mean temperature in turbulent RBC have all been predicted well by Theerthan & Arakeri (Reference Theerthan and Arakeri1998) using a model that assumes that the flow near the plate is made up of a uniform array of pairs of local laminar boundary layers, each pair giving rise to a laminar line plume, so that the plumes are equally spaced, equal to the mean plume spacing observed in experiments.
The majority of the temperature drop near the plate occurs across these local boundary layers, and hence, the formation of the dust-free region, driven by the temperature difference induced thermophoresis, will occur within the local boundary layers, which, as we saw earlier, is anyway within the global boundary layer. The local boundary layer and not the global boundary layer is hence the relevant flow and temperature field that needs to be studied to analyse the formation of a dust-free region near horizontal hot surfaces in turbulent RBC. The flow field used in the present study could then be considered to be that within the local boundary layers in turbulent RBC, which could be very well assumed to be laminar natural convection boundary layers due to the reasons mentioned above. In the present study, as seen in figure 6, the dimensionless height of the dust-free regions (![]() $\eta _{df}$), except for large values of
$\eta _{df}$), except for large values of ![]() $Th_m$ (
$Th_m$ (![]() $Th_m>10^4$) and low values of Prandtl number (
$Th_m>10^4$) and low values of Prandtl number (![]() $Pr<0.3$), is around
$Pr<0.3$), is around ![]() $10$ for
$10$ for ![]() $Pr=0.3$ and around
$Pr=0.3$ and around ![]() $5$ for
$5$ for ![]() $Pr=10$ at
$Pr=10$ at ![]() $Th_m=10^4$; the heights being less at lower
$Th_m=10^4$; the heights being less at lower ![]() $Th_m$. The corresponding dimensionless heights of natural convection boundary layers for
$Th_m$. The corresponding dimensionless heights of natural convection boundary layers for ![]() $0.1 < Pr< 10$, as given by Rotem & Claassen (Reference Rotem and Claassen1969) are
$0.1 < Pr< 10$, as given by Rotem & Claassen (Reference Rotem and Claassen1969) are ![]() $15<\eta < 6$. As expected, then
$15<\eta < 6$. As expected, then ![]() $\eta _{df}/\eta <1$ for
$\eta _{df}/\eta <1$ for ![]() $Th_m<10^4$ and
$Th_m<10^4$ and ![]() $Pr>0.3$, the dust-free region heights are much smaller than the corresponding local boundary layer thicknesses. The above assumption of laminar natural convection nature of the local boundary layers turbulent RBC is likely to be violated at large
$Pr>0.3$, the dust-free region heights are much smaller than the corresponding local boundary layer thicknesses. The above assumption of laminar natural convection nature of the local boundary layers turbulent RBC is likely to be violated at large ![]() $Ra$ where the shear due to the global boundary layer changes the nature of these local boundary layers to that of a mixed convection boundary layer; the limit for such a change is given in Appendix E.
$Ra$ where the shear due to the global boundary layer changes the nature of these local boundary layers to that of a mixed convection boundary layer; the limit for such a change is given in Appendix E.