I. INTRODUCTION
Microalloying technology, i.e., usually alloying with <2 at.% alloying elements, Reference Lu and Liu1 has traditionally been used as a metallurgical technique for developing new metallic crystalline materials with improved performance. For example, it can refine the microstructure of steels (adding V, Nb or Ti) and aluminum alloys (adding Zr). It has been recently shown, that minor addition of Sc (0.3 wt%) to bulk ultrafine grained/nanocrystal Al–Cu alloy increases the ductility and strength of the alloy to about 275 and 50%, respectively, compared to that of the alloy without Sc. Reference Jiang, Li, Cheng, Liu, Wang, Chen, Zhang, Sun, Yang and Yang2 The prolongation of the ductility was attributed to the promotion of intragranular precipitation of θ′-Al2Cu nanosized particles. This precipitation has two effects, first, the particles encourage dislocation trapping in the grain interior, and second, they help in accumulating dislocations and sustain work hardening. The work hardening behavior can be also detected in shape memory materials such as those corresponding to the NiTi Reference Carvalho and Harris3 system or in the ZrCu alloy. Reference Koval, Firstov and Kotko4 However, in shape memory alloys, work-hardening occurs due to the mechanically driven transformation of austenite into a harder phase, martensite.
A relatively new class of material is metallic glass, which is formed when a metallic liquid is cooled fast enough to lead to the formation of a vitreous solid. This material lacks a crystalline lattice and does not exhibit a long-range atomic order but exhibits short to medium range order clusters. Reference Miracle5–Reference Hirata, Guan, Fujita, Hirotsu, Inoue, Yavari, Sakurai and Chen7 Since the first metallic glass Au81Si19 was obtained by splat quenching in 1960, Reference Klement, Willens and Duwez8 new compositions have been discovered over the years. The basic idea for metallic glass formation is to suppress the homogeneous nucleation of crystals Reference Turnbull9 and to control their growth to avoid crystallization. The GFA was reported to be directly linked to the crystal growth rate in the supercooled liquid region. Reference Laughin and Hono10 Generally, the crystal growth rate is inversely proportional to the viscosity of the supercooled liquid. As bulk metallic glass (BMG) forming liquids are densely packed, they show high viscosity and sluggish crystallization kinetics when compared with other metallic liquids and they exhibit an intermediate behavior between the strongest and the most fragile liquids. To predict the GFA, many parameters have been used over the years such as the supercooled liquid region ΔT x = T x − T g, the reduced glass transition temperature T rg = T g/T l and the γ parameter [T x/(T g + T l)], where T x is the crystallization temperature, T g is the glass transition temperature and T l is the liquidus temperature. Reference Lu and Liu11–Reference Jiao, Zhao, Ding, Bai and Wang13 Additionally, for Ti-based BMGs, the electronic structure (electrons per atom: e/a) is an important parameter to predict the GFA. Reference Oak, Louzguine-Luzgin and Inoue14
Metallic glasses exhibit interesting properties, such as high yield strength and low elastic modulus. However, they are generally brittle because they exhibit shear softening, a phenomenon that originates from shear-induced dilatation Reference Spaepen15–Reference Greer, Cheng and Ma18 that causes highly localized shear bands and catastrophic failures. Metallic glasses can be ductilized by promoting the multiplication and arrest of shear bands, which can be attained by promoting the formation of crystalline phases by destabilizing the liquid phase through microalloying. The addition of minor alloying elements can affect the stability of the crystalline phase during quenching and thus determine the evolution of the microstructure. For example, in shape memory metallic glass composites it influences the eutectoid decomposition of the high temperature metastable crystalline B2 CuZr phase, which affects the formation and competition among B2 CuZr, the low-temperature equilibrium crystalline phase and the amorphous phase during quenching as reported by Song et al. Reference Song, Pauly, Zhang, Gargarella, Li, Barekar, Kühn, Stoica and Eckert19 The shifting in the B2 CuZr phase transformation is significantly influenced by minor element addition because it can significantly change the electronic structure and bonding energies of the B2 phase. The impact of the electronic structure on the stability of the B2 phase can be assessed from the number of valence electrons per atom (e/a). These authors proposed a new strategy for the formation of CuZr-based shape memory BMG composites and suggested a new parameter K = T f/T L, where T f and T L are the final temperature of B2 CuZr phase formation and T L the liquidus temperature, to predict and select a compositional region for different sized BMGs and composites (BMGC).
The question that arises is that whether the techniques used to tune the mechanical properties of crystalline materials can be applied in metallic glasses. Pauly et al. Reference Pauly, Das, Bednarcik, Mattern, Kim, Kim and Eckert20 showed that crystalline particles present in Cu–Zr–(Al–Ti) BMG composites can exhibit martensitic transformation that lead to work hardening and enhanced plastic deformation. The martensitic phase transformation from B2 austenite to B19′ martensite resulted in plastic strains of up to 15%. Similar results are traditionally obtained with fully crystalline shape memory materials, thus confirming the validity of the techniques for BMGs. In fact, Wu et al. Reference Wu, Zhou, Song, Wang, Zhang, Ma, Wang and Lu21 found a few years later that promoting twinning of the reinforcing crystals present in BMGs through microalloying can improve the ductility of the BMGC similarly to that detected in microalloyed nanocrystalline materials. Reference Ma, Wang, Lu, Sui, Lu and Lu22,Reference Lu, Lu and Suresh23
Microalloying technology can be also used to tailor the structure of the amorphous phase and thus control the mechanical performance of the material. Microalloying can have different effects on the melt, depending on the composition of the BMG and the nature of the elements added (rare earth element ≡ RE, transition metal ≡ TM, etc). For example, they can change the density of states, Reference Gendelman, Joy, Mishra, Procaccia and Samwer24 stabilize the liquid phase by approaching the composition closer to a deep eutectic, Reference Xu, Duan and Johnson25 impair the thermodynamic driving force for crystal precipitation by introducing an atomic-level strain energy into the liquid, Reference Zhang, Chen, Chen and Liu26 favor the formation of local crystal-like orders at the atomic scale Reference Wang, Liu, Yang, Liu, Dong and Lu27 or scavenge oxygen from the melt. Reference Miracle5 Over the years, the vast majority of the studies have been focused on studying the effect of adding one minor alloying element to tune the GFA and the mechanical properties of BMGs and BMGCs. Only few studies have dealt with the effect that minor co-addition of various elements Reference Li, Pang, Ma and Zhang28–Reference Fan, Fu, Qiao, Choo, Liaw, Browning and Löffler30 has on the properties of these materials, despite the fact that the synergistic effect of minor element co-addition can result in compositions with very different properties as shown in this paper.
Another issue of interest is the dramatic change in the structure and thus on mechanical performance that microalloying could have in the properties of these materials. This is especially important for BMGs whose structure is very sensitive to compositional changes and for samples with dimensions close to the critical amorphous diameter (D c) since the amorphous phase can turn crystalline with a small composition change. Although this offers the opportunity to tailor the properties of alloys with high efficiency (i.e., small alloying addition has a large effect on the material structure), it implies a technological challenge for controlling the composition.
In this paper, we summarize the effect of microalloying in the glass forming ability and mechanical properties of alloys that are very sensitive to minor additions with focus on our results. This sensitivity is either because their glass forming ability is relatively low and thus a small composition change can lead to crystallization [i.e., for Mg–Ni–RE, Reference González, Figueroa, Zhao, Davies, Todd and Adeva31 Mg–Zn–Ca, Reference Zberg, Uggowitzer and Löffler32 and Al–Ni–Co–Y Reference Louzguine-Luzgin and Inoue33 systems] or because the alloy system itself is very sensitive to minor additions (i.e., for Zr–Cu alloys). Reference Xu, Duan and Johnson25,Reference Yu, Bai and Wang34
II. INFLUENCE ON PROPERTIES
A. Tuning the glass forming ability
1. Addition of RE elements
Due to the affinity of RE elements for oxygen, they can scavenge oxygen impurity and promote the formation of innocuous oxides which results in an increase of the GFA. Reference Koval, Firstov and Kotko4 However, a drawback for using RE elements is that microalloying element is relatively expensive. A cheaper and sometimes more effective option, to increase the GFA, is to use mischmetal (MM), i.e., a mixture of RE elements in naturally occurring proportions. There are several types of MMs such as LaMM (lanthanum mischmetal) and CeMM (cerium mischmetal), which are named according to the most abundant element present in the mixture.
Table I lists the critical amorphous diameter (D c) of various alloys corresponding to the Mg–Ni–La system and the same alloys when La is fully substituted by LaMM. Reference González, Figueroa, Zhao, Davies, Todd and Adeva31 Among these compositions, the Mg65Ni20LaMM15 is the one which exhibits the highest D c. Also, it is the composition for which the critical amorphous diameter increases the most, from 0.5 to 2 mm, after the substitution of La by LaMM. The GFA parameters ΔT x, T rg, and γ were calculated from the measured parameters (glass transition temperature: T g, crystallization onset temperature: T x, solidus temperature: T m and liquidus temperature: T l), however, no clear correlation between D c and either ΔT x or T rg was observed. Moreover, the magnitude of the γ parameter is relatively insensitive to compositional changes. These results suggest that the parameters ΔT x, T rg, and γ are not reliable indicators of the GFA for this set of alloys. Reference González, Figueroa, Zhao, Davies, Todd and Adeva31
TABLE I. Critical amorphous diameter (Dc) for the Mg–Ni–La and Mg–Ni–LaMM systems. Reference Pauly, Das, Bednarcik, Mattern, Kim, Kim and Eckert20

To understand the reason for the high GFA of the Mg65Ni20LaMM15 alloy, the ratio of Mg:Ni:REs was held constant at 65:20:15, as the original alloy. Table II shows the value of D c for alloys with the same proportion of RE elements as in the LaMM. Reference González, Figueroa, Zhao, Davies, Todd and Adeva31 The value of D c increases with increasing content of Nd, which is consistent with the results of Lu et al. Reference Lu, Bei and Liu35 These results thus confirm the important role of the Nd content in the glass forming ability. However, small addition of Ce also appears to be beneficial in increasing the D c. This behavior cannot be explained from differences in heat of mixing the RE elements with Mg since they are very similar: Mg–La (−7 KJ/mol), Mg–Ce (−7 KJ/mol), Mg–Pr (−6 KJ/mol), and Mg–Nd (−6 KJ/mol). Reference Takeuchi and Inoue36 It can neither be explained from the point of view of differences in atomic radii since they are practically the same for La (0.195 nm), Ce (0.185 nm), Pr (0.185 nm) and Nd (0.182 nm). Reference Slater37 Li et al. Reference Li, Pang, Ma and Zhang28 studied the influence of RE elements on the GFA of Mg65Ni20La9.29Nd5.71 alloy and observed that when La and Nd atoms were partially replaced by Ce atoms, the GFA increased due to a decrease in the Gibbs free energy of mixing. This phenomenon could also explain the beneficial effect of the Ce addition in enhancing the D c for Mg65Ni20La8.91Nd5.45Ce0.59 alloy. Reference Zberg, Uggowitzer and Löffler32 These results suggest that when microalloying elements coexist in a proper ratio in the MM, the mixture can be useful to substitute expensive RE elements, such as La, 38 to increase the GFA of metallic glasses at a relatively low cost. Similar effectiveness in changing the GFA of alloys corresponding to the Mg–Ni–La system is observed when minor Si–La, Y–Si–La and B–La elements are co-added. Reference González, Figueroa and Todd39 Minor element co-addition has thus great potential for tuning the GFA of metallic glasses.
TABLE II. Critical amorphous diameter of alloys containing 15 at% RE with the same proportion of RE elements as in the LaMM. Reference Pauly, Das, Bednarcik, Mattern, Kim, Kim and Eckert20

The main drawback for using the MM is the lack of an exact formulation, i.e., the composition depends on the location from where the ore is extracted. Differences in composition of the MM could have different effects on the GFA of the alloy and lead to the formation of crystalline phases of different natures, which in turn results in alloys with very different mechanical performances. Reference Pérez, González, Garcés and Adeva40 This limitation makes it impractical to implement the use of the MM by industries. Moreover, the MM contains naturally present impurities coming from the ore that could have a negative effect on the GFA. An efficient technique to better control the final properties of BMGs at a relatively lower cost than using La is the addition of Yttrium (Y). This explains the wide use of this element in controlling the properties of BMGs. The main interest for using Y to increase the GFA generally stems from its effectiveness in scavenging oxygen. However, Wang et al. Reference Wang, Liu, Yang, Liu, Dong and Lu27 reported recently that the mechanism for increasing the GFA of the Cu–Zr–Al alloy system when 2 at.% Y is added can be attributed to the formation of the local crystal-like orders at the atomic scale. In principle, one could expect that an increase in the crystal-like order should accelerate the crystallization rate of the glass-forming liquid; however, the GFA of the alloy increases. This paradox was explained based on the previous investigation of Cheng et al. Reference Cheng, Ma and Sheng41 where the authors identified the co-existence in the Cu–Zr based BMG of a competition process of the crystal growth and formation of icosahedra-like orders. These icosahedra-like clusters can pin the boundaries of crystal-like clusters and limit their growth, Reference Zhang, Chen, Chen and Liu26 thus resulting in an overall structural ordering that reduces the thermodynamic driving force for crystallization. This process slows down the crystallization rate and thus enhances the GFA.
2. Addition of transition elements
Microalloying with most transition metals can improve the GFA of Al-based BMGs. For example, partial substitution of Al by 0.5 at.% TM (with the exception of the late transition elements Ni and Cu) in the Al88Y7Fe5 alloy increases the GFA. Reference Bondi, Gangopadhyay, Marine, Kim, Mukhopadhyay, Goldman, Buhro and Kelton42 Among these elements, the early TM elements (Ti, V, and Cr) exhibit larger ΔT x, from 34 to 44 °C, than the mid TM elements (Co and Fe), ΔT x from 22 to 27 °C, which is consistent with the larger GFA of the former elements. From synchroton diffraction and EXAFS, it was concluded that the GFA increase with minor Ti addition was due to the formation of short- and medium-range orders that decrease the atomic mobility in the glass, enhancing the glass stability (i.e., increasing the nucleation barrier) and thus making the nucleation of α-Al more difficult.
In other alloy systems the superior GFA is attributed to a covalent atomic interaction. For example, it was observed in Ce–Al–Cu BMGs that addition of 0.2–3.0 at.% of Fe, Co Ni, Nb, Si, C or B increases the maximum amorphous diameter from 2 to 10 mm (Ref. Reference Zhang, Zhao, Pan, Wang and Wang43) due to the strong covalent interaction between Fe, Co, Ni, Nb, and the Al matrix. Chathoth et al. Reference Chathoth, Damaschke, Embs and Samwer44 detected, for the same Ce–Al–Cu alloy system, strong variations in the relaxational dynamics while microalloying with 1 at.% Nb and the enhancement of GFA was correlated with a decrease of the self-diffusivity close to the melting temperature by about 74% and also to a temperature dependence change from Arrhenius to non-Arrhenius behavior. Reference Chathoth, Damaschke, Embs and Samwer44 However, TMs can also have a negative effect on the GFA of some systems. Partial substitution of Ni by 3–5 at.% Pd decreases the GFA of the Zr55Al10Cu30Ni(5−x)Pd x alloy. Reference Qin, Zhang, Wang, Ding and Hu45 Addition of Pd promotes the formation of Zr–Pd and Zr–Al pairs that can form Zr–Pd and Zr–Al clusters acting as homogeneous nucleation sites for the Zr2(Pd,Ni)-type phase and the Zr3Al2-type phase, respectively. This is consistent with the decrease of the supercooled liquid region ΔT x from 82 K for Zr55Al10Cu30Ni5 to 77 K for Zr55Al10Cu30Pd5 alloy. A decrease in the GFA was also observed in the Zr–Cu–Al system with the addition of TM elements, such as Co and Fe, as can be deduced from the diffraction patterns of Fig. 1. For Zr48Cu48Al4 alloy, the GFA decreases when Cu is partly substituted by up to 1 at.% Co. Reference González, Pérez, Rossinyol, Suriñach, Baró, Pellicer and Sort46 This effect is more remarkable with the addition of Fe since only 0.5 at.% is enough to dramatically decrease the volume fraction of the amorphous phase. The predicted heats of mixing are −41 kJ/mol for Co–Zr, +6 kJ/mol for Co–Cu, −19 kJ/mol for Co–Al, −25 kJ/mol for Fe–Zr, +13 kJ/mol for Fe–Cu and −11 kJ/mol for Fe–Al pair. Reference Lu, Bei and Liu35 Consequently, the atomic bondings with Co are stronger than with Fe, which can explain the lower GFA with the addition of Fe. Partial substitution of Cu by Co also decreases the GFA of the Zr–Cu system as observed by Javid et al. Reference Javid, Mattern, Pauly and Eckert47,Reference Javid, Mattern, Samadi Khoshkhoo, Stoica, Pauly and Eckert48 These authors observed that Co shifts the temperatures of the eutectoid reaction, CuZr ↔ Cu10Zr7 + CuZr2, to lower values and thus, increases the thermal stability of the B2 ZrCu phase.

FIG. 1. A) Diffraction patterns corresponding to (a) Zr48Cu48Al4, (b) Zr48Cu47.5Al4Co0.5, and (c) Zr48Cu47Al4Co1 as-cast rods. B) Diffraction patterns corresponding to (a) Zr48Cu48Al4, (b) Zr48Cu47.5Al4Fe0.5, and (c) Zr48Cu47Al4Fe1 as-cast rods.
Depending on the transition element added and composition of the alloy, the effect on the GFA may be different. For Zr66.7−x Ni33.3Pd x alloys, partial substitution of Zr by 1 and 3 at.% Pd (a late TM) enhances the GFA and the activation energy for crystallization Reference Wang, Wang and Li49 due to the increase of topological short-range order. Similar GFA increase is observed in Ni57Zr20Ti23−x Pd x alloys when Ti is partly substituted by 3, 5, 7, and 10 at.% Pd. Reference Lee, Kim and Kim50 The addition of Pd to Ni57Zr20Ti23 alloy suppresses the formation of the primary cubic Ni(Ti,Zr) phase and increases the GFA. According to Inoue Reference Inoue51 the supercooled liquid state is stabilized when multicomponent systems have large negative heat of mixing. The large negative heat of mixing of Pd–Zr (−91 kJ/mol) and Pd–Ti (−65 kJ/mol) Reference Takeuchi and Inoue36 may explain the suppression of Ni(Ti,Zr) phase formation.
Metallic glasses corresponding to the Al–Y–Ni–Co–Pd system, exhibit a decrease in the GFA with the addition of Pd and leads to the formation of different crystalline phases depending on the concentration of the alloying element. For Al85Y8−x Ni5Co2Pd x ribbon samples, a broad diffraction peak was observed for Al85Y8Ni5Co2 alloy and the peak tends to form two shoulders with increasing addition of Pd up to 4 at.%, Reference Louzguine-Luzgin and Inoue33 suggesting a decrease in the GFA. Similarly, for 1 mm diameter rods of Al86Ni6Y6−x Co2Pd x , partial substitution of Y by 0.5 and 1 at.% Pd results in a dramatic decrease of the GFA, Reference González, Sort, Louzguine-Luzgin, Perepezko, Baró and Inoue52 as can be deduced from the diffraction patterns of Fig. 2. For Al86Ni6Y6Co2 alloy, peaks corresponding to α-Al and Al3Ni are superimposed on a broad halo, suggesting the presence of an amorphous or nanocrystalline matrix. For Al86Ni6Y5.5Co2Pd0.5 and Al86Ni6Y5Co2Pd1 alloys, additional peaks corresponding to Al9Co2 and Al3Y are detected while the broad halo is practically nonexistent. This reduction of the GFA could be explained considering the high binding potential of the Pd–Y pair (i.e., −84 kJ/mol) compared to the relatively low binding potential of the Co–Y (−1 kJ/mol) and Ni–Y (0 kJ/mol) pairs, Reference Takeuchi and Inoue36 which would lead to a dramatic driving force for nucleation from Pd–Y pair sites. However, the promotion of α-Al phase formation with Pd addition cannot be explained from the heats of mixing due to the strength of the attractive interaction of the Al–Pd atomic pair. The small atomic size difference between Al and Pd does not favor the atomic packing, which may explain why the GFA decreases. Reference Louzguine-Luzgin and Inoue33

FIG. 2. Diffraction patterns of 1 mm diameter Al86Ni6Y6-xCo2Pdx (x = 0, 0.5 and 1) rods.
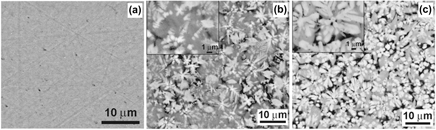
The addition of Pd to Mg72Zn23Ca5 alloy also decreases the GFA, Reference González, Pellicer, Fornell, Blanquer, Barrios, Ibañez, Solsona, Suriñach, Baró, Nogués and Sort53 as can be deduced from the diffraction patterns in Fig. 3. For the alloy without Pd, a broad halo centered at around 39° and a broad peak at about 37° are detected . The results suggest that the alloy consists of an amorphous matrix and nanocrystals embedded in it. However, for Mg70Zn23Ca5Pd2 and Mg66Zn23Ca5Pd6 alloys, narrow reflections are present, suggesting the development of a fully crystalline structure. These diffraction patterns are assigned to CaZn5 MgZn, Mg6Pd, and Mg6Ca2Zn3 phases. From the relative change in intensity of the diffraction peaks with increasing content of Pd, it can be deduced that the volume fraction of the different phases change with the alloy composition. The backscattered SEM image in Fig. 4 shows the microstructure of the 3 samples of different compositions. For Mg72Zn23Ca5 alloy, a rather featureless contrast is observed, which is consistent with the diffraction result. However, the microstructures for Mg70Zn23Ca5Pd2 and Mg66Zn23Ca5Pd6 alloys are completely different. The microstructures are crystalline and consist of a large volume fraction of dendrites. The dendrites correspond to Mg6Pd, the gray regions to CaZn5, the black regions to MgZn and the Mg6Zn3Ca2 phase corresponds to the areas of darkest tonality that lie within the dark regions associated MgZn. The mechanism responsible for the GFA decrease with Pd addition in this system should be different from that of the Al86Ni6Y6Co2 alloy since the binding potential of the alloying element with the other elements of the alloy are relatively similar [i.e., Pd–Mg (−40 kJ/mol), Pd–Zn (−33 kJ/mol) and Pd–Ca (−63 kJ/mol)]. Reference Takeuchi and Inoue36 The formation of Mg6Pd probably comes from partial crystallization of the Pd–Mg atomic clusters upon cooling since Mg6Pd is the first crystalline phase detected in the Mg–Pd system. Reference Yamaura, Kimura and Inoue54 The heat of mixing of Mg6Pd (−21 kJ/mol) is larger than that of MgZn (−4 kJ/mol), Reference Boer, Boom, Matterns, Miedema and Niessen55 thus the formation of Mg6Pd is more thermodynamically favored than the formation of MgZn. However, due to the high concentration of Zn atoms in the alloy, they have high probability to be surrounded by Mg atoms, which can lead to the formation of the intermetallic MgZn. As Pd enters the alloy in the substitution of Mg, the quantity of Mg6Pd phase that is formed increases, which brings about a decrease in the amount of Mg available to constitute the Mg6Ca2Zn3 phase.

FIG. 3. Diffraction patterns of the as-cast Mg72-xZn23Ca5Pdx (x = 0, 2 and 6) rods.

FIG. 4. SEM images (backscattered electrons) of (a) Mg72Zn23Ca5, (b) Mg70Zn23Ca5Pd2, and (c) Mg66Zn23Ca5Pd6 rod. The insets show the magnified details of the microstructure of the Mg70Zn23Ca5Pd2 and Mg66Zn23Ca5Pd6 samples (in panels (b) and (c)).
B. Effect on the mechanical properties
1. BMGs
The minor addition of elements is very helpful to tune the mechanical properties of BMGs, such as the strength, ductility, toughness, etc. These properties can change, despite a small compositional change might not be enough to change the electronic structure or chemistry of the glass-forming liquid. Reference Garret, Demetriou, Chen and Johnson56 Garret et al. Reference Garret, Demetriou, Chen and Johnson56 studied the effect that small additions of Si and Sn has on the toughness of Cu47Ti34Zr11Ni8 BMG. For the three compositions studied, Cu47Ti34Zr11Ni8, Cu47Ti33Zr11Ni8Si1, and Cu47Ti33Zr11Ni8Si1Sn2, they observed a decrease in toughness with increasing content in the alloying element. This behavior was attributed to an increase in the shear modulus (G), yield strength (σy), and glass transition temperature (T g) and a decrease in Poisson's ratio (υ). The minor shifts of the elastic constants (G and υ) with small composition change suggest atomic structural arrangements that have an effect on the fracture behavior. For the most highly alloyed composition, i.e., Cu47Ti33Zr11Ni8Si1Sn2, cominor alloying with Si and Sn does not appear to have a synergistic effect on the fracture toughness (although the issue of co-addition was not dealt in the manuscript) since the effect on the of G, σy, T g, and υ values could be expected considering that the concentration of minor elements in Cu47Ti33Zr11Ni8Si1Sn2 is 3 times higher than those in Cu47Ti33Zr11Ni8Si1 alloy.
The toughness of the Zr–Cu–Al BMG system was studied by He et al. Reference He, Cheng, Ma and Xu57 for different concentrations of Zr and Al. The atomic structure of the Zr–Cu–Al BMG system consists of rigid icosahedral clusters in different volume fractions depending on the Zr and Al content of the alloy. According to these authors, higher toughness is expected for compositions with high Zr-content since the internal structure contains a lower fraction of the rigid icosahedral clusters. They also suggested to avoid the addition to the Zr–Cu–Al system of TM elements with the high-lying partially-filled d band and strong orbital hybridization with Al since it would be detrimental to toughness. Substitution of Cu with Ni/Co enhances the degree of covalency, and thus decreases the toughness, because these elements elevate the TM-d band which interacts more strongly with the sp valence electrons of Al. Reference He, Cheng, Ma and Xu57 Recent studies report that microalloying binary Zr–Cu alloys with Al is related to the changes in the cluster's composition, which results in considerable deviation from the random mixing behavior Reference Bokas, Evangelakis and Lekka58 due to the strongly attractive interaction between Al and Zr atoms. Reference Georgarakis, Yavari, Louzguine-Luzgin, Antowicz, Stoica, Li, Satta, LeMoulec, Vaugham and Inoue59 The late transition element Ni was also reported to decrease the toughness of Zr–Ti–Be–LTM BMGs. Reference Kim, Suh, West, Lind, Dale Conner and Johnson60 Similarly, minor addition of Fe (0.5 at.%) into Vitreloy 1 (Zr41.2Ti13.8Cu12.5Ni10Be22.5) results in the degradation in fracture toughness from 48.5 to 25 MPa m1/2. This phenomenon was associated with the variation in free volume distribution in the glass with minor Fe addition.
Minor alloying addition can also have a dramatic effect on the thermoplastic behavior of BMGs. For example, minor addition of RE elements has a significant effect on the performance of the Zr–Cu–Ni–Al system. The thermoplastic formability of (Zr65Cu17.5Ni10Al7.5)100−x RE x (x = 0.25–3.25 at.%, RE: Y, Gd, Tb, Dy, Ho, Er, Tm, Yb, and Lu) BMGs, was studied by analyzing the relative length shrinkage parameter (ΔL/L 0), Reference Hu, Fu and Zeng61 which is related with the malleability. The value of the parameter ΔL/L 0 tends to increase with increasing content in the alloying element but the above certain concentration drops dramatically. Among all the RE elements investigated, maximum ΔL/L 0 is attained with the addition of a critical concentration of 2 at.% Er and it drops with further addition of the alloying element. This critical concentration, however, varies with the type of element added. When Y is added, the maximum ΔL/L 0 is reached for a concentration of about 1.2 at.%. The variation in the thermoplastic formability was attributed to the different abilities of RE elements to scavenge oxygen, a phenomenon that has a direct effect on the supercooled liquid region interval.
For certain BMG systems with low GFA, the addition of RE elements can result in partial crystallization of the material and lead to a significant change in the mechanical performance. Partial substitution of Mg by 1 at.% Y in the Mg69Zn27Ca4 alloy is enough to partly crystallize a rod sample of 1 mm diameter and increase the strength from 550 to 1012 MPa. Reference Wang, Li, Huang, Wei, Xi, Cai and Pan62 This behavior was attributed to the formation of the crystalline phases Mg12YZn and Ca2Mg6Zn3. However, addition of Y to Cu45Zr48Al7 BMG improves the GFA and decreases the compressive fracture strength from 1892 MPa for Cu45Zr48Al7 to 1465 MPa for Cu46Zr48Al7Y5 due to the reduction in the binding energy. Reference Hong-wei, Yu-lei and Yu63
2. Metallic glass composites
The effect of transition elements on the mechanical performance of alloys depends not only on the type of element (early, middle and late TM) added but also on the composition of the alloy. For example, partial substitution of Zr by Nb does not have a significant change in the mechanical properties of Zr(46−x)Nb x Cu37.6Ag8.4Al8 alloys. Reference Nie, Xu, Jiang, Chen, Xu, Fang, Xie, Luo, Wu, Wang, Cao and Jiang64 Other transition elements such as Co and Fe seem to have a larger influence on the mechanical behavior of BMG composites corresponding to the Zr–Cu–Al system. For CuZr-based metallic glass composites, the second-phase particles consist of B2-CuZr phase that transforms into B19′ martensite upon loading. Reference Pauly, Gorantla, Wang, Kühn and Eckert65 This transformation, which occurs through twinning, Reference Pauly, Gorantla, Wang, Kühn and Eckert65,Reference Seo and Schryvers66 leads to overall work hardening and thus enhances the plasticity of the BMG. Larger values of plasticity can be attained when the twinning propensity of the particles upon loading is favored. Wu et al. Reference Wu, Zhou, Song, Wang, Zhang, Ma, Wang and Lu21 found that the capability of the B2-CuZr phase for deformation twinning could be tailored through microalloying with transition metals since they allow the tuning of the stacking fault energy of the primary slip system of B2-CuZr phase. The purpose of microalloying is to reduce the stacking fault energy of the primary slip system of reinforcing crystals to improve the twinning propensity thus favoring the martensitic transformation.
The stacking fault energy of base B2-CuZr phase (i.e., 381 mJ/m2) decreases when half of Cu on the (011) [100] slip plane is replaced by Cr, Fe, Co, Ni, and Ag. Among these elements, the maximum decrease of the stacking fault energy to 75 mJ/m2 is obtained with Co addition. This substitution promotes martensitic transformation of the crystalline phase and favors work-hardening during the test which contributes to delay necking of the BMG composite. Reference Wu, Zhou, Song, Wang, Zhang, Ma, Wang and Lu21
Another interesting element for microalloying is Fe since it not only is useful for decreasing the stacking fault energy of B2-CuZr but also is cost-effective. To further understand the influence of Co and Fe addition and their concentration on the mechanical properties of the Zr–Cu–Al system, the mechanical properties of Zr48Cu48−x Al4M x (M ≡ Co or Fe, x = 0, 0.5, 1 at.%) BMG composites were studied. Reference González, Pérez, Rossinyol, Suriñach, Baró, Pellicer and Sort46 Figure 5 shows the compressive stress–strain curves of the Zr48Cu48Al4, Zr48Cu47.5Al4Co0.5, Zr48Cu47Al4Co1, Zr48Cu47.5Al4Fe0.5 and Zr48Cu47Al4Fe1 as-cast rods. From these results, it can be deduced that microalloying not only has an influence on the plastic deformation but also on the yield stress. The maximum compressive plasticity of 6.2% is attained for 0.5 at.% Fe. However, the yield stress decreases gradually from 1390 MPa (for 0.5 at.% Fe) to 1355 MPa (for 1 at.% Fe) with increasing content of Fe. The change in the plastic deformation and yield stress is due to the propensity for the mechanically-driven martensitic transformation of the pristine austenite phase. Other factors such as the co-existence of the amorphous and crystalline counterparts, the nature of the crystalline phase in the as-cast condition (i.e., austenite or martensite), the volume fraction of crystalline (B2) phase and the tendency for deformation-induced nanocrystallization inside shear bands play an important role in the mechanical performance of these alloys.

FIG. 5. Compressive stress–strain curves of the Zr48Cu48Al4, Zr48Cu47.5Al4Co0.5, Zr48Cu47Al4Co1, Zr48Cu47.5Al4Fe0.5, and Zr48Cu47Al4Fe1 as-cast rods. The insets are optical micrographs showing the fracture angle for (a) Zr48Cu47.5Al4Co0.5 and (b) Zr48Cu47.5Al4Fe0.5 rods. The compression curves have been shifted horizontally for the sake of clarity.
To further confirm the role of the stress-induced martensitic transformation on the mechanical performance, all the as-cast samples of the same composition were compressed to 2100 MPa for 4 min, i.e., under similar conditions in the compression test. The occurrence of the deformation-induced martensitic transformation and intragranular nanotwinning was evidenced from TEM (Fig. 6). The SAED pattern [Fig. 6(b)] of various crystallites reveals the coexistence of austenite B2 and martensite B19′ phases. Several intragranular nanotwins are generated inside many of the crystals [Figs. 6(a), 6(c), and 6(d)]. These nanotwins can hinder dislocation motion through the twin boundaries resulting in a hardness increase by the dislocation pile-up mechanism similar to that observed at grain boundaries. Reference Sort, Zhilyaev, Zielinska, Nogués, Suriñach, Thibault and Baró67

FIG. 6. TEM images of the Zr48Cu47.5Al4Fe0.5 alloy compressed to 2100 MPa for 4 min. Panels (a), (c), and (d) show examples of intragranular nanotwins formed inside the crystalline particles during compression. Panel (b) is a SAED pattern of these crystals, revealing the coexistence of B2 (austenite) and B19′ (martensite) phases.
For aluminum alloys corresponding to the Al–Ni–Y–Co system, the addition of Pd was reported to be an effective transition element to control the microstructure and thus the mechanical performance of these alloys. Figure 7 shows the load–displacement (P–h) curves of Al86Ni6Y6Co2, Al86Ni6Y5.5Co2Pd0.5, and Al86Ni6Y5Co2Pd1 alloys with a maximum applied force of 300 mN. This load is high enough to get information from all the phases composing the microstructure and thus it provides a representative average value. The mechanical properties were evaluated measuring the hardness (H), reduced elastic modulus (E r), ratio U plastic/U total, and maximum nanoindentation depth (h max) as shown in the table associated to Fig. 7. Among the three compositions, the softest alloy is the Al86Ni6Y5.5Co2Pd0.5 because it exhibits the lowest H and largest h max. However, the maximum hardness is attained by the Al86Ni6Y6Co2 alloy (i.e., 5.5 GPa), probably because of its amorphous state, but decreases sharply to 2.5 GPa with the addition of 0.5 at.% Pd. For this composition the value of E r is also the lowest. Further addition of Pd to 1 at.% increases the hardness to 3 GPa. The composition with the largest U plastic/U total corresponds to Al86Ni6Y5.5Co2Pd0.5, corroborating its larger ability to store plastic energy during deformation.

FIG. 7. P–h curves of (a) Al86Ni6Y6Co2, (b) Al86Ni6Y5.5Co2Pd0.5, and (c) Al86Ni6Y5Co2Pd1. The corresponding table lists the hardness (H), reduced elastic modulus (Er), ratio Uplastic/Utotal and maximum nanoindentation depth (hmax).
Microalloying with Pd also has a large effect on the mechanical properties of alloys corresponding to the Mg–Zn–Ca–Pd system. The P–h nanoindentation curves with a maximum load of 500 mN are shown in Fig. 8(a). Some of the indentation impressions obtained at half the radius of the Mg70Zn23Ca5Pd2 rod are shown in Fig. 8(b). These indentation marks are large enough to embrace all the existing crystalline phases, thus the mechanical properties obtained are representative of the average behavior of the composite. The addition of 2 at.% Pd increases the hardness from 2.71 GPa (x = 0) to 3.56 GPa (x = 2). The hardness increase is mainly attributed to the lack of free volume in the crystalline alloy (x = 2) compared to the mainly amorphous one (for x = 0). The hardness further increases for x = 6, up to 3.90 GPa, which is consistent with the decrease of the maximum indentation depth from 2.905 μm (for x = 2) to 2.673 μm (for x = 6). This behavior could be mainly attributed to the presence of MgZn and Mg6Pd. Hardness is usually regarded to be an important property for evaluating wear resistance but some authors have shown that the wear resistance can be better estimated from the H/E r ratio. Reference Oberle68 Another parameter related to the wear performance is the resistance to plastic deformation, Reference Tsui, Pharr, Oliver, Bhatia, White, Anders, Anders and Brown69 which is proportional to H 3/E r 2.

FIG. 8. (a) (P–h) nanoindentation curves of Mg72-xZn23Ca5Pdx (x = 0, 2 and 6) alloys and (b) backscattered SEM image showing some indents made close to the center of the Mg70Zn23Ca5Pd2.
For Al-based alloys, the small addition of Pd Reference González, Sort, Louzguine-Luzgin, Perepezko, Baró and Inoue52 has a large influence on the microstructure and mechanical performance of Mg-based alloys. This behavior could be partly attributed to the low glass forming ability of alloys corresponding to these systems, which implies that small compositional change, such as microalloying would turn the microstructure from amorphous to crystalline. This work shows the usefulness to work with samples of dimensions close to the critical amorphous diameter so that microalloying could be more effective by turning the material from amorphous to partly or fully crystalline.
III. CONCLUSIONS
Previous discoveries have shown that microalloying technology is beneficial to tune the GFA and properties including mechanical, chemical, and physical properties of BMGs and BMG composites. From this review paper the following conclusions and tentative outlook have been presented:
-
(1) Studies about minor co-addition of alloying elements and their potential synergistic effects to produce BMGs with an excellent performance have been mainly overlooked in the past.
The chances of finding a composition with outstanding properties increase with the number of elements added but the analysis becomes more complex, which can be a limiting factor for the development on novel alloys through microalloying. To deal with such volume of information, the use of models can be very helpful. For example, a simplified thermodynamic model is proved to be useful to predict the change in GFA when two elements were co-added. Reference Li, Pang, Ma and Zhang28 However, new models will be required in the future to handle the complexity of multiple element co-addition.
-
(2) Traditionally, microalloying has been used to tune the properties of crystalline or amorphous materials. For BMG composites, it has been recently shown that microalloying not only can be used to tune the properties of the amorphous matrix (i.e., changing the atomic short-range order, promoting the formation of clusters, etc.) but also can be used to tune the mechanical properties of the dispersed crystalline phase (i.e., the twinning propensity). It would be of interest to explore the possibility of multiple element co-addition to tune the properties of the amorphous phase with various microelements and the crystalline phase with others.
-
(3) Fabrication of BMGs with sizes close to the critical amorphous diameter is of interest for their application in industrial products. It also makes microalloying more effective to control the mechanical properties of BMGs since a small element addition can easily turn an amorphous material into a crystalline material upon cooling thus changing dramatically the mechanical behavior. Although it is currently possible to control the formation of crystalline phases in the amorphous matrix through proper microalloying, an accurate control is challenging mainly due to the high compositional sensitivity. Moreover, the chemical heterogeneity and variation in concentration of impurities across the parent sample might be of the same order as differences in the concentration of minor element/s added. One of the obstacles to be surmounted is the relatively low resolution of fast and cost-effective techniques to check alloy compositions, such as microanalysis in scanning electron microscope. This issue should be tackled to make the most of the microalloying technology.