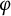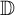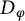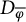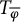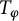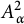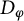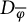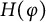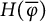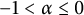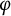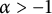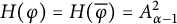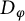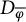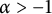1 Introduction
Let
![]() $\mathcal {H}$
be a Hilbert space, and let
$\mathcal {H}$
be a Hilbert space, and let
![]() $B(\mathcal {H})$
be the space of all bounded linear operators on
$B(\mathcal {H})$
be the space of all bounded linear operators on
![]() $\mathcal {H}$
. If
$\mathcal {H}$
. If
![]() $T\in B(\mathcal {H})$
is a contraction, we use
$T\in B(\mathcal {H})$
is a contraction, we use
![]() $H(T)$
to denote the range space of the defect operator
$H(T)$
to denote the range space of the defect operator
![]() $(I-TT^*)^{1/2}$
. It is well known that
$(I-TT^*)^{1/2}$
. It is well known that
![]() $H(T)$
is a Hilbert space with the inner product
$H(T)$
is a Hilbert space with the inner product
where
![]() $x, y \in \mathcal {H} \ominus \ker (I-TT^*)^{1/2}$
. Spaces of the type
$x, y \in \mathcal {H} \ominus \ker (I-TT^*)^{1/2}$
. Spaces of the type
![]() $H(T)$
have been studied extensively in the literature, mostly in connection with operator models.
$H(T)$
have been studied extensively in the literature, mostly in connection with operator models.
There are two special cases that are especially interesting. First, if
![]() $\mathcal {H}=H^2$
is the classical Hardy space on the unit disk
$\mathcal {H}=H^2$
is the classical Hardy space on the unit disk
![]() $\mathbb {D}$
, and if
$\mathbb {D}$
, and if
![]() $T=T_\varphi $
is the analytic Toeplitz operator (multiplication operator) induced by a function
$T=T_\varphi $
is the analytic Toeplitz operator (multiplication operator) induced by a function
![]() $\varphi $
in the unit ball
$\varphi $
in the unit ball
![]() $H^\infty _1$
of
$H^\infty _1$
of
![]() $H^\infty $
, then
$H^\infty $
, then
![]() $H(T_\varphi )$
is called a sub-Hardy space (or a de Branges–Rovnyak space). Such spaces appeared in the work [Reference de Branges11] of de Branges concerning the Bieberbach conjecture and were studied systematically in Sarason’s monograph [Reference Sarason21]. See also the recent monograph [Reference Fricain and Mashreghi12].
$H(T_\varphi )$
is called a sub-Hardy space (or a de Branges–Rovnyak space). Such spaces appeared in the work [Reference de Branges11] of de Branges concerning the Bieberbach conjecture and were studied systematically in Sarason’s monograph [Reference Sarason21]. See also the recent monograph [Reference Fricain and Mashreghi12].
Second, if
![]() $\mathcal {H}=A^2$
is the classical Bergman space on the unit disk and if
$\mathcal {H}=A^2$
is the classical Bergman space on the unit disk and if
![]() $T=T_\varphi $
is the analytic Toeplitz operator (multiplication operator) on
$T=T_\varphi $
is the analytic Toeplitz operator (multiplication operator) on
![]() $A^2$
for some
$A^2$
for some
![]() $\varphi \in H^\infty _1$
, then
$\varphi \in H^\infty _1$
, then
![]() $H(T_\varphi )$
is naturally called a sub-Bergman space. Such spaces have been studied by several authors in the literature, beginning with [Reference Zhu25, Reference Zhu26] and including [Reference Abkar and Jafarzadeh1, Reference Chu8–Reference Chu10, Reference Gu13, Reference Gu, Hwang, Lee and Park14, Reference Nowak and Rososzczuk18, Reference Rososzczuk and Symesak20, Reference Sultanic22, Reference Symesak23].
$H(T_\varphi )$
is naturally called a sub-Bergman space. Such spaces have been studied by several authors in the literature, beginning with [Reference Zhu25, Reference Zhu26] and including [Reference Abkar and Jafarzadeh1, Reference Chu8–Reference Chu10, Reference Gu13, Reference Gu, Hwang, Lee and Park14, Reference Nowak and Rososzczuk18, Reference Rososzczuk and Symesak20, Reference Sultanic22, Reference Symesak23].
In this paper, we focus on sub-Bergman spaces in the weighted case. More specifically, we will consider a family of “generalized Bergman spaces”
![]() $A^2_\alpha $
. With the definition of generalized Bergman spaces
$A^2_\alpha $
. With the definition of generalized Bergman spaces
![]() $A^2_\alpha $
deferred to the next section, we mention the following special cases:
$A^2_\alpha $
deferred to the next section, we mention the following special cases:
![]() $A^2_0=A^2$
is the ordinary Bergman space,
$A^2_0=A^2$
is the ordinary Bergman space,
![]() $A^2_{-1}=H^2$
is the Hardy space, and
$A^2_{-1}=H^2$
is the Hardy space, and
![]() $A^2_{-2}=\mathcal {D}$
is the Dirichlet space. We will also consider multiplications operators
$A^2_{-2}=\mathcal {D}$
is the Dirichlet space. We will also consider multiplications operators
![]() $T_\varphi =T_\varphi ^\alpha : A^2_\alpha \to A^2_\alpha $
induced by functions from
$T_\varphi =T_\varphi ^\alpha : A^2_\alpha \to A^2_\alpha $
induced by functions from
![]() $\mathcal {M}_1(A^2_\alpha )$
, the closed unit ball of the multiplier algebra
$\mathcal {M}_1(A^2_\alpha )$
, the closed unit ball of the multiplier algebra
![]() $\mathcal {M}(A^2_\alpha )$
of
$\mathcal {M}(A^2_\alpha )$
of
![]() $A^2_\alpha $
. It is natural for us to use the notation
$A^2_\alpha $
. It is natural for us to use the notation
![]() $H^\alpha (\varphi )$
for the space
$H^\alpha (\varphi )$
for the space
![]() $H(T_\varphi )$
. Similarly, we will write
$H(T_\varphi )$
. Similarly, we will write
![]() $H^\alpha (\overline \varphi )$
for the space
$H^\alpha (\overline \varphi )$
for the space
![]() $H(T)$
when T is the adjoint operator
$H(T)$
when T is the adjoint operator
![]() $T^*_\varphi : A^2_\alpha \to A^2_\alpha $
. Note that for
$T^*_\varphi : A^2_\alpha \to A^2_\alpha $
. Note that for
![]() $\alpha \ge -1$
, we have
$\alpha \ge -1$
, we have
![]() $\mathcal {M}(A^2_\alpha )=H^\infty $
.
$\mathcal {M}(A^2_\alpha )=H^\infty $
.
Motivated by the main results obtained in [Reference Chu10, Reference Zhu26], we will study the following three problems:
-
(a) When does
 $H^\alpha (\varphi )$
have a complete Nevanlinna–Pick (CNP) kernel?
$H^\alpha (\varphi )$
have a complete Nevanlinna–Pick (CNP) kernel? -
(b) When do we have
 $H^\alpha (\varphi )=H^\alpha (\overline \varphi )=A^2_{\alpha -1}$
?
$H^\alpha (\varphi )=H^\alpha (\overline \varphi )=A^2_{\alpha -1}$
? -
(c) When do we have
 $(I-T_\varphi T_{\overline \varphi })(A^2_\alpha )=(I-T_{\overline \varphi }T_\varphi )(A^2_\alpha )=A^2_{\alpha -2}$
?
$(I-T_\varphi T_{\overline \varphi })(A^2_\alpha )=(I-T_{\overline \varphi }T_\varphi )(A^2_\alpha )=A^2_{\alpha -2}$
?
Our main results are Theorems A–C below.
Theorem A For
![]() $-1<\alpha \le 0$
, the space
$-1<\alpha \le 0$
, the space
![]() $H^\alpha (\varphi )$
has a CNP kernel if and only if
$H^\alpha (\varphi )$
has a CNP kernel if and only if
![]() $\varphi $
is a Möbius map. When
$\varphi $
is a Möbius map. When
![]() $\alpha> 0$
,
$\alpha> 0$
,
![]() $H^\alpha (\varphi )$
does not have a CNP kernel.
$H^\alpha (\varphi )$
does not have a CNP kernel.
A (more subtle) characterization is also obtained when
![]() $-2<\alpha <-1$
. Here, even the result for the case
$-2<\alpha <-1$
. Here, even the result for the case
![]() $\alpha =0$
is new. The case
$\alpha =0$
is new. The case
![]() $\alpha =-1$
was studied in [Reference Chu10].
$\alpha =-1$
was studied in [Reference Chu10].
Theorem B For
![]() $\alpha>-1$
, we have
$\alpha>-1$
, we have
![]() $H^\alpha (\varphi )=H^\alpha (\overline \varphi ) =A^2_{\alpha -1}$
if and only if
$H^\alpha (\varphi )=H^\alpha (\overline \varphi ) =A^2_{\alpha -1}$
if and only if
![]() $\varphi $
is a finite Blaschke product, which is also equivalent to the corresponding defect operators being compact.
$\varphi $
is a finite Blaschke product, which is also equivalent to the corresponding defect operators being compact.
Our methods rely on the assumption
![]() $\alpha>-1$
in a very critical way. In particular, the result above is definitely invalid when
$\alpha>-1$
in a very critical way. In particular, the result above is definitely invalid when
![]() $\alpha =-1$
(the Hardy space case). Some special cases of this result can be found in [Reference Abkar and Jafarzadeh1, Reference Chu8, Reference Chu9, Reference Gu, Hwang, Lee and Park14, Reference Sultanic22, Reference Zhu26].
$\alpha =-1$
(the Hardy space case). Some special cases of this result can be found in [Reference Abkar and Jafarzadeh1, Reference Chu8, Reference Chu9, Reference Gu, Hwang, Lee and Park14, Reference Sultanic22, Reference Zhu26].
Theorem C For
![]() $\alpha>-1$
, we have
$\alpha>-1$
, we have
![]() $(I-T_\varphi T_{\overline \varphi })(A^2_\alpha )= (I-T_{\overline \varphi }T_\varphi )(A^2_\alpha )=A^2_{\alpha -2}$
if and only if
$(I-T_\varphi T_{\overline \varphi })(A^2_\alpha )= (I-T_{\overline \varphi }T_\varphi )(A^2_\alpha )=A^2_{\alpha -2}$
if and only if
![]() $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product.
The special case
![]() $\alpha =0$
was proved in [Reference Zhu26]. Once again, the assumption
$\alpha =0$
was proved in [Reference Zhu26]. Once again, the assumption
![]() $\alpha>-1$
is critical here.
$\alpha>-1$
is critical here.
2 Generalized Bergman spaces
For any real number
![]() $\alpha $
, we fix some nonnegative integer k such that
$\alpha $
, we fix some nonnegative integer k such that
![]() $2k+\alpha>-1$
and let
$2k+\alpha>-1$
and let
![]() $A^2_\alpha $
denote the space of analytic functions f on
$A^2_\alpha $
denote the space of analytic functions f on
![]() $\mathbb {D}$
such that
$\mathbb {D}$
such that
where
Here,
![]() $dA$
is the normalized area measure on
$dA$
is the normalized area measure on
![]() $\mathbb {D}$
. It is easy to see that the weighted area measure
$\mathbb {D}$
. It is easy to see that the weighted area measure
![]() $dA_\alpha $
is finite if and only if
$dA_\alpha $
is finite if and only if
![]() $\alpha>-1$
, in which case we will normalize
$\alpha>-1$
, in which case we will normalize
![]() $dA_\alpha $
so that
$dA_\alpha $
so that
![]() $A_\alpha (\mathbb {D})=1$
.
$A_\alpha (\mathbb {D})=1$
.
It is well known that the space
![]() $A^2_\alpha $
is independent of the choice of the integer k used in (2.1). Two particular examples are worth mentioning:
$A^2_\alpha $
is independent of the choice of the integer k used in (2.1). Two particular examples are worth mentioning:
![]() $A^2_{-1}=H^2$
and
$A^2_{-1}=H^2$
and
![]() $A^2_{-2}=\mathcal {D}$
, the Hardy and Dirichlet spaces, respectively. See [Reference Zhao and Zhu24] for more information about the “generalized weighted Bergman spaces”
$A^2_{-2}=\mathcal {D}$
, the Hardy and Dirichlet spaces, respectively. See [Reference Zhao and Zhu24] for more information about the “generalized weighted Bergman spaces”
![]() $A^p_\alpha $
.
$A^p_\alpha $
.
Each space
![]() $A^2_\alpha $
is a Hilbert space with a certain choice of inner product. For example, if
$A^2_\alpha $
is a Hilbert space with a certain choice of inner product. For example, if
![]() $\alpha>-1$
, we can choose
$\alpha>-1$
, we can choose
![]() $k=0$
in (2.1) and simply use the natural inner product in
$k=0$
in (2.1) and simply use the natural inner product in
![]() $L^2(\mathbb {D}, dA_\alpha )$
for
$L^2(\mathbb {D}, dA_\alpha )$
for
![]() $A^2_\alpha $
:
$A^2_\alpha $
:
More generally, for any
![]() $\alpha>-2$
, it is easy to show that an analytic function
$\alpha>-2$
, it is easy to show that an analytic function
![]() $f(z)=\sum _{n=0}^\infty a_nz^n$
belongs to
$f(z)=\sum _{n=0}^\infty a_nz^n$
belongs to
![]() $A^2_\alpha $
if and only if
$A^2_\alpha $
if and only if
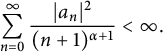 $$ \begin{align*}\sum_{n=0}^\infty\frac{|a_n|^2}{(n+1)^{\alpha+1}}<\infty.\end{align*} $$
$$ \begin{align*}\sum_{n=0}^\infty\frac{|a_n|^2}{(n+1)^{\alpha+1}}<\infty.\end{align*} $$
Since
as
![]() $n\to \infty $
, we see that
$n\to \infty $
, we see that
 $$ \begin{align*}\langle f,g\rangle=\sum_{n=0}^\infty\frac{n!\,\Gamma(2+\alpha)}{\Gamma(n+2+\alpha)}\,a_n\overline b_n,\qquad f(z)=\sum_{n=0}^\infty a_nz^n,\quad g(z)=\sum_{n=0}^\infty b_nz^n\end{align*} $$
$$ \begin{align*}\langle f,g\rangle=\sum_{n=0}^\infty\frac{n!\,\Gamma(2+\alpha)}{\Gamma(n+2+\alpha)}\,a_n\overline b_n,\qquad f(z)=\sum_{n=0}^\infty a_nz^n,\quad g(z)=\sum_{n=0}^\infty b_nz^n\end{align*} $$
defines an inner product on
![]() $A^2_\alpha $
. With this inner product, the functions
$A^2_\alpha $
. With this inner product, the functions
 $$ \begin{align*}e_n(z)=\sqrt{\frac{\Gamma(n+2+\alpha)}{n!\,\Gamma(2+\alpha)}}\,z^n,\qquad n\ge0,\end{align*} $$
$$ \begin{align*}e_n(z)=\sqrt{\frac{\Gamma(n+2+\alpha)}{n!\,\Gamma(2+\alpha)}}\,z^n,\qquad n\ge0,\end{align*} $$
form an orthonormal basis for
![]() $A^2_\alpha $
, which yields the reproducing kernel of
$A^2_\alpha $
, which yields the reproducing kernel of
![]() $A^2_\alpha $
as follows:
$A^2_\alpha $
as follows:
 $$ \begin{align} K(z,w)=\sum_{n=0}^\infty e_n(z)\overline{e_n(w)}=\sum_{n=0}^\infty\frac{\Gamma(n+2+\alpha)}{n!\,\Gamma(2+\alpha)} \,(z\overline w)^n=\frac1{(1-z\overline w)^{2+\alpha}}. \end{align} $$
$$ \begin{align} K(z,w)=\sum_{n=0}^\infty e_n(z)\overline{e_n(w)}=\sum_{n=0}^\infty\frac{\Gamma(n+2+\alpha)}{n!\,\Gamma(2+\alpha)} \,(z\overline w)^n=\frac1{(1-z\overline w)^{2+\alpha}}. \end{align} $$
Although all spaces
![]() $A^2_\alpha $
, when
$A^2_\alpha $
, when
![]() $\alpha>-2$
, have the same type of reproducing kernel as given in (2.2), their multiplier algebras depend on
$\alpha>-2$
, have the same type of reproducing kernel as given in (2.2), their multiplier algebras depend on
![]() $\alpha $
in a critical way. It is well known that
$\alpha $
in a critical way. It is well known that
![]() $\mathcal {M}(A^2_\alpha ) =H^\infty $
for
$\mathcal {M}(A^2_\alpha ) =H^\infty $
for
![]() $\alpha \ge -1$
. When
$\alpha \ge -1$
. When
![]() $\alpha <-1$
,
$\alpha <-1$
,
![]() $\mathcal {M}(A^2_\alpha )$
is a proper sub-algebra of
$\mathcal {M}(A^2_\alpha )$
is a proper sub-algebra of
![]() $H^\infty $
.
$H^\infty $
.
We will consider the defect operators
and the associated operators
where
![]() $\varphi \in \mathcal {M}_1(A^2_\alpha )$
and
$\varphi \in \mathcal {M}_1(A^2_\alpha )$
and
![]() $T_\varphi : A^2_\alpha \to A^2_\alpha $
is the (contractive) multiplication operator.
$T_\varphi : A^2_\alpha \to A^2_\alpha $
is the (contractive) multiplication operator.
Recall that
which are the generalized sub-Bergman Hilbert spaces defined in the Introduction. For any
![]() $\alpha>-2$
, just like the unweighted case
$\alpha>-2$
, just like the unweighted case
![]() $\alpha =0$
,
$\alpha =0$
,
![]() $H^\alpha (\varphi )$
is a reproducing kernel Hilbert space whose kernel function is given by
$H^\alpha (\varphi )$
is a reproducing kernel Hilbert space whose kernel function is given by
 $$ \begin{align} K^{\alpha,\varphi}(z,w)=K^{\alpha,\varphi}_w(z)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}. \end{align} $$
$$ \begin{align} K^{\alpha,\varphi}(z,w)=K^{\alpha,\varphi}_w(z)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}. \end{align} $$
Similarly,
![]() $H^\alpha (\overline \varphi )$
is a reproducing kernel Hilbert space whose kernel function is given by
$H^\alpha (\overline \varphi )$
is a reproducing kernel Hilbert space whose kernel function is given by
 $$ \begin{align*} K^{\alpha,\overline\varphi}(z,w)=K^{\alpha,\overline\varphi}_w(z)=\int_{\mathbb{D}}\frac{1-|\varphi(u)|^2} {(1-z\overline u)^{2+\alpha}(1-u\overline w)^{2+\alpha}}\,dA_\alpha(u). \end{align*} $$
$$ \begin{align*} K^{\alpha,\overline\varphi}(z,w)=K^{\alpha,\overline\varphi}_w(z)=\int_{\mathbb{D}}\frac{1-|\varphi(u)|^2} {(1-z\overline u)^{2+\alpha}(1-u\overline w)^{2+\alpha}}\,dA_\alpha(u). \end{align*} $$
The spaces
![]() $H^\alpha (\varphi )$
and
$H^\alpha (\varphi )$
and
![]() $H^\alpha (\overline \varphi )$
have been studied by several authors, mostly in the case
$H^\alpha (\overline \varphi )$
have been studied by several authors, mostly in the case
![]() $\alpha \ge 0$
. See [Reference Chu9, Reference Sultanic22] for example. We will generalize several results in the literature to weighted Bergman spaces
$\alpha \ge 0$
. See [Reference Chu9, Reference Sultanic22] for example. We will generalize several results in the literature to weighted Bergman spaces
![]() $A^2_\alpha $
with
$A^2_\alpha $
with
![]() $\alpha>-1$
.
$\alpha>-1$
.
3 Complete Nevanlinna–Pick kernels
In this section, we will determine exactly when the reproducing kernel function
![]() $K_w^{\alpha ,\varphi }$
in (2.3) is a CNP kernel. The following definition is from Theorem 8.2 in [Reference Agler and McCarthy3].
$K_w^{\alpha ,\varphi }$
in (2.3) is a CNP kernel. The following definition is from Theorem 8.2 in [Reference Agler and McCarthy3].
Definition 3.1 Suppose
![]() $K=K(z,w)=K_w(z)$
is an irreducible kernel function on a set
$K=K(z,w)=K_w(z)$
is an irreducible kernel function on a set
![]() $\Omega \,$
. K is called a CNP kernel if there are an auxiliary Hilbert space
$\Omega \,$
. K is called a CNP kernel if there are an auxiliary Hilbert space
![]() $\mathcal {L}$
, a function
$\mathcal {L}$
, a function
![]() $b: \Omega \rightarrow \mathcal {L}$
, and a nowhere vanishing function
$b: \Omega \rightarrow \mathcal {L}$
, and a nowhere vanishing function
![]() $\delta $
on
$\delta $
on
![]() $\Omega $
such that
$\Omega $
such that
 $$ \begin{align*}K_w(z) = \frac{\delta(z) \overline{\delta(w)}}{1-\langle b(z), b(w)\rangle},\qquad z,w\in\Omega.\end{align*} $$
$$ \begin{align*}K_w(z) = \frac{\delta(z) \overline{\delta(w)}}{1-\langle b(z), b(w)\rangle},\qquad z,w\in\Omega.\end{align*} $$
If K is a CNP kernel, the corresponding Hilbert space
![]() $\mathcal {H}(K)$
with kernel K is called a CNP space. CNP spaces share many properties with the Hardy space
$\mathcal {H}(K)$
with kernel K is called a CNP space. CNP spaces share many properties with the Hardy space
![]() $H^2$
, and they have been studied extensively in the literature (see, e.g., [Reference Agler and McCarthy2, Reference Aleman, Hartz, McCarthy and Richter4–Reference Aleman, Hartz, McCarthy and Richter7] and the references therein for recent developments). In 2020, Chu [Reference Chu10] determined which de Branges–Rovnyak spaces (sub-Hardy spaces) have CNP kernel. We will characterize which sub-Bergman spaces have CNP kernel.
$H^2$
, and they have been studied extensively in the literature (see, e.g., [Reference Agler and McCarthy2, Reference Aleman, Hartz, McCarthy and Richter4–Reference Aleman, Hartz, McCarthy and Richter7] and the references therein for recent developments). In 2020, Chu [Reference Chu10] determined which de Branges–Rovnyak spaces (sub-Hardy spaces) have CNP kernel. We will characterize which sub-Bergman spaces have CNP kernel.
The reproducing kernel for the Hardy space
![]() $H^2$
is
$H^2$
is
If
![]() $\varphi \in H^\infty _1$
is not a constant, we let
$\varphi \in H^\infty _1$
is not a constant, we let
Then
 $$ \begin{align*}K^{H^2}\circ \varphi(z,w) = K^{H^2}(\varphi(z),\varphi(w)) = \frac{1}{1-\varphi(z)\overline{\varphi(w)}}\end{align*} $$
$$ \begin{align*}K^{H^2}\circ \varphi(z,w) = K^{H^2}(\varphi(z),\varphi(w)) = \frac{1}{1-\varphi(z)\overline{\varphi(w)}}\end{align*} $$
is a kernel function and
![]() $C_\varphi : H^2 \rightarrow H(K^{H^2}\circ \varphi )$
defined by
$C_\varphi : H^2 \rightarrow H(K^{H^2}\circ \varphi )$
defined by
![]() $C_\varphi f = f \circ \varphi $
is a unitary (see [Reference Paulsen and Raghupathi19, p. 71]).
$C_\varphi f = f \circ \varphi $
is a unitary (see [Reference Paulsen and Raghupathi19, p. 71]).
Given
![]() $a\in \mathbb {D}$
, we let
$a\in \mathbb {D}$
, we let
denote the Möbius map that interchanges the points
![]() $0$
and a. If we take
$0$
and a. If we take
![]() $a=\varphi (0)$
and define
$a=\varphi (0)$
and define
 $$ \begin{align*}\psi(z)=\varphi_a(\varphi(z)),\qquad g(z)=\frac{\sqrt{1-|a|^2}}{1-\overline a\varphi(z)},\end{align*} $$
$$ \begin{align*}\psi(z)=\varphi_a(\varphi(z)),\qquad g(z)=\frac{\sqrt{1-|a|^2}}{1-\overline a\varphi(z)},\end{align*} $$
then an easy calculation shows that
See, e.g., [Reference Luo, Gu and Richter17, p. 18]. So
![]() $K^{\alpha ,\varphi }_w(z)$
is a CNP kernel if and only if
$K^{\alpha ,\varphi }_w(z)$
is a CNP kernel if and only if
![]() $K^{\alpha ,\psi }_w(z)$
is a CNP kernel.
$K^{\alpha ,\psi }_w(z)$
is a CNP kernel.
The following result can be obtained from [Reference Paulsen and Raghupathi19, Theorem 6.28].
Lemma 3.1 Let
![]() $\mathcal {H}_1$
and
$\mathcal {H}_1$
and
![]() $\mathcal {H}_2$
be reproducing kernel Hilbert spaces of functions on a set
$\mathcal {H}_2$
be reproducing kernel Hilbert spaces of functions on a set
![]() $\Omega $
with reproducing kernels
$\Omega $
with reproducing kernels
![]() $K_1$
and
$K_1$
and
![]() $K_2$
, respectively. Let
$K_2$
, respectively. Let
![]() $\mathcal {F}$
be a Hilbert space, and let
$\mathcal {F}$
be a Hilbert space, and let
![]() $\Phi : \Omega \rightarrow \mathcal {B}(\mathcal {F},\mathbb {C})$
be a function. Then the following are equivalent:
$\Phi : \Omega \rightarrow \mathcal {B}(\mathcal {F},\mathbb {C})$
be a function. Then the following are equivalent:
-
1.
 $\Phi $
is a contractive multiplier from
$\Phi $
is a contractive multiplier from
 $\mathcal {H}_1\otimes \mathcal {F}$
to
$\mathcal {H}_1\otimes \mathcal {F}$
to
 $\mathcal {H}_2$
.
$\mathcal {H}_2$
. -
2.
 $K_2(z,w)-K_1(z,w)\Phi (z)\Phi (w)^*$
is positive-definite.
$K_2(z,w)-K_1(z,w)\Phi (z)\Phi (w)^*$
is positive-definite.
We will use
![]() $\mathcal {M}_1(\mathcal {H}_1, \mathcal {H}_2)$
to denote the set of contractive multipliers from
$\mathcal {M}_1(\mathcal {H}_1, \mathcal {H}_2)$
to denote the set of contractive multipliers from
![]() $\mathcal {H}_1$
to
$\mathcal {H}_1$
to
![]() $\mathcal {H}_2$
. When
$\mathcal {H}_2$
. When
![]() $\mathcal {H}_1=\mathcal {H}_2=\mathcal {H}$
, we will simplify the notation to
$\mathcal {H}_1=\mathcal {H}_2=\mathcal {H}$
, we will simplify the notation to
![]() $\mathcal {M}_1(\mathcal {H})$
.
$\mathcal {M}_1(\mathcal {H})$
.
Lemma 3.2 Let
![]() $\varphi \in H^\infty _1$
be a nonconstant function. Then
$\varphi \in H^\infty _1$
be a nonconstant function. Then
Proof This follows easily from the fact that
![]() $C_{\varphi }: H^2 \rightarrow H(K^{H^2}\circ \varphi )$
is a unitary.
$C_{\varphi }: H^2 \rightarrow H(K^{H^2}\circ \varphi )$
is a unitary.
In what follows, we will use the notation
![]() $K(z,w)\succeq 0$
or
$K(z,w)\succeq 0$
or
![]() $0\preceq K(z,w)$
to mean that
$0\preceq K(z,w)$
to mean that
![]() $K(z,w)$
is a reproducing kernel, that is,
$K(z,w)$
is a reproducing kernel, that is,
![]() $K(z,w)=\overline {K(w,z)}$
and it is positive-definite in the sense that
$K(z,w)=\overline {K(w,z)}$
and it is positive-definite in the sense that
 $$ \begin{align*}\sum_{i,j=1}^NK(z_i,z_j)c_i\overline c_j\ge0\end{align*} $$
$$ \begin{align*}\sum_{i,j=1}^NK(z_i,z_j)c_i\overline c_j\ge0\end{align*} $$
for all
![]() $z_i\in \mathbb {D}$
and
$z_i\in \mathbb {D}$
and
![]() $c_i\in \mathbb {C}$
,
$c_i\in \mathbb {C}$
,
![]() $1\le i\le N$
, and
$1\le i\le N$
, and
![]() $N\ge 1$
. We will begin with the following result for the ordinary Bergman space, which illustrates the main techniques we use in this section.
$N\ge 1$
. We will begin with the following result for the ordinary Bergman space, which illustrates the main techniques we use in this section.
Theorem 3.3 Let
![]() $\varphi \in H^\infty _1$
and
$\varphi \in H^\infty _1$
and
![]() $\alpha =0$
. Then
$\alpha =0$
. Then
![]() $K^\varphi _w(z)=:K^{0,\varphi }_w(z)$
is a CNP kernel if and only if
$K^\varphi _w(z)=:K^{0,\varphi }_w(z)$
is a CNP kernel if and only if
![]() $\varphi $
is a Möbius map.
$\varphi $
is a Möbius map.
Proof If
![]() $\varphi $
is a Möbius map, say
$\varphi $
is a Möbius map, say
then it is easy to check that
 $$ \begin{align*}K^\varphi_w(z) = \frac{1-|a|^2}{(1-\overline az)(1-a\overline{w})}\frac{1}{1-z\overline{w}},\end{align*} $$
$$ \begin{align*}K^\varphi_w(z) = \frac{1-|a|^2}{(1-\overline az)(1-a\overline{w})}\frac{1}{1-z\overline{w}},\end{align*} $$
which is clearly a CNP kernel.
Conversely, suppose
![]() $K^\varphi _w(z)$
is a CNP kernel. If
$K^\varphi _w(z)$
is a CNP kernel. If
![]() $a = \varphi (0) \neq 0$
, then we consider
$a = \varphi (0) \neq 0$
, then we consider
![]() $\psi (z)=\varphi _a(\varphi (z))$
. By (3.1), we have that
$\psi (z)=\varphi _a(\varphi (z))$
. By (3.1), we have that
![]() $K^\psi _w(z)$
is a CNP kernel and
$K^\psi _w(z)$
is a CNP kernel and
![]() $\psi (0) = 0$
. So we will assume that
$\psi (0) = 0$
. So we will assume that
![]() $\varphi $
also satisfies
$\varphi $
also satisfies
![]() $\varphi (0) = 0$
, which implies
$\varphi (0) = 0$
, which implies
![]() $K^\varphi _0(z) = 1$
for all
$K^\varphi _0(z) = 1$
for all
![]() $z \in \mathbb {D}$
.
$z \in \mathbb {D}$
.
It is well known that if a reproducing kernel function
![]() $K_w(z)=K(z,w)$
on
$K_w(z)=K(z,w)$
on
![]() $\mathbb {D}$
satisfies
$\mathbb {D}$
satisfies
![]() $K(z,0)=1$
for all
$K(z,0)=1$
for all
![]() $z\in \mathbb {D}$
, then it is a CNP kernel if and only if
$z\in \mathbb {D}$
, then it is a CNP kernel if and only if
See [Reference Agler and McCarthy3, p. 88] for example. Since
 $$ \begin{align*}1 - \frac{1}{K^\varphi_w(z)} = 1 - \frac{(1-z\overline{w})^2}{1-\varphi(z)\overline{\varphi(w)}} = \frac{2z\overline{w}-z^2\overline{w^2}-\varphi(z)\overline{\varphi(w)}}{1-\varphi(z)\overline{\varphi(w)}},\end{align*} $$
$$ \begin{align*}1 - \frac{1}{K^\varphi_w(z)} = 1 - \frac{(1-z\overline{w})^2}{1-\varphi(z)\overline{\varphi(w)}} = \frac{2z\overline{w}-z^2\overline{w^2}-\varphi(z)\overline{\varphi(w)}}{1-\varphi(z)\overline{\varphi(w)}},\end{align*} $$
we have
 $$ \begin{align*}\frac{1-\frac{z}{\sqrt{2}}\frac{\overline{w}}{\sqrt{2}}-\frac{\varphi(z)}{\sqrt{2}z}\frac{\overline{\varphi(w)}}{\sqrt{2}\overline{w}}}{1-\varphi(z)\overline{\varphi(w)}} \succeq 0.\end{align*} $$
$$ \begin{align*}\frac{1-\frac{z}{\sqrt{2}}\frac{\overline{w}}{\sqrt{2}}-\frac{\varphi(z)}{\sqrt{2}z}\frac{\overline{\varphi(w)}}{\sqrt{2}\overline{w}}}{1-\varphi(z)\overline{\varphi(w)}} \succeq 0.\end{align*} $$
It follows from this and Lemma 3.1 that
 $$ \begin{align} \Phi(z) = \left(\frac{z}{\sqrt{2}}, \frac{\varphi(z)}{\sqrt{2}z}\right) \in \mathcal{M}_1\Bigl(H(K^{H^2}\circ \varphi) \otimes \mathbb{C}^2, H(K^{H^2}\circ \varphi)\Bigr). \end{align} $$
$$ \begin{align} \Phi(z) = \left(\frac{z}{\sqrt{2}}, \frac{\varphi(z)}{\sqrt{2}z}\right) \in \mathcal{M}_1\Bigl(H(K^{H^2}\circ \varphi) \otimes \mathbb{C}^2, H(K^{H^2}\circ \varphi)\Bigr). \end{align} $$
Thus,
 $$ \begin{align*}\frac{z}{\sqrt{2}}\in \mathcal{M}_1(H(K^{H^2}\circ \varphi)),\quad \frac{\varphi(z)}{\sqrt{2}z} \in \mathcal{M}_1(H(K^{H^2}\circ \varphi)).\end{align*} $$
$$ \begin{align*}\frac{z}{\sqrt{2}}\in \mathcal{M}_1(H(K^{H^2}\circ \varphi)),\quad \frac{\varphi(z)}{\sqrt{2}z} \in \mathcal{M}_1(H(K^{H^2}\circ \varphi)).\end{align*} $$
Using
![]() $z/\sqrt {2} \in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
and
$z/\sqrt {2} \in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
and
![]() $1 \in H(K^{H^2}\circ \varphi )$
, we can find a function
$1 \in H(K^{H^2}\circ \varphi )$
, we can find a function
![]() $h \in H^2$
such that
$h \in H^2$
such that
Therefore,
![]() $\varphi $
is injective, and by Lemma 3.2,
$\varphi $
is injective, and by Lemma 3.2,
![]() $h \in \mathcal {M}_1(H^2) = H^\infty _1$
and
$h \in \mathcal {M}_1(H^2) = H^\infty _1$
and
![]() $h(0) = 0$
. Similarly, we deduce from
$h(0) = 0$
. Similarly, we deduce from
![]() $\varphi (z)/(\sqrt {2}\,z) \in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
that
$\varphi (z)/(\sqrt {2}\,z) \in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
that
![]() $z/(2h) \in H^\infty _1$
. Then (3.2) implies that
$z/(2h) \in H^\infty _1$
. Then (3.2) implies that
Since
we conclude that
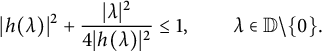 $$ \begin{align*}|h(\lambda)|^2 + \frac{|\lambda|^2}{4|h(\lambda)|^2} \leq 1, \qquad\lambda \in \mathbb{D}\setminus\{0\}.\end{align*} $$
$$ \begin{align*}|h(\lambda)|^2 + \frac{|\lambda|^2}{4|h(\lambda)|^2} \leq 1, \qquad\lambda \in \mathbb{D}\setminus\{0\}.\end{align*} $$
Passing to boundary limits, we obtain
for almost all
![]() $\lambda \in \mathbb {T}$
. It follows that
$\lambda \in \mathbb {T}$
. It follows that
![]() $|h(\lambda )| = \frac {1}{\sqrt {2}}$
for almost all
$|h(\lambda )| = \frac {1}{\sqrt {2}}$
for almost all
![]() $\lambda \in \mathbb {T}$
. Thus,
$\lambda \in \mathbb {T}$
. Thus,
![]() $\sqrt {2}h$
is an inner function. By the Schwarz lemma, the inequality
$\sqrt {2}h$
is an inner function. By the Schwarz lemma, the inequality
![]() $\sqrt 2|h(z)|\le 1$
together with
$\sqrt 2|h(z)|\le 1$
together with
![]() $h(0)=0$
implies that
$h(0)=0$
implies that
![]() $\sqrt 2|h(z)|\le |z|$
on
$\sqrt 2|h(z)|\le |z|$
on
![]() $\mathbb {D}$
. This along with
$\mathbb {D}$
. This along with
![]() $z/(2h)\in H^\infty _1$
shows that
$z/(2h)\in H^\infty _1$
shows that
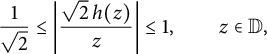 $$ \begin{align*}\frac1{\sqrt2}\le\left|\frac{\sqrt2\,h(z)}{z}\right|\le1,\qquad z\in\mathbb{D},\end{align*} $$
$$ \begin{align*}\frac1{\sqrt2}\le\left|\frac{\sqrt2\,h(z)}{z}\right|\le1,\qquad z\in\mathbb{D},\end{align*} $$
which implies that the inner function
![]() $\sqrt 2 h(z)/z$
has no zero inside
$\sqrt 2 h(z)/z$
has no zero inside
![]() $\mathbb {D}$
and has no singular factor. Therefore,
$\mathbb {D}$
and has no singular factor. Therefore,
![]() $\sqrt {2}h(z) = \zeta z$
for some
$\sqrt {2}h(z) = \zeta z$
for some
![]() $\zeta \in \mathbb {T}$
. It then follows from (3.3) that
$\zeta \in \mathbb {T}$
. It then follows from (3.3) that
![]() $\varphi (z) = \overline {\zeta }z$
, which finishes the proof of the theorem.
$\varphi (z) = \overline {\zeta }z$
, which finishes the proof of the theorem.
The characterization of CNP kernels for the sub-
![]() $A^2_\alpha $
spaces
$A^2_\alpha $
spaces
![]() $H^\alpha (\varphi )$
are more subtle though. The results we obtain will depend on the range of the parameter
$H^\alpha (\varphi )$
are more subtle though. The results we obtain will depend on the range of the parameter
![]() $\alpha $
.
$\alpha $
.
Theorem 3.4 Suppose
![]() $\varphi \in H^\infty _1$
and
$\varphi \in H^\infty _1$
and
![]() $-1<\alpha \le 0$
. Then the reproducing kernel of
$-1<\alpha \le 0$
. Then the reproducing kernel of
![]() $H^\alpha (\varphi )$
in (2.3) is a CNP kernel if and only if
$H^\alpha (\varphi )$
in (2.3) is a CNP kernel if and only if
![]() $\varphi $
is a Möbius map.
$\varphi $
is a Möbius map.
Proof The case
![]() $\alpha =0$
concerns the ordinary Bergman space, which is Theorem 3.3. So we assume
$\alpha =0$
concerns the ordinary Bergman space, which is Theorem 3.3. So we assume
![]() $-1<\alpha <0$
for the rest of this proof.
$-1<\alpha <0$
for the rest of this proof.
First, assume that
![]() $\varphi $
is a Möbius map, say
$\varphi $
is a Möbius map, say
![]() $\varphi (z)=\zeta \,\frac {a-z}{1-\overline az}$
with
$\varphi (z)=\zeta \,\frac {a-z}{1-\overline az}$
with
![]() $\zeta \in \mathbb {T}$
and
$\zeta \in \mathbb {T}$
and
![]() $a\in \mathbb {D}$
. Then an easy computation shows that the reproducing kernel for
$a\in \mathbb {D}$
. Then an easy computation shows that the reproducing kernel for
![]() $H^\alpha (\varphi )$
can be written as
$H^\alpha (\varphi )$
can be written as
 $$ \begin{align*}K(z,w)=\frac{1-|a|^2}{(1-\overline az)(1-a\overline w)}\,\frac1{(1-z\overline w)^{1+\alpha}},\end{align*} $$
$$ \begin{align*}K(z,w)=\frac{1-|a|^2}{(1-\overline az)(1-a\overline w)}\,\frac1{(1-z\overline w)^{1+\alpha}},\end{align*} $$
which is known to be a CNP kernel. See [Reference Agler and McCarthy3].
Next, we assume that the kernel for
![]() $H^\alpha (\varphi )$
in (2.3) is a CNP kernel. Once again, by considering
$H^\alpha (\varphi )$
in (2.3) is a CNP kernel. Once again, by considering
![]() $\psi (z)=\varphi _a\circ \varphi (z)$
with
$\psi (z)=\varphi _a\circ \varphi (z)$
with
![]() $a=\varphi (0)$
and using (3.1), we may assume that
$a=\varphi (0)$
and using (3.1), we may assume that
![]() $\varphi (0)=0$
.
$\varphi (0)=0$
.
When
![]() $\varphi (0)=0$
, we have
$\varphi (0)=0$
, we have
![]() $K^{\alpha ,\varphi }_0(z)=1$
for all
$K^{\alpha ,\varphi }_0(z)=1$
for all
![]() $z\in \mathbb {D}$
. In this case, it is known that the kernel
$z\in \mathbb {D}$
. In this case, it is known that the kernel
![]() $K^{\alpha ,\varphi }_w(z)$
is CNP if and only if
$K^{\alpha ,\varphi }_w(z)$
is CNP if and only if
![]() $1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
(see [Reference Agler and McCarthy3] for example). Since
$1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
(see [Reference Agler and McCarthy3] for example). Since
 $$ \begin{align*} 1&-\frac1{K^{\alpha,\varphi}_w(z)}=1-\frac{(1-z\overline w)^{2+\alpha}}{1-\varphi(z)\overline{\varphi(w)}}\\ &=\left[sz\overline w-\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\, z^n\overline w^n-\varphi(z)\overline{\varphi(w)}\,\right]\,\frac1{1-\varphi(z)\overline{\varphi(w)}}, \end{align*} $$
$$ \begin{align*} 1&-\frac1{K^{\alpha,\varphi}_w(z)}=1-\frac{(1-z\overline w)^{2+\alpha}}{1-\varphi(z)\overline{\varphi(w)}}\\ &=\left[sz\overline w-\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\, z^n\overline w^n-\varphi(z)\overline{\varphi(w)}\,\right]\,\frac1{1-\varphi(z)\overline{\varphi(w)}}, \end{align*} $$
where
![]() $s=\alpha +2\in (1,2)$
, we must have
$s=\alpha +2\in (1,2)$
, we must have
 $$ \begin{align*}\left[1-\sum_{n=2}^\infty\frac{(s-1)\,\Gamma(n-s)}{n!\,\Gamma(2-s)}\,z^{n-1}\overline w^{n-1}- \frac{\varphi(z)}{\sqrt s z}\,\frac{\overline{\varphi(w)}}{\sqrt s\overline w}\right]\,\frac1{1-\varphi(z)\overline{\varphi(w)}} \succeq 0.\end{align*} $$
$$ \begin{align*}\left[1-\sum_{n=2}^\infty\frac{(s-1)\,\Gamma(n-s)}{n!\,\Gamma(2-s)}\,z^{n-1}\overline w^{n-1}- \frac{\varphi(z)}{\sqrt s z}\,\frac{\overline{\varphi(w)}}{\sqrt s\overline w}\right]\,\frac1{1-\varphi(z)\overline{\varphi(w)}} \succeq 0.\end{align*} $$
Let
 $$ \begin{align*}\Phi(z)=\left(\frac{\varphi(z)}{\sqrt sz},\sqrt{\frac{s-1}{2!}}\,z,\ldots,\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\, z^{n-1},\ldots\right).\end{align*} $$
$$ \begin{align*}\Phi(z)=\left(\frac{\varphi(z)}{\sqrt sz},\sqrt{\frac{s-1}{2!}}\,z,\ldots,\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\, z^{n-1},\ldots\right).\end{align*} $$
By Lemma 3.1, we have
Thus,
 $$ \begin{align*}\frac{\varphi(z)}{\sqrt sz},\quad\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,z^{n-1}\in \mathcal{M}_1\Bigl(H(K^{H^2}\circ\varphi)\Bigr),\qquad n\ge2.\end{align*} $$
$$ \begin{align*}\frac{\varphi(z)}{\sqrt sz},\quad\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,z^{n-1}\in \mathcal{M}_1\Bigl(H(K^{H^2}\circ\varphi)\Bigr),\qquad n\ge2.\end{align*} $$
It follows from
 $$ \begin{align*}\sqrt{\frac{s-1}{2!}}\,z\in\mathcal{M}_1\Bigl(H(K^{H^2}\circ\varphi\Bigr),\quad 1\in H(K^{H^2}\circ\varphi),\end{align*} $$
$$ \begin{align*}\sqrt{\frac{s-1}{2!}}\,z\in\mathcal{M}_1\Bigl(H(K^{H^2}\circ\varphi\Bigr),\quad 1\in H(K^{H^2}\circ\varphi),\end{align*} $$
that there exists some function
![]() $h\in H^2$
such that
$h\in H^2$
such that
 $$ \begin{align} \sqrt{\frac{s-1}{2}}\,z=\sqrt{\frac{s-1}{2}}\,h(\varphi(z)),\qquad z\in\mathbb{D}. \end{align} $$
$$ \begin{align} \sqrt{\frac{s-1}{2}}\,z=\sqrt{\frac{s-1}{2}}\,h(\varphi(z)),\qquad z\in\mathbb{D}. \end{align} $$
Therefore,
![]() $\varphi $
is injective, and by Lemma 3.2,
$\varphi $
is injective, and by Lemma 3.2,
 $$ \begin{align*}\sqrt{\frac{s-1}{2}}\,h\in\mathcal{M}_1(H^2)=H^\infty_1\end{align*} $$
$$ \begin{align*}\sqrt{\frac{s-1}{2}}\,h\in\mathcal{M}_1(H^2)=H^\infty_1\end{align*} $$
with
![]() $h(0)=0$
. Then we also have
$h(0)=0$
. Then we also have
 $$ \begin{align*}\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,z^{n-1}= \sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,h(\varphi(z))^{n-1},\qquad n\ge2.\end{align*} $$
$$ \begin{align*}\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,z^{n-1}= \sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,h(\varphi(z))^{n-1},\qquad n\ge2.\end{align*} $$
Similarly, from
![]() $\varphi (z)/\sqrt sz\in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
, we obtain
$\varphi (z)/\sqrt sz\in \mathcal {M}_1(H(K^{H^2}\circ \varphi ))$
, we obtain
![]() $z/\sqrt sh\in H^\infty _1$
.
$z/\sqrt sh\in H^\infty _1$
.
By (3.4), we must have
 $$ \begin{align*} T(z)&:=\left(\frac z{\sqrt sh},\sqrt{\frac{s-1}{2!}}\,h,\ldots,\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,h^{n-1}, \ldots\right)\\ &\in\mathcal{M}_1(H^2\otimes l^2, H^2). \end{align*} $$
$$ \begin{align*} T(z)&:=\left(\frac z{\sqrt sh},\sqrt{\frac{s-1}{2!}}\,h,\ldots,\sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,h^{n-1}, \ldots\right)\\ &\in\mathcal{M}_1(H^2\otimes l^2, H^2). \end{align*} $$
Note that
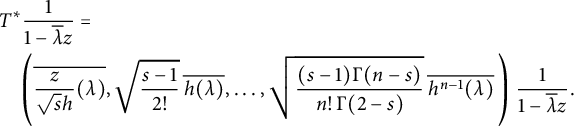 $$ \begin{align*} &T^*\frac1{1-\overline\lambda z}=\\ &\quad\left(\overline{\frac z{\sqrt sh}(\lambda)},\sqrt{\frac{s-1}{2!}}\,\overline{h(\lambda)},\ldots, \sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,\overline{h^{n-1}(\lambda)}\,\right)\,\frac1{1-\overline\lambda z}. \end{align*} $$
$$ \begin{align*} &T^*\frac1{1-\overline\lambda z}=\\ &\quad\left(\overline{\frac z{\sqrt sh}(\lambda)},\sqrt{\frac{s-1}{2!}}\,\overline{h(\lambda)},\ldots, \sqrt{\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}}\,\overline{h^{n-1}(\lambda)}\,\right)\,\frac1{1-\overline\lambda z}. \end{align*} $$
It follows that
 $$ \begin{align*}\frac{|\lambda|^2}{s|h(\lambda)|^2}+\sum_{n=2}^\infty\frac{(s-1)\Gamma(n-2)}{n!\,\Gamma(2-s)} \,|h(\lambda)|^{2n-2}\le1,\qquad\lambda\in\mathbb{D}\setminus\{0\}.\end{align*} $$
$$ \begin{align*}\frac{|\lambda|^2}{s|h(\lambda)|^2}+\sum_{n=2}^\infty\frac{(s-1)\Gamma(n-2)}{n!\,\Gamma(2-s)} \,|h(\lambda)|^{2n-2}\le1,\qquad\lambda\in\mathbb{D}\setminus\{0\}.\end{align*} $$
Passing to radial limits, we obtain
 $$ \begin{align*}\frac1{s|h(\lambda)|^2}+\sum_{n=2}^\infty\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,|h(\lambda)|^{2n-2}\le1\end{align*} $$
$$ \begin{align*}\frac1{s|h(\lambda)|^2}+\sum_{n=2}^\infty\frac{(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,|h(\lambda)|^{2n-2}\le1\end{align*} $$
or
 $$ \begin{align*}1+\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,|h(\lambda)|^{2n}\le s|h(\lambda)|^2\end{align*} $$
$$ \begin{align*}1+\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,|h(\lambda)|^{2n}\le s|h(\lambda)|^2\end{align*} $$
for almost all
![]() $\lambda \in \mathbb {T}$
. We necessarily have
$\lambda \in \mathbb {T}$
. We necessarily have
![]() $|h(\lambda )|^2\le 1$
. Comparing the above inequality with the classical Taylor series
$|h(\lambda )|^2\le 1$
. Comparing the above inequality with the classical Taylor series
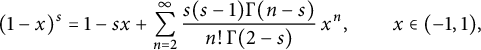 $$ \begin{align*}(1-x)^s=1-sx+\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,x^n,\qquad x\in(-1,1),\end{align*} $$
$$ \begin{align*}(1-x)^s=1-sx+\sum_{n=2}^\infty\frac{s(s-1)\Gamma(n-s)}{n!\,\Gamma(2-s)}\,x^n,\qquad x\in(-1,1),\end{align*} $$
we obtain
![]() $(1-|h(\lambda )|^2)^s\le 0$
for almost all
$(1-|h(\lambda )|^2)^s\le 0$
for almost all
![]() $\lambda \in \mathbb {T}$
, so h is an inner function. This together with
$\lambda \in \mathbb {T}$
, so h is an inner function. This together with
![]() $z/\sqrt sh\in H^\infty _1$
implies that
$z/\sqrt sh\in H^\infty _1$
implies that
![]() $h(z)=\zeta z$
for some constant
$h(z)=\zeta z$
for some constant
![]() $\zeta \in \mathbb {T}$
. By (3.5), we have
$\zeta \in \mathbb {T}$
. By (3.5), we have
![]() $\varphi (z)=\overline \zeta \,z$
. This completes the proof of the theorem.
$\varphi (z)=\overline \zeta \,z$
. This completes the proof of the theorem.
Note that, in the case when
![]() $\alpha =-1$
, a characterization for
$\alpha =-1$
, a characterization for
![]() $\varphi \in H^\infty _1$
was obtained in [Reference Chu10] in order for the kernel
$\varphi \in H^\infty _1$
was obtained in [Reference Chu10] in order for the kernel
 $$ \begin{align*}K(z,w)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}= \frac{1-\varphi(z)\overline{\varphi(w)}}{1-z\overline w}\end{align*} $$
$$ \begin{align*}K(z,w)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}= \frac{1-\varphi(z)\overline{\varphi(w)}}{1-z\overline w}\end{align*} $$
to be CNP. The necessary and sufficient condition for
![]() $\varphi $
is the following: there exists a function
$\varphi $
is the following: there exists a function
![]() $h\in H^\infty _1$
such that
$h\in H^\infty _1$
such that
![]() $\psi (z)=zh(\psi (z))$
, where
$\psi (z)=zh(\psi (z))$
, where
![]() $\psi (z)=\varphi _a(\varphi (z))$
with
$\psi (z)=\varphi _a(\varphi (z))$
with
![]() $a=\varphi (0)$
.
$a=\varphi (0)$
.
When
![]() $-2<\alpha <-1$
, we have the following result.
$-2<\alpha <-1$
, we have the following result.
Theorem 3.5 Suppose
![]() $-2<\alpha <-1$
and
$-2<\alpha <-1$
and
![]() $\varphi \in \mathcal {M}_1(A^2_\alpha )$
. Let
$\varphi \in \mathcal {M}_1(A^2_\alpha )$
. Let
![]() $a=\varphi (0)$
and
$a=\varphi (0)$
and
![]() $\psi =\varphi _a\circ \varphi $
. Then the function
$\psi =\varphi _a\circ \varphi $
. Then the function
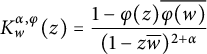 $$ \begin{align*}K^{\alpha,\varphi}_w(z)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}\end{align*} $$
$$ \begin{align*}K^{\alpha,\varphi}_w(z)=\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline w)^{2+\alpha}}\end{align*} $$
is a CNP kernel if and only if there exists
such that
 $$ \begin{align*}\psi(z)=\sum_{n=1}^\infty\sqrt{\frac{(2+\alpha)\Gamma(n-\alpha-2)}{n!\,\Gamma(-1-\alpha)}}\,z^nh_n(\psi(z))\end{align*} $$
$$ \begin{align*}\psi(z)=\sum_{n=1}^\infty\sqrt{\frac{(2+\alpha)\Gamma(n-\alpha-2)}{n!\,\Gamma(-1-\alpha)}}\,z^nh_n(\psi(z))\end{align*} $$
on
![]() $\mathbb {D}$
.
$\mathbb {D}$
.
Proof Recall from (3.1) that
![]() $K^{\alpha ,\varphi }_w(z)$
is a CNP kernel if and only if
$K^{\alpha ,\varphi }_w(z)$
is a CNP kernel if and only if
![]() $K^{\alpha ,\psi }_w(z)$
is a CNP kernel. So we will assume that
$K^{\alpha ,\psi }_w(z)$
is a CNP kernel. So we will assume that
![]() $\varphi (0)=0$
. In this case, we have
$\varphi (0)=0$
. In this case, we have
![]() $K^{\alpha ,\varphi }_0(z)=1$
for all
$K^{\alpha ,\varphi }_0(z)=1$
for all
![]() $z\in \mathbb {D}$
and
$z\in \mathbb {D}$
and
![]() $1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
.
$1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
.
Let
![]() $s=\alpha +2$
and write
$s=\alpha +2$
and write
 $$ \begin{align*} 1-\frac1{K^{\alpha,\varphi}_w(z)}&=1-\frac{(1-z\overline w)^s}{1-\varphi(z)\overline{\varphi(w)}}\\ &=\left(\sum_{n=1}^\infty\frac{s\Gamma(n-s)}{n!\,\Gamma(1-s)}\,z^n\overline w^n-\varphi(z)\overline{\varphi(w)}\right) \,\frac1{1-\varphi(z)\overline{\varphi(w)}}. \end{align*} $$
$$ \begin{align*} 1-\frac1{K^{\alpha,\varphi}_w(z)}&=1-\frac{(1-z\overline w)^s}{1-\varphi(z)\overline{\varphi(w)}}\\ &=\left(\sum_{n=1}^\infty\frac{s\Gamma(n-s)}{n!\,\Gamma(1-s)}\,z^n\overline w^n-\varphi(z)\overline{\varphi(w)}\right) \,\frac1{1-\varphi(z)\overline{\varphi(w)}}. \end{align*} $$
Since
![]() $1/(1-\varphi (z)\overline {\varphi (w)})$
is a CNP kernel, it follows from Theorem 8.57 of [Reference Agler and McCarthy3] that
$1/(1-\varphi (z)\overline {\varphi (w)})$
is a CNP kernel, it follows from Theorem 8.57 of [Reference Agler and McCarthy3] that
![]() $1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
if and only if there exists
$1-[1/K^{\alpha ,\varphi }_w(z)]\succeq 0$
if and only if there exists
such that
 $$ \begin{align*}\varphi(z)=\sum_{n=1}^\infty\sqrt{\frac{s\Gamma(n-s)}{n!\,\Gamma(1-s)}}\,z^n\varphi_n(z).\end{align*} $$
$$ \begin{align*}\varphi(z)=\sum_{n=1}^\infty\sqrt{\frac{s\Gamma(n-s)}{n!\,\Gamma(1-s)}}\,z^n\varphi_n(z).\end{align*} $$
By Lemma 3.2, there exist
![]() $h=(h_n)\subset H^\infty _1$
such that
$h=(h_n)\subset H^\infty _1$
such that
![]() $\varphi _n(z)=h_n(\varphi (z))$
for all n and
$\varphi _n(z)=h_n(\varphi (z))$
for all n and
![]() $h\in \text {Mult}_1(H^2, H^2\otimes l^2)$
. This proves the desired result.
$h\in \text {Mult}_1(H^2, H^2\otimes l^2)$
. This proves the desired result.
For an example of a CNP kernel
![]() $K^{\alpha ,\varphi }_w(z)$
when
$K^{\alpha ,\varphi }_w(z)$
when
![]() $-2<\alpha <-1$
, fix any positive integer n and consider
$-2<\alpha <-1$
, fix any positive integer n and consider
 $$ \begin{align*}\varphi(z)=\sqrt{\frac{(2+\alpha)\Gamma(n-2-\alpha)}{n!\,\Gamma(-1-\alpha)}}\,z^n.\end{align*} $$
$$ \begin{align*}\varphi(z)=\sqrt{\frac{(2+\alpha)\Gamma(n-2-\alpha)}{n!\,\Gamma(-1-\alpha)}}\,z^n.\end{align*} $$
It is easy to see that
![]() $\varphi \in \mathcal {M}_1(A^2_\alpha )$
and, by the theorem above,
$\varphi \in \mathcal {M}_1(A^2_\alpha )$
and, by the theorem above,
![]() $K^{\alpha ,\varphi }_w(z)$
is a CNP kernel. Also, if
$K^{\alpha ,\varphi }_w(z)$
is a CNP kernel. Also, if
![]() $h = (\frac {1}{2}, \frac {1}{4}, \ldots , \frac {1}{2^n}, \ldots )$
, and
$h = (\frac {1}{2}, \frac {1}{4}, \ldots , \frac {1}{2^n}, \ldots )$
, and
 $$ \begin{align*}\varphi(z) = \sum_{n=1}^\infty\sqrt{\frac{(2+\alpha)\Gamma(n-2-\alpha)}{n!\,\Gamma(-1-\alpha)}}\,\frac{z^n}{2^n},\end{align*} $$
$$ \begin{align*}\varphi(z) = \sum_{n=1}^\infty\sqrt{\frac{(2+\alpha)\Gamma(n-2-\alpha)}{n!\,\Gamma(-1-\alpha)}}\,\frac{z^n}{2^n},\end{align*} $$
then
![]() $h \in \text {Mult}_1(H^2, H^2\otimes l^2)$
,
$h \in \text {Mult}_1(H^2, H^2\otimes l^2)$
,
![]() $\varphi \in \mathcal {M}_1(A^2_\alpha )$
, and
$\varphi \in \mathcal {M}_1(A^2_\alpha )$
, and
![]() $K^{\alpha ,\varphi }_w(z)$
is a CNP kernel.
$K^{\alpha ,\varphi }_w(z)$
is a CNP kernel.
When
![]() $\alpha>0$
, the identity function
$\alpha>0$
, the identity function
![]() $\varphi (z)=z$
belongs to
$\varphi (z)=z$
belongs to
![]() $H^\infty _1= \mathcal {M}_1(A^2_\alpha )$
, but
$H^\infty _1= \mathcal {M}_1(A^2_\alpha )$
, but
is NOT a CNP kernel (see [Reference Agler and McCarthy3]). In fact, when
![]() $\alpha> 0$
,
$\alpha> 0$
,
![]() $K^{\alpha ,\varphi }_w(z)$
is not a CNP kernel for any
$K^{\alpha ,\varphi }_w(z)$
is not a CNP kernel for any
![]() $\varphi \in \mathcal {M}_1(A^2_\alpha ) = H^\infty _1$
. The following result was communicated to us by Michael Hartz.
$\varphi \in \mathcal {M}_1(A^2_\alpha ) = H^\infty _1$
. The following result was communicated to us by Michael Hartz.
Theorem 3.6 [Reference Hartz16]
Suppose
![]() $\alpha> 0$
and
$\alpha> 0$
and
![]() $\varphi \in H^\infty _1$
. Then
$\varphi \in H^\infty _1$
. Then
![]() $K^{\alpha ,\varphi }_w(z)$
is not a CNP kernel.
$K^{\alpha ,\varphi }_w(z)$
is not a CNP kernel.
Proof We prove it by contradiction. Suppose
![]() $K^{\alpha ,\varphi }_w(z)$
is a CNP kernel. By the same observation as before, we may assume
$K^{\alpha ,\varphi }_w(z)$
is a CNP kernel. By the same observation as before, we may assume
![]() $\varphi (0) = 0$
. Note that when
$\varphi (0) = 0$
. Note that when
![]() $\alpha> 0$
,
$\alpha> 0$
,
 $$ \begin{align*}\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline{w})^{1+\alpha}} \succeq 0.\end{align*} $$
$$ \begin{align*}\frac{1-\varphi(z)\overline{\varphi(w)}}{(1-z\overline{w})^{1+\alpha}} \succeq 0.\end{align*} $$
Thus, let
![]() $S_w(z) = 1/(1-z\overline {w})$
be the Szegő kernel, then
$S_w(z) = 1/(1-z\overline {w})$
be the Szegő kernel, then
![]() $K^{\alpha , \varphi }/S$
is positive-definite. Then an application of the Schur product theorem shows that
$K^{\alpha , \varphi }/S$
is positive-definite. Then an application of the Schur product theorem shows that
![]() $H^\infty (\mathbb {D}) = \mathcal {M}(H^2)$
is contractively contained in
$H^\infty (\mathbb {D}) = \mathcal {M}(H^2)$
is contractively contained in
![]() $\mathcal {M}(H^\alpha (\varphi ))$
(see [Reference Hartz15, Corollary 3.5] or the proof in Lemma 4.2). Since
$\mathcal {M}(H^\alpha (\varphi ))$
(see [Reference Hartz15, Corollary 3.5] or the proof in Lemma 4.2). Since
![]() $\mathcal {M}(H^\alpha (\varphi ))$
is also contractively contained in
$\mathcal {M}(H^\alpha (\varphi ))$
is also contractively contained in
![]() $H^\infty (\mathbb {D})$
, we conclude that
$H^\infty (\mathbb {D})$
, we conclude that
![]() $\mathcal {M}(H^\alpha (\varphi )) = H^\infty (\mathbb {D})$
with equality of norms.
$\mathcal {M}(H^\alpha (\varphi )) = H^\infty (\mathbb {D})$
with equality of norms.
Now, a normalized CNP kernel is uniquely determined by its multiplier algebra (see [Reference Hartz15, Corollary 3.2]). Since
![]() $K^{\alpha , \varphi }_w(z)$
and
$K^{\alpha , \varphi }_w(z)$
and
![]() $S_w(z)$
are CNP kernels, it follows that
$S_w(z)$
are CNP kernels, it follows that
![]() $K^{\alpha , \varphi }_w(z) = S_w(z)$
. Thus,
$K^{\alpha , \varphi }_w(z) = S_w(z)$
. Thus,
Setting
![]() $w =z$
, we obtain that
$w =z$
, we obtain that
But by the Schwarz lemma,
![]() $|\varphi (z)| \leq |z|$
, from which we see that the above equation cannot be held when
$|\varphi (z)| \leq |z|$
, from which we see that the above equation cannot be held when
![]() $\alpha> 0$
. This contraction then finishes the proof.
$\alpha> 0$
. This contraction then finishes the proof.
The above argument also works for
![]() $\alpha = 0$
, and it provides a different proof of Theorem 3.3.
$\alpha = 0$
, and it provides a different proof of Theorem 3.3.
4 Compactness of defect operators
In this section, we will characterize functions
![]() $\varphi \in H^\infty _1$
such that the defect operators
$\varphi \in H^\infty _1$
such that the defect operators
![]() $D^\alpha _\varphi $
and
$D^\alpha _\varphi $
and
![]() $D^\alpha _{\overline \varphi }$
, where
$D^\alpha _{\overline \varphi }$
, where
![]() $\alpha>-1$
, are compact. The following result follows from I-9 of [Reference Sarason21].
$\alpha>-1$
, are compact. The following result follows from I-9 of [Reference Sarason21].
Lemma 4.1 Let
![]() $\alpha> -1$
,
$\alpha> -1$
,
![]() $\varphi \in H^\infty _1$
, and
$\varphi \in H^\infty _1$
, and
![]() $M^\alpha (\varphi ) = \varphi A^2_\alpha $
. Then
$M^\alpha (\varphi ) = \varphi A^2_\alpha $
. Then
The following result was proved in [Reference Nowak and Rososzczuk18, Reference Symesak23]. We provide a different proof here.
Lemma 4.2 Let
![]() $\alpha> -1$
and
$\alpha> -1$
and
![]() $\varphi \in H^\infty _1$
. If
$\varphi \in H^\infty _1$
. If
![]() $\varphi $
is a finite Blaschke product, then
$\varphi $
is a finite Blaschke product, then
Proof By the definition of
![]() $A^2_{\alpha -1}$
, it is not hard to see that any function that is analytic on the closed unit disk is a multiplier of
$A^2_{\alpha -1}$
, it is not hard to see that any function that is analytic on the closed unit disk is a multiplier of
![]() $A^2_{\alpha -1}$
. In particular,
$A^2_{\alpha -1}$
. In particular,
![]() $T_\varphi $
is a bounded operator on
$T_\varphi $
is a bounded operator on
![]() $A^2_{\alpha -1}$
. If
$A^2_{\alpha -1}$
. If
![]() $\|T_{\varphi }\|_{B(A^2_{\alpha -1})} = C < \infty $
, then
$\|T_{\varphi }\|_{B(A^2_{\alpha -1})} = C < \infty $
, then
 $$ \begin{align*}(I- T_{\varphi} T_{\varphi}^*/C^2)K^{\alpha-1}_w(z) = \frac{1-\varphi(z)\overline{\varphi(w)}/C^2}{(1-z\overline{w})^{1+\alpha}} \succeq 0.\end{align*} $$
$$ \begin{align*}(I- T_{\varphi} T_{\varphi}^*/C^2)K^{\alpha-1}_w(z) = \frac{1-\varphi(z)\overline{\varphi(w)}/C^2}{(1-z\overline{w})^{1+\alpha}} \succeq 0.\end{align*} $$
Thus, by the Schur product theorem [Reference Paulsen and Raghupathi19],
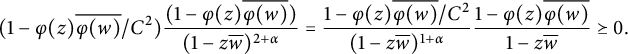 $$ \begin{align*}(1-\varphi(z)\overline{\varphi(w)}/C^2)\frac{(1-\varphi(z)\overline{\varphi(w)})}{(1-z\overline{w})^{2+\alpha}} = \frac{1-\varphi(z)\overline{\varphi(w)}/C^2}{(1-z\overline{w})^{1+\alpha}}\frac{1-\varphi(z)\overline{\varphi(w)}}{1-z\overline{w}} \succeq 0.\end{align*} $$
$$ \begin{align*}(1-\varphi(z)\overline{\varphi(w)}/C^2)\frac{(1-\varphi(z)\overline{\varphi(w)})}{(1-z\overline{w})^{2+\alpha}} = \frac{1-\varphi(z)\overline{\varphi(w)}/C^2}{(1-z\overline{w})^{1+\alpha}}\frac{1-\varphi(z)\overline{\varphi(w)}}{1-z\overline{w}} \succeq 0.\end{align*} $$
It follows that
![]() $\varphi /C$
is a contractive multiplier of
$\varphi /C$
is a contractive multiplier of
![]() $H^{\alpha }(\varphi )$
. Thus,
$H^{\alpha }(\varphi )$
. Thus,
![]() $\varphi H^{\alpha }(\varphi ) \subseteq H^{\alpha }(\varphi )$
. Combining this with
$\varphi H^{\alpha }(\varphi ) \subseteq H^{\alpha }(\varphi )$
. Combining this with
![]() $H^{\alpha }(\varphi ) \subseteq A^2_\alpha $
, we obtain
$H^{\alpha }(\varphi ) \subseteq A^2_\alpha $
, we obtain
By Lemma 4.1, we then have
![]() $\varphi H^{\alpha }(\varphi ) \subseteq \varphi H^{\alpha }(\overline {\varphi })$
, so
$\varphi H^{\alpha }(\varphi ) \subseteq \varphi H^{\alpha }(\overline {\varphi })$
, so
![]() $H^{\alpha }(\varphi ) \subseteq H^{\alpha }(\overline {\varphi })$
.
$H^{\alpha }(\varphi ) \subseteq H^{\alpha }(\overline {\varphi })$
.
To finish the proof, we note
![]() $H^{\alpha }(\varphi ) = A^2_{\alpha -1}$
[Reference Sultanic22] and use the fact that the subnormality of
$H^{\alpha }(\varphi ) = A^2_{\alpha -1}$
[Reference Sultanic22] and use the fact that the subnormality of
![]() $T_\varphi $
gives
$T_\varphi $
gives
![]() $H^{\alpha }(\overline {\varphi }) \subseteq H^{\alpha }(\varphi )$
in general.
$H^{\alpha }(\overline {\varphi }) \subseteq H^{\alpha }(\varphi )$
in general.
Lemma 4.3 Let
![]() $\varphi $
be a nonconstant function in
$\varphi $
be a nonconstant function in
![]() $H^\infty _1$
. Then the following conditions are equivalent.
$H^\infty _1$
. Then the following conditions are equivalent.
-
(a)
 $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product. -
(b)
 $1-|\varphi (z)|^2\to 0$
as
$1-|\varphi (z)|^2\to 0$
as
 $|z|\to 1^-$
.
$|z|\to 1^-$
. -
(c)
 $(1-|\varphi (z)|^2)/(1-|z|^2)$
is bounded both above and below on
$(1-|\varphi (z)|^2)/(1-|z|^2)$
is bounded both above and below on
 $\mathbb {D}$
.
$\mathbb {D}$
.
Proof The equivalence of (a) and (c) was proved in [Reference Zhu26]. It is trivial that (c) implies (b).
If (b) holds, then
![]() $|\varphi (z)|\to 1$
uniformly as
$|\varphi (z)|\to 1$
uniformly as
![]() $|z|\to 1^-$
, so
$|z|\to 1^-$
, so
![]() $\varphi $
is an inner function. It is clear that
$\varphi $
is an inner function. It is clear that
![]() $\varphi $
cannot have infinitely many zeros. If
$\varphi $
cannot have infinitely many zeros. If
![]() $\varphi $
contains a singular inner factor S, then there exists at least one point
$\varphi $
contains a singular inner factor S, then there exists at least one point
![]() $\zeta \in \mathbb {T}$
such that
$\zeta \in \mathbb {T}$
such that
![]() $S(z)\to 0$
as z approaches
$S(z)\to 0$
as z approaches
![]() $\zeta $
radially, which contradicts with the limit
$\zeta $
radially, which contradicts with the limit
![]() $|\varphi (z)|\to 1$
as
$|\varphi (z)|\to 1$
as
![]() $|z|\to 1^-$
. Thus,
$|z|\to 1^-$
. Thus,
![]() $\varphi $
cannot contain any singular inner factor. Hence,
$\varphi $
cannot contain any singular inner factor. Hence,
![]() $\varphi $
must be a finite Blaschke product. This shows that (b) implies (a) and completes the proof of the lemma.
$\varphi $
must be a finite Blaschke product. This shows that (b) implies (a) and completes the proof of the lemma.
Lemma 4.4 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $T: A^2_\alpha \to A^2_\alpha $
is a bounded linear operator. If the range of T is contained in
$T: A^2_\alpha \to A^2_\alpha $
is a bounded linear operator. If the range of T is contained in
![]() $A^2_\gamma $
for some
$A^2_\gamma $
for some
![]() $\gamma <\alpha $
, then T belongs to the Schatten class
$\gamma <\alpha $
, then T belongs to the Schatten class
![]() $S_p$
for all
$S_p$
for all
![]() $p>2/(\alpha -\gamma )$
.
$p>2/(\alpha -\gamma )$
.
Proof It is well known that if
![]() $\gamma <\alpha $
, then
$\gamma <\alpha $
, then
![]() $A^2_\gamma \subset A^2_\alpha $
, and the inclusion mapping
$A^2_\gamma \subset A^2_\alpha $
, and the inclusion mapping
![]() $i: A^2_\gamma \to A^2_\alpha $
is bounded. If T maps
$i: A^2_\gamma \to A^2_\alpha $
is bounded. If T maps
![]() $A^2_\alpha $
into
$A^2_\alpha $
into
![]() $A^2_\gamma $
, then by the closed graph theorem, there exists a constant
$A^2_\gamma $
, then by the closed graph theorem, there exists a constant
![]() $C>0$
such that
$C>0$
such that
![]() $\|Tf\|_{A^2_\gamma }\le C\|f\|_{A^2_\alpha }$
for all
$\|Tf\|_{A^2_\gamma }\le C\|f\|_{A^2_\alpha }$
for all
![]() $f\in A^2_\alpha $
, that is, T can be thought of as a bounded linear operator from
$f\in A^2_\alpha $
, that is, T can be thought of as a bounded linear operator from
![]() $A^2_\alpha $
into
$A^2_\alpha $
into
![]() $A^2_\gamma $
. We can then write
$A^2_\gamma $
. We can then write
![]() $T=iT$
and
$T=iT$
and
![]() $T^*T=T^*(i^*i)T$
.
$T^*T=T^*(i^*i)T$
.
The operator
![]() $i^*i: A^2_\gamma \to A^2_\gamma $
is positive. With respect to the monomial orthonormal basis
$i^*i: A^2_\gamma \to A^2_\gamma $
is positive. With respect to the monomial orthonormal basis
![]() $\{e_n=c_nz^n\}$
of
$\{e_n=c_nz^n\}$
of
![]() $A^2_\gamma $
from Section 2, the operator
$A^2_\gamma $
from Section 2, the operator
![]() $i^*i$
is diagonal with the corresponding eigenvalues given by
$i^*i$
is diagonal with the corresponding eigenvalues given by
 $$ \begin{align*}\langle i^*ie_n, e_n\rangle_{A^2_\gamma}=c_n^2\langle z^n, z^n\rangle_{A^2_\alpha} =\frac{\Gamma(n+2+\gamma)}{n!\,\Gamma(2+\gamma)}\,\frac{n!\,\Gamma(2+\alpha)}{\Gamma(n+2+\alpha)} \sim\frac1{(n+1)^{\alpha-\gamma}},\end{align*} $$
$$ \begin{align*}\langle i^*ie_n, e_n\rangle_{A^2_\gamma}=c_n^2\langle z^n, z^n\rangle_{A^2_\alpha} =\frac{\Gamma(n+2+\gamma)}{n!\,\Gamma(2+\gamma)}\,\frac{n!\,\Gamma(2+\alpha)}{\Gamma(n+2+\alpha)} \sim\frac1{(n+1)^{\alpha-\gamma}},\end{align*} $$
as
![]() $n\to \infty $
. This shows that
$n\to \infty $
. This shows that
![]() $i^*i$
belongs to the Schatten class
$i^*i$
belongs to the Schatten class
![]() $S_p$
of
$S_p$
of
![]() $A^2_\gamma $
for all p with
$A^2_\gamma $
for all p with
![]() $p(\alpha -\gamma )>1$
. Thus, T belongs to the Schatten class
$p(\alpha -\gamma )>1$
. Thus, T belongs to the Schatten class
![]() $S_p$
of
$S_p$
of
![]() $A^2_\alpha $
whenever
$A^2_\alpha $
whenever
![]() $p>2/(\alpha -\gamma )$
.
$p>2/(\alpha -\gamma )$
.
Note that the result above remains true even if the parameters
![]() $\alpha $
and
$\alpha $
and
![]() $\gamma $
fall below
$\gamma $
fall below
![]() $-1$
, although the proof needs to be modified. Details are omitted. We now prove the main results of this section in the next two theorems.
$-1$
, although the proof needs to be modified. Details are omitted. We now prove the main results of this section in the next two theorems.
Recall that
are the defect operators, and
Theorem 4.5 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
$\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
-
(a) The defect operator
 $D^\alpha _\varphi $
is compact on
$D^\alpha _\varphi $
is compact on
 $A^2_\alpha $
.
$A^2_\alpha $
. -
(b) The function
 $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product. -
(c) The space
 $H^\alpha (\varphi )$
equals
$H^\alpha (\varphi )$
equals
 $A^2_{\alpha -1}$
.
$A^2_{\alpha -1}$
. -
(d) The space
 $H^\alpha (\varphi )$
is contained in
$H^\alpha (\varphi )$
is contained in
 $A^2_{\alpha -1}$
.
$A^2_{\alpha -1}$
.
Proof To prove (a) implies (b), we consider the normalized reproducing kernels
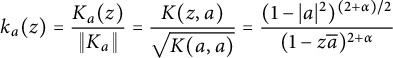 $$ \begin{align*}k_a(z)=\frac{K_a(z)}{\|K_a\|}=\frac{K(z,a)}{\sqrt{K(a,a)}}=\frac{(1-|a|^2)^{(2+\alpha)/2}}{(1-z\overline a)^{2+\alpha}}\end{align*} $$
$$ \begin{align*}k_a(z)=\frac{K_a(z)}{\|K_a\|}=\frac{K(z,a)}{\sqrt{K(a,a)}}=\frac{(1-|a|^2)^{(2+\alpha)/2}}{(1-z\overline a)^{2+\alpha}}\end{align*} $$
for
![]() $A^2_\alpha $
. It is easy to see that
$A^2_\alpha $
. It is easy to see that
![]() $k_a\to 0$
weakly in
$k_a\to 0$
weakly in
![]() $A^2_\alpha $
as
$A^2_\alpha $
as
![]() $|a|\to 1^-$
. If
$|a|\to 1^-$
. If
![]() $D^\alpha _\varphi $
is compact, then so is
$D^\alpha _\varphi $
is compact, then so is
![]() $E^\alpha _\varphi $
, which implies that
$E^\alpha _\varphi $
, which implies that
![]() $\langle E^\alpha _\varphi k_a, k_a\rangle \to 0$
as
$\langle E^\alpha _\varphi k_a, k_a\rangle \to 0$
as
![]() $|a|\to 1^-$
. It is easy to see that
$|a|\to 1^-$
. It is easy to see that
![]() $T^*_\varphi k_a=\overline {\varphi (a)}\,k_a$
, so we have
$T^*_\varphi k_a=\overline {\varphi (a)}\,k_a$
, so we have
Thus, the compactness of
![]() $D^\alpha _\varphi $
implies
$D^\alpha _\varphi $
implies
![]() $1-|\varphi (a)|^2\to 0$
as
$1-|\varphi (a)|^2\to 0$
as
![]() $|a|\to 1^-$
, which, according to Lemma 4.3, shows that
$|a|\to 1^-$
, which, according to Lemma 4.3, shows that
![]() $\varphi $
is a finite Blaschke product. This proves (a) implies (b).
$\varphi $
is a finite Blaschke product. This proves (a) implies (b).
Lemma 4.2 states that (b) implies (c). It is trivial that (c) implies (d). It follows from Lemma 4.4 that (d) implies (a). This completes the proof of the theorem.
Theorem 4.6 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
$\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
-
(a) The defect operator
 $D^\alpha _{\overline \varphi }$
is compact on
$D^\alpha _{\overline \varphi }$
is compact on
 $A^2_\alpha $
.
$A^2_\alpha $
. -
(b) The function
 $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product. -
(c) The space
 $H^\alpha (\overline \varphi )$
equals
$H^\alpha (\overline \varphi )$
equals
 $A^2_{\alpha -1}$
.
$A^2_{\alpha -1}$
. -
(d) The space
 $H^\alpha (\overline \varphi )$
is contained in
$H^\alpha (\overline \varphi )$
is contained in
 $A^2_{\alpha -1}$
.
$A^2_{\alpha -1}$
.
Proof First, assume that condition (a) holds. Taking the square of
![]() $D^\alpha _{\overline \varphi }$
, we see that the Toeplitz operator
$D^\alpha _{\overline \varphi }$
, we see that the Toeplitz operator
![]() $T_{1-|\varphi |^2}$
(with nonnegative symbol) is compact on
$T_{1-|\varphi |^2}$
(with nonnegative symbol) is compact on
![]() $A^2_\alpha $
. It follows from Corollary 7.9 of [Reference Zhu27] that for any positive
$A^2_\alpha $
. It follows from Corollary 7.9 of [Reference Zhu27] that for any positive
![]() $r>0$
, we have
$r>0$
, we have
where
![]() $D(a,r)=\{z\in \mathbb {D}: \beta (z,a)<r\}$
is the Bergman metric ball with center a and radius r, and
$D(a,r)=\{z\in \mathbb {D}: \beta (z,a)<r\}$
is the Bergman metric ball with center a and radius r, and
![]() $A_\alpha (D(a,r))$
is the
$A_\alpha (D(a,r))$
is the
![]() $dA_\alpha $
measure of
$dA_\alpha $
measure of
![]() $D(a,r)$
. Equivalently,
$D(a,r)$
. Equivalently,
We claim that this implies
![]() $|\varphi (z)|^2\to 1$
uniformly as
$|\varphi (z)|^2\to 1$
uniformly as
![]() $|z|\to 1^-$
. In fact, if this conclusion is not true, then there exist a constant
$|z|\to 1^-$
. In fact, if this conclusion is not true, then there exist a constant
![]() $\sigma \in (0,1)$
and a sequence
$\sigma \in (0,1)$
and a sequence
![]() $\{a_n\}$
in
$\{a_n\}$
in
![]() $\mathbb {D}$
such that
$\mathbb {D}$
such that
![]() $|a_n|\to 1$
as
$|a_n|\to 1$
as
![]() $n\to \infty $
and
$n\to \infty $
and
![]() $|\varphi (a_n)|<\sigma $
for all
$|\varphi (a_n)|<\sigma $
for all
![]() $n\ge 1$
.
$n\ge 1$
.
If
![]() $z\in D(a_n, r)$
, then by Theorem 5.5 of [Reference Zhu27],
$z\in D(a_n, r)$
, then by Theorem 5.5 of [Reference Zhu27],
where
is Bloch seminorm of
![]() $\varphi $
(recall that every function in
$\varphi $
(recall that every function in
![]() $H^\infty $
belongs to the Bloch space). If we use a sufficiently small radius r such that the constant
$H^\infty $
belongs to the Bloch space). If we use a sufficiently small radius r such that the constant
![]() $\delta =\|\varphi \|_{\mathcal {B}}r+\sigma <1$
, then
$\delta =\|\varphi \|_{\mathcal {B}}r+\sigma <1$
, then
for all
![]() $n\ge 1$
. This is a contradiction to (4.1).
$n\ge 1$
. This is a contradiction to (4.1).
Thus, we must have
![]() $|\varphi (z)|^2\to 1$
uniformly as
$|\varphi (z)|^2\to 1$
uniformly as
![]() $|z|\to 1^-$
. By Lemma 4.3,
$|z|\to 1^-$
. By Lemma 4.3,
![]() $\varphi $
is a finite Blaschke product. This proves that (a) implies (b).
$\varphi $
is a finite Blaschke product. This proves that (a) implies (b).
It follows from Lemma 4.2 that (b) implies (c). It is trivial that (c) implies (d). That (d) implies (a) follows from Lemma 4.4.
It follows from the proof of the theorem above that, for
![]() $\alpha>-1$
,
$\alpha>-1$
,
![]() $k>0$
, and
$k>0$
, and
![]() $\varphi \in H^\infty _1$
, the Toeplitz operator
$\varphi \in H^\infty _1$
, the Toeplitz operator
![]() $T_{(1-|\varphi |^2)^k}$
is compact on
$T_{(1-|\varphi |^2)^k}$
is compact on
![]() $A^2_\alpha $
if and only if
$A^2_\alpha $
if and only if
![]() $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product.
5 The range of
 $I-T_\varphi T_{\varphi }^*$
and
$I-T_\varphi T_{\varphi }^*$
and
 $I-T_{\varphi }^*T_\varphi $
$I-T_{\varphi }^*T_\varphi $
In this section, we study the range of the operators
![]() $E^\alpha _\varphi $
and
$E^\alpha _\varphi $
and
![]() $E^\alpha _{\overline \varphi }$
. The special case
$E^\alpha _{\overline \varphi }$
. The special case
![]() $\alpha =0$
was considered in [Reference Zhu26]. It is clear that
$\alpha =0$
was considered in [Reference Zhu26]. It is clear that
![]() $D^\alpha _\varphi $
is compact on
$D^\alpha _\varphi $
is compact on
![]() $A^2_\alpha $
if and only if
$A^2_\alpha $
if and only if
![]() $E^\alpha _\varphi $
is compact on
$E^\alpha _\varphi $
is compact on
![]() $A^2_\alpha $
. Similarly,
$A^2_\alpha $
. Similarly,
![]() $D^\alpha _{\overline \varphi }$
is compact on
$D^\alpha _{\overline \varphi }$
is compact on
![]() $A^2_\alpha $
if and only if
$A^2_\alpha $
if and only if
![]() $E^\alpha _{\overline \varphi }$
is compact on
$E^\alpha _{\overline \varphi }$
is compact on
![]() $A^2_\alpha $
.
$A^2_\alpha $
.
Proposition 5.1 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi $
is a finite Blaschke product. Then
$\varphi $
is a finite Blaschke product. Then
 $$ \begin{align} A^2_{\alpha-1}&=\left\{f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,g(w)\,dA_\alpha(w): g\in A^2_{\alpha+1}\right\} \end{align} $$
$$ \begin{align} A^2_{\alpha-1}&=\left\{f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,g(w)\,dA_\alpha(w): g\in A^2_{\alpha+1}\right\} \end{align} $$
 $$ \begin{align} &=\left\{f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,g(w)\,dA_\alpha(w): g\in L^2(\mathbb{D}, dA_{\alpha+1})\right\}. \end{align} $$
$$ \begin{align} &=\left\{f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,g(w)\,dA_\alpha(w): g\in L^2(\mathbb{D}, dA_{\alpha+1})\right\}. \end{align} $$
Proof Let
and let
![]() $A^2_{\varphi ,\alpha }$
denote the space of analytic functions in
$A^2_{\varphi ,\alpha }$
denote the space of analytic functions in
![]() $L^2(\mathbb {D}, dA_{\varphi ,\alpha })$
. It follows from Lemma 4.3 that
$L^2(\mathbb {D}, dA_{\varphi ,\alpha })$
. It follows from Lemma 4.3 that
with equivalent norms. Consider the integral operator
![]() $S_\varphi : A^2_{\varphi ,\alpha }\to A^2_\alpha $
defined by
$S_\varphi : A^2_{\varphi ,\alpha }\to A^2_\alpha $
defined by
 $$ \begin{align} S_\varphi f(z)=P_\alpha[(1-|\varphi|^2)f](z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w), \end{align} $$
$$ \begin{align} S_\varphi f(z)=P_\alpha[(1-|\varphi|^2)f](z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w), \end{align} $$
where
![]() $P_\alpha : L^2(\mathbb {D}, dA_\alpha )\to A^2_\alpha $
is the orthogonal projection. It is clear that
$P_\alpha : L^2(\mathbb {D}, dA_\alpha )\to A^2_\alpha $
is the orthogonal projection. It is clear that
![]() $S_\varphi $
is simply the operator
$S_\varphi $
is simply the operator
![]() $E^\alpha _{\overline \varphi }$
with its domain extended to the larger space
$E^\alpha _{\overline \varphi }$
with its domain extended to the larger space
![]() $A^2_{\varphi ,\alpha }$
.
$A^2_{\varphi ,\alpha }$
.
Now, the first desired equality (5.1) follows from the proof of Proposition 3.5 in [Reference Zhu25], word by word, together with the fact that
![]() $H^\alpha (\overline \varphi )=A^2_{\alpha -1}$
from the previous section. The second equality (5.2) follows from the same argument by replacing the operator
$H^\alpha (\overline \varphi )=A^2_{\alpha -1}$
from the previous section. The second equality (5.2) follows from the same argument by replacing the operator
![]() $S_\varphi $
above by its extension
$S_\varphi $
above by its extension
![]() $S_\varphi : L^2(\mathbb {D}, dA_{\varphi ,\alpha })\to A^2_\alpha $
, still defined by (5.3). We leave the details to the interested reader but summarize the main points of this omitted argument as follows.
$S_\varphi : L^2(\mathbb {D}, dA_{\varphi ,\alpha })\to A^2_\alpha $
, still defined by (5.3). We leave the details to the interested reader but summarize the main points of this omitted argument as follows.
For both
the adjoint
![]() $S_\varphi ^*$
is simply the inclusion, the image H of
$S_\varphi ^*$
is simply the inclusion, the image H of
![]() $S_\varphi $
is a reproducing kernel Hilbert space with the inner product
$S_\varphi $
is a reproducing kernel Hilbert space with the inner product
and the reproducing kernel of H at w is
![]() $S_\varphi S_\varphi ^*K_w ^\alpha $
, where
$S_\varphi S_\varphi ^*K_w ^\alpha $
, where
![]() $K^\alpha _w$
is the reproducing kernel of
$K^\alpha _w$
is the reproducing kernel of
![]() $A^2_\alpha $
at w. Consequently, the reproducing kernel of H is given by
$A^2_\alpha $
at w. Consequently, the reproducing kernel of H is given by
 $$ \begin{align*}S_\varphi K_w^\alpha(z)=\int_{\mathbb{D}}\frac{1-|\varphi(u)|^2}{(1-z\overline u)^{2+\alpha} (1-u\overline w)^{2+\alpha}}\,dA_\alpha(u),\end{align*} $$
$$ \begin{align*}S_\varphi K_w^\alpha(z)=\int_{\mathbb{D}}\frac{1-|\varphi(u)|^2}{(1-z\overline u)^{2+\alpha} (1-u\overline w)^{2+\alpha}}\,dA_\alpha(u),\end{align*} $$
which coincides with the reproducing kernel of
![]() $H^\alpha (\overline \varphi )$
. By uniqueness of the reproducing kernel, we must have
$H^\alpha (\overline \varphi )$
. By uniqueness of the reproducing kernel, we must have
![]() $H=H^\alpha (\overline \varphi ) =A^2_{\alpha -1}$
, which yields the desired representations in (5.1) and (5.2).
$H=H^\alpha (\overline \varphi ) =A^2_{\alpha -1}$
, which yields the desired representations in (5.1) and (5.2).
Lemma 5.2 If
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi $
is a finite Blaschke product, then
$\varphi $
is a finite Blaschke product, then
Proof As a Toeplitz operator on
![]() $A^2_\alpha $
, we can write
$A^2_\alpha $
, we can write
 $$ \begin{align*}E^\alpha_{\overline\varphi}f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w), \qquad f\in A^2_\alpha.\end{align*} $$
$$ \begin{align*}E^\alpha_{\overline\varphi}f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w), \qquad f\in A^2_\alpha.\end{align*} $$
It follows that
 $$ \begin{align*}(E^\alpha_{\overline\varphi}f)'(z)=\int_{\mathbb{D}}\frac{\Phi(w)}{(1-z\overline w)^{3+\alpha}}\,f(w)\,dA_{\alpha+1}(w) =P_{\alpha+1}(\Phi f)(z),\end{align*} $$
$$ \begin{align*}(E^\alpha_{\overline\varphi}f)'(z)=\int_{\mathbb{D}}\frac{\Phi(w)}{(1-z\overline w)^{3+\alpha}}\,f(w)\,dA_{\alpha+1}(w) =P_{\alpha+1}(\Phi f)(z),\end{align*} $$
where
![]() $P_{\alpha +1}: L^2(\mathbb {D}, dA_{\alpha +1})\to A^2_{\alpha +1}$
is the orthogonal projection and
$P_{\alpha +1}: L^2(\mathbb {D}, dA_{\alpha +1})\to A^2_{\alpha +1}$
is the orthogonal projection and
 $$ \begin{align*}\Phi(w)=\frac{(\alpha+1)\overline w(1-|\varphi(w)|^2)}{1-|w|^2}.\end{align*} $$
$$ \begin{align*}\Phi(w)=\frac{(\alpha+1)\overline w(1-|\varphi(w)|^2)}{1-|w|^2}.\end{align*} $$
By Lemma 4.3,
![]() $\Phi \in L^\infty (\mathbb {D})$
. It follows from Theorem 3.11 of [Reference Zhu27] that
$\Phi \in L^\infty (\mathbb {D})$
. It follows from Theorem 3.11 of [Reference Zhu27] that
![]() $P_{\alpha +1}$
maps
$P_{\alpha +1}$
maps
![]() $L^2(\mathbb {D}, dA_\alpha )$
boundedly to
$L^2(\mathbb {D}, dA_\alpha )$
boundedly to
![]() $A^2_\alpha $
. Therefore,
$A^2_\alpha $
. Therefore,
![]() $f\in A^2_\alpha $
implies
$f\in A^2_\alpha $
implies
![]() $(E^\alpha _{\overline \varphi }f)'\in A^2_{\alpha }$
, which is clearly equivalent to
$(E^\alpha _{\overline \varphi }f)'\in A^2_{\alpha }$
, which is clearly equivalent to
![]() $E^\alpha _{\overline \varphi }f \in A^2_{\alpha -2}$
. This proves that
$E^\alpha _{\overline \varphi }f \in A^2_{\alpha -2}$
. This proves that
![]() $E^\alpha _{\overline \varphi }$
maps
$E^\alpha _{\overline \varphi }$
maps
![]() $A^2_\alpha $
into
$A^2_\alpha $
into
![]() $A^2_{\alpha -2}$
.
$A^2_{\alpha -2}$
.
To show that the mapping
![]() $E^\alpha _{\overline \varphi }: A^2_\alpha \to A^2_{\alpha -2}$
is onto, we switch from the ordinary derivative
$E^\alpha _{\overline \varphi }: A^2_\alpha \to A^2_{\alpha -2}$
is onto, we switch from the ordinary derivative
![]() $(E^\alpha _{\overline \varphi }f)'$
to a certain fractional radial differential operator R (
$(E^\alpha _{\overline \varphi }f)'$
to a certain fractional radial differential operator R (
![]() $=R^{2+\alpha ,1}$
using the notation from [Reference Zhao and Zhu24]) of order
$=R^{2+\alpha ,1}$
using the notation from [Reference Zhao and Zhu24]) of order
![]() $1$
:
$1$
:
 $$ \begin{align*}RE^\alpha_{\overline\varphi}f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{3+\alpha}}\,f(w)\,dA_\alpha(w).\end{align*} $$
$$ \begin{align*}RE^\alpha_{\overline\varphi}f(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{3+\alpha}}\,f(w)\,dA_\alpha(w).\end{align*} $$
It is still true that
![]() $E^\alpha _{\overline \varphi }f\in A^2_{\alpha -2}$
if and only if
$E^\alpha _{\overline \varphi }f\in A^2_{\alpha -2}$
if and only if
![]() $RE^\alpha _{\overline \varphi }f\in A^2_\alpha $
. See [Reference Zhao and Zhu24].
$RE^\alpha _{\overline \varphi }f\in A^2_\alpha $
. See [Reference Zhao and Zhu24].
Fix any function
![]() $g\in A^2_{\alpha -2}$
. Then the function
$g\in A^2_{\alpha -2}$
. Then the function
![]() $Rg$
belongs to
$Rg$
belongs to
![]() $A^2_\alpha $
. It follows from Proposition 5.1, with
$A^2_\alpha $
. It follows from Proposition 5.1, with
![]() $\alpha $
in (5.1) and (5.2) replaced by
$\alpha $
in (5.1) and (5.2) replaced by
![]() $\alpha +1$
, that there exists a function
$\alpha +1$
, that there exists a function
![]() $h\in L^2(\mathbb {D}, dA_{\alpha +2})$
such that
$h\in L^2(\mathbb {D}, dA_{\alpha +2})$
such that
 $$ \begin{align*}Rg(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{3+\alpha}}\,h(w)\,dA_{\alpha+1}(w).\end{align*} $$
$$ \begin{align*}Rg(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{3+\alpha}}\,h(w)\,dA_{\alpha+1}(w).\end{align*} $$
Applying the inverse of R to both sides, we obtain
 $$ \begin{align*}g(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,h(w)\,dA_{\alpha+1}(w).\end{align*} $$
$$ \begin{align*}g(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,h(w)\,dA_{\alpha+1}(w).\end{align*} $$
Let
![]() $\widetilde h(w)=(1-|w|^2)h(w)$
. Then
$\widetilde h(w)=(1-|w|^2)h(w)$
. Then
![]() $\widetilde h\in L^2(\mathbb {D}, dA_\alpha )$
and
$\widetilde h\in L^2(\mathbb {D}, dA_\alpha )$
and
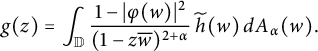 $$ \begin{align*}g(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,\widetilde h(w)\,dA_\alpha(w).\end{align*} $$
$$ \begin{align*}g(z)=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,\widetilde h(w)\,dA_\alpha(w).\end{align*} $$
By Proposition 5.1 again, there exists a function
![]() $f\in A^2_\alpha $
such that
$f\in A^2_\alpha $
such that
 $$ \begin{align*}g=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w),\end{align*} $$
$$ \begin{align*}g=\int_{\mathbb{D}}\frac{1-|\varphi(w)|^2}{(1-z\overline w)^{2+\alpha}}\,f(w)\,dA_\alpha(w),\end{align*} $$
or
![]() $g=E^\alpha _{\overline \varphi }f$
. Thus, we have shown that
$g=E^\alpha _{\overline \varphi }f$
. Thus, we have shown that
![]() $E^\alpha _{\overline \varphi }(A^2_\alpha ) =A^2_{\alpha -2}$
.
$E^\alpha _{\overline \varphi }(A^2_\alpha ) =A^2_{\alpha -2}$
.
Next, we show that
![]() $E^\alpha _\varphi (A^2_\alpha )=A^2_{\alpha -2}$
. Note that
$E^\alpha _\varphi (A^2_\alpha )=A^2_{\alpha -2}$
. Note that
![]() $T_\varphi $
is a Fredholm operator. So
$T_\varphi $
is a Fredholm operator. So
![]() $\varphi A^2_\alpha $
is closed in
$\varphi A^2_\alpha $
is closed in
![]() $A^2_\alpha $
,
$A^2_\alpha $
,
![]() $\ker (T_\varphi ^*) = A^2_\alpha \ominus \varphi A^2_\alpha $
, and
$\ker (T_\varphi ^*) = A^2_\alpha \ominus \varphi A^2_\alpha $
, and
![]() $A^2_\alpha = (A^2_\alpha \ominus \varphi A^2_\alpha )\oplus \varphi A^2_\alpha $
. Since
$A^2_\alpha = (A^2_\alpha \ominus \varphi A^2_\alpha )\oplus \varphi A^2_\alpha $
. Since
it follows that
Since
![]() $A^2_\alpha \ominus \varphi A^2_\alpha $
consists of the reproducing kernels or the derivative of the reproducing kernels in
$A^2_\alpha \ominus \varphi A^2_\alpha $
consists of the reproducing kernels or the derivative of the reproducing kernels in
![]() $A^2_\alpha $
, we have
$A^2_\alpha $
, we have
![]() $A^2_\alpha \ominus \varphi A^2_\alpha \subseteq A^2_{\alpha -2}$
. Also,
$A^2_\alpha \ominus \varphi A^2_\alpha \subseteq A^2_{\alpha -2}$
. Also,
Thus, we obtain that
completing the proof of the lemma.
We can now prove the main result of this section, namely, the next two theorems.
Theorem 5.3 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
$\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
-
(a) The operator
 $E^\alpha _{\varphi }$
is compact on
$E^\alpha _{\varphi }$
is compact on
 $A^2_\alpha $
.
$A^2_\alpha $
. -
(b) The function
 $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product. -
(c) The range of
 $E^\alpha _\varphi $
equals
$E^\alpha _\varphi $
equals
 $A^2_{\alpha -2}$
.
$A^2_{\alpha -2}$
. -
(d) The range of
 $E^\alpha _\varphi $
is contained in
$E^\alpha _\varphi $
is contained in
 $A^2_{\alpha -2}$
.
$A^2_{\alpha -2}$
.
Proof Since
![]() $E^\alpha _\varphi =(D^\alpha _\varphi )^2$
, the operator
$E^\alpha _\varphi =(D^\alpha _\varphi )^2$
, the operator
![]() $E^\alpha _\varphi $
is compact if and only if
$E^\alpha _\varphi $
is compact if and only if
![]() $D^\alpha _\varphi $
is compact. Thus, the equivalence of (a) and (b) follows from Theorem 4.5.
$D^\alpha _\varphi $
is compact. Thus, the equivalence of (a) and (b) follows from Theorem 4.5.
Lemma 5.2 shows that (b) implies (c). It is trivial that (c) implies (d). Finally, that (d) implies (a) follows from Lemma 4.4.
Theorem 5.4 Suppose
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
$\varphi \in H^\infty _1$
. Then the following conditions are equivalent.
-
(a) The operator
 $E^\alpha _{\overline \varphi }$
is compact on
$E^\alpha _{\overline \varphi }$
is compact on
 $A^2_\alpha $
.
$A^2_\alpha $
. -
(b) The function
 $\varphi $
is a finite Blaschke product.
$\varphi $
is a finite Blaschke product. -
(c) The range of
 $E^\alpha _{\overline \varphi }$
equals
$E^\alpha _{\overline \varphi }$
equals
 $A^2_{\alpha -2}$
.
$A^2_{\alpha -2}$
. -
(d) The range of
 $E^\alpha _{\overline \varphi }$
is contained in
$E^\alpha _{\overline \varphi }$
is contained in
 $A^2_{\alpha -2}$
.
$A^2_{\alpha -2}$
.
Proof It is similar to the proof of Theorem 5.3.
Finally, we note that the main results of this and the previous section cannot be extended to the Hardy space
![]() $H^2$
(the case
$H^2$
(the case
![]() $\alpha =-1$
). For example, in this case, if
$\alpha =-1$
). For example, in this case, if
![]() $\varphi (z)=z$
, then
$\varphi (z)=z$
, then
![]() $I-T_{\overline \varphi }T_{\varphi }=0$
and
$I-T_{\overline \varphi }T_{\varphi }=0$
and
![]() $I-T_\varphi T_{\overline \varphi }$
is a rank-one operator. More generally, if
$I-T_\varphi T_{\overline \varphi }$
is a rank-one operator. More generally, if
![]() $\varphi $
is any inner function, then
$\varphi $
is any inner function, then
![]() $I-T_{\overline \varphi }T_\varphi =0$
.
$I-T_{\overline \varphi }T_\varphi =0$
.
Acknowledgment
We would like to thank Michael Hartz for permitting us to include his proof of Theorem 3.6.