Published online by Cambridge University Press: 01 February 2016
We define and study quasidialectical systems, which are an extension of Magari’s dialectical systems, designed to make Magari’s formalization of trial and error mathematics more adherent to the real mathematical practice of revision: our proposed extension follows, and in several regards makes more precise, varieties of empiricist positions à la Lakatos. We prove several properties of quasidialectical systems and of the sets that they represent, called quasidialectical sets. In particular, we prove that the quasidialectical sets are 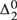 ${\rm{\Delta }}_2^0$ sets in the arithmetical hierarchy. We distinguish between “loopless” quasidialectal systems, and quasidialectical systems “with loops”. The latter ones represent exactly those coinfinite c.e. sets, that are not simple. In a subsequent paper we will show that whereas the dialectical sets are ω-c.e., the quasidialectical sets spread out throughout all classes of the Ershov hierarchy of the
${\rm{\Delta }}_2^0$ sets in the arithmetical hierarchy. We distinguish between “loopless” quasidialectal systems, and quasidialectical systems “with loops”. The latter ones represent exactly those coinfinite c.e. sets, that are not simple. In a subsequent paper we will show that whereas the dialectical sets are ω-c.e., the quasidialectical sets spread out throughout all classes of the Ershov hierarchy of the  ${\rm{\Delta }}_2^0$ sets.
${\rm{\Delta }}_2^0$ sets.