No CrossRef data available.
Published online by Cambridge University Press: 15 April 2004
In this paper we study the existence of subharmonic solutions of the Hamiltonian system $$ J\dot x+ u^* \nabla G(t,u(x)) =e(t)$$ 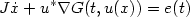 where u is a linear map, G is a C 1-function and e is a continuous function.
where u is a linear map, G is a C 1-function and e is a continuous function.