Published online by Cambridge University Press: 23 December 2015
We establish that the intrinsic distance dE associated with an indecomposable plane set E of finite perimeter is infinitesimally Euclidean; namely,
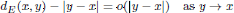 in E. By this result, we prove through a standard argument that a conservative vector field in a plane set of finite perimeter has a potential. We also provide some applications to complex analysis. Moreover, we present a collection of results that would seem to suggest the possibility of developing a De Rham cohomology theory for integral currents.
in E. By this result, we prove through a standard argument that a conservative vector field in a plane set of finite perimeter has a potential. We also provide some applications to complex analysis. Moreover, we present a collection of results that would seem to suggest the possibility of developing a De Rham cohomology theory for integral currents.