Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cozzi, Matteo
Dávila, Juan
and
del Pino, Manuel
2020.
Long-time asymptotics for evolutionary crystal dislocation models.
Advances in Mathematics,
Vol. 371,
Issue. ,
p.
107242.
Sun, Liming
Wei, Jun-cheng
and
Zhang, Qidi
2022.
Bubble towers in the ancient solution of energy-critical heat equation.
Calculus of Variations and Partial Differential Equations,
Vol. 61,
Issue. 6,
Liu, Chao
and
Yang, Jun
2023.
Solutions with single radial interface of the generalized Cahn–Hilliard flow.
Calculus of Variations and Partial Differential Equations,
Vol. 62,
Issue. 4,
Liu, Chao
and
Yang, Jun
2024.
Solutions with multiple interfaces to the generalized parabolic Cahn–Hilliard equation in one and three space dimensions.
Journal of the London Mathematical Society,
Vol. 109,
Issue. 6,
The Nguyen, Huy
and
Wang, Shengwen
2024.
Second-Order Estimates for Transition Layers and a Curvature Estimate for the Parabolic Allen–Cahn.
International Mathematics Research Notices,
Vol. 2024,
Issue. 8,
p.
6759.
Taniguchi, Masaharu
2024.
Entire solutions with and without radial symmetry in balanced bistable reaction–diffusion equations.
Mathematische Annalen,
Vol. 390,
Issue. 3,
p.
3931.
Gkikas, Konstantinos T
2025.
Ancient Solutions to the Allen–Cahn Equation in Catenoids.
Asymptotic Analysis,
Taniguchi, Masaharu
2025.
Polyhedral entire solutions in reaction-diffusion equations.
Journal of Differential Equations,
Vol. 429,
Issue. ,
p.
529.

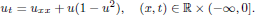
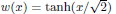 connects, as a ‘transition layer’, the stable phases –1 and +1. We construct a solution
connects, as a ‘transition layer’, the stable phases –1 and +1. We construct a solution