No CrossRef data available.
Study of a singular equation set in the half-space
Published online by Cambridge University Press: 20 September 2012
Abstract
This work is dedicated to the resolution of a singular equation set in the half-space, with a diffusion coefficient that blows up on the boundary. More precisely, for a datum g: ℝ+3 → ℝ, our problem involves seeking u: ℝ+3 → ℝ as a formal solution to
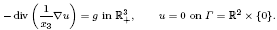
We give existence and uniqueness results of weak and strong solutions in suitable weighted spaces, where the weight depends on x3.
Information
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 142 , Issue 5 , October 2012 , pp. 897 - 915
- Copyright
- Copyright © Royal Society of Edinburgh 2012

