Article contents
Prescribing nearly constant curvatures on balls
Published online by Cambridge University Press: 27 October 2023
Abstract
In this paper, we address two boundary cases of the classical Kazdan–Warner problem. More precisely, we consider the problem of prescribing the Gaussian and boundary geodesic curvature on a disk of $\mathbb {R}^2$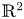 , and the scalar and mean curvature on a ball in higher dimensions, via a conformal change of the metric. We deal with the case of negative interior curvature and positive boundary curvature. Using a Ljapunov–Schmidt procedure, we obtain new existence results when the prescribed functions are close to constants.
, and the scalar and mean curvature on a ball in higher dimensions, via a conformal change of the metric. We deal with the case of negative interior curvature and positive boundary curvature. Using a Ljapunov–Schmidt procedure, we obtain new existence results when the prescribed functions are close to constants.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh
References
- 2
- Cited by



