Article contents
7.—Elliptic Singular Perturbations of First-order Operators with Critical Points
Published online by Cambridge University Press: 14 February 2012
Synopsis
We study the asymptotic behaviour for ɛ→+0 of the solution Φ of the elliptic boundary value problem
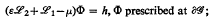
 is a bounded domain in ℝ2,
is a bounded domain in ℝ2, 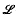 2 is asecond-order uniformly elliptic operator,
2 is asecond-order uniformly elliptic operator, 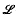 1 is a first-order operator, which has critical points in the interior of
1 is a first-order operator, which has critical points in the interior of  , i.e. points at which the coefficients of the first derivatives vanish, ɛ and μ are real parameters and h is a smooth function on
, i.e. points at which the coefficients of the first derivatives vanish, ɛ and μ are real parameters and h is a smooth function on  . We construct firstorder approximations to Φ for all types of nondegenerate critical points of
. We construct firstorder approximations to Φ for all types of nondegenerate critical points of 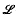 1 and prove their validity under some restriction on the range of μ.
1 and prove their validity under some restriction on the range of μ.
In a number of cases we get internal layers of nonuniformity (which extend to the boundary in the saddle-point case) near the critical points; this depends on the position of the characteristics of 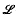 1 and their direction. At special values of the parameter μ outside the range in which we could prove validity we observe ‘resonance’, a sudden displacement of boundary layers; these points are connected with the spectrum of the operator ɛ
1 and their direction. At special values of the parameter μ outside the range in which we could prove validity we observe ‘resonance’, a sudden displacement of boundary layers; these points are connected with the spectrum of the operator ɛ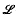 2 +
2 + 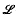 1 subject to boundary conditions of Dirichlet type.
1 subject to boundary conditions of Dirichlet type.
Information
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 74 , 1976 , pp. 91 - 113
- Copyright
- Copyright © Royal Society of Edinburgh 1976
References
- 3
- Cited by

