No CrossRef data available.
Article contents
Upper and lower limit oscillation conditions for first-order difference equations
Published online by Cambridge University Press: 07 July 2022
Abstract
In this work, we consider the first-order difference equation with general argument\[ \varDelta x(n)+p(n)x\left( \tau (n)\right) =0,\quad n\geq 0, \]where $(p(n))$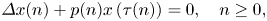
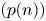 is a sequence of non-negative real numbers, $(\tau (n))$
is a sequence of non-negative real numbers, $(\tau (n))$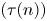 is a sequence of integers such that $\tau (n)< n$
is a sequence of integers such that $\tau (n)< n$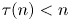 for$\ n\in \mathbf {N},\,$
for$\ n\in \mathbf {N},\,$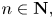 and$\ \lim _{n\rightarrow \infty }\tau (n)=\infty.$
and$\ \lim _{n\rightarrow \infty }\tau (n)=\infty.$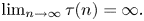 Under the assumption that the deviating argument is not necessarily monotone, we obtain some new oscillation conditions and improve the all known results for the above equation in the literature, involving only upper and only lower limit conditions. Two examples illustrating the results are also given.
Under the assumption that the deviating argument is not necessarily monotone, we obtain some new oscillation conditions and improve the all known results for the above equation in the literature, involving only upper and only lower limit conditions. Two examples illustrating the results are also given.
MSC classification
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 65 , Issue 3 , August 2022 , pp. 618 - 631
- Copyright
- Copyright © The Author(s), 2022. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society
References
Braverman, E. and Karpuz, B., On oscillation of differential and difference equations with non-monotone delays, Appl. Math. Comput. 218 (2011), 3880–3887.Google Scholar
Chatzarakis, G. E., Koplatadze, R. and Stavroulakis, I. P., Optimal oscillation criteria for first order difference equations with delay argument, Pacific J. Math. 235 (2008), 15–33.CrossRefGoogle Scholar
Chatzarakis, G. E., Koplatadze, R. and Stavroulakis, I. P., Oscillation criteria of first order linear difference equations with delay argument, Nonlinear Anal. 68 (2008), 994–1005.CrossRefGoogle Scholar
Chatzarakis, G. E., Philos, Ch. G. and Stavroulakis, I. P., On the oscillation of the solutions to linear difference equations with variable delay, Electron. J. Differ. Equ. 50 (2008), 1–5.Google Scholar
Chatzarakis, G. E., Philos, Ch. G. and Stavroulakis, I. P., An oscillation criterion for linear difference equations with general delay argument, Portugal. Math. (N.S.) 66 (4) (2009), 513–533.CrossRefGoogle Scholar
Chen, M. P. and Yu, J. S., Oscillations of delay difference equations with variable coefficients, in Proceedings of the First International Conference on Difference Equations, pp. 105–114 (Gordon and Breach, London, 1994).Google Scholar
Erbe, L. H. and Zhang, B. G., Oscillation of discrete analogues of delay equations, Differ. Int. Equ. 2 (1989), 300–309.Google Scholar
Erbe, L. H., Kong, Q. and Zhang, B. G., Oscillation theory for functional differential equations, (Marcel Dekker, New York, 1995).Google Scholar
Györi, I. and Ladas, G., Linearized oscillations for equations with piecewise constant arguments, Differ. Int. Equ. 2 (1989), 123–131.Google Scholar
Györi, I. and Ladas, G., Oscillation Theory of Delay Differential Equations with Applications (Oxford, Clarendon Press, 1991).Google Scholar
Ladas, G., Explicit conditions for the oscillation of difference equations, J. Math. Anal. Appl 153 (1990), 276–287.CrossRefGoogle Scholar
Ladas, G., Philos, Ch. G. and Sficas, Y. G., Sharp conditions for the oscillation of delay difference equations, J. Appl. Math. Simul. 2 (1989), 101–111.CrossRefGoogle Scholar
Ladde, G. S., Lakshmikantham, V. and Zhang, B. G., Oscillation theory of differential equations with deviating arguments (New York, Marcel Dekker, 1987).Google Scholar
Philos, Ch. G., On oscillations of some difference equations, Funkcial. Ekvac. 34 (1991), 157–172.Google Scholar
Öcalan, Ö., An improved oscillation criterion for first order difference equations, Bull. Math. Soc. Sci. Math. Roumanie (N.S.) 59 (107) (2016), 65–73.Google Scholar
Öcalan, Ö., Oscillation of first-order dynamic equations with nonmonotone delay, Math. Methods Appl. Sci. 43 (2020), 3954–3964.Google Scholar
Yan, W., Meng, Q. and Yan, J., Oscillation criteria for difference equation of variable delays, Dyn. Contin. Discrete Impuls. Syst. Ser. A Math. Anal. 13A (2006), 641–647. Part 2, suppl.Google Scholar
Zhang, B. G. and Tian, C. J., Nonexistence and existence of positive solutions for difference equations with unbounded delay, Comput. Math. Appl. 36 (1998), 1–8.CrossRefGoogle Scholar
Zhang, B. G. and Tian, C. J., Oscillation criteria for difference equations with unbounded delay, Comput. Math. Appl. 35 (4) (1998), 19–26.CrossRefGoogle Scholar


