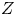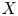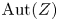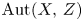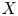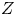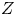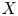Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jia, Jia
and
Meng, Sheng
2025.
Moishezon manifolds with no nef and big classes.
Proceedings of the Edinburgh Mathematical Society,
Vol. 68,
Issue. 1,
p.
198.