Published online by Cambridge University Press: 20 January 2009
1. The chief purpose of this paper is to demonstrate the existence of a plane quartic curve with eight undulations, an “undulation” being a point at which the tangent has four-point contact. It is shown that the curve
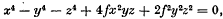
where (x, y, z) are homogeneous point-coordinates and f a constant, has undulations at the eight points

The curve has, in addition to these undulations, eight inflections which are; in general, distinct. But there are two geometrically different possibilities of their not being distinct, and in either instance they coincide in pairs at four further undulations. Thus two types of curve arise without any ordinary inflections at all, their 24 inflections coinciding in pairs at 12 undulations.
page 147 note 1 Rendiconto dell' accademia delle science Jisiche e matematiche, 21 (Naples, 1882) 45–69.Google Scholar
page 159 note 1 Math. Annalen, 17 (1880), 512.Google Scholar
page 160 note 1 Proc. Edinburgh Math. Soc. (2), 7 (1945), 154.Google Scholar