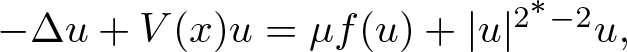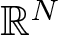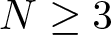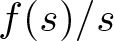Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Razani, Abdolrahman
Costa, Gustavo S. A.
and
Oliveira Junior, José C.
2025.
Multiplicity of solutions for a Kirchhoff equation with non‐linearity concave at the origin.
Mathematical Methods in the Applied Sciences,
Vol. 48,
Issue. 4,
p.
4195.
Costa, G. S. A.
and
Oliveira Junior, J. C.
2025.
Critical and supercritical problems influenced by the negative part of the spectrum.
Zeitschrift für angewandte Mathematik und Physik,
Vol. 76,
Issue. 2,
Rubim, César Augusto
and
Toon, Eduard
2025.
Functional Differential Equations and Dynamic Equations on Time Scales.
Vol. 52,
Issue. ,
p.
197.