Published online by Cambridge University Press: 20 January 2009
Let F = GF(q). To any polynomial G ∈ F[x] there is associated a mapping Ĝ on the set IF of monic irreducible polynomials over F. We present a natural and effective theory of the dynamics of Ĝ for the case in which G is a monic q-linearized polynomial. The main outcome is the following theorem.
Assume that G is not of the form 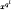 , where l ≥ 0 (in which event the dynamics is trivial). Then, for every integer n ≥ 1 and for every integer k ≥ 0, there exist infinitely many μ ∈ IF. having preperiod k and primitive period n with respect to Ĝ.
, where l ≥ 0 (in which event the dynamics is trivial). Then, for every integer n ≥ 1 and for every integer k ≥ 0, there exist infinitely many μ ∈ IF. having preperiod k and primitive period n with respect to Ĝ.
Previously, Morton, by somewhat different means, had studied the primitive periods of Ĝ when G = xq – ax, α a non-zero element of F. Our theorem extends and generalizes Morton's result. Moreover, it establishes a conjecture of Morton for the class of q-linearized polynomials.