No CrossRef data available.
Published online by Cambridge University Press: 05 June 2015
Given a finite field 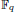 of q elements, we consider a trajectory of the map
of q elements, we consider a trajectory of the map  associated with a polynomial
associated with a polynomial 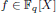 ]. Using bounds of character sums, under some mild condition on f, we show that for an appropriate constant C > 0 no N ⩾ Cq½ distinct consecutive elements of such a trajectory are contained in a small subgroup
]. Using bounds of character sums, under some mild condition on f, we show that for an appropriate constant C > 0 no N ⩾ Cq½ distinct consecutive elements of such a trajectory are contained in a small subgroup 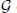 of
of 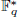 , improving the trivial lower bound
, improving the trivial lower bound 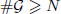 . Using a different technique, we also obtain a similar result for very small values of N. These results are multiplicative analogues of several recently obtained bounds on the length of intervals containing N distinct consecutive elements of such a trajectory.
. Using a different technique, we also obtain a similar result for very small values of N. These results are multiplicative analogues of several recently obtained bounds on the length of intervals containing N distinct consecutive elements of such a trajectory.