Article contents
2-subnormal quadratic offenders and Oliver's p-group conjecture
Published online by Cambridge University Press: 10 December 2012
Abstract
Bob Oliver conjectures that if p is an odd prime and S is a finite p-group, then the Oliver subgroup 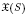 contains the Thompson subgroup Je(S). A positive resolution of this conjecture would give the existence and uniqueness of centric linking systems for fusion systems at odd primes. Using the ideas and work of Glauberman, we prove that if p ≥ 5, G is a finite p-group, and V is an elementary abelian p-group which is an F-module for G, then there exists a quadratic offender which is 2-subnormal (normal in its normal closure) in G. We apply this to show that Oliver's Conjecture holds provided that the quotient
contains the Thompson subgroup Je(S). A positive resolution of this conjecture would give the existence and uniqueness of centric linking systems for fusion systems at odd primes. Using the ideas and work of Glauberman, we prove that if p ≥ 5, G is a finite p-group, and V is an elementary abelian p-group which is an F-module for G, then there exists a quadratic offender which is 2-subnormal (normal in its normal closure) in G. We apply this to show that Oliver's Conjecture holds provided that the quotient 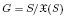 has class at most log2(p − 2) + 1, or p ≥ 5 and G is equal to its own Baumann subgroup.
has class at most log2(p − 2) + 1, or p ≥ 5 and G is equal to its own Baumann subgroup.
MSC classification
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 56 , Issue 1 , February 2013 , pp. 211 - 222
- Copyright
- Copyright © Edinburgh Mathematical Society 2012
References
- 4
- Cited by

