Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kochar, Subhash
and
Xu, Maochao
2008.
CORRECTION TO “STOCHASTIC COMPARISONS OF PARALLEL SYSTEMS WHEN COMPONENTS HAVE PROPORTIONAL HAZARD RATES”.
Probability in the Engineering and Informational Sciences,
Vol. 22,
Issue. 3,
p.
473.
Genest, Christian
Kochar, Subhash C.
and
Xu, Maochao
2009.
On the range of heterogeneous samples.
Journal of Multivariate Analysis,
Vol. 100,
Issue. 8,
p.
1587.
Zhao, Peng
Li, Xiaohu
and
Balakrishnan, N.
2009.
Likelihood ratio order of the second order statistic from independent heterogeneous exponential random variables.
Journal of Multivariate Analysis,
Vol. 100,
Issue. 5,
p.
952.
Kochar, Subhash
and
Xu, Maochao
2009.
Comparisons of Parallel Systems According to the Convex Transform Order.
Journal of Applied Probability,
Vol. 46,
Issue. 2,
p.
342.
Li, Xiaohu
Yan, Rongfang
and
Zuo, Ming J.
2009.
Evaluating a warm standby system with components having proportional hazard rates.
Operations Research Letters,
Vol. 37,
Issue. 1,
p.
56.
Kochar, Subhash
and
Xu, Maochao
2009.
Comparisons of Parallel Systems According to the Convex Transform Order.
Journal of Applied Probability,
Vol. 46,
Issue. 02,
p.
342.
Zhao, Peng
and
Li, Xiaohu
2009.
STOCHASTIC ORDER OF SAMPLE RANGE FROM HETEROGENEOUS EXPONENTIAL RANDOM VARIABLES.
Probability in the Engineering and Informational Sciences,
Vol. 23,
Issue. 1,
p.
17.
Zhao, Peng
and
Balakrishnan, N.
2009.
Characterization of MRL order of fail-safe systems with heterogeneous exponential components.
Journal of Statistical Planning and Inference,
Vol. 139,
Issue. 9,
p.
3027.
Mao, Tiantian
and
Hu, Taizhong
2010.
EQUIVALENT CHARACTERIZATIONS ON ORDERINGS OF ORDER STATISTICS AND SAMPLE RANGES.
Probability in the Engineering and Informational Sciences,
Vol. 24,
Issue. 2,
p.
245.
Da, Gaofeng
Ding, Weiyong
and
Li, Xiaohu
2010.
On hazard rate ordering of parallel systems with two independent components.
Journal of Statistical Planning and Inference,
Vol. 140,
Issue. 7,
p.
2148.
Zhao, Peng
and
Balakrishnan, N.
2011.
Some characterization results for parallel systems with two heterogeneous exponential components.
Statistics,
Vol. 45,
Issue. 6,
p.
593.
Zhao, Peng
Li, Xiaohu
and
Da, Gaofeng
2011.
Right Spread Order of the Second-Order Statistic from Heterogeneous Exponential Random Variables.
Communications in Statistics - Theory and Methods,
Vol. 40,
Issue. 17,
p.
3070.
Zhao, Peng
and
Balakrishnan, N.
2011.
Dispersive ordering of fail-safe systems with heterogeneous exponential components.
Metrika,
Vol. 74,
Issue. 2,
p.
203.
Kochar, Subhash
and
Xu, Maochao
2011.
On the Skewness of Order Statistics in Multiple-Outlier Models.
Journal of Applied Probability,
Vol. 48,
Issue. 01,
p.
271.
Kochar, Subhash
and
Xu, Maochao
2011.
Advances in Directional and Linear Statistics.
p.
113.
Zhao, Peng
2011.
ON PARALLEL SYSTEMS WITH HETEROGENEOUS GAMMA COMPONENTS.
Probability in the Engineering and Informational Sciences,
Vol. 25,
Issue. 3,
p.
369.
Kochar, Subhash
and
Xu, Maochao
2011.
On the Skewness of Order Statistics in Multiple-Outlier Models.
Journal of Applied Probability,
Vol. 48,
Issue. 1,
p.
271.
Zhao, Peng
and
Balakrishnan, N.
2011.
New results on comparisons of parallel systems with heterogeneous gamma components.
Statistics & Probability Letters,
Vol. 81,
Issue. 1,
p.
36.
Zhao, Peng
and
Balakrishnan, N.
2011.
MRL ordering of parallel systems with two heterogeneous components.
Journal of Statistical Planning and Inference,
Vol. 141,
Issue. 2,
p.
631.
Zhao, Peng
and
Zhang, Yiying
2012.
On sample ranges in multiple-outlier models.
Journal of Multivariate Analysis,
Vol. 111,
Issue. ,
p.
335.

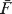
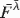 , where
, where 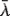 = ∑
= ∑