Article contents
On history-dependent mixed shock models
Published online by Cambridge University Press: 06 August 2021
Abstract
In this paper, we consider a history-dependent mixed shock model which is a combination of the history-dependent extreme shock model and the history-dependent 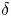 $\delta$-shock model. We assume that shocks occur according to the generalized Pólya process that contains the homogeneous Poisson process, the non-homogeneous Poisson process and the Pólya process as the particular cases. For the defined survival model, we derive the corresponding survival function, the mean lifetime and the failure rate. Further, we study the asymptotic and monotonicity properties of the failure rate. Finally, some applications of the proposed model have also been included with relevant numerical examples.
$\delta$-shock model. We assume that shocks occur according to the generalized Pólya process that contains the homogeneous Poisson process, the non-homogeneous Poisson process and the Pólya process as the particular cases. For the defined survival model, we derive the corresponding survival function, the mean lifetime and the failure rate. Further, we study the asymptotic and monotonicity properties of the failure rate. Finally, some applications of the proposed model have also been included with relevant numerical examples.
Keywords
Information
- Type
- Research Article
- Information
- Probability in the Engineering and Informational Sciences , Volume 36 , Issue 4 , October 2022 , pp. 1080 - 1097
- Copyright
- Copyright © The Author(s), 2021. Published by Cambridge University Press
References
- 8
- Cited by

