Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yin, Yunhui
and
Zhu, Peng
2019.
The streamline-diffusion finite element method on graded meshes for a convection–diffusion problem.
Applied Numerical Mathematics,
Vol. 138,
Issue. ,
p.
19.
Li, Hongping
and
Cheng, Lizheng
2021.
Particle Swarm Optimization algorithm for Solving Singular Perturbed Problemsation Problems on Layer Adaptive Mesh.
p.
466.
Zhou, Qin
2022.
Solving Two-dimensional Elliptic Singular Perturbation Problems by Particle Swarm Optimization Algorithm.
p.
627.

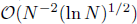 convergent rate in a discrete streamline-diffusion norm is established under certain regularity assumptions. Finally, through numerical experiments, we verified the theoretical results.
convergent rate in a discrete streamline-diffusion norm is established under certain regularity assumptions. Finally, through numerical experiments, we verified the theoretical results.