Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ohsawa, Takeo
2009.
A Reduction Theorem for Stable sets of Holomorphic Foliations on Complex Tori.
Nagoya Mathematical Journal,
Vol. 195,
Issue. ,
p.
41.
Ohsawa, Takeo
2012.
On the complement of effective divisors with semipositive normal bundle.
Kyoto Journal of Mathematics,
Vol. 52,
Issue. 3,
Ohsawa, Takeo
2014.
Classification of real analytic Levi flat hypersurfaces of 1-concave type in Hopf surfaces.
Kyoto Journal of Mathematics,
Vol. 54,
Issue. 3,
Adachi, Masanori
2015.
A local expression of the Diederich-Fornaess exponent and the exponent of conformal harmonic measures.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 46,
Issue. 1,
p.
65.
Ohsawa, Takeo
2015.
L² Approaches in Several Complex Variables.
p.
153.
Ohsawa, Takeo
2015.
L² Approaches in Several Complex Variables.
p.
1.
Ohsawa, Takeo
2015.
L² Approaches in Several Complex Variables.
p.
93.
Ohsawa, Takeo
2015.
L² Approaches in Several Complex Variables.
p.
127.
Ohsawa, Takeo
2015.
Complex Geometry and Dynamics.
Vol. 10,
Issue. ,
p.
211.
Ohsawa, Takeo
2015.
L² Approaches in Several Complex Variables.
p.
41.
Ohsawa, Takeo
2018.
Geometric Complex Analysis.
Vol. 246,
Issue. ,
p.
329.
Biard, Séverine
and
Iordan, Andrei
2020.
Nonexistence of Levi flat hypersurfaces with positive normal bundle in compact Kähler manifolds of dimension ≥3.
International Journal of Mathematics,
Vol. 31,
Issue. 01,
p.
2050004.
Adachi, Masanori
2022.
On weighted Bergman spaces of a domain with Levi-flat boundary II.
Complex Analysis and its Synergies,
Vol. 8,
Issue. 2,
Adachi, Masanori
and
Biard, Séverine
2022.
On Levi flat hypersurfaces with transversely affine foliation.
Mathematische Zeitschrift,
Vol. 301,
Issue. 1,
p.
373.
Shafikov, Rasul
2025.
Levi-Flats in $$\mathbb{C}\mathbb{P}^n$$: A Survey for Nonexperts.
The Journal of Geometric Analysis,
Vol. 35,
Issue. 6,

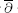 -equation, it is shown that compact Kähler manifolds of dimension ≥ 3 admit no Levi flat real analytic hypersurfaces whose complements are Stein.
-equation, it is shown that compact Kähler manifolds of dimension ≥ 3 admit no Levi flat real analytic hypersurfaces whose complements are Stein.