Article contents
Le Groupe GLn Tordu, Sur un Corps Fini
Published online by Cambridge University Press: 11 January 2016
Abstract
Let 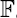 q be a finite field, G = GLn (
q be a finite field, G = GLn (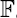 q), θ be the outer automorphism of G, suitably normalized. Consider the non-connected group G ⋊ {1, θ} and its connected component
q), θ be the outer automorphism of G, suitably normalized. Consider the non-connected group G ⋊ {1, θ} and its connected component  = Gθ. We know two ways to produce functions on
= Gθ. We know two ways to produce functions on  , with complex values and invariant by conjugation by G: on one hand, let π be an irreducible representation of G we can and do extend to a representation π+ of G ⋊ {1, θ}, then the restriction trace
, with complex values and invariant by conjugation by G: on one hand, let π be an irreducible representation of G we can and do extend to a representation π+ of G ⋊ {1, θ}, then the restriction trace  to
to  of the character of π+ is such a function; on the other hand, Lusztig define character-sheaves a, whose characteristic functions ϕ(a) are such functions too. We consider only “quadratic-unipotent” representations. For all such representation π, we define a suitable extension π+ , a character-sheave f(π) and we prove an identity trace
of the character of π+ is such a function; on the other hand, Lusztig define character-sheaves a, whose characteristic functions ϕ(a) are such functions too. We consider only “quadratic-unipotent” representations. For all such representation π, we define a suitable extension π+ , a character-sheave f(π) and we prove an identity trace = γ(π)ϕ(f(π)) with an explicit complex number γ(π).
= γ(π)ϕ(f(π)) with an explicit complex number γ(π).
Information
- Type
- Research Article
- Information
- Nagoya Mathematical Journal , Volume 182: Special Issue Celebrating the 60th Birthday of George Lusztig , June 2006 , pp. 313 - 379
- Copyright
- Copyright © Editorial Board of Nagoya Mathematical Journal 2006
References
Bibliographie
- 6
- Cited by

