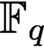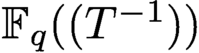Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kekeç, Gülcan
2023.
On Transcendental Regular Continued Fractions.
Mathematica Slovaca,
Vol. 73,
Issue. 6,
p.
1399.
Datta, Shreyasi
and
Xu, Yewei
2025.
Singular Vectors and ψ-Dirichlet Numbers over Function Field.
Journal de théorie des nombres de Bordeaux,
Vol. 36,
Issue. 3,
p.
1021.