Article contents
MOYENNES DE FONCTIONS ARITHMÉTIQUES DE FORMES BINAIRES
Published online by Cambridge University Press: 19 January 2012
Abstract
Extending classical results of Nair and Tenenbaum, we provide general, sharp upper bounds for sums of the type 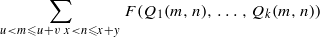 where x,y,u,v have comparable logarithms, F belongs to a class defined by a weak form of sub-multiplicativity, and the Qj are arbitrary binary forms. A specific feature of the results is that the bounds are uniform within the F-class and that, as in a recent version given by Henriot, the dependency with respect to the coefficients of the Qj is made explicit. These estimates play a crucial rôle in the proof, published separately by the authors, of Manin’s conjecture for Châtelet surfaces.
where x,y,u,v have comparable logarithms, F belongs to a class defined by a weak form of sub-multiplicativity, and the Qj are arbitrary binary forms. A specific feature of the results is that the bounds are uniform within the F-class and that, as in a recent version given by Henriot, the dependency with respect to the coefficients of the Qj is made explicit. These estimates play a crucial rôle in the proof, published separately by the authors, of Manin’s conjecture for Châtelet surfaces.
Résumé
Généralisant des résultats classiques de Nair et Tenenbaum, nous fournissons des majorations générales et optimales pour des sommes du type 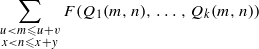 où les paramètres x,y,u,v ont des logarithmes comparables, F décrit une classe de fonctions définie par une condition de sous-multiplicativité faible, et les Qj sont des formes binaires arbitraires. Ces résultats sont caractérisés par leur uniformité dans la classe des fonctions F et, à l’instar d’une version récente donnée par Henriot, une dépendance explicite en fonction des coefficients des Qj. Ces estimations jouent un rôle crucial dans la preuve, publiée séparément par les auteurs, de la conjecture de Manin pour les surfaces de Châtelet.
où les paramètres x,y,u,v ont des logarithmes comparables, F décrit une classe de fonctions définie par une condition de sous-multiplicativité faible, et les Qj sont des formes binaires arbitraires. Ces résultats sont caractérisés par leur uniformité dans la classe des fonctions F et, à l’instar d’une version récente donnée par Henriot, une dépendance explicite en fonction des coefficients des Qj. Ces estimations jouent un rôle crucial dans la preuve, publiée séparément par les auteurs, de la conjecture de Manin pour les surfaces de Châtelet.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2012
References
- 7
- Cited by

