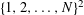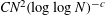Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Koh, Doowon
Lee, Sujin
Pham, Thang
and
Shen, Chun-Yen
2023.
Configurations of rectangles in Fq2.
Finite Fields and Their Applications,
Vol. 86,
Issue. ,
p.
102147.
Peluse, Sarah
Prendiville, Sean
and
Shao, Xuancheng
2024.
Bounds in a popular multidimensional nonlinear Roth theorem.
Journal of the London Mathematical Society,
Vol. 110,
Issue. 5,
Peluse, Sarah
2024.
Subsets of without L-shaped configurations.
Compositio Mathematica,
Vol. 160,
Issue. 1,
p.
176.
ACKELSBERG, ETHAN
2025.
Khintchine-type double recurrence in abelian groups.
Ergodic Theory and Dynamical Systems,
Vol. 45,
Issue. 1,
p.
1.