Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Galicer, Daniel
Merzbacher, Mariano
and
Pinasco, Damián
2019.
The Minimal Volume of Simplices Containing a Convex Body.
The Journal of Geometric Analysis,
Vol. 29,
Issue. 1,
p.
717.
Sarkar, Sherry
Xue, Alexander
and
Soberón, Pablo
2021.
Quantitative combinatorial geometry for concave functions.
Journal of Combinatorial Theory, Series A,
Vol. 182,
Issue. ,
p.
105465.
Damásdi, Gábor
Földvári, Viktória
and
Naszódi, Márton
2021.
Colorful Helly-type theorems for the volume of intersections of convex bodies.
Journal of Combinatorial Theory, Series A,
Vol. 178,
Issue. ,
p.
105361.
Dillon, Travis
and
Soberón, Pablo
2021.
A Mélange of Diameter Helly-Type Theorems.
SIAM Journal on Discrete Mathematics,
Vol. 35,
Issue. 3,
p.
1615.
Ivanov, Grigory
and
Naszódi, Márton
2022.
A Quantitative Helly-Type Theorem: Containment in a Homothet.
SIAM Journal on Discrete Mathematics,
Vol. 36,
Issue. 2,
p.
951.
Fernandez Vidal, Tomás
Galicer, Daniel
and
Merzbacher, Mariano
2022.
Continuous quantitative Helly-type results.
Proceedings of the American Mathematical Society,
Ivanov, Grigory
and
Naszódi, Márton
2022.
Functional John ellipsoids.
Journal of Functional Analysis,
Vol. 282,
Issue. 11,
p.
109441.
Jung, Attila
and
Naszódi, Márton
2022.
Quantitative Fractional Helly and (p,q)-Theorems.
European Journal of Combinatorics,
Vol. 99,
Issue. ,
p.
103424.
Messina, John A.
and
Soberón, Pablo
2022.
Isometric and affine copies of a set in volumetric Helly results.
Computational Geometry,
Vol. 103,
Issue. ,
p.
101855.
Brazitikos, Silouanos
and
Giannopoulos, Apostolos
2022.
Continuous Version of the Approximate Geometric Brascamp–Lieb Inequalities.
The Journal of Geometric Analysis,
Vol. 32,
Issue. 6,
Almendra-Hernández, Víctor Hugo
Ambrus, Gergely
and
Kendall, Matthew
2023.
Quantitative Helly-Type Theorems via Sparse Approximation.
Discrete & Computational Geometry,
Vol. 70,
Issue. 4,
p.
1707.
Satomi, Takashi
2024.
Inequality on the optimal constant of Young’s convolution inequality for locally compact groups and their closed subgroups.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 203,
Issue. 2,
p.
805.
Ivanov, Grigory
2025.
Quantitative Steinitz Theorem and Polarity.
Discrete & Computational Geometry,
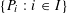 $\{P_{i}:i\in I\}$ of closed half-spaces in
$\{P_{i}:i\in I\}$ of closed half-spaces in  $\mathbb{R}^{n}$ such that
$\mathbb{R}^{n}$ such that 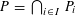 $P=\bigcap _{i\in I}P_{i}$ has positive volume, there exist
$P=\bigcap _{i\in I}P_{i}$ has positive volume, there exist 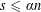 $s\leqslant \unicode[STIX]{x1D6FC}n$ and
$s\leqslant \unicode[STIX]{x1D6FC}n$ and 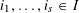 $i_{1},\ldots ,i_{s}\in I$ such that
$i_{1},\ldots ,i_{s}\in I$ such that 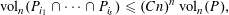 $$\begin{eqnarray}\operatorname{vol}_{n}(P_{i_{1}}\cap \cdots \cap P_{i_{s}})\leqslant (Cn)^{n}\operatorname{vol}_{n}(P),\end{eqnarray}$$
$$\begin{eqnarray}\operatorname{vol}_{n}(P_{i_{1}}\cap \cdots \cap P_{i_{s}})\leqslant (Cn)^{n}\operatorname{vol}_{n}(P),\end{eqnarray}$$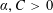 $\unicode[STIX]{x1D6FC},C>0$ are absolute constants. These results complement and improve previous work of Bárány et al and Naszódi. Our method combines the work of Srivastava on approximate John’s decompositions with few vectors, a new estimate on the corresponding constant in the Brascamp–Lieb inequality and an appropriate variant of Ball’s proof of the reverse isoperimetric inequality.
$\unicode[STIX]{x1D6FC},C>0$ are absolute constants. These results complement and improve previous work of Bárány et al and Naszódi. Our method combines the work of Srivastava on approximate John’s decompositions with few vectors, a new estimate on the corresponding constant in the Brascamp–Lieb inequality and an appropriate variant of Ball’s proof of the reverse isoperimetric inequality.
