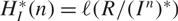Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Goel, Kriti
Koley, Mitra
and
Verma, J. K.
2021.
Hilbert-Kunz function and Hilbert-Kunz multiplicity of some ideals of the Rees algebra.
Communications in Algebra,
Vol. 49,
Issue. 7,
p.
3066.
Banerjee, Arindam
Goel, Kriti
and
Verma, J.
2021.
Commutative Algebra.
Vol. 773,
Issue. ,
p.
207.
Dubey, Saipriya
Mukundan, Vivek
and
Verma, Jugal
2021.
Tight closure of powers of parameter ideals in hypersurface rings and their tight Hilbert polynomials.
Research in the Mathematical Sciences,
Vol. 8,
Issue. 4,
Dubey, Saipriya
Quy, Pham Hung
and
Verma, Jugal
2023.
Tight Hilbert polynomial and F-rational local rings.
Research in the Mathematical Sciences,
Vol. 10,
Issue. 1,
Puthenpurakal, Tony J.
2024.
Itoh’s conjecture for normal ideals.
Mathematische Zeitschrift,
Vol. 307,
Issue. 1,
Ma, Linquan
and
Quy, Pham Hung
2024.
Vanishing and Non-negativity of the First Normal Hilbert Coefficient.
Acta Mathematica Vietnamica,
Vol. 49,
Issue. 3,
p.
311.
Duong, Thi Huong
Nguyen, Tuan Long
and
Pham, Hung Quy
2025.
The vanishing of the first tight Hilbert coefficient for Buchsbaum rings.
Communications in Algebra,
Vol. 53,
Issue. 9,
p.
3934.

 ) be an analytically unramified local ring of positive prime characteristic
) be an analytically unramified local ring of positive prime characteristic