Article contents
Integrals with a large parameter and several nearly coincident saddle points; the continuation of uniformly asymptotic expansions
Published online by Cambridge University Press: 24 October 2008
Abstract
In the contour integral
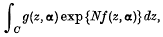
f and g are regular functions of z in a neighbourhood of the contour C and of the complex parameters (α1, α2, …, αp) = α in a domain of 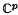 . N is a positive parameter and asymptotic expansions are considered as N → + ∞. The method of steepest descents provides an asymptotic expansion for each fixed value of α, but this is non-uniform with respect to α near critical values α0 at which certain saddle points tend to coincidence. A more complicated expansion, involving Airy functions or generalizations thereof, is valid and uniform for α near α0. This expansion is known to be valid in a neighbourhood of α0 which does not depend on N, in contrast to certain other expansions having regions of validity which contract to a point or surface as N → ∞. It is thereby suggested that the actual region of validity has a definite geometrical shape determined by the functions f and g and the contour C, just as steepest descents expansions are valid in regions bounded by Stokes' lines. In this paper, procedures are derived for the determination of such regions, subject to assumptions concerning f, g and C. The validity of the expansion is established in regions so determined.
. N is a positive parameter and asymptotic expansions are considered as N → + ∞. The method of steepest descents provides an asymptotic expansion for each fixed value of α, but this is non-uniform with respect to α near critical values α0 at which certain saddle points tend to coincidence. A more complicated expansion, involving Airy functions or generalizations thereof, is valid and uniform for α near α0. This expansion is known to be valid in a neighbourhood of α0 which does not depend on N, in contrast to certain other expansions having regions of validity which contract to a point or surface as N → ∞. It is thereby suggested that the actual region of validity has a definite geometrical shape determined by the functions f and g and the contour C, just as steepest descents expansions are valid in regions bounded by Stokes' lines. In this paper, procedures are derived for the determination of such regions, subject to assumptions concerning f, g and C. The validity of the expansion is established in regions so determined.
Information
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 76 , Issue 1 , July 1974 , pp. 211 - 231
- Copyright
- Copyright © Cambridge Philosophical Society 1974
References
REFERENCES
- 8
- Cited by

