No CrossRef data available.
Article contents
Fillability of small Seifert fibered spaces
Published online by Cambridge University Press: 25 November 2022
Abstract
On small Seifert fibered spaces 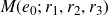 $M(e_0;\,r_1,r_2,r_3)$ with
$M(e_0;\,r_1,r_2,r_3)$ with 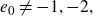 $e_0\neq-1,-2,$ all tight contact structures are Stein fillable. This is not the case for
$e_0\neq-1,-2,$ all tight contact structures are Stein fillable. This is not the case for 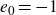 $e_0=-1$ or
$e_0=-1$ or 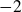 $-2$. However, for negative twisting structures it is expected that they are all symplectically fillable. Here, we characterise fillable structures among zero-twisting contact structures on small Seifert fibered spaces of the form
$-2$. However, for negative twisting structures it is expected that they are all symplectically fillable. Here, we characterise fillable structures among zero-twisting contact structures on small Seifert fibered spaces of the form 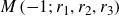 $M\left({-}1;\,r_1,r_2,r_3\right)$. The result is obtained by analysing monodromy factorizations of associated planar open books.
$M\left({-}1;\,r_1,r_2,r_3\right)$. The result is obtained by analysing monodromy factorizations of associated planar open books.
Information
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 174 , Issue 3 , May 2023 , pp. 585 - 604
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Cambridge Philosophical Society
References
Cox, D. A., Little, J. B. and Schenck, H. K.. Toric Varieties. Graduate Studies in Math. 124 (Amer Math. Soc., Providence, RI, 2011).CrossRefGoogle Scholar
Ding, F. and Geiges, H.. Handle moves in contact surgery diagrams. J. Topol. 2 (2009), 105–122.Google Scholar
Endo, H., Mark, T. and Van Horn-Morris, J.. Monodromy substitutions and rational blowdowns. J. Topol. 4 (2011), 227–253.Google Scholar
Ghiggini, P.. On tight contact structures with negative maximal twisting number on small Seifert manifolds, Algebr. Geom. Topol. 8 (2008), 381–396.CrossRefGoogle Scholar
Ghiggini, P., Lisca, P. and Stipsicz, A.. Classification of tight contact structures on small Seifert 3-manifolds with
 $e_0\geq 0$
, Proc. Amer. Math. Soc. 134 (2006), 909–916.CrossRefGoogle Scholar
$e_0\geq 0$
, Proc. Amer. Math. Soc. 134 (2006), 909–916.CrossRefGoogle Scholar
Ghiggini, P., Lisca, P. and Stipsicz, A.. Tight contact structures on some small Seifert fibered 3-manifolds, Amer. J. Math. 129(5) (2007), 1403–1447.CrossRefGoogle Scholar
Ghiggini, P. and Van Horn–Morris, J.. Tight contact structures on the Brieskorn spheres.
 $-\Sigma(2,3,6n-1)$
and contact invariants, J. Reine Angew. Math. 718 (2016), 1–24.Google Scholar
$-\Sigma(2,3,6n-1)$
and contact invariants, J. Reine Angew. Math. 718 (2016), 1–24.Google Scholar
Gompf, R.. Handlebody construction of Stein surfaces. Ann. of Math. 148 (1998), 619–693.Google Scholar
Lecuona, A. G. and Lisca, P.. Stein fillable Seifert fibered 3–manifolds, Algebr. Geom. Topol. 11 (2011), 625–642.CrossRefGoogle Scholar
Lisca, P. and Stipsicz, A.. Ozsváath-Szabó variants and tight contact 3-manifolds III, J. Symplectic Geom. 5(4) (2007), 357–384.Google Scholar
Lisca, P. and Stipsicz, A.. On the existence of tight contact structures on Seifert fibered 3-manifolds, Duke Math. J. 148(2) (2009), 175–209.CrossRefGoogle Scholar
Margalit, D. and McCammond, J.. Geometric presentations for the pure braid group, J. Knot Theory Ramifications 18 (2009), 1–20.Google Scholar
Matkovič, I.. Classification of tight contact structures on small Seifert fibered L-spaces, Algebr. Geom. Topol. 18 (2018), 111–152.CrossRefGoogle Scholar
Plamenevskaya, O. and Van Horn–Morris, J.. Planar open books, monodromy factorisations and symplectic fillings, Geom. Topol. 14 (2010), 2077–2101.CrossRefGoogle Scholar
Schöberger, S.. Determining symplectic fillings from planar open books, J. Symplectic Geom., 5(1) (2007), 19–41.Google Scholar
Stipsicz, A.. Ozsváath-Szabó variants and 3-dimensional contact topology. Proceedings of the International Congress of Mathematicians (Hyderabad 2010), vol. II, 1159–1178.Google Scholar
Tosun, B.. Tight small Seifert fibered spaces with.
 $e_0=-2$
, Algebr. Geom. Topol. 20 (2020), 1–27.Google Scholar
$e_0=-2$
, Algebr. Geom. Topol. 20 (2020), 1–27.Google Scholar
Wendl, C.. Strongly fillable contact manifolds and J-holomorphic foliations, Duke Math. J. 151 (2010), 337–384.CrossRefGoogle Scholar
Wu, H.. Legendrian vertical circles in small Seifert spaces, Commun. Contemp. Math. 8 (2006), 219–246.Google Scholar

