Article contents
On the bivariate Erdős–Kac theorem and correlations of the Möbius function
Published online by Cambridge University Press: 14 August 2019
Abstract
Given a positive integer n let ω (n) denote the number of distinct prime factors of n, and let a be fixed positive integer. Extending work of Kubilius, we develop a bivariate probabilistic model to study the joint distribution of the deterministic vectors (ω(n), ω(n + a)), with n ≤ x as x → ∞, where n and n + a belong to a subset of ℕ with suitable properties. We thus establish a quantitative version of a bivariate analogue of the Erdős–Kac theorem on proper subsets of ℕ.
We give three applications of this result. First, if y = x0(1) is not too small then we prove (in a quantitative way) that the y-truncated Möbius function μy has small binary autocorrelations. This gives a new proof of a result due to Daboussi and Sarkőzy. Second, if μ(n; u) :=e(uω(n)), where u ∈ ℝ then we show that μ(.; u) also has small binary autocorrelations whenever u = o(1) and 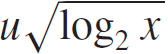 $u\sqrt {\mathop {\log }\nolimits_2 x}\to \infty$, as x → ∞. These can be viewed as partial results in the direction of a conjecture of Chowla on binary correlations of the Möbius function.
$u\sqrt {\mathop {\log }\nolimits_2 x}\to \infty$, as x → ∞. These can be viewed as partial results in the direction of a conjecture of Chowla on binary correlations of the Möbius function.
Our final application is related to a problem of Erdős and Mirsky on the number of consecutive integers less than x with the same number of divisors. If  $y = x^{{1 \over \beta }}$, where β = β(x) satisfies certain mild growth conditions, we prove a lower bound for the number of consecutive integers n ≤ x that have the same number of y-smooth divisors. Our bound matches the order of magnitude of the one conjectured for the original Erdős-Mirsky problem.
$y = x^{{1 \over \beta }}$, where β = β(x) satisfies certain mild growth conditions, we prove a lower bound for the number of consecutive integers n ≤ x that have the same number of y-smooth divisors. Our bound matches the order of magnitude of the one conjectured for the original Erdős-Mirsky problem.
Information
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 169 , Issue 3 , November 2020 , pp. 547 - 605
- Copyright
- © Cambridge Philosophical Society 2019
References
REFERENCES
- 3
- Cited by

