A friend of mine [1] mentioned a problem to me, which he was told had an interesting solution involving an unexpected square root. I have not seen this problem described elsewhere, so I have carried out my own analysis, which I will present here. In fact the solution involves not only square roots, but also higher roots … and a logarithm.

We denote the real logarithm of a positive number a by ln a, so that ax = exp (x ln a), and we shall discuss what is known about the real solutions x of the equation (1)

$$x = {a^x},\;\,a > 0.$$

Many players know that the secret of winning the game of nim (and other “impartial” combinatorial games) is to write the sizes of the game’s piles in base 2 and then add them together without carry. The proof of this well-known procedure (described below) is both straightforward and convincing. Nonetheless, the procedure still appears magical, as though a rabbit has been pulled out of a hat. Astute students (and frustrated professors) often ask why the winning strategy for such games involves base 2, and not some other base. After all, nothing about the game of nim itself – the game rules, the configuration of the tokens, etc. – provides any hints about the origin of base 2 in this setting. Minimal insight is offered by most published proofs, which themselves tend to either appear almost wizardly in nature (i.e. assume the base-2 method and show that it miraculously solves the problem) or employ combinatorial arguments that supply little abstract intuition (at least to the authors of this article).

The business of making mathematical approximations in the physical sciences has a long and noble history. For example, in the earliest days of pyramid construction in ancient Egypt it was necessary to approximate lengths required in construction, especially when they involved irrational numbers. Similarly, surveyors in early Greece seeking to lay out profiles of right-angle triangles or circles on the ground invariably ended up making approximations regarding measurements of required lengths, as indeed is the case today. Practitioners have always faced the problem of having to decide when parameters in theory have been met satisfactorily in the practice of measurement. Further, before the advent of hand-held calculators, students in schools in the UK would have been very familiar with the approximation 22/7 for the transcendental number π, obtained perhaps by comparing (as this author did) the measured circumferences of many laboriously drawn circles of different sizes with their diameters. Despite the advent of sophisticated calculating devices and facilities, such as computers and spreadsheets, the practice of making approximations is still much in evidence in theoretical work in fields associated with physical phenomena. Such approximations often result in formulae that are easy to use and remember, and moreover can produce theoretical results that support directly, or otherwise, results from measurements. In this respect, the practical mathematician does not have to seek results to many decimal places when measurement facilities allow for accuracy to only a few. The purpose of this Article is to illustrate this point by discussing an example drawn from the realms of antenna theory, relating to the performance of a dipole antenna. It is not the purpose here to delve into the derivation of dipole theory, but to extract the relevant information and show how useful mathematical approximations can be employed to simplify a relationship between parameters of interest to an antenna engineer. To this end, it will first be necessary to introduce some antenna concepts that might be new to the reader.

The product over the integers 1 to N is written as N!, is called N factorial, and is defined as: (1)

$${\rm{N! = 1 \times 2 \times 3 \times }} \ldots {\rm{ \times (N - 1) \times N}}{\rm{.}}$$

Let us define a Fibonacci-Lucas hyperbola as a hyperbola passing through an infinite number of points of the form (Fm, Ln), where the Fm are distinct Fibonacci numbers (0, 1, 1, 2, 3, 5, 8, 13, 21,…, where F0 = 0), and the Ln are distinct Lucas numbers (2, 1, 3, 4, 7, 11, 18, 29,…,. where L0 = 2). The simplest examples are 5x2 - y2 = 4, which contains the points (Fk, Lk) with odd subscripts, e.g. (1, 1), (2, 4), (5, 11), and 5x2 - y2 = -4, which contains the points with even subscripts, e.g. (0, 2), (1, 3), (3, 7); (see [1, 2]). These follow immediately from the identity (1)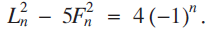

$$L_n^2 - 5F_n^2 = 4{( - 1)^n}.$$

On his website dedicated to questions and investigations arising out of dynamic geometry technology, Michael de Villiers has a series called Geometry Loci Doodling [1]. These are locus problems connected to the centroids of cyclic quadrilaterals – ‘centroids’ in the plural, for there are three different kinds of centroid depending whether one understands the quadrilateral in terms of its vertices, perimeter or area. The corresponding centroids are the point-mass centroid, the perimeter-centroid, and the lamina-centroid. In each case, de Villiers keeps three vertices of the quadrilateral fixed on the circumcircle, and then traces the locus of the different centroids as the fourth point moves round the circle. In this paper, I shall take a brief look at the point-mass centroid and then a lingering view of the lamina-centroid.

Many papers and indeed books have been written about the problem of dissecting a number of squares of different integer side length and reassembling the pieces to form a single square (see [1], [2] and [3]). For example, in the case of 32 + 42 = 52, we can achieve a four-piece dissection as follows:

In [1] de Villiers points out the duality between sides and angles of quadrilaterals. The first objective of this article is make explicit two new dualities in the quadrilaterals. For this we will consider the diagonal segments: if diagonals AC and BD of a quadrilateral ABCD intersect at O, we call the diagonal segments OA, OB, OC, OD. According to [2, pp. 179-188], hierarchical classifications of the convex quadrilaterals are made taking as classification criteria the quantity and position of sides, angles and diagonal segments. In hierarchical classifications the more particular concepts form subsets of the more general concepts. Through classification according to the number and position of equal sides it is possible to define six families: four sides equal, at least three equal, two opposite pairs equal, two consecutive pairs equal, at least one opposite pair equal, at least one consecutive pair equal. In the families two opposite pairs equal and two consecutive pairs equal, the pairs may be equal to each other and then the four sides are equal. So in these two families the possibility DA = AB and AB = BC is excluded. Families analogous to the previous ones can be defined taking as a criterion of hierarchical classification the quantity and position of equal angles or the quantity and position of equal diagonal segments.

Figure 1 shows the photograph of a tractrix made of cards. This is a simple yet captivating way to make a tractrix — an arrangement that has recently appeared in some engaging pedagogical resources and literature [1, 2]. When closely spaced cards lean like this, one after another, over a horizontal plane, the contour created is a tractrix — a curve of significance in mathematics, since its revolution around its asymptote produces a pseudosphere: a curved surface with constant negative Gaussian curvature [3, 4].

Any mathematics student who has ever used the cosine rule to investigate simple properties of an integer triangle will immediately have realised that the cosine of each angle of the triangle must be a rational number. It is clear, however, that the same is not in general true for the sines. In [1], it is shown how to use a property of the sines of the angles of an integer triangle to categorise the triangle as being of a particular class. In this article, we develop some of the concepts and results of [1] to derive a method for generating integer triangles of a given class. Finally, we apply our results to find all primitive integer triangles in the particular case of Heronian triangles.

We describe various methods to derive monotonic infinite series for fractions near π and obtain a variety of series for the special case of its convergents. These series immediately show that π is clearly different from these fractions, replicating with series the results in Dalzell [1, 2] and Lucas that used integrals with non-negative integrands to represent the gaps between π and fractions.