No CrossRef data available.
Article contents
Which is bigger? An intriguing ‘double alternation’
Published online by Cambridge University Press: 23 January 2015
Extract
The following three inequalities hold:

The first inequality is trivial. The second one was proved without calculating aids in note [1], and the third along similar lines in note [2]. The author of note [2] also suggested an extension to the relation between
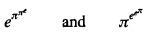
How best to continue the sequence of inequalities is not obvious and we return to that point shortly. Before doing so, we note that an interesting geralisation is to replace π by a variable x, and to determine the precise interval of x in which the regularity of the ‘alternation of inequality signs’ is maintained. We need no longer consider particular properties of π.
Information
- Type
- Articles
- Information
- Copyright
- Copyright © The Mathematical Association 2014
References
2.
Pinter, K., Which is bigger: ee
π or ππ
e?, Math. Gaz. 89 (November 2005) pp.470–471.Google Scholar

