Hostname: page-component-66644f4456-5jvrx Total loading time: 0 Render date: 2025-02-12T13:16:05.282Z Has data issue: true hasContentIssue false
  • English
  • Français

107.27 The discrete renewal theorem with bounded interevent times

Published online by Cambridge University Press:  03 July 2023

Rohan Manojkumar Shenoy
Rohan Manojkumar Shenoy*
Affiliation:
Thatch, Baildon Close, Nottingham NG8 1BS e-mail: rohan.shenoy22@imperial.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
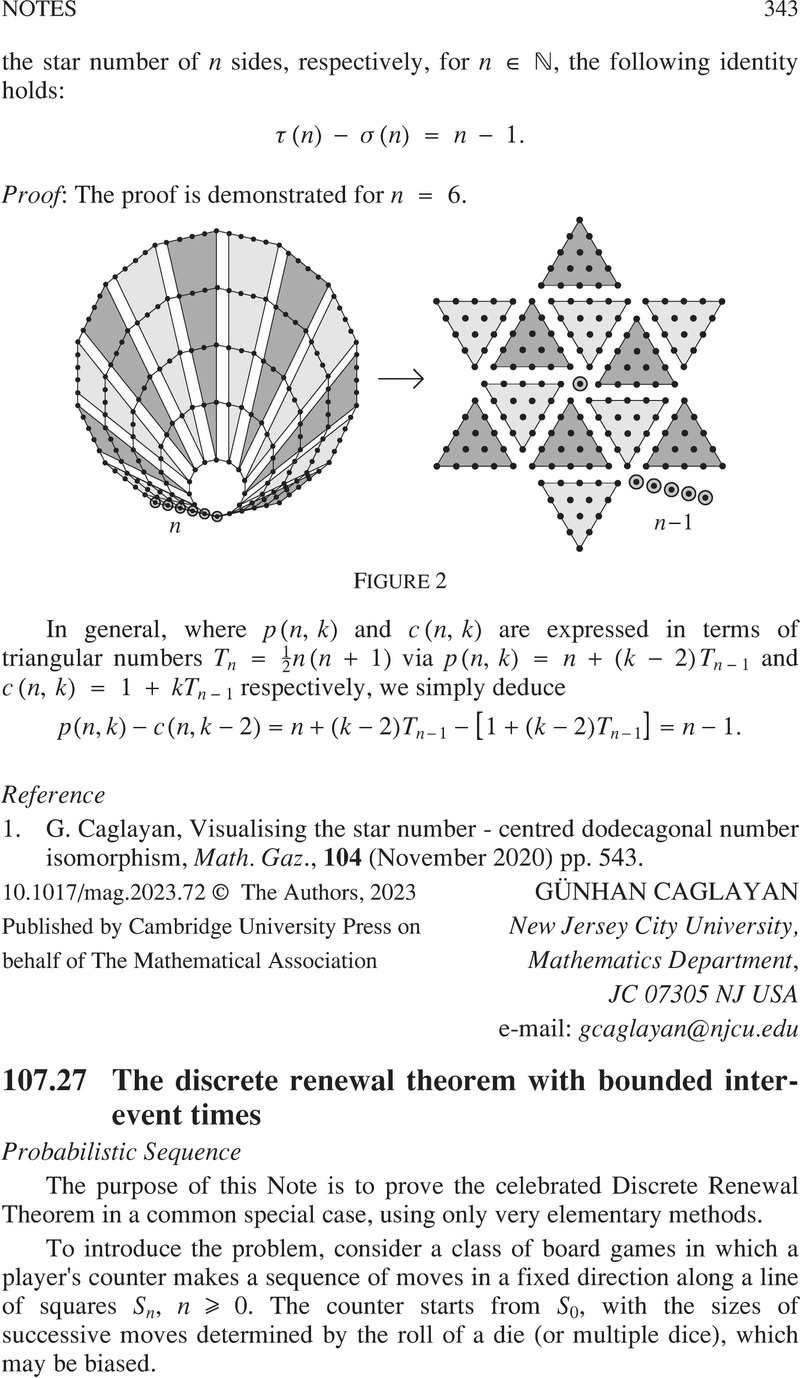
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Notes
Information
The Mathematical Gazette , Volume 107 , Issue 569 , July 2023 , pp. 343 - 348
Check for updates
Copyright
© The Authors, 2023. Published by Cambridge University Press on behalf of The Mathematical Association

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Feller, William, An introduction to probability theory and its applications, Volume I, (3rd edition), Princeton University (1968).Google Scholar
Cox, D. R., Point processes and renewal theory: a brief survey. Electronic systems effectiveness and life cycle costing, (1983) pp. 107–112.CrossRefGoogle Scholar
Adu-Sackey, Albert, Oduro, Francis T., Fosu, G. O., Inequalities approach in determination of convergence of recurrence sequences, Open Journal of Mathematical Sciences, 5(1) (2021) pp. 65–72.CrossRefGoogle Scholar
Fuller, William R, Sequences, Springer (1977).Google Scholar
Erdős, Paul, Feller, William and Pollard, Harry, A property of power series with positive coefficients, Bulletin of the American Mathematical Society, 55(2) (1949) pp. 201–204.CrossRefGoogle Scholar
Smith, Walter L., Renewal theory and its ramifications, Journal of the Royal Statistical Society: Series B (Methodological), 20(2) (1958) pp. 243–284.Google Scholar
Resnick, Sidney I., Adventures in stochastic processes, Springer Science & Business Media (1992).Google Scholar