1. Introduction
The p-11B fusion reaction produces 3 α-particles with a Q-value of about 8.7 MeV. A mixture of H and 11B has been proposed as an advanced fusion fuel because of certain attractive features [Reference Dawson1, Reference Hora, Eliezer and Kirchhoff2]. With regard to the reactants, they are abundant in nature (implying that no breeding would be needed), stable (meaning that issues like those associated with the radioactivity of tritium in DT fusion would be avoided), and cheap. With regard to the fusion products, there are only charged particles so that all the reaction energy can be released to the fuel (it is also worth noticing the possibility of direct energy conversion into electricity, without passing through a thermodynamic cycle). More importantly, no neutron is generated, meaning no induced activation of the environment surrounding the fuel (actually, there is still a residual neutron production in the fuel through the (p,n) and (α,n) side reactions on 11B, though the rate is very low). Finally, as an inertial confinement fusion (ICF) fuel, the target would not need to be cryogenic.
A plot of the fusion cross section, σf, as a function of the centre of mass (CM) energy, ECM, is shown in Figure 1(a). Resonances of major interest for H-11B fusion are bounded by dashed lines: at 148 keV (with a width of just 5 keV) and 612 keV (with a width of 300 keV) [Reference Nevins and Swain3]. At 612 keV,σf reaches its maximum value, 1.4 barn [Reference Sikora and Weller6]. Below approximately 3.5 MeV, the reaction proceeds through 3 channels [Reference Sikora and Weller6, Reference Becker, Rolfs and Trautvetter7]: the low branching-ratio 12C direct breakup and the sequential decays via the first excited state or the ground state of 8Be, i.e.:
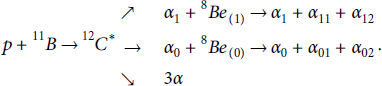
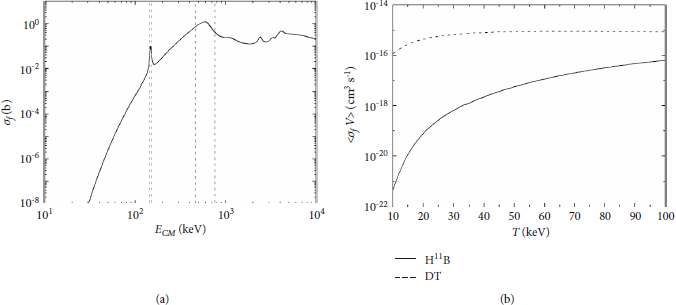
Figure 1: Fusion cross section and reactivity of H-11B fuel. (a) Fusion cross section as a function of the CM energy, based on the analytic approximation of Nevins and Swain [Reference Nevins and Swain3] below 3.5 MeV and, above, on TENDL evaluated data. Resonances of major interest for H-11B fusion are bounded by dashed lines. (b) Reactivity as a function of ion temperature for H-11B fuel and, as a term of comparison, DT fuel. Plots are based on the analytic approximations of Nevins and Swain [Reference Nevins and Swain3] and Bosch and Hale [Reference Bosch and Hale4], respectively. Republished from Belloni [Reference Belloni5]. © IOP Publishing Ltd. 2021.
Summed over the reaction channels, the energy spectrum of the generated α-particles is a continuum; for an incoming proton with energy at the cross section maximum, it extends up to about 6.7 MeV in the laboratory. The spectrum is strongly peaked around 4 MeV. One can say that, on average, two α’s are emitted at 4 MeV, while one is emitted at 1 MeV [Reference Stave, Ahmed and France8]. The α’s angular distribution is isotropic in the CM system (nearly in the laboratory, because of the proton momentum).
In Figure 1(b), the reactivity,
![]() , is shown as a function of ion temperature for H-11B fuel and, as a term of comparison, DT fuel [Reference Nevins and Swain3, Reference Bosch and Hale4]. We recall that the thermonuclear specific reaction rate is given by
, is shown as a function of ion temperature for H-11B fuel and, as a term of comparison, DT fuel [Reference Nevins and Swain3, Reference Bosch and Hale4]. We recall that the thermonuclear specific reaction rate is given by
![]() , where nX and nY are the number densities of the fusing species. The low reactivity represents a major drawback of H-11B fuel: for instance, at temperatures of the order of those currently achievable in magnetic and inertial confinement experiments (around 10 keV), the H-11B reactivity is 5 orders of magnitude lower than the DT reactivity.
, where nX and nY are the number densities of the fusing species. The low reactivity represents a major drawback of H-11B fuel: for instance, at temperatures of the order of those currently achievable in magnetic and inertial confinement experiments (around 10 keV), the H-11B reactivity is 5 orders of magnitude lower than the DT reactivity.
The first comprehensive assessment of the viability of H-11B fuel for thermonuclear fusion was carried out by Moreau [Reference Moreau9] at the Princeton Plasma Physics Laboratory, mid-seventies. A steady-state, two-temperature (T
e
, T
i
) analysis of the balance of the power fluxes was applied: namely, from the fusion products the heat goes entirely to the fuel ions, then from the ions to the electrons, finally into radiation. Note that this power flow scheme requires
![]() . In the case Ti = Te, the plasma was found to not ignite because of the predominance of radiation losses. We recall that the fusion power per unit volume, PF, is given by the product RQ, where Q is the reactionQ-value; the specific power transfer from ions to electrons,
. In the case Ti = Te, the plasma was found to not ignite because of the predominance of radiation losses. We recall that the fusion power per unit volume, PF, is given by the product RQ, where Q is the reactionQ-value; the specific power transfer from ions to electrons,
![]() , is proportional to
, is proportional to
![]() , while the radiation power lost by bremsstrahlung, PB, scales as
, while the radiation power lost by bremsstrahlung, PB, scales as
![]() and the synchrotron radiation power, PS, as
and the synchrotron radiation power, PS, as
![]() , where B is the magnetic field. Confinement requires that
, where B is the magnetic field. Confinement requires that
![]() , where β is the beta ratio, the main parameter for confinement efficiency (typically,
, where β is the beta ratio, the main parameter for confinement efficiency (typically,
![]() in tokamaks, though higher values are desirable for fusion power production). For ignition to occur, the curves
in tokamaks, though higher values are desirable for fusion power production). For ignition to occur, the curves
![]() and
and
![]() , where η is the recirculating power fraction, must intersect in the T
e
-T
i
plane.
, where η is the recirculating power fraction, must intersect in the T
e
-T
i
plane.
For the case of magnetic confinement, upon realistic assumptions for the recirculating power and confinement conditions, this analysis showed that no ignition point could arise in the T e -T i plane when, in addition to bremsstrahlung, synchrotron radiation losses were also taken into account. The conclusion was that H-11B fusion is unfeasible in tokamaks. A chance, however, could come from inertial confinement, where only bremsstrahlung losses count. In this case, working at much higher densities, ignition points do exist in the T e -T i plane (Figure 2). For example, upon the hypothesis of a 70% fraction of fusion power to fuel ions, an ignition point exists for T e = 140 keV and T i = 280 keV, though these values are very high. At a fuel density of 1027 ion cm−3 (boron-to-proton concentration just lower than 10%), the Lawson criterion requires a minimum confinement time, τ 0, of 16 ps. Then, igniting the smallest possible pellet, with radius τ 0 times the speed of sound, would require a laser energy of 7 MJ. Such a figure is still challenging today, 40 years after Moreau’s study. This explains why H-11B fusion was put on hold, at least on the ground of experiment, and it took almost 30 years before having its first demonstration by lasers [Reference Belyaev, Matafonov and Vinogradov10]. Moreover, the demonstration was achieved very far from the prescribed thermonuclear regime, indeed by exploiting an effect unknown at the time of Moreau’s work, which is laser acceleration of ions.
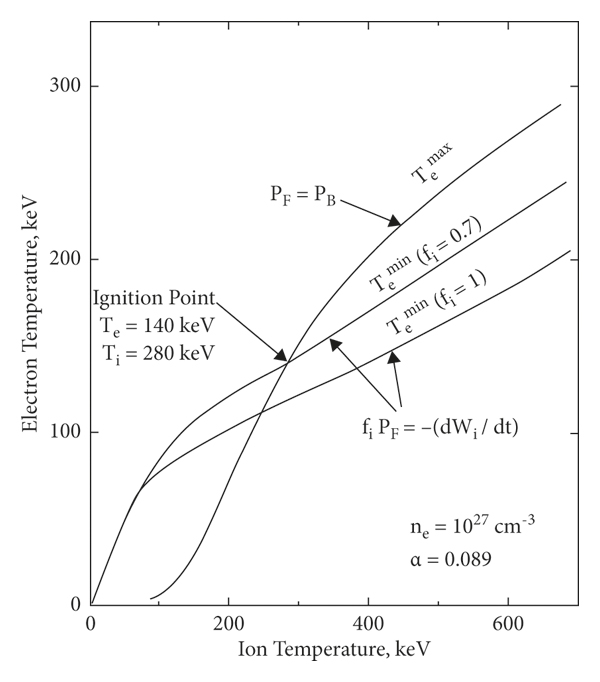
Figure 2: Ignition points in the T e -T i plane for high-density H-11B plasma. f i is the fraction of fusion power to plasma ions; α is the boron-to-proton ion concentration. Reproduced from Moreau [Reference Moreau9] with the permission of the publisher. © IAEA 1977.
This article is intended to give an overview of the nonthermal effects which can complement and supplement the thermonuclear burn of H-11B fuel, through fusion events’ multiplication or chain-like mechanisms. The following processes and concepts are reviewed:
-
(a) Fusion chain progressing via intermediate nuclear reactions;
-
(b) Suprathermal fusion chain, i.e., the chain sustained by suprathermal fuel ions elastically scattered by the fusion-born α’s; and
-
(c) The energy multiplication (or amplification) factor in a beam-driven fusion scheme, which is relevant to proton fast ignition.
2. Multiplication Processes
2.1. Fusion Chain via Intermediate Nuclear Reactions
The possibility of a fusion chain progressing via intermediate nuclear reactions in H-B targets has been presented in two works by Belyaev et al. [Reference Belyaev, Krainov, Zagreev and Matafonov11, Reference Belyaev, Krainov, Matafonov and Zagreev12]. The basic chain relies on the fact that a fusion-born α-particle can in turn react with 11B through the reaction:
which generates a high-energy proton. This proton can in turn fuse with 11B, possibly giving rise to a chain reaction. However, there is the competing reaction:
which acts as a sink of α’s and generates neutrons. The neutron- and proton-generating reactions have a comparable cross section.
To compensate for the loss of α-particles in the fuel due to the neutron channel, and to reabsorb the neutron inside the fuel, one should exploit neutron capture on 10B, i.e.:
This means that natural boron should be used in the fuel, or 11B should be adequately supplemented with 10B.
Along all the reaction pathways in the chain, the authors find that the number of protons, α-particles, and neutrons in the fuel grows up as an avalanche over times of the order of 1 μs, approximately; cfr. Figure 1 in ref. [Reference Belyaev, Krainov, Matafonov and Zagreev12]. One has to remark, however, that this approach lies upon highly idealised assumptions, in particular,
-
(i) maximum values of the reaction cross sections have been used,
-
(ii) particles’ energy losses have been neglected, and
-
(iii) a too long confinement time is required, which is unrealistic for warm, solid-density fuel.
Shmatov [Reference Shmatov13] has finally shown that at least for temperatures up to 100 keV, only a tiny fraction of α-particles would be capable to react with 11B because of their loss of energy in the fuel, thus preventing the development of the chain. On another note, if Belyaev et al.’s chain developed, fuel neutronicity would become considerably high, which would jeopardise the most appealing feature of H-11B fusion.
It is also worth mentioning that experiments have been done by Labaune et al. [Reference Labaune, Baccou, Yahia, Neuville and Rafelski14] at LULI, France, to test the possibility of inducing a chain reaction in natural-boron or boron-nitride targets under irradiation by laser-accelerated protons (generated from a thin foil). Targets were solid or conditioned in a plasma state by laser irradiation. The authors intended to exploit several nuclear reaction pathways, as detailed in Figure 3. Even in the absence of a self-sustaining chain, they hoped that secondary reactions could substantially increase the energy yield compared with a pure p-11B fusion scenario. While secondary reactions have successfully been induced and measured under such schemes, one has nevertheless to conclude that their rate is too low to induce any significant avalanche process or increase in the energy yield. For instance, in the case of a solid boron-nitride target, the overall number of the X(p,11C) reactions, with X = 11B or 14N (Figure 3(b)), was estimated at 106 per shot by means of 11C decay measurements. This figure appears to be at least 1000 times smaller than the number of 11B(p, 3α) fusion reactions (>109 per shot).
Figure 3: Scheme of the main primary and secondary nuclear reactions produced by the interaction between a laser-accelerated proton beam and (a) a natural boron target and (b) a boron-nitride target. Reproduced from Labaune et al. [Reference Labaune, Baccou, Yahia, Neuville and Rafelski14], under the terms of the Creative Commons CC BY License.
2.2. Suprathermal Fusion Chain
The fact that three charged, massive, energetic particles are produced in the p-11B reaction suggests that the fusion yield could effectively be enhanced by the elastic scattering of fuel ions to energies corresponding to the highest values of σf. This particularly applies to protons because of their higher charge-to-mass ratio compared with 11B ions. While thermalising, some of the protons in these showers can undergo fusion, eventually setting a chain reaction up. At high matter density, moreover, α’s tend to lose energy mostly to plasma ions rather than to electrons. This happens when the electrons’ Fermi velocity (or their thermal velocity) becomes comparable to the α-particle velocity, while the ion thermal velocity remains substantially lower [Reference Gryzinski15–Reference Son and Fisch17].
The suprathermal fusion chain in an infinite, homogeneous H-11B plasma can be effectively parameterised in terms of two multiplication (or reproduction) factors. An α-particle emitted at a certain energy
![]() in a primary fusion event is characterised by the multiplication factor
in a primary fusion event is characterised by the multiplication factor
![]() , i.e., the average number of secondary α-particles generated via suprathermal processes during the slowing down of the primary particle. Likewise, the multiplication factor can be expressed in terms of fusion events. Then, one defines k ∞ as the average number of secondary fusion events per primary event. k ∞ can be estimated through the integration of kα over the α-emission spectrum,
, i.e., the average number of secondary α-particles generated via suprathermal processes during the slowing down of the primary particle. Likewise, the multiplication factor can be expressed in terms of fusion events. Then, one defines k ∞ as the average number of secondary fusion events per primary event. k ∞ can be estimated through the integration of kα over the α-emission spectrum,
![]() [Reference Belloni5]:
[Reference Belloni5]:
where
![]() . Strictly speaking, the concept of k ∞ is well grounded as long as the emitted α-particles have a comparable slowing-down time, and this quantity is in turn comparable to the (average) period between two consecutive generations of fusion events, τg. It is not difficult, then, to calculate the cumulative number of fusion events per unit volume at the time t,
. Strictly speaking, the concept of k ∞ is well grounded as long as the emitted α-particles have a comparable slowing-down time, and this quantity is in turn comparable to the (average) period between two consecutive generations of fusion events, τg. It is not difficult, then, to calculate the cumulative number of fusion events per unit volume at the time t,
![]() , in regime of multiplication, upon the thermonuclear specific rate R. Depending on the value of k ∞, one can distinguish three cases for the time evolution of nf (hence, of the energy yield):
, in regime of multiplication, upon the thermonuclear specific rate R. Depending on the value of k ∞, one can distinguish three cases for the time evolution of nf (hence, of the energy yield):
-
(1)
 increases linearly with time, asymptotically to
increases linearly with time, asymptotically to
 , for
, for
 ;
; -
(2)
 increases quadratically with time; in detail,
increases quadratically with time; in detail,
 ; and
; and -
(3)
 diverges exponentially, with the growth rate
diverges exponentially, with the growth rate
 .
.
It goes without saying that the capability to achieve a chain reaction with multiplicity k ∞ higher of or comparable to 1 would play a significant—if not indispensable—role in the possible exploitation of H-11B fuel as an energy source.
The question is also how and how much a weak multiplication regime, namely when
![]() (and especially
(and especially
![]() ), can enhance the pure thermonuclear burn. Using the full expression of
), can enhance the pure thermonuclear burn. Using the full expression of
![]() , it is easy to calculate the ratio I of the suprathermal-to-thermonuclear energy yield in the confinement time τc [Reference Belloni5]; indeed, the total energy per unit volume is
, it is easy to calculate the ratio I of the suprathermal-to-thermonuclear energy yield in the confinement time τc [Reference Belloni5]; indeed, the total energy per unit volume is
![]() , the energy stemming from the sole thermonuclear burn is just
, the energy stemming from the sole thermonuclear burn is just
![]() , and the suprathermal yield is given by the difference between the first two. I is shown in Figure (4) as a function of k ∞ for several orders of magnitude of the parameter
, and the suprathermal yield is given by the difference between the first two. I is shown in Figure (4) as a function of k ∞ for several orders of magnitude of the parameter
![]() , where
, where
![]() is assumed and τα is the thermalisation time of the α particle at its most probable emission energy. Note that in typical ICF conditions, the quantity
is assumed and τα is the thermalisation time of the α particle at its most probable emission energy. Note that in typical ICF conditions, the quantity
![]() can reach the order of 103. One can distinguish the following noticeable limits for I. For
can reach the order of 103. One can distinguish the following noticeable limits for I. For
![]() and
and
![]() , I scales as k ∞. On the contrary, when k ∞ approaches 1, I tends to
, I scales as k ∞. On the contrary, when k ∞ approaches 1, I tends to
![]() , which opens the possibility of very large increments in the energy output (consequently, high fusion gains). This means that k ∞ does not have to be necessarily larger than 1 to have a sizeable enhancement of the fusion yield.
, which opens the possibility of very large increments in the energy output (consequently, high fusion gains). This means that k ∞ does not have to be necessarily larger than 1 to have a sizeable enhancement of the fusion yield.
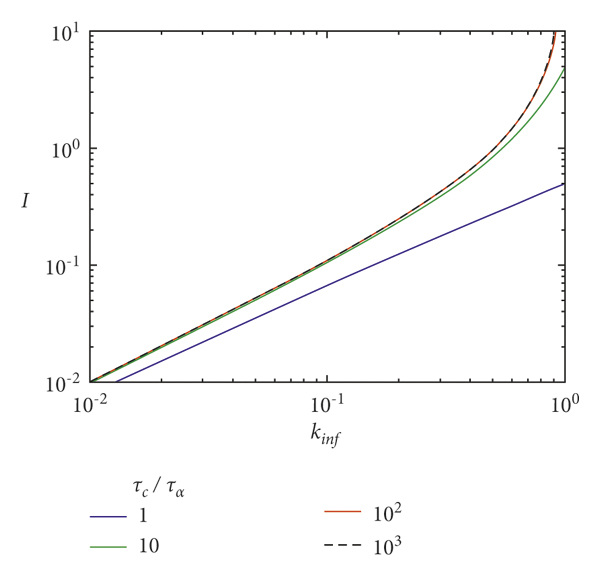
Figure 4: Suprathermal-to-thermonuclear energy ratio by the effect of a weak chain reaction. Republished from Belloni [Reference Belloni5]. © IOP Publishing Ltd. 2021.
The earliest studies were not encouraging, however. In the early 70s, Weaver et al. [Reference Weaver, Zimmerman and Wood18] estimated the increase in the H-11B reaction rate due to nonthermal effects to vary between 5% and 15% in the density range of 1016–1026 cm−3 and in the temperature range of 150–350 keV. Subsequent calculations by Moreau [Reference Moreau9, Reference Moreau19] returned multiplication factors of the order of 10−2 in a plasma with
![]() , cold ions, and Coulomb logarithm
, cold ions, and Coulomb logarithm
![]() . In both cases, however, important details have not been given; moreover, only the Coulomb interaction has been taken into account in the α-ion scattering in the case of Moreau, or poorly known nuclear data have been used for this purpose in the case of Weaver et al.
. In both cases, however, important details have not been given; moreover, only the Coulomb interaction has been taken into account in the α-ion scattering in the case of Moreau, or poorly known nuclear data have been used for this purpose in the case of Weaver et al.
The recent study of Putvinski et al. [Reference Putvinski, Ryutov and Yushmanov20] has substantially confirmed the findings of Weaver et al. [Reference Weaver, Zimmerman and Wood18]. The H-11B reactivity has been calculated using a proton spectrum, which included kinetic effects at high energy: besides α-scattering, cooling on colder electrons (
![]() ) and depletion of the spectrum tail by the fusion burn. The proton spectrum was self-consistently calculated by solving the steady-state Fokker–Planck equation upon a simple burn model. For reference parameters
) and depletion of the spectrum tail by the fusion burn. The proton spectrum was self-consistently calculated by solving the steady-state Fokker–Planck equation upon a simple burn model. For reference parameters
![]() ,
,
![]() ,
,
![]() , and
, and
![]() , the resulting reactivity showed a 10% increase compared with its purely Maxwellian form. Note that this treatment is formally independent of absolute densities as long as a fixed value is used for
, the resulting reactivity showed a 10% increase compared with its purely Maxwellian form. Note that this treatment is formally independent of absolute densities as long as a fixed value is used for
![]() (details are not given, however). Also in this case, the nuclear interaction does not appear to have been taken into account in the α-ion scattering.
(details are not given, however). Also in this case, the nuclear interaction does not appear to have been taken into account in the α-ion scattering.
Recently, a supposed experimental manifestation of the suprathermal chain reaction has been the subject of some controversies [Reference Hora, Lalousis and Giuffrida21–Reference Belloni, Margarone, Picciotto, Schillaci and Giuffrida26], which have finally been resolved in favour of the impossibility to induce this effect in plasma conditions such as those achievable at the Prague Asterix Laser System (PALS), Czech Republic [Reference Picciotto, Margarone and Velyhan27, Reference Margarone, Velyhan and Krasa28]. A later study [Reference Belloni5] has confirmed that in high-density, nondegenerate H-11B plasma, k ∞ turns out to be of the order of 10−2 at most. The domain investigated is given by
![]() ,
,
![]() ,
,
![]() , where EF is the Fermi energy;
, where EF is the Fermi energy;
![]() . This represents a low-T
i
regime, where the thermonuclear burn is very modest and is just used to seed the chain reaction; the hope was that the suprathermal chain could drive the plasma burn towards ignition, by increasing T
i
quickly.
. This represents a low-T
i
regime, where the thermonuclear burn is very modest and is just used to seed the chain reaction; the hope was that the suprathermal chain could drive the plasma burn towards ignition, by increasing T
i
quickly.
If T i is sufficiently low, one can assume that, at least for the first few generations, the suprathermal showers elicited by the α particles do not interact with each other and do not significantly affect the background (thermal) Maxwell–Boltzmann distribution of plasma ions. In a scenario of this kind, each primary α-particle or fusion event can be treated independently through a simplified model compared with more sophisticated kinetic-theory approaches, which are indispensable at high reaction rates; see, e.g., refs. [Reference Peres and Shvarts29–Reference Kumar, Ligou and Nicli31] for the case of DT fusion, and [Reference Putvinski, Ryutov and Yushmanov20] for H-11B fusion. The simplified approach of ref. [Reference Belloni5], in particular, assesses whether the medium is multiplicative or not. Without entering details, the contribution to kα of suprathermal H and 11B ions (kαp and kαB, respectively) is calculated separately, i.e.:
Denoting by j the generic ion species and by E j,0 its energy just after the scattering by an α particle, kαj is related to the scattered ion spectrum,
![]() , and the fusion probability of j, Pj, by the relation:
, and the fusion probability of j, Pj, by the relation:
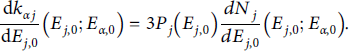
The spectrum
![]() in turn depends on the α-ion differential scattering cross section, σαj, and the α-particle stopping power,
in turn depends on the α-ion differential scattering cross section, σαj, and the α-particle stopping power,
![]() , according to the relation:
, according to the relation:
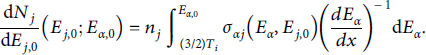
Pj
depends on σf and the stopping power of j,
![]() , in a similar fashion:
, in a similar fashion:
From ref. [Reference Belloni5], there are several points to remark and discuss. First of all, the contribution of suprathermal 11B ions to kα and k ∞ is of the order of 1% only. Nevertheless, the effect of the nuclear interaction in the α-11B scattering should be counterchecked, in the light of the elastic cross section measurements at
![]() performed by Spraker et al. [Reference Spraker, Ahmed and Blackston32]. In ref. [Reference Belloni5],
performed by Spraker et al. [Reference Spraker, Ahmed and Blackston32]. In ref. [Reference Belloni5],
![]() is calculated as the Rutherford cross section only.
is calculated as the Rutherford cross section only.
In the case of the scattered proton, the complete elastic cross section, accounting also for the nuclear interaction, must definitely be used in calculations. In Figure 5(a), kαp is plotted as a function of Eα,0 for different values of T
e
and
![]() . As a term of comparison, a curve based only on the Rutherford α-p scattering cross section, σR, is shown for T
e
= 50 keV. One can appreciate that for the most probable α-emission energy, 4 MeV, kαp is more than twice that found for a pure Coulomb scattering. At
. As a term of comparison, a curve based only on the Rutherford α-p scattering cross section, σR, is shown for T
e
= 50 keV. One can appreciate that for the most probable α-emission energy, 4 MeV, kαp is more than twice that found for a pure Coulomb scattering. At
![]() = 10 MeV, the difference reaches a factor of 8.
= 10 MeV, the difference reaches a factor of 8.
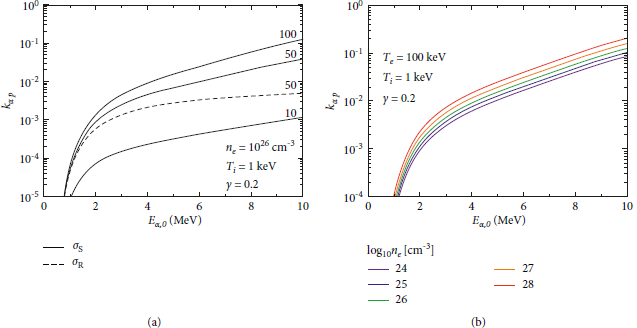
Figure 5: α-particle multiplication factor via suprathermal protons as a function of the initial α-energy, for different values of T e at a fixed n e (a), and of n e at a fixed T e (b). In (a), T e values (in keV) are indicated next to the curves; σs is the complete α-p elastic scattering cross section, accounting also for the nuclear interaction. In (b), γ is the boron-to-proton ion concentration. Republished from Belloni [Reference Belloni5]. © IOP Publishing Ltd. 2021.
A parametric analysis shows that kαp increases with both T
e
and n
e
, though it is much more sensitive to T
e
. From Figure 5(b), one notes that kαp drops quickly below
![]() , while above 4 MeV, the shape of the curves is approximately linear in the semilog plot, meaning an exponential increase with
, while above 4 MeV, the shape of the curves is approximately linear in the semilog plot, meaning an exponential increase with
![]() (up to at least 10 MeV). Even at the highest values of ne and T
e
considered (1028 cm−3 and 100 keV, respectively), kαp (hence, k ∞) remains significantly lower than 1; for instance,
(up to at least 10 MeV). Even at the highest values of ne and T
e
considered (1028 cm−3 and 100 keV, respectively), kαp (hence, k ∞) remains significantly lower than 1; for instance,
![]() for
for
![]() MeV, and
MeV, and
![]() over the actual fusion spectrum. A fit of kαp-vs-E α,0 curves with an exponential function returns a common growth rate such that kαp increases by a factor of about 2.5 each time E α,0 increases by 2 MeV. At
over the actual fusion spectrum. A fit of kαp-vs-E α,0 curves with an exponential function returns a common growth rate such that kαp increases by a factor of about 2.5 each time E α,0 increases by 2 MeV. At
![]() and Te = 100 keV, one extrapolates
and Te = 100 keV, one extrapolates
![]() for
for
![]() .
.
This means that if we could boost the energy of α’s—let us say—above 10 MeV, we could substantially increase the multiplication factor. How might this be achieved? In principle, two ways can be identified at present. One way is to use high-energy protons to trigger the fusion reaction, which can be referred to as a kinematic boost; α particles with energies up to 20 MeV have recently been generated by Bonvalet et al. [Reference Bonvalet, Nicolaï and Raffestin33] in a laser-driven pitcher-catcher experiment. Another way is to accelerate the fusion-born α’s; for instance, in the same laser-induced electric field which accelerates the protons in direct-target-irradiation experiments. Evidence of this effect has recently been reported by Giuffrida et al. [Reference Giuffrida, Belloni and Margarone34]. With either of these means, it is not obvious, however, how E α,0 could be kept so high for more than one generation. In the case of laser acceleration of the α particles, it is neither obvious how this effect, observed under irradiation of planar solid targets, could be reproduced on actual ICF targets.
It is also worth mentioning that the concept of a possible H-11B fusion reactor has been proposed (but in a low-density plasma, in this case) [Reference Eliezer and Martinez-Val35,Reference Eliezer, Schweitzer, Nissim and Martinez-Val36], which is based on α’s acceleration by the application of an external electric field to counterbalance the stopping power and induce an avalanche of reactions.
To summarise, values of k ∞ very close to 1 are needed in an ICF scheme to enhance the suprathermal-to-thermonuclear energy yield by factors of up to 103. Early computations by Weaver et al. [Reference Weaver, Zimmerman and Wood18] estimated the increase in the H-11B reaction rate due to suprathermal effects to vary only between 5% and 15% in the density range of 1016–1026 cm−3 (
![]() ) and temperature range of 150–350 keV (
) and temperature range of 150–350 keV (
![]() ). Subsequent calculations [Reference Moreau9, Reference Moreau19] returned multiplication factors of the order of 10−2 in a plasma with
). Subsequent calculations [Reference Moreau9, Reference Moreau19] returned multiplication factors of the order of 10−2 in a plasma with
![]() , Ti = 0, and
, Ti = 0, and
![]() . Recently, Putvinski et al. [Reference Putvinski, Ryutov and Yushmanov20] have substantially confirmed the findings of Weaver et al., whereas in the high-density, low-Ti domain
. Recently, Putvinski et al. [Reference Putvinski, Ryutov and Yushmanov20] have substantially confirmed the findings of Weaver et al., whereas in the high-density, low-Ti domain
![]() ,
,
![]() , and
, and
![]() (non-degenerate plasma), it has been found
(non-degenerate plasma), it has been found
![]() at most [Reference Belloni5]. This latest work has also shown that particularly for the α-p scattering, the complete elastic cross section, which includes the nuclear interaction, is needed in calculations. Furthermore, kα has been found to increase exponentially with the α-particle energy, at least in the range of 4–10 MeV, with a growth rate that is independent of ne. This exponential growth could in principle be exploited in cases where the energy of the fusion-born α’s is boosted, e.g., kinematically [Reference Bonvalet, Nicolaï and Raffestin33] or electrodynamically [Reference Giuffrida, Belloni and Margarone34]. Finally, no experimental evidence of suprathermal multiplication has been achieved so far.
at most [Reference Belloni5]. This latest work has also shown that particularly for the α-p scattering, the complete elastic cross section, which includes the nuclear interaction, is needed in calculations. Furthermore, kα has been found to increase exponentially with the α-particle energy, at least in the range of 4–10 MeV, with a growth rate that is independent of ne. This exponential growth could in principle be exploited in cases where the energy of the fusion-born α’s is boosted, e.g., kinematically [Reference Bonvalet, Nicolaï and Raffestin33] or electrodynamically [Reference Giuffrida, Belloni and Margarone34]. Finally, no experimental evidence of suprathermal multiplication has been achieved so far.
2.3. Energy Multiplication Factor in a Beam-Driven Fusion Scheme
Fast ignition may provide an option to ignite non-DT fuels inasmuch as it significantly relaxes implosion symmetry requirements (compared with central hot-spot ignition) and allows for nonspherical target configurations or fuel seeding [Reference Atzeni and Meyer-ter-Vehn37]. Among the concepts for fast ignition, it has been proposed to use intense laser-accelerated proton beams [Reference Roth, Cowan and Key38]. In this approach, the energy deposited in the precompressed fuel by a proton beam generated outside the target bootstraps the fusion flame. Ideally, between 5 and 10% of the laser pulse energy is converted into kinetic energy of the beam as the result of the interaction of the pulse with a thin foil.
Here, we wish to emphasise that proton beam fast ignition is a particularly advantageous option for the case of H-11B fuel. Indeed, while the protons transfer their energy to the plasma, additional heating is provided by in-flight fusion reactions. This is an exclusive effect of H-11B fuel as it cannot obviously occur for other proposed fusion fuels under proton irradiation. On the quantitative ground, it is useful to make recourse to the so-called energy multiplication factor, a fundamental quantity in beam fusion. It is defined as the ratio of the fusion energy produced via in-flight reactions to the overall beam energy, i.e.:
where F is the energy multiplication factor, E 0 is the initial proton energy, Q is the fusion Q-value, and Pp is given by Equation (9) as long as it is sufficiently lower than 1. Denoting by Eb the beam energy, the overall energy deposited in the fuel, Ed, is then:
An estimate of F is important to set the value of E 0 to be achieved in the laser acceleration of the protons.
A calculation of F vs E 0 for beam-driven H-11B fusion has been carried out by Moreau [Reference Moreau9]. This author considered protons injected into a 11B plasma with warm electrons and cold ions. The results are shown in Figure 6 for several values of Te. In all cases, there is a maximum at E 0 around 1 MeV; at high values of Te, other two maxima appear just below 3 MeV and between 4 and 5 MeV, respectively. It is hard, however, to achieve a multiplication factor better than 30%. Anyway, Moreau’s calculation should be redone with a more accurate fusion cross section (which was barely known at that time) and stopping power model (Sivukhin’s model [Reference Sivukhin39] was used).
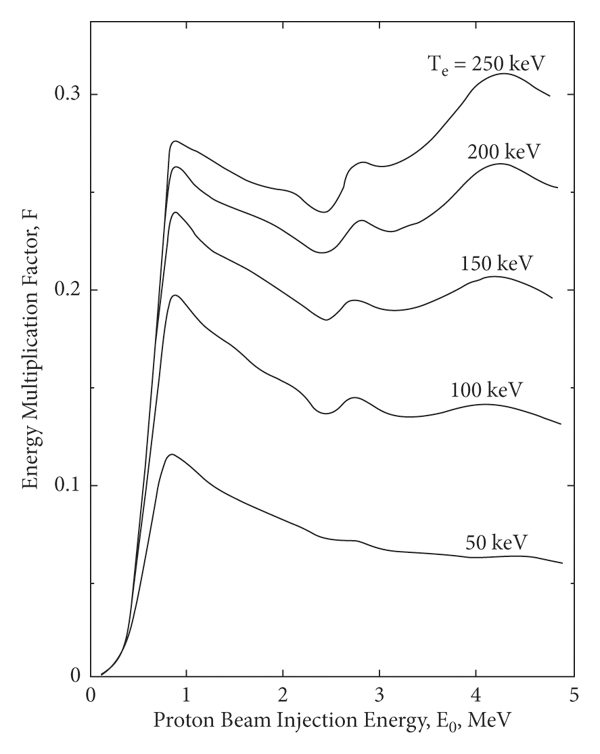
Figure 6: Energy multiplication factor vs proton injection energy for various electron temperatures. Protons are injected into a 11B plasma with cold ions and warm electrons. The results are independent of plasma density when the value of
![]() is fixed. Reproduced from Moreau [Reference Moreau9] with the permission of the publisher. © IAEA 1977.
is fixed. Reproduced from Moreau [Reference Moreau9] with the permission of the publisher. © IAEA 1977.
Note that for a Maxwellian plasma, F is formally independent of density when the value of
![]() is kept fixed. This comes from an implicit cancellation of the density in the product between nB and
is kept fixed. This comes from an implicit cancellation of the density in the product between nB and
![]() in Equation (9), with a residual density dependence holding through the expression of
in Equation (9), with a residual density dependence holding through the expression of
![]() in
in
![]() [Reference Belloni5]. This residual dependence is very weak, however. It is also worth noticing that in a fully degenerate plasma, the electronic component of
[Reference Belloni5]. This residual dependence is very weak, however. It is also worth noticing that in a fully degenerate plasma, the electronic component of
![]() would become independent of ne and proportional to the proton velocity, under certain conditions. (In general, the stopping power of an ion in a fully degenerate plasma scales roughly linearly with ne. However, when the velocity of the ion is much smaller than the Fermi velocity and the parameter
would become independent of ne and proportional to the proton velocity, under certain conditions. (In general, the stopping power of an ion in a fully degenerate plasma scales roughly linearly with ne. However, when the velocity of the ion is much smaller than the Fermi velocity and the parameter
![]() —where m is the electron mass—is much smaller than 1, the stopping power becomes independent of ne and proportional to the ion velocity. The condition
—where m is the electron mass—is much smaller than 1, the stopping power becomes independent of ne and proportional to the ion velocity. The condition
![]() holds for
holds for
![]() ) [Reference Son and Fisch17, Reference Fermi and Teller40, Reference Lindhard41]. As long as the ion-ion component of
) [Reference Son and Fisch17, Reference Fermi and Teller40, Reference Lindhard41]. As long as the ion-ion component of
![]() can be neglected, Pp would then become truly proportional to nB. This effect could boost Pp towards 1 even at low (possibly sub-MeV) values of E 0, given the linear velocity dependence of the electronic stopping power. As a consequence, F could rise up significantly, well above unity.
can be neglected, Pp would then become truly proportional to nB. This effect could boost Pp towards 1 even at low (possibly sub-MeV) values of E 0, given the linear velocity dependence of the electronic stopping power. As a consequence, F could rise up significantly, well above unity.
Another aspect to emphasise is that the energy multiplication factor can be further increased if suprathermal chain reaction effects take place. It is easy to show that in this case, Ed is augmented by a term
![]() compared with Equation (11), where the factor Sl depends on the number of generations, l, and is essentially a partial summation of the geometric series with common ratio k ∞, according to the relation:
compared with Equation (11), where the factor Sl depends on the number of generations, l, and is essentially a partial summation of the geometric series with common ratio k ∞, according to the relation:
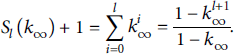
Explicitly,
where the energy multiplication factor can be redefined as follows:
Note that Sl converges to
![]() when
when
![]() and l is sufficiently large, while
and l is sufficiently large, while
![]() when
when
![]() . Now, if one was capable to keep the chain going for just two generations with k ∞ sufficiently close to 1, assuming F = 0.3, Equation (13) would return
. Now, if one was capable to keep the chain going for just two generations with k ∞ sufficiently close to 1, assuming F = 0.3, Equation (13) would return
![]() , which is quite a significant amplification. It is particularly relevant to this case what has been mentioned in the previous section, namely, that k ∞ could be made close to 1 by exploiting the kinematic boost of the proton beam.
, which is quite a significant amplification. It is particularly relevant to this case what has been mentioned in the previous section, namely, that k ∞ could be made close to 1 by exploiting the kinematic boost of the proton beam.
3. Conclusions
Recent laser-based experiments [Reference Picciotto, Margarone and Velyhan27, Reference Margarone, Velyhan and Krasa28, Reference Giuffrida, Belloni and Margarone34, Reference Labaune, Baccou and Depierreux42, Reference Baccou, Depierreux and Yahia43], basic ICF physics considerations, and current advances in laser technology suggest that a possible scheme to burn H-11B fuel is based on laser-driven proton fast ignition. By itself, this scheme will likely not be enough to achieve high gains. We are confident, however, that it can be complemented by suprathermal effects and strategies for the containment of bremsstrahlung losses in order to increase the fusion yield and relax ignition and burn requirements. In this article, we have reviewed and discussed nonthermal processes of interest, such as the progression of fusion chains via intermediate nuclear reactions, suprathermal multiplication, and beam energy amplification in proton fast ignition.
Fusion chain processes based on intermediate nuclear reactions do not show the potential to make a substantial contribution to ignition and burn of H-11B fuel. Increasing suprathermal fusion’s k ∞ above the order of 10−2 also appears problematic in present-day laser-driven plasma conditions; nevertheless, promising directions for further investigation can be drawn. In particular, the work of Belloni [Reference Belloni5] should be extended to calculate suprathermal effects.
-
(i) at higher T i , by adopting more refined kinetic approaches to the problem (e.g., steady-state spectral conditions, via the so-called Boltzmann–Fokker–Planck equation [Reference Przybylski and Ligou44]);
-
(ii) in (partially) degenerate plasmas, a regime that is also of interest for bremsstrahlung reduction [Reference Son and Fisch17, Reference Eliezer, León, Martinez-Val and Fisher45].
After Moreau [Reference Moreau9], the energy multiplication factor for a proton ignitor should be reassessed against the latest measurements of the fusion cross section [Reference Sikora and Weller6]. The energy multiplication factor should account not only for the in-flight fusion reactions but also for possible suprathermal multiplication of the fusion products. Calculations should include realistic fuel compositions and degenerate plasma regimes.
Finally, on the experimental side, it is to remark that accurate calculations of nonthermal effects, including beam fusion, need reliable fusion cross section measurements well beyond 3 MeV.
Data Availability
This is a review article. Underlying data can be found in the references.
Conflicts of Interest
The author declares that there are no conflicts of interest regarding the publication of this study.
Acknowledgments
This article is based on the content of the talk given by the author at the 29th European Fusion Programme Workshop (EFPW 2021) and of his seminar in the context of the virtual topical series HB11 Seminar, organised by HB11 Energy Holdings Pty Ltd.








