Published online by Cambridge University Press: 18 February 2010
We show that for any type III1 free Araki–Woods factor 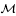 = (HR, Ut)″ associated with an orthogonal representation (Ut) of R on a separable real Hilbert space HR, the continuous core M =
= (HR, Ut)″ associated with an orthogonal representation (Ut) of R on a separable real Hilbert space HR, the continuous core M = 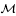 ⋊σR is a semisolid II∞ factor, i.e. for any non-zero finite projection q ∈ M, the II1 factor qM q is semisolid. If the representation (Ut) is moreover assumed to be mixing, then we prove that the core M is solid. As an application, we construct an example of a non-amenable solid II1 factor N with full fundamental group, i.e.
⋊σR is a semisolid II∞ factor, i.e. for any non-zero finite projection q ∈ M, the II1 factor qM q is semisolid. If the representation (Ut) is moreover assumed to be mixing, then we prove that the core M is solid. As an application, we construct an example of a non-amenable solid II1 factor N with full fundamental group, i.e. 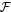 (N) = R*+, which is not isomorphic to any interpolated free group factor L(Ft), for 1 < t ≤ = +∞.
(N) = R*+, which is not isomorphic to any interpolated free group factor L(Ft), for 1 < t ≤ = +∞.