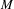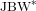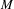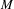Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Huang, Xujian
and
Tan, Dongni
2019.
A Tingley’s type problem in n-normed spaces.
Aequationes mathematicae,
Vol. 93,
Issue. 5,
p.
905.
Cueto-Avellaneda, María
and
Peralta, Antonio M.
2020.
Metric Characterisation of Unitaries in $$\hbox {JB}^*$$-Algebras.
Mediterranean Journal of Mathematics,
Vol. 17,
Issue. 4,
Tan, Dongni
and
Zhao, Kexin
2021.
A Property in Vector-Valued Function Spaces.
Results in Mathematics,
Vol. 76,
Issue. 1,
Kalenda, Ondřej F. K.
and
Peralta, Antonio M.
2021.
Extension of isometries from the unit sphere of a rank-2 Cartan factor.
Analysis and Mathematical Physics,
Vol. 11,
Issue. 1,
Cueto-Avellaneda, María
and
Peralta, Antonio M.
2022.
Can one identify two unital JB*-algebras by the metric spaces determined by their sets of unitaries?.
Linear and Multilinear Algebra,
Vol. 70,
Issue. 22,
p.
7702.
Peralta, Antonio
2022.
On the extension of surjective isometries whose domain is the unit sphere of a space of compact operators.
Filomat,
Vol. 36,
Issue. 9,
p.
3075.
Cabezas, David
Cueto-Avellaneda, María
Hirota, Daisuke
Miura, Takeshi
and
Peralta, Antonio M.
2022.
Every commutative JB$$^*$$-triple satisfies the complex Mazur–Ulam property.
Annals of Functional Analysis,
Vol. 13,
Issue. 4,
Moslehian, M. S.
Muñoz-Fernández, G. A.
Peralta, A. M.
and
Seoane-Sepúlveda, J. B.
2022.
Similarities and differences between real and complex Banach spaces: an overview and recent developments.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 116,
Issue. 2,
Jung, Soon-Mo
2022.
Extension of isometries in real Hilbert spaces.
Open Mathematics,
Vol. 20,
Issue. 1,
p.
1353.
Cueto-Avellaneda, María
Enami, Yuta
Hirota, Daisuke
Miura, Takeshi
and
Peralta, Antonio M.
2022.
Surjective isometries between unitary sets of unital JB⁎-algebras.
Linear Algebra and its Applications,
Vol. 643,
Issue. ,
p.
39.
Cueto-Avellaneda, María
Hirota, Daisuke
Miura, Takeshi
and
Peralta, Antonio M.
2023.
Exploring new solutions to Tingley’s problem for function algebras.
Quaestiones Mathematicae,
Vol. 46,
Issue. 7,
p.
1315.
Blecher, David P.
Neal, Matthew
Peralta, Antonio M.
and
Su, Shanshan
2025.
M-ideals, yet again: the case of real $$\hbox {JB}^*$$-triples.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 119,
Issue. 1,
Peralta, Antonio M.
and
Švarc, Radovan
2025.
A strengthened Kadison’s transitivity theorem for unital JB$$^*$$-algebras with applications to the Mazur–Ulam property.
Analysis and Mathematical Physics,
Vol. 15,
Issue. 4,